A three-DOF ultrasonic motor using four piezoelectric ceramic plates in bonded-type structure

2.1. Geometry

The structure of the proposed motor is shown in Fig. 1. The proposed motor consists of four pieces of piezoelectric ceramic plates and a metal base as shown in Fig. 1(a). It can be seen in Fig. 1(b) that the metal base is an integration of a cuboid to be attached with piezoelectric ceramic plates and a flange with eight holes to fix the motor on a rack. There is a through-hole in the metal base to tune the resonance frequencies of three vibration modes and to work as a driving foot. The polarization directions and serial numbers of the piezoelectric ceramic plates are shown in Fig. 1(c). These four pieces of piezoelectric ceramic plates are all polarized along the direction of thickness and each two parallel piezoelectric ceramic plates are polarized in same direction. Thus, the hybrid of two bending vibration modes can be excited by two driving signals. The hybrid of the longitudinal and a bending vibration mode need three driving signals.

Fig. 1The structure of the proposed motor: a) the three-dimensional model of the proposed stator, b) the metal base of the proposed motor, c) the polarizations of PZT ceramics

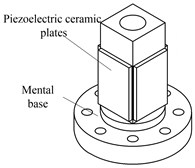
a)

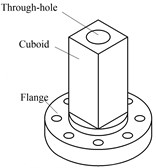
b)

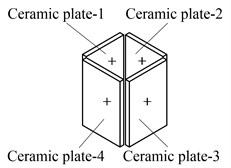
c)

2.2. Driving principle

The first order longitudinal vibration mode and two second order bending vibration modes are selected and their mode shapes are shown in Fig. 2(a), (b) and (c), respectively. The proposed motor will be excited by two or three driving signals shown in Fig. 3, with the metal base connected to ground. It can be seen from the Fig. 3 that there is a phase shift of 90 degrees between signal-1 and signal-2; the voltage change of signal-3 is converse with the one of signal-1.

Fig. 2Selected vibration modes of the proposed motor: a) the first order longitudinal vibration mode, b) the second order bending vibration mode, c) the second order bending vibration mode

a)

b)

c)

Fig. 3Driving signals of the proposed motor

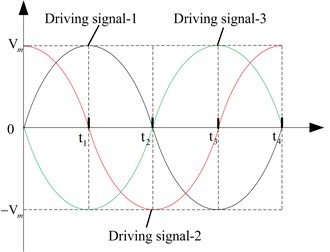
The proposed motor can realize rotation along axis-$Z$ by the hybrid of two orthogonal bending vibration modes. The driving signal-1 and driving signal-2 are applied on each two parallel pieces of piezoelectric ceramic plates, namely, the driving signal-1 is applied to ceramic plate-1, 3 and the driving signal-2 is applied to ceramic plate-2, 4, respectively. Under this circumstance, the bending vibration in plane-$YOZ$ is excited by the ceramic plate-1, 3 while the bending vibration in plane-$XOZ$ is excited by the ceramic plate-2, 4. Since there is a phase shift of 90 degrees between driving signal-1 and driving signal-2, an elliptical movement trajectory in plane-$XOY$ of the driving foot can be accomplished by the superposition of these two bending vibrations, as shown in Fig. 4.

Fig. 4Operating sequence of rotation along axis-Z: a) the vibration state of the proposed motor when t=t0 or t=t4, b) the vibration state of the proposed motor when t=t1, c) the vibration state of the proposed motor when t=t2, d) the vibration state of the proposed motor when t=t3

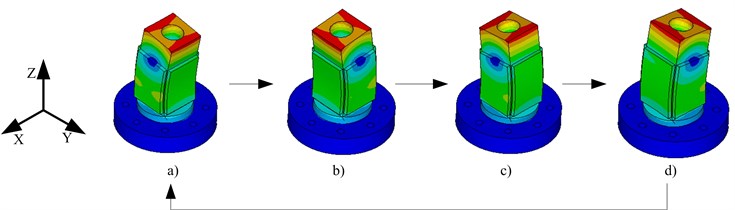
When $t=t_0$or $t=t_4$, $\left|V_1\right|=$ 0 and $\left|V_2\right|=V_m$, the deformations of ceramic plate-2, 4 reaches maximum with ceramic plate-1, 3 getting no deformation and the vibration state of the proposed motor is shown in Fig. 4(a). Then, $\left|V_1\right|$ increases and $\left|V_2\right|$ decreases. The deformations of corresponding ceramic plates are in identical tendency with the voltage changes. At the same time, the vibration state of the proposed motor changes from what is shown in Fig. 4(a) to the one shown in Fig. 4(b). The next two states can be reached in rest part of a period. Finally, the rotation along axis-$Z$ of a runner can be actuated.

The proposed motor can realize rotation along axis-$Y$ by the hybrid of the bending vibration in plane-$XOZ$ and the longitudinal vibration. The driving signal-2 is applied on the two pieces of piezoelectric ceramic plates parallel with plane-$YOZ$, namely, ceramic plate-2, 4. The driving signal-1and 3 are applied on the two pieces of piezoelectric ceramic plates parallel with plane-$XOZ$, namely, ceramic plate-1 and 3, respectively. In this condition, one of the ceramic plate-2 and 4 extends with the other shrinking; both ceramic plate-1 and 3 extend or shrink at the same time. Thus, the bending vibration in plane-$XOZ$ and the longitudinal vibration are excited. With the phase shifts between these three driving signals, the elliptical movement trajectory in plane-YOZ of the driving foot can be realized by the superposition of these two vibrations. When $t=t_0$ or $t=t_4$, $\left|V_1\right|=\left|V_3\right|=$ 0 and $\left|V_2\right|=V_m$, the deformations of ceramic plate-2 and 4 reaches maximum with ceramic plate-1 and 3 getting no deformation. The state of the proposed motor is shown as Fig. 5(a). Then, $\left|V_2\right|$ decreases, $\left|V_1\right|$ and $\left|V_3\right|$ increase. The deformations of ceramic plate-2, 4 and the degree of the bending vibration diminish. The deformations of ceramic plate-1, 3 and the degree of the longitudinal vibration are enhanced. The state of the proposed motor changes from what is shown in Fig. 5(a) to the one shown in Fig. 5(b). In the rest of a period, states shown in Fig. 5(c) and (d) will be accomplished. Finally, the rotation along axis-$Y$ of a runner can be actuated.

Fig. 5Operating sequence of rotation along axis-Y: a) the vibration state of the proposed motor when t=t0 or t=t4, b) the vibration state of the proposed motor when t=t1, c) the vibration state of the proposed motor when t=t2, d) the vibration state of the proposed motor when t=t3

In a similar way, the proposed motor can realize rotation along axis-$X$ by the hybrid of the bending vibration in plane-$YOZ$ and the longitudinal vibration. The driving signal-2 is applied on the two pieces of piezoelectric ceramic plates parallel with plane-$XOZ$, namely, ceramic plate-1 and 3. The driving signal-1 and 3 are applied on the two pieces of piezoelectric ceramic plates parallel with plane-$YOZ$, namely, ceramic plate-2 and 4, respectively.

3.1. Modal analysis

The proposed motor takes advantage of three vibration modes a longitudinal vibration mode and two orthogonal bending vibration modes. The structural parameters of the proposed motor are adjusted to tune the resonance frequencies of these vibration modes close to each other. And it can be found out that the resonance frequencies of the two bending vibration modes will keep the same with the structure symmetric in plane-$XOY$. Therefore, the main work in this part is simplified and just to tune the resonance frequency of the longitudinal vibration mode close to the one of the bending vibration mode. The resonance frequencies of the longitudinal vibration mode and the bending vibration mode are calculated to be about 40.95 kHz and 41.12 kHz, respectively, and the final dimensions are shown in Fig. 6.

3.2. Transient analysis

After establishing FEM model and accomplishing the parameter design of the proposed motor, it needs to verify the driving principle presented in second section. And the cardinal work in this part is to observe if the driving foot moves in the desired elliptical trajectory.

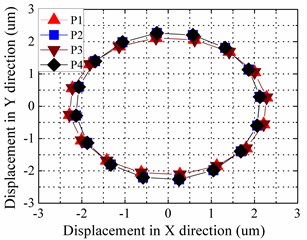
The transient analysis was executed by ANSYS. The driving signals were applied on the FEM model in the ways as shown in driving principle. The frequency and virtual voltage of the driving signals are 40.95 kHz and 100 V, respectively. There are four special points on the driving foot shown in Fig. 7 and the motion trajectories of these four points in last period of the simulation are shown in Fig. 8. The Fig. 8(a) and (b) show the motion trajectories of rotation along axis-$Z$ and axis-$Y$, respectively. Except the difference in direction, the driving principle of rotation along axis-$X$ is same with the one of rotation along axis-$Y$. Thus, the rotation along axis-$X$ needn’t to be presented.

Fig. 6Dimensions of the proposed motor (unit: mm)

a)

b)

Fig. 7Selected nodes on the driving foot

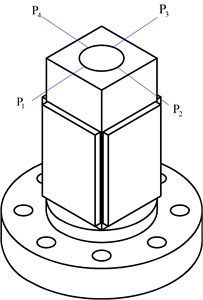
Fig. 8Motion trajectories of selected nodes in the last simulation period: a) trajectories of rotation along axis-Z, b) trajectories of rotation along axis-Y

a)

b)

It can be seen from the Fig. 8(a) that the motion trajectories of the four points are nearly circular. They aren’t overlapped because the selected points of elements in FEM model aren’t exactly at the points shown in Fig. 7. The amplitudes of the two bending vibrations are almost 2.1 um. It can be seen from the Fig. 8(b) that the trajectories of four points are elliptical. $P_2$ and $P_4$ are at the plane-$YOZ$ and long axes of their motion trajectories are parallel with axis-$X$. The long axes of the $P_1$ and $P_3$’s motion trajectories are a little inclined with the axis-$X$. The minimal amplitude is the one of the longitudinal vibration, which is about 0.72 um. According to transient analysis, the driving principle is feasible.

4.1. Vibration shape test

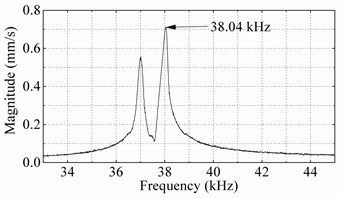
The prototype of the proposed motor used the three vibration modes presented in the second section. The longitudinal vibration mode will be excited by ceramic plate-1, 3 and ceramic plate-2, 4, respectively. Therefore, there are four vibrations need to be tested- two bending vibrations and two longitudinal vibrations. This experiment was executed by a scanning laser Doppler vibrometer (Psv-400-m2, Polytec, Germany), to obtain the actual resonance frequencies and vibration shapes. The vibration shapes and the frequency-magnitude curves of four exciting ways are shown in Fig. 10 and Fig. 11.

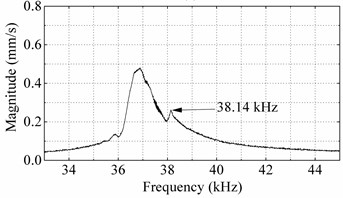
Fig. 10Bending vibration scanning results of the prototype: a) vibration velocity response spectrums under bending mode excited by ceramic-1 and ceramic-3, b) second order bending vibration shape excited by ceramic-1 and ceramic-3, c) vibration velocity response spectrums under bending mode excited by ceramic-2 and ceramic-4, d) second order bending vibration shape excited by ceramic-2 and ceramic-4

a)

b)

c)

d)

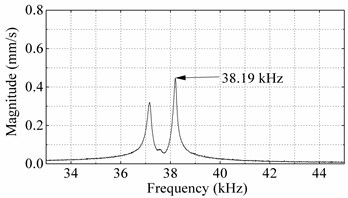
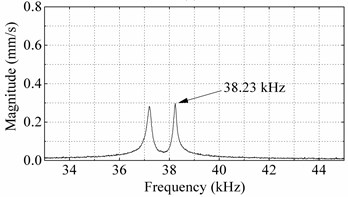
Fig. 10 shows that the practical resonance frequencies of two bending vibration modes are 38.04 kHz and 38.14 kHz, respectively, and their vibration shapes are all second order bending vibration. Fig. 11 shows that the vibration shapes of two longitudinal shapes and practical resonance frequencies are 38.19 kHz and 38.23 kHz, respectively. The resonance frequencies in this experiment are different from ones in the FEM analyses. It might result from the differences of materials and constraint condition in practice and the FEM, and also the fabricating and assembling errors. But the practical resonance frequencies of four vibrations are closed with each other and their vibration shapes are the same with the ones gained by the FEM analyses.

Fig. 11Longitudinal vibration scanning results of the prototype: a) vibration velocity response spectrums under longitudinal mode excited by ceramic-1 and ceramic-3, b) first order longitudinal vibration shape excited by ceramic-1 and ceramic-3, c) vibration velocity response spectrums under longitudinal mode excited by ceramic-2 and ceramic-4, d) first order longitudinal vibration excited by ceramic-2 and ceramic-4

a)

b)

c)

d)

4.2. Output characteristics test

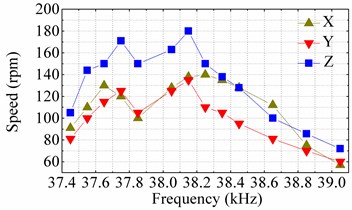
In order to obtain practical actuating capacity of the proposed motor, output velocities in three directions of the prototype were tested. Actuation of each direction was excited as the driving principle. And the runner was a steel ball with the diameter of 25 mm. Firstly, the frequency-velocity curves were obtained and shown in Fig. 12(a). The peak-to-peak amplitudes of driving signals applied were 100 V and the frequencies of the driving signals ranged from 37.45 kHz to 39.05 kHz. Although the frequency corresponding to the highest velocity of rotation along axis-$X$ is 0.1 kHz higher than the ones of the other two, the velocity of rotation along axis-$X$ corresponding to 38.15 kHz is just 2 rpm lower than the one corresponding to 38.25 kHz. Thus, the frequencies of driving signals were chosen as 38.15 kHz. With excited by these driving signals, the velocities of rotation along axis-$X$, $Y$ and $Z$ are 138 rpm, 135 rpm and 180 rpm, respectively.

Secondly, the voltage-velocity curves were obtained and shown in Fig. 12(b). The frequencies of driving signals were 38.15 kHz and the peak-to-peak amplitudes of three driving signals ranged from zero to 200 V, to observe changes of velocity. It can be seen from the Fig. 11(b) that the velocities of rotation along three directions are in positive correlation with the amplitude of the driving signals. And the minimal amplitude needed to realize rotation along axis-$X$ or $Y$ is about half of the one needed to realize the rotation along axis-$Z$. Their starting voltages are 10 Vp-p, 9 Vp-p and 22 Vp-p, respectively. But after the rotation along axis-$Z$ was excited, its velocity increased swiftly than the other two. The trends of rotation along axis-$X$ and $Y$ are similar. Under driving voltages of 200 Vp-p, the output velocities of three DOF can reach 280 rpm, 277 rpm and 327 rpm, respectively.

Fig. 12Mechanical output characteristics of the prototype: a) frequency-speed curves tested under driving signals with peak-to-peak voltages of 100 V, b) voltage-speed curves tested under driving signals with frequencies of 38.15 kHz

a)

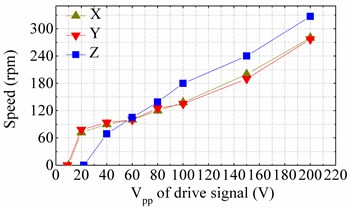
b)