Pressure ripple of gear pumps affected by air content on trapped volume

2.1. Governing equations

This paper adopt mixture model to simulate the pressure fluctuation of trapped volume through an intermeshing industrial-scale gear pump. A multiphase system is defined as a mixture of the phases of solid, liquid and gas. Multi-phase flow phenomena are typically dominated by one phase and another non-dominating phase [20]. Ignoring thermodynamical effects, two phases are assumed to move at the same velocity, the dynamics of a two-continua mixture of oil and air is governed by the following equations that model the conservation of mass and momentum [21]:

in which:

where ${\rho }_m$ is the mixture density, ${\mathbf{u}}_m$ is the mixture velocity, $p_m$ is the mixture pressure and ${\mathbf{T}}_m$ is the mixture’s viscous stress tensor. ${\alpha }_k$, ${\rho }_k$, ${\mathbf{u}}_k$, $p_k$and ${\mathbf{T}}_k$ respectively represent the volume fraction, density, velocity, pressure and stress tensor of the phase $k$. It is assumed that two phases have no slip velocity, so the convective momentum transfer between phases is equal to zero that is ${\mathbf{T}}_{dm}=0$. Considering the influence of interaction forces $\mathbf{F}$, the momentum equation of mixture Eq. (2) can be written as:

2.2. Turbulence model

As a mature turbulence model, the re-normalization group (RNG) $k$-$\epsilon$ turbulence model is suitable to simulate the complex flow in rotating and high curvature computational domain, such as turbomachines, centrifugal machines, gear machines and so on. Here, the RNG $k$-$\epsilon$ model is adopted for analyses and proposed by Yakhot et al. [22]. The turbulence kinetic energy $k$ and the dissipation rate of turbulent kinetic energy $\epsilon$ are obtained from the following transport equations:

in which:

where $u$ is the mixture velocity vector, ${\alpha }_{k1}$ and ${\alpha }_{\epsilon }$ are the inverse effective Prandtl numbers for $k$ and $\epsilon$, the effective viscosity ${\mu }_{eff}={\mu }_m+{\mu }_t$, ${\mu }_m$ is the mixture viscosity, the turbulent eddy viscosity ${\mu }_t={\rho }_m{C}_{\mu }{k}^2/\epsilon$, $G_k$ represents the generation of turbulence kinetic energy due to mean velocity gradients, $E_{ij}$ is the dependent time shear rate. The constants for the turbulent flow model have the following default values [23]: $C_{\mu }=\mathrm{}$0.0845, ${\alpha }_{k1}={\alpha }_{\epsilon }=\mathrm{}$1.39, $C_{1\epsilon }=\mathrm{}$1.42, $C_{2\epsilon }=$ 1.68, ${\eta }_0=\mathrm{}$4.38, $\beta =\mathrm{}$0.012.

3.1. External gear pump geometry

The geometry of external gear pump with an identical number of teeth on each pair of meshing gears is illustrated in Fig. 1(a). Which is simple in its operating principle, as the gears rotate the fluid is transferred from inlet port to outlet port around the outside of the gears, and the displacement of the fluid is accomplished by two meshing gears rotating against each other. When gear teeth meshing with each other, it will form a closed cavity which separates suction side and discharge side, known as the trapped volume (Fig. 1(b)). The trapped volume of fluid with rapid changes results in the generation of pressures above and below the inlet and discharge pressures, cause vibrations and noises, affecting the stationarity and stress of the gear machine. Parameters of the external gear pump are shown in Table 1.

Fig. 1a) Schematic geometry of the external gear pump and b) details of the meshing zone

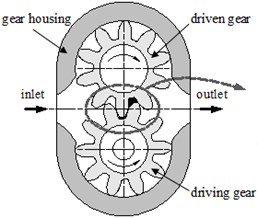
a)

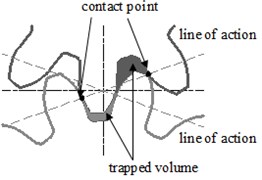
b)

3.2. Calculation methods

According to the internal flow characteristics of a rotary positive displacement pump, the three-dimensional computational model was generated in Pro/E software. The model’s grids, which were composed of an unstructured tetra/mixed, were developed using ICEM. The size of the mesh in the computational region is controlled by the octree in the ICEM. A mesh with about 1 million cells in total was chosen for the simulations. The geometrical operation of the gear pump stems from the rotation of boundaries. So, an arbitrary Lagrangian-Eulerian (ALE) formulation with smoothing and remeshing are employed to the numerical procedure.

The pressure inlet boundary conditions and the pressure outlet boundary conditions were applied at the intake region and the discharge region of the positive displacement gear pump, respectively. At the inlet boundary, the gauge total pressure is set as the atmospheric pressure. At the outlet boundary, the gauge pressure is assigned to 2.5 MPa. The specification methods of the turbulence flow in the inlet and the outlet are described by the turbulent intensity and the hydraulic diameter. The turbulent intensities of inlet and outlet have the same value of 5 %.

The gear pump is able to pump high-viscosity fluids with high efficiency. It is well known that such lubricating oils, with a large quantity of high molecular-weight polymers as the viscosity index improver added, exhibit the same behavior as pseudo-plastic fluids. In 1971, Wada and Hayashi [24] clarified the non-Newtonian characteristics of oil. In 1990, Gecim [25] used the power-law model to study the non-Newtonian effects of two oils under different temperature by the experimental. Therefore, this paper adopt power-law model [26] to simulate the pressure ripple changes of the non-Newtonian fluid in trapped volume of gear pumps. Here, the power-law parameters select of oil A in [25] that is consistency coefficient $K=\mathrm{}$0.046, and liquidity index $n=\mathrm{}$0.9111. The working fluid is the mixture fluid of air and oil. The reference pressure is 101325 Pa. No slip condition is used in the solid wall.

The time derivative is calculated with a first-order implicit scheme, since this is the only method that the software allows with dynamic mesh [16]. The SIMPLEC algorithm are used for the coupling of velocity with pressure. The gradient is calculated with the Green-Gauss cell based method and the second-order upwind discretization scheme is applied to solve turbulent kinetic energy and turbulent dissipation rate equations. The under-relaxation factors for pressure, momentum, turbulent kinetic energy, and turbulent dissipation rate are 0.2, 0.1, 0.5, and 0.5, respectively. In addition, the minimum residual for all parameters in each time step is 10^-6.

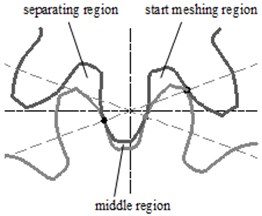
In order to calculate the pressure ripple of trapped volume under different air content, the trapped oil region is divided into three stages: start meshing region, middle region and separating region. That is shown in Fig. 2. Assuming these points $T_1$, $T_2$, $T_3$ located in the start meshing region, $T_4$, $T_5$, $T_6$ located in the middle region and $T_7$, $T_8$, $T_9$ located in the separating region.

Fig. 2Schematic of the trapped volume

4.1. The pressure ripple of trapped volume

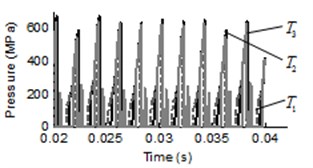
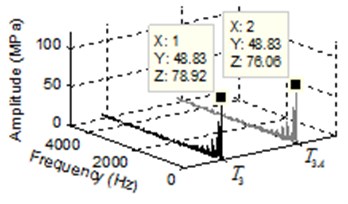
In order to estimate the influence of air dissolved in oil on pressure ripple, the air content value of 8 percent is calculated that is ${\alpha }_k=\mathrm{}$0.08 in Eq. (1-6). The pressure ripple and pressure frequency of start meshing region shown in Fig. 3. It can be seen from Fig. 3(a) that the points $T_1$, $T_2$, $T_3$ have the same pressure fluctuation cycle when the time vary between 0.02 second to 0.04 second. The rotary speed of the rotors is 3000 r/min that means the rotation period of the gear is 0.02 second, the rotational frequency of gear is 50 Hz, and the gear meshing frequency is 500 Hz. The pressure peak appears ten times during a rotation period of gear, it is equivalent to the number of teeth. The pressure fluctuation data in time domain is transformed into frequency spectrum in frequency domain with Fast Fourier Transform (FFT), and the result can be seen in Fig. 3(b). It is obvious from Fig. 3(b) that the dominant frequency of three points are all 48.83 Hz. In theory, the dominant frequency of these points are proportional to the gear meshing frequency, the true value has a certain deviation under influence of bearing rotational frequency and other factors.

Fig. 4 shows the pressure ripple and spectrum of pressure ripple in middle region of trapped volume in external gear pump. The pressure of positive displacement pump varies with time in a cycle of rotor rotation ($T=$0.02 s), as shown in Fig. 4(a), where the start time is 0.02 s. It can be seen that the pressure curves present a symmetric distribution and appear ten times pressure peak in the range of 0.02-0.04 s, where, the plus or minus sign only represents the direction. Considering the discrete points on different position gives insight onto the varying pressure field surrounding the trapped volume. The FFT of the pressure ripple is shown in Fig. 4(b), it is shown that the dominant frequency of three points $T_4$, $T_5$ and $T_6$ are 48.83 Hz, which is 0.1 times of gear meshing frequency. Where, the amplitude of $T_5$ is smaller than $T_4$ and $T_6$, because the pressure appear positive and negative values along with the time changes.

Fig. 3a) Pressure transients and b) frequency response of pressure ripple of start meshing region

a)

b)

Fig. 4a) Pressure transients and b) spectrum of pressure ripple of middle region

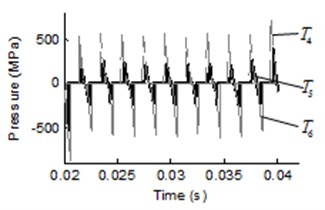
a)

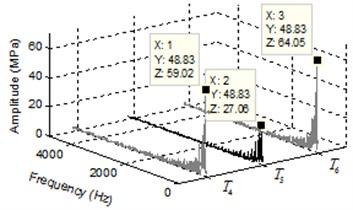
b)

Fig. 5a) Pressure transients and b) spectrum of pressure ripple of separating region

a)

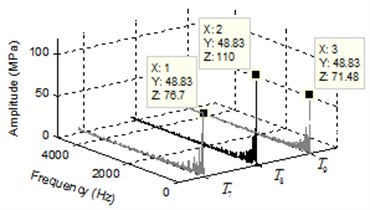
b)

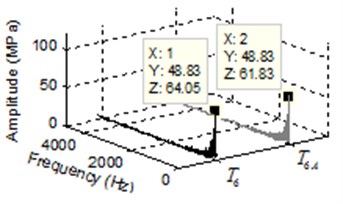
The pressure ripple and spectrum of pressure ripple in separating region of trapped volume are shown in Fig. 5. As evident form Fig. 5(a), the pressure ripple curves of points $T_7$, $T_8$ and $T_9$ represent sawtooth variation with a cycle time and have the same pressure fluctuation period. The spectrum of pressure ripple in Fig. 5(b) illustrate that the points $T_7$, $T_8$ and $T_9$ have the same dominant frequency 48.83 Hz. Comparing the Fig. 3(a), Fig. 4(a) and Fig. 5(a), it can be seen that these points in the trapped volume have the same pressure fluctuation period within the rotation period of the gear. From the Fig. 3(b), Fig. 4(b) and Fig. 5(b), the dominant frequency of these points are all 48.83 Hz that is the 1/10 times of gear meshing frequency.

4.2. The pressure ripple in different air content

In order to assess the effect degree of air content on pressure ripple, the author predict the pressure ripple in different air content on the same pump under the same operating conditions. These predictions were compared with experimental measurements in reference [19].

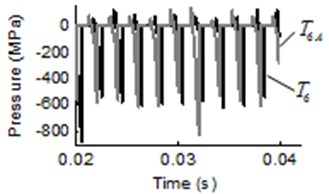
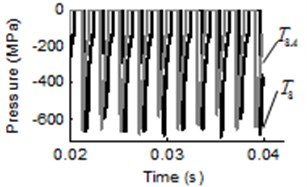
For the air content dissolved in oil and pressure ripple analysis, the pressure ripple and amplitude frequency of trapped volume under different air content are shown in Fig. 6, where the black line represent the pressure ripple with air content value of 8 percent and the gray line represent the pressure ripple with air content value of 10 percent. In Fig. 6, the lines of two cases are represented by different alphabet $T_{\mathrm{*}}$ and $T_{\mathrm{*}A}$. As can be seen from Fig. 6(a), Fig. 6(c) and Fig. 6(e), the pressure ripple at time domain with different air content did not change significantly in start meshing region, middle region and separating region. The FFT of pressure with different air content are illustrated in Fig. 6(b), Fig. 6(d) and Fig. 6(f). It is shown that the dominant frequency of all points are 48.83 Hz, this indicates that the air content has no effect on dominant frequency of trapped volume. The amplitude of pressure ripple with air content value of 10 percent is less than air content value of 8 percent, such as the amplitude of $T_{3A}<T_3$, $T_{6A}<T_6$ and $T_{8A}<T_8$, which difference value between the two cases is 2.86 MPa, 2.22 MPa and 2.2 MPa, respectively. The result is in line with the previous studies [19].

Fig. 6Pressure transients and spectrum of pressure ripple in trapped volume under different air content: a), c) and e) are pressure ripple of start meshing region, middle region and separating region, respectively, b), d) and f) are the corresponding spectrum of pressure ripple

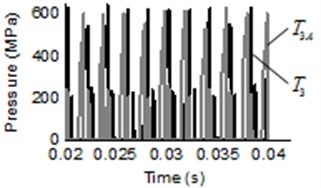
a)

b)

c)

d)

e)

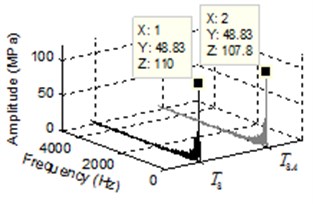
f)