Research on stress through photoelastic experiment and finite element method considering sliding wear

2.1. Photoelastic experiment

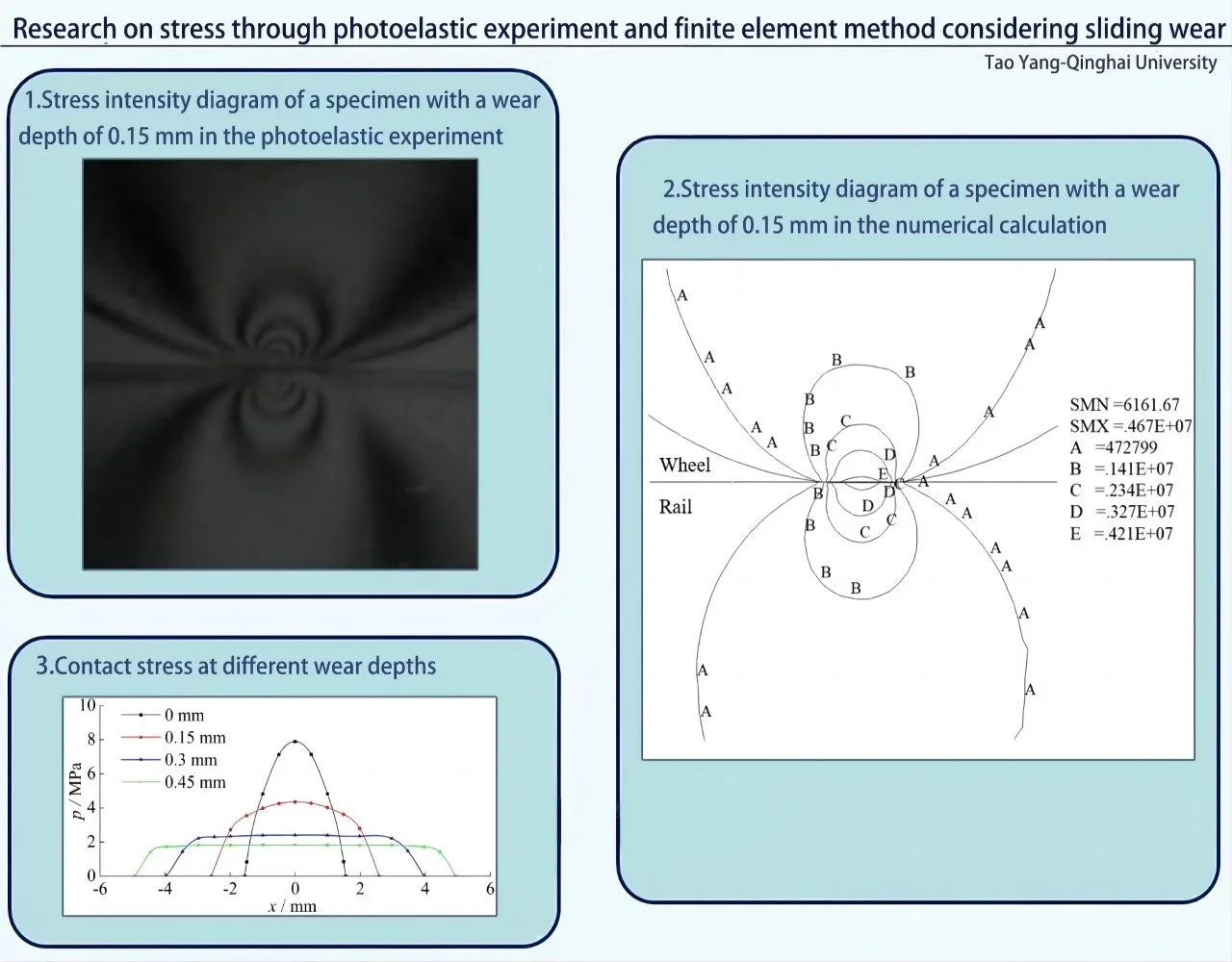
The photoelastic method is an experimental method that can provide information about the full-field stress distribution. The photoelastic streak map contains two important physical quantities, namely isobars and isotropes [22], and the photoelastic experiment can present the stress distribution law in the contact region very well [23]-[26]. Fig. 2 is the sketch of the circularly polarized light field arrangement. During the photoelastic experiment, the incident light will be decomposed into two beams of polarised light along the two principal stresses when it passes through the birefringent material. Due to the different propagation velocities of the two beams of polarised light, a phase difference of ∆ will generate when it is ejected from the specimen. The birefringence is proportional to the applied stress according to the law of planar stresses-optics. That is:

where $C$ is the material optical constant. ${\sigma }_1$ and ${\sigma }_3$ are the first and third principal stress. $h$ is the thickness of the specimen. $\lambda$ is the wavelength of the incident light.

When incident light with an intensity of $I_0$ passes through the detector mirror, the intensity of light is:

From Eqs. (1) and (2), it can be seen that two beams of polarised light with a phase difference will produce interference fringes. While there is no phase difference when the principal stress are equal, in which case dark fringes will appear. The more concentrated the stress on the specimen, the greater the change in the principal stress difference, and therefore the denser the interference fringes, and vice versa. The information from the interference fringes can be used to characterize the stress distribution in the specimen. The relationship between stress intensity and principal stresses is:

Fig. 2Sketch of circularly polarized light field arrangement

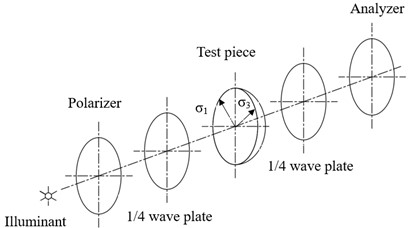
Fig. 3Sliding wear finite element model

2.2. Numerical model

In this paper, a sliding wear finite element model is built, and is shown in Fig. 3. In the model, the friction pair is simulated with PLANE182 element, and the contact behaviour is simulated with the CONTA171 and TARGE169 elements. The model has a total of 13411 elements and 13350 nodes. After trial and error, the minimum element size is set to 0.15 mm under the conditions of meeting calculation speed and accuracy. Meanwhile, the wear elements are created based on the Archard wear theory. The wear can be calculated by:

where $v$ is the amount of wear per unit area per unit time, or is called the wear rate. $k_w$ is the wear coefficient. $v_s$ is the sliding speed. $H$ is the hardness of the material. And $p\left(x,z\right)$ is the contact stress. In the finite element model, the extended Lagrangian algorithm is used to calculate the contact stress [27]. The expression is:

where $K_{con}$ is the normal contact stiffness, $u_{con}$ is the contact gap, ${\mathrm{\Omega }}_{i+1}$ is:

where $\mathrm{\Theta }$ is the intrusion tolerance, ${\mathrm{\Omega }}_{i+1}$ is the Lagrange multiplier component of the $i$-th iteration step.

2.3. Analysis parameter

In the experiment, the photoelastic specimen consists of a hollow circle and a rectangle, and they are made of epoxy resin. The inner and outer diameter of the hollow circle is 10 mm and 50 mm, respectively. The height and length of the rectangle is 50 mm and 130 mm, respectively. The thickness of the specimen is 6 mm (Fig. 4). In the finite element wear model, the dimensions of the contact pair are the same as the photoelastic specimen. The vertical displacement at the bottom of the rectangle is constrained [28]. The modulus and Poisson’s ratio of the material is 3 GPa and 0.38, respectively, and the density is 1200 kg/m³. The wear coefficient is 4×10^-4 [29], and the hardness is 85 HD. The vertical load applied on the top of the hollow circle is the same as that of the photoelastic experiment, both are 100 N. The sliding speed is 1 m/s.

Fig. 4Photoelastic experiment

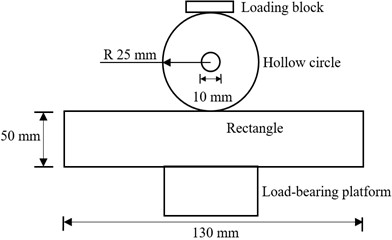
a) Schematic diagram of photoelastic experiment

b) Hollow circle

c) Rectangle

3.1. Experiment results

The stress intensity at different wear depths are illustrated as Fig. 5. Fig. 5(a) shows the photoelastic stripes are symmetrically distributed, with a closed-curve distribution at the position of the contact centre. And the number of stripes is the most intensive, in other words the stress is very concentrated. Fig. 5(a) also shows the stripe gap gradually increases from the contact centre outwards, and it indicates the stress in the contact centre is the highest, and decreases gradually from the contact centre outwards. Fig. 5(b), Fig. 5(c) and Fig. 5(d) show that as the depth of wear increases, the number of stripes near the contact surface decreases, and the gap between the stripes increases.

Fig. 5Stress intensity diagram at different wear depths

a) Unworn (0 mm )

b) 0.15 mm

c) 0.3 mm

d) 0.45 mm

3.2. Finite element calculation results

Stress intensity contours obtained by finite element model are shown as Fig. 6. The contact half-widths, contact stress and von Mises stress at the contact centre under the condition of different wear depths are respectively shown as Fig. 7, Fig. 8 and Fig. 9.

Fig. 6Stress intensity contour maps at different wear depths (Pa)

a) Unworn (0 mm)

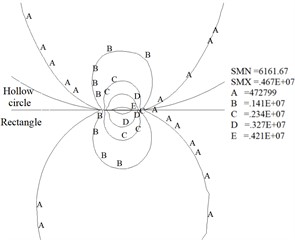
b) 0.15 mm

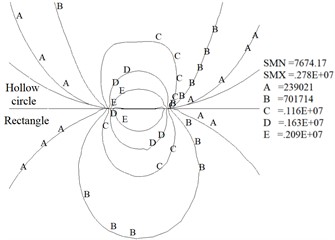
c) 0.3 mm

d) 0.45 mm

Fig. 6 shows the maximum stress intensity for the four cases of wear depth of 0 mm, 0.15 mm, 0.3 mm and 0.45 mm is 8.65 MPa, 4.67 MPa, 2.78 MPa and 2.3 MPa, respectively. The results indicate the maximum value of stress intensity decreases rapidly with the growth of the wear depth. Fig. 6 also shows the shape of the contour line near the contact surface is gradually flat with the increase of wear depth. Meanwhile, the results show the stress distribution laws obtained from numerical calculation are consistent with the experimental results (Fig. 5 and Fig. 6).

Fig. 7Contact half-width at different wear depths

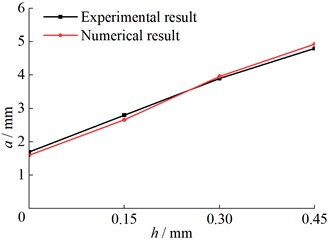
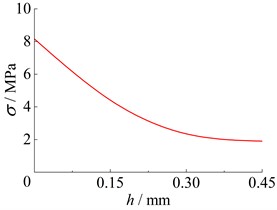
Fig. 7 shows with the growth of wear depth ($h$), the contact half-width ($a$) on the contact surface gradually increases. Moreover, Fig. 7 shows the results obtained from the finite element wear model fit well with the experimental results. Fig. 8 shows as the depth of wear increases, the contact stress on the contact surface is gradually transformed from the initial sharp convex distribution to a flat shape, the contact size is gradually increasing, and the maximum contact stress is gradually decreasing. In other words, the distribution of contact stress gradually becomes uniform. Fig. 9 shows the von Mises stress in the contact centre descends with the increase of the wear depth. And when the wear depth is more than 0.3 mm, the influence of wear on the von Mises stress is small.

Fig. 8Contact stress at different wear depths

Fig. 9Von Mises stress at contact centre