Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter

Korendiy, Vitaliy; Parashchyn, Oleh; Heletiy, Volodymyr; Pasika, Viacheslav; Gurey, Volodymyr; Maherus, Nadiia

doi:10.21595/vp.2023.23728

Vibroengineering Procedia

Browse Procedia

Published: 25 November 2023

Check for updates

Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter

Vitaliy Korendiy¹

Oleh Parashchyn²

Volodymyr Heletiy³

Viacheslav Pasika⁴

Volodymyr Gurey⁵

Nadiia Maherus⁶

^{1, 2, 3, 4}Department of Technical Mechanics and Engineering Graphics, Lviv Polytechnic National University, Lviv, Ukraine

^{5, 6}Department of Robotics and Integrated Mechanical Engineering Technologies, Lviv Polytechnic National University, Lviv, Ukraine

Corresponding Author:

Vitaliy Korendiy

Cite the article Download PDF

Downloads 152

CrossRef Citations 1

Abstract

Vibration exciters are of most important units of any vibratory equipment defining its design peculiarities, operational features, functional purpose, and performance characteristics. Among a great variety of vibration exciters, the unbalanced rotors are of the most widely used. The present research considers the possibilities of providing specific motion trajectories of the vibratory machines’ working members with the help of the planetary-type vibration exciter. The methodology of this study is divided into two main stages: deriving motion equations of an unbalanced mass located on a planet gear and analyzing the possibilities of generating rectilinear, elliptical, and circular motion paths by choosing the appropriate geometrical parameters of the planetary gear train. The results of the performed kinematic analysis are presented in the form of the unbalanced mass trajectories, velocities, and accelerations at different design parameters of the planetary-type mechanism. The main scientific novelty of the present study is substantiating the possibilities of using the single-degree-of-freedom planetary-type mechanism for generating the controllable motion trajectories of the unbalanced mass of an inertial vibration exciter. The obtained results can be practically implemented while developing novel adjustable drives for various vibratory equipment, particularly compactors, sieves, screens, and conveyors.

Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter

Highlights

In an improved excitation mechanism, it is proposed to attach an unbalanced mass to the planet gear and to define the appropriate design parameters providing specific trajectories of the mass motion.
The kinematic diagram of the mechanism is considered, and analytical expressions for calculating kinematic parameters (displacements, speeds, and accelerations) of the unbalanced mass are derived.
The possibilities of generating circular, elliptical, and rectilinear motion of the unbalanced mass are substantiated. In addition, the speeds and accelerations of the unbalanced mass are analyzed.

1. Introduction

Compacting, sieving, screening, and conveying machines are of the most widespread vibratory equipment used in various industries, e.g., machine-building, mining, food-processing, pharmaceutical, agriculture, etc. In order to provide the best performance of the mentioned operations while processing different products, the used vibration exciter should be able to adjust its inertial characteristics according to the prescribed technological conditions [1]. In addition, the design peculiarities of the exciter influence the startup duration and energy consumption of the vibratory equipment [2], as well as the kinematic and dynamic characteristics of the working member (trajectory, velocity, acceleration, overloading, etc.) [3]. Considering the prospective fields of application of the controllable vibration exciters, they can be effectively implemented for such purposes as vibration-driven locomotion systems [4] and wheeled vibratory robots [5].

Numerous scientific papers are dedicated to the development and investigation of adjustable vibration exciters. The possibilities of equipping the vibratory machines with multi-motor drives actuating several unbalanced rotors that work synchronously are comprehensively studied in [6] and [7]. An enhanced design of the coaxial dual-rotor inertial vibration exciter is presented, theoretically investigated, and experimentally tested in [8]. The use of the controllable crank-type mechanism for driving various vibratory equipment is considered in [9], while the possibilities of its implementation for actuating the semidefinite oscillatory system are studied in [10].

From the scientific and practical viewpoint, the interesting and prospective designs of inertial vibration exciters can be developed on the basis of planetary-type mechanisms. For instance, the papers [11] and [12] consider the implementation of such exciters for actuating various construction and road-building machinery, snowplows, etc. The possibilities of developing multi-purpose adjustable planetary-type vibration exciters are thoroughly studied in [13, 14]. The paper [15] is focused on investigating the resonance effects that occur during the operation of a vibratory system actuated by the planetary-type exciter. In [16], the authors consider the torsional oscillations of a planetary gear train. The present investigations continue the authors’ previous research published in [17] and dedicated to generating triangular, rectangular, and similar multi-angular oscillations of a single-mass vibratory system driven by the planetary-type exciter. The main scientific novelty of the present study is substantiating the possibilities of using the single-degree-of-freedom planetary-type mechanism for generating the controllable motion trajectories (circular, elliptical, and rectilinear) of the unbalanced mass of an inertial vibration exciter.

2. Research methodology

2.1. General design of the laboratory prototype being studied

The laboratory prototype of the single-mass vibratory system (Fig. 1) consists of the planetary-type mechanism 1 driven by the electric motor 2 through the toothed belt transmission 3. The driving pulley 4 is fixed on the motor’s shaft. The ring gear 5 of the mechanism is mounted on the machine’s body 6 suspended from the stationary supporting surface 7 by the set of four coil-type cylindrical springs 8, which perform the vibration isolation function. The arm or, so-called, planet carrier 9 is fixed to the shaft of the driven pulley 10 of the toothed belt transmission 3. Another end of the carrier 9 is hinged to the axle, on which the planet gear 11 is installed. The unbalanced mass 12 is to be connected to the planet gear 11 at different points, and the dependence of the connection point location on the mass 12 trajectory is to be studied in the present paper. The major idea of this research is choosing the appropriate design (geometrical) parameters of the planetary gear train that allow for generating circular, elliptical, and rectilinear motion trajectories of the unbalanced mass. This, in turn, could provide the corresponding vibrations of the machine’s body.

Fig. 1General design of the laboratory prototype being studied

2.2. Kinematic diagram of the planetary-type mechanism of the vibration exciter

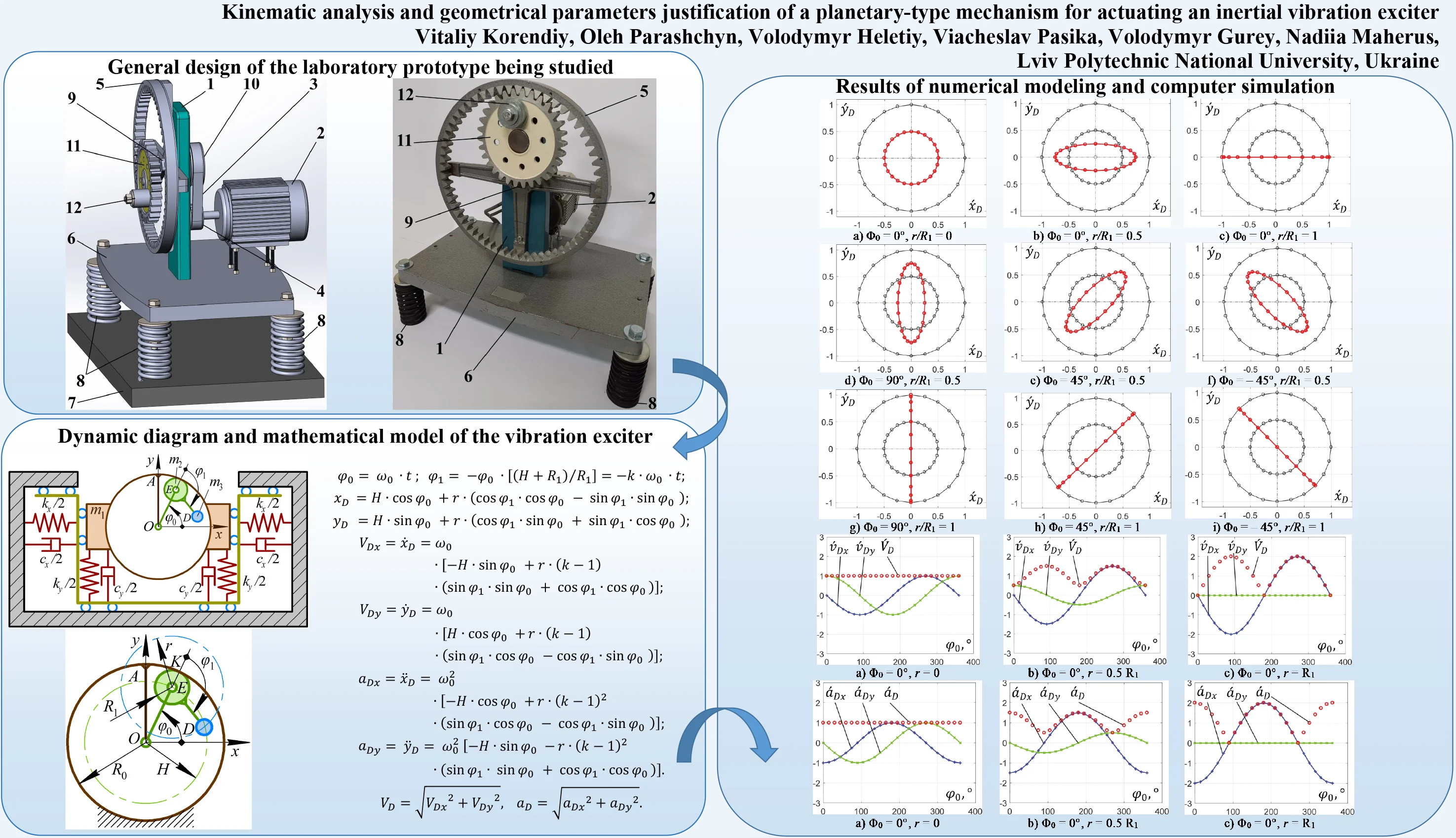
The dynamic diagram of the single-mass vibratory system equipped with the planetary-type vibration exciter is presented in Fig. 2(a). The rigid body of the mass $m_{1}$ can perform planar oscillations along the horizontal ( $O x$ ) and vertical ( $O y$ ) axes and is suspended from the stationary base by a set of spring-damper elements. The latter are characterized by the stiffness coefficients $k_{x}$ , $k_{y}$ and viscous friction factors $c_{x}$ , $c_{y}$ . The system motion is generated due to the action of the inertial forces exerted on the planet gear (mass $m_{2}$ ) and unbalanced mass $m_{3}$ during their uniform rotation around two mutually perpendicular axes located at hinges $O$ and $E$ .

Taking into account the main idea of this research mentioned in the previous section, let us consider the corresponding kinematic diagram of the planetary-type mechanism used for actuating the proposed inertial vibration exciter (see Fig. 1(b)). The ring gear of the planetary-type mechanism is supposed to be fixed, while the rotation of the arm (planet carrier) $O E$ is characterized by the angular coordinate $φ_{0}$ . Considering the geometrical parameters of the planetary-type mechanism ( $R_{0} = O K$ , $R_{1} = E K$ , $H = O E$ , $r = E D$ ) and assuming the constant angular speed of the arm $ω_{0}$ , let us define the basic relations between the angles $φ_{0}$ and $φ_{1}$ :

1

φ_{0} = ω_{0} ∙ t, φ_{1} = - φ_{0} ∙ [(H + R_{1}) / R_{1}] = - k ∙ ω_{0} ∙ t,

where $k = (H + R_{1}) / R_{1}$ .

Fig. 2a) Dynamic diagram of the oscillatory system and b) kinematic diagram of the planetary-type mechanism of the vibration exciter

a)

b)

2.3. Analytical dependencies for determining the kinematic parameters of the mechanism

Considering the coordinate system $x O y$ with its origin at point $O$ , let us derive the analytical expression for determining the horizontal and vertical position of the unbalanced mass $D$ :

2

x_{D} = H ∙ \cos φ_{0} + r ∙ (\cos φ_{1} ∙ \cos φ_{0} - \sin φ_{1} ∙ \sin φ_{0}),

3

y_{D} = H ∙ \sin φ_{0} + r ∙ (\cos φ_{1} ∙ \sin φ_{0} + \sin φ_{1} ∙ \cos φ_{0}) .

Based on Eqs. (2) and (3), the corresponding formulas for calculating the horizontal and vertical velocities and accelerations of the unbalanced mass can be deduced:

4

V_{D x} = {\dot{x}}_{D} = ω_{0} ∙ [- H ∙ \sin φ_{0} + r ∙ (k - 1) ∙ (\sin φ_{1} ∙ \sin φ_{0} + \cos φ_{1} ∙ \cos φ_{0})],

5

V_{D y} = {\dot{y}}_{D} {= ω}_{0} ∙ [H ∙ \cos φ_{0} + r ∙ (k - 1) ∙ (\sin φ_{1} ∙ \cos φ_{0} - \cos φ_{1} ∙ \sin φ_{0})],

6

a_{D x} = {\ddot{x}}_{D} = ω_{0}^{2} ∙ [- H ∙ \cos φ_{0} + r ∙ {(k - 1)}^{2} ∙ (\sin φ_{1} ∙ \cos φ_{0} - \cos φ_{1} ∙ \sin φ_{0})],

7

a_{D y} = {\ddot{y}}_{D} = ω_{0}^{2} [- H ∙ \sin φ_{0} - r ∙ {(k - 1)}^{2} ∙ (\sin φ_{1} ∙ \sin φ_{0} + \cos φ_{1} ∙ \cos φ_{0})] .

The full speed and acceleration of the unbalanced mass are following:

8

V_{D} = \sqrt{{V_{D x}}^{2} + {V_{D y}}^{2}}, a_{D} = \sqrt{{a_{D x}}^{2} + {a_{D y}}^{2}} .

While performing kinematic analysis, it is more informative to consider the dimensionless analogues of the displacements, speeds, and accelerations instead of their absolute values:

9

{\overset{´}{x}}_{D} = \frac{x_{D}}{R_{0}}, {\overset{´}{y}}_{D} = \frac{y_{D}}{R_{0}},

10

{\overset{´}{v}}_{D x} = \frac{V_{D x}}{(ω_{0} ∙ H)}, {\overset{´}{v}}_{D y} = \frac{V_{D y}}{(ω_{0} ∙ H)},

11

{\overset{´}{a}}_{D x} = \frac{a_{D x}}{(ω_{0}^{2} ∙ H)}, {\overset{´}{a}}_{D y} = \frac{a_{D y}}{(ω_{0}^{2} ∙ H)} .

3. Results and discussion

3.1. Simulating the trajectories of the unbalanced mass motion

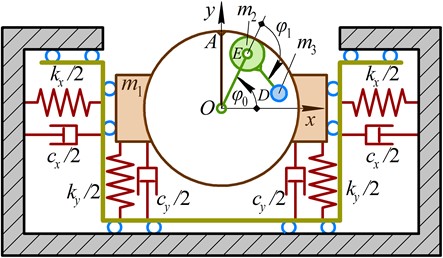
During the rotation of an arm (planet carrier) at a constant angular speed, it is enough to consider its single revolution to plot the corresponding trajectories of the unbalanced mass in the dimensionless values (see Eq. (9)). The results (Fig. 3) were obtained for the following constant relations between the mechanism geometrical parameters: $H / R_{0} =$ 0.5 and $R_{1} / R_{0} =$ 0.5, whilst the ratio $r / R_{1}$ and the initial angle of the arm $φ_{0}$ ( $t =$ 0, $φ_{1} =$ 0) $= Ф_{0}$ are considered changeable.

Fig. 3Dimensionless trajectories of the unbalanced mass motion

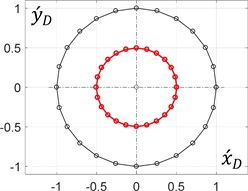
a) $Φ_{0} =$ 0°, $r / R_{1} =$ 0

b) $Φ_{0} =$ 0°, $r / R_{1} =$ 0.5

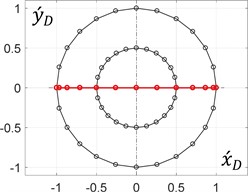
c) $Φ_{0} =$ 0°, $r / R_{1} =$ 1

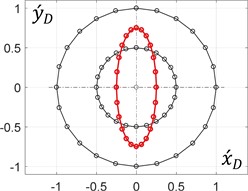
d) $Φ_{0} =$ 90°, $r / R_{1} =$ 0.5

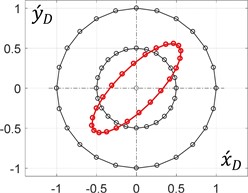
e) $Φ_{0} =$ 45°, $r / R_{1} =$ 0.5

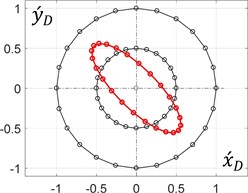
f) $Φ_{0} =$ – 45°, $r / R_{1} =$ 0.5

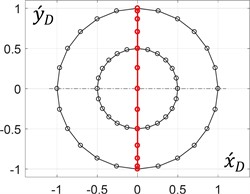
g) $Φ_{0} =$ 90°, $r / R_{1} =$ 1

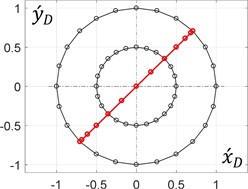
h) $Φ_{0} =$ 45°, $r / R_{1} =$ 1

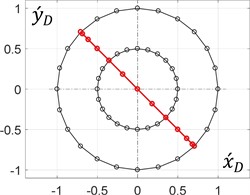
i) $Φ_{0} =$ – 45°, $r / R_{1} =$ 1

The obtained results allow for the conclusion that the circular trajectory of the unbalanced mass can be obtained when the distance between the mass and the planet gear axis is equal to zero ( $r / R_{1}$ = 0). All the other ratios $r / R_{1}$ within the range of (0…1) provide elliptical paths, whose major axes make the angles $Φ_{0}$ with the $O x$ axis. Similarly, the ratio $r / R_{1}$ = 1 allows for generating rectilinear motion of the unbalanced mass at different angles $Φ_{0}$ with the horizontal axis.

Considering the possible practical implementation of the obtained results, the following conclusions can be drawn. Vertical rectilinear and elliptical trajectories of the unbalanced mass and, as a consequence, of the working member can be used in vibratory compacting machines. Horizontal rectilinear and circular paths can be implemented in screening and sieving equipment. In addition, a circular trajectory of a working member should be provided in vibratory lapping and polishing machines. Inclined rectilinear and elliptical trajectories are preferable for conveying equipment. Various rectilinear paths can be used in vibration-driven locomotion systems.

3.2. Analyzing the speeds and accelerations of the unbalanced mass

In order to perform further analysis, let us consider three basic cases of the unbalanced mass motion (see Figs. 3(a)-(c)): $H / R_{0} =$ 0.5, $R_{1} / R_{0} =$ 0.5, $Φ_{0} =$ 0°, at the following changeable ratios $r / R_{1} =$ 0, 0.5, 1. The plots describing the dependencies of the analogues of speeds and accelerations of the unbalanced mass at different ratios $r / R_{1}$ are presented in Figs. 4 and 5. Under the conditions of $r =$ 0, the full speed of the unbalanced mass is equal to the speed of the planet gear hinge, while the corresponding horizontal and vertical components periodically reach the maximal values with the phase shift of 90°. Similarly, the full acceleration is equal to the centrifugal acceleration of the unbalanced mass rotating around the crankshaft. The elliptical motion characterized by the horizontal position of the ellipse major axis is also accompanied by the periodical change in the horizontal and vertical speeds and accelerations. In this case, the horizontal quantities take larger peak values than the vertical ones. Considering the rectilinear motion, the vertical components of the speeds and accelerations are equal to zero, while the full speed and acceleration are equal to the horizontal ones and periodically change reaching the maximal values that are twice larger than the ones obtained under the conditions of circular motion. This substantiates the possibilities of implementing the considered planetary-type mechanism with an unbalanced mass for actuating the inertial vibration exciter, which, in turn, can be used for actuating various vibratory equipment, e.g., screens, conveyors, compactors, etc.

Fig. 4Dependencies of analogues of the unbalanced mass speeds on the rotation angle

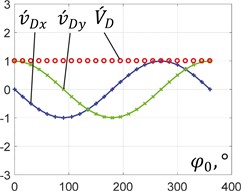
a) $Φ_{0} =$ 0°, $r =$ 0

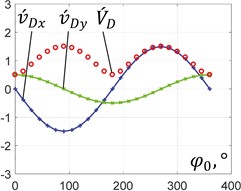
b) $Φ_{0} =$ 0°, $r = 0.5 R_{1}$

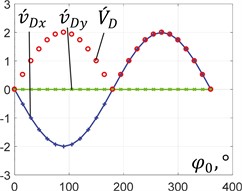
c) $Φ_{0} =$ 0°, $r = R_{1}$

Fig. 5Dependencies of analogues of the unbalanced mass accelerations on the rotation angle

a) $Φ_{0} =$ 0°, $r =$ 0

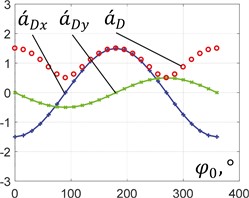
b) $Φ_{0} =$ 0°, $r = 0.5 R_{1}$

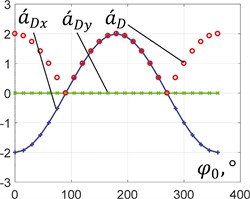
c) $Φ_{0} =$ 0°, $r = R_{1}$

4. Conclusions

The paper considers the possibilities of implementing the planetary-type mechanism for actuating the inertial vibration exciter with the aim of generating circular, elliptical, and rectilinear oscillations of the single-mass vibratory system. It is proposed to attach an unbalanced mass to the planet gear and to define the appropriate design (geometrical) parameters of the mechanism providing the mentioned trajectories of the unbalanced mass motion. The corresponding kinematic diagram of the mechanism is considered, and the analytical expressions for calculating the basic kinematic parameters (horizontal and vertical displacements, speeds, and accelerations) of the unbalanced mass are derived. The trajectories of the unbalanced mass are plotted at different geometrical parameters of the planetary-type mechanism. The possibilities of generating circular, elliptical, and rectilinear motion of the unbalanced mass are substantiated. In addition, the speeds and accelerations of the unbalanced mass are analyzed for the case when the arm (planet carrier) is initially located in the horizontal position. The obtained results can be used while developing novel adjustable drives for various vibratory equipment. Particularly, vertical rectilinear and elliptical trajectories can be used in vibratory compactors; horizontal rectilinear and circular paths can be implemented in sieves and screens; inclined rectilinear and elliptical trajectories can be used in vibratory conveyors. Further investigations on the subject of the paper may be focused on studying the dynamic behavior of various vibratory systems equipped with the considered exciter.

References

S. Kilikevičius, K. Liutkauskienė, R. Česnavičius, A. Keršys, and R. Makaras, “Investigation of the motion characteristics of parts on a platform subjected to planar oscillations,” Applied Sciences, Vol. 13, No. 17, p. 9576, Aug. 2023, https://doi.org/10.3390/app13179576

Publisher
J. Feliks and P. Tomach, “The impact of vibrating screen startup time on vibration amplitude and energy consumption in transient state,” Energies, Vol. 16, No. 20, p. 7129, Oct. 2023, https://doi.org/10.3390/en16207129

Publisher
O. Kachur and V. Korendiy, “Dynamic behavior of vibratory screening conveyor equipped with crank-type exciter,” in Lecture Notes in Mechanical Engineering, pp. 44–53, 2023, https://doi.org/10.1007/978-3-031-32774-2_5

Publisher
V. Korendiy, V. Gursky, O. Kachur, P. Dmyterko, O. Kotsiumbas, and O. Havrylchenko, “Mathematical model and motion analysis of a wheeled vibro-impact locomotion system,” Vibroengineering Procedia, Vol. 41, pp. 77–83, Apr. 2022, https://doi.org/10.21595/vp.2022.22422

Publisher
V. Korendiy and O. Kachur, “Locomotion characteristics of a wheeled vibration-driven robot with an enhanced pantograph-type suspension,” Frontiers in Robotics and AI, Vol. 10, p. 1239137, Aug. 2023, https://doi.org/10.3389/frobt.2023.1239137

Publisher
M. Zou, P. Fang, Y. Hou, and H. Peng, “Investigation on multiple-frequency synchronization experiment of vibration system with dual-rotor actuation,” Mechanical Systems and Signal Processing, Vol. 164, p. 108261, Feb. 2022, https://doi.org/10.1016/j.ymssp.2021.108261

Publisher
Y. Jia, N. Zhang, and Z. An, “Self-synchronization theory of circular symmetrical four motors,” Journal of Physics: Conference Series, Vol. 2174, No. 1, p. 012078, Jan. 2022, https://doi.org/10.1088/1742-6596/2174/1/012078

Publisher
V. Gurskyi, V. Korendiy, P. Krot, R. Zimroz, O. Kachur, and N. Maherus, “On the dynamics of an enhanced coaxial inertial exciter for vibratory machines,” Machines, Vol. 11, No. 1, p. 97, Jan. 2023, https://doi.org/10.3390/machines11010097

Publisher
O. Lanets, O. Kachur, V. Korendiy, and V. Lozynskyy, “Controllable crank mechanism for exciting oscillations of vibratory equipment,” in Lecture Notes in Mechanical Engineering, Cham: Springer International Publishing, 2021, pp. 43–52, https://doi.org/10.1007/978-3-030-77823-1_5

Publisher
V. Korendiy, V. Gursky, O. Kachur, V. Gurey, O. Havrylchenko, and O. Kotsiumbas, “Mathematical modeling of forced oscillations of semidefinite vibro-impact system sliding along rough horizontal surface,” Vibroengineering PROCEDIA, Vol. 39, pp. 164–169, Dec. 2021, https://doi.org/10.21595/vp.2021.22298

Publisher
A. Kim, M. Doudkin, A. Ermilov, G. Kustarev, M. Sakimov, and M. Mlynczak, “Analysis of vibroexciters working process of the improved efficiency for ice breaking, construction and road machines,” Journal of Vibroengineering, Vol. 22, No. 3, pp. 465–485, May 2020, https://doi.org/10.21595/jve.2020.20446

Publisher
M. Marek, G. Kustarev, and N. Andrjuchov, “Theoretical studies and conditions analysis of the inertial slider slippage of asymmetric planetary vibration exciter for snowplow,” Bulletin of D. Serikbayev EKTU, No. 3, pp. 63–78, 2021, https://doi.org/10.51885/1561-4212_2021_3_63

Publisher
V. V. Mikheyev and S. V. Saveliev, “Planetary adjustable vibratory exciter with chain gear,” in Journal of Physics: Conference Series, Vol. 1210, No. 1, p. 012097, Mar. 2019, https://doi.org/10.1088/1742-6596/1210/1/012097

Publisher
V. V. Mikheyev, “New type of vibration generator with vibratory force oriented in preferred direction,” Journal of Vibration Engineering and Technologies, Vol. 6, No. 2, pp. 149–154, Apr. 2018, https://doi.org/10.1007/s42417-018-0025-4

Publisher
R. Palevicius and K. Ragulskis, “The self-resonance effect of the planetary vibration excitation systems,” Journal of Vibroengineering, Vol. 14, No. 1, pp. 244–249, Mar. 2012.

Search CrossRef
G. Huo et al., “Torsional vibration modelling of a two-stage closed differential planetary gear train,” in Proceedings of the 11th IFToMM International Conference on Rotordynamics, Vol. 140, pp. 188–201, 2024, https://doi.org/10.1007/978-3-031-40459-7_13

Publisher
V. Korendiy, V. Gurey, V. Borovets, O. Kotsiumbas, and V. Lozynskyy, “Generating various motion paths of single-mass vibratory system equipped with symmetric planetary-type vibration exciter,” Vibroengineering Procedia, Vol. 43, pp. 7–13, Jun. 2022, https://doi.org/10.21595/vp.2022.22703

Publisher

Cited by

Force analysis of the planetary-type mechanisms of the enhanced vibration exciters

(2024)

About this article

Received

24 October 2023

Accepted

06 November 2023

Published

25 November 2023

SUBJECTS

Mechanical vibrations and applications

DOI

https://doi.org/10.21595/vp.2023.23728

Keywords

vibratory equipment

design parameters

unbalanced rotor

planetary-type vibration exciter

planet gear

motion equation

motion trajectory

single-degree-of-freedom mechanism

Acknowledgements

The authors have not disclosed any funding.

Data Availability

The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request.

Conflict of interest

The authors declare that they have no conflict of interest.

This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Previous article in issue Previous Next article in issue Next

Research article

2024 10 18

Trajectory-based synthesis of a slider-crank mechanism for applications in inertial vibration exciters

Vitaliy Korendiy, Taras Vilchynskyi, Vasyl Lozynskyy, Roman Kachmar, Yurii Porokhovskyi, Roman Litvin

Research article

2024 04 04

Force analysis of the planetary-type mechanisms of the enhanced vibration exciters

Vitaliy Korendiy, Oleh Parashchyn, Andrew Stetsko, Roman Litvin, Oleh Kotsiumbas, Roman Pelo

Research article

2023 10 20

Generating rectilinear, elliptical, and circular oscillations of a single-mass vibratory system equipped with an enhanced twin crank-type exciter

Vitaliy Korendiy, Oleksandr Kachur, Rostyslav Predko, Oleh Kotsiumbas, Rostyslav Stotsko, Mykola Ostashuk

Research article

2023 06 26

On the effect of vibrational capture of rotation of an unbalanced rotor

Nikolay Yaroshevich, Vitalij Grabovets, Тetjana Yaroshevich, Irina Pavlova, Irina Bandura

V. Korendiy, O. Parashchyn, V. Heletiy, V. Pasika, V. Gurey, and N. Maherus, “Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter,” Vibroengineering Procedia, Vol. 52, pp. 35–41, Nov. 2023, https://doi.org/10.21595/vp.2023.23728

Copy Extrica

Copied to clipboard!

TY  - JOUR
DO  - 10.21595/vp.2023.23728
UR  - https://doi.org/10.21595/vp.2023.23728
TI  - Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter
T2  - Vibroengineering Procedia
AU  - Korendiy, Vitaliy
AU  - Parashchyn, Oleh
AU  - Heletiy, Volodymyr
AU  - Pasika, Viacheslav
AU  - Gurey, Volodymyr
AU  - Maherus, Nadiia
PY  - 2023
DA  - 2023/11/25
PB  - JVE International Ltd.
SP  - 35-41
VL  - 52
SN  - 2345-0533
SN  - 2538-8479
ER  - 

Copy Ris

Copied to clipboard!

 @article{Korendiy_2023, title={Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter}, volume={52}, ISSN={2538-8479}, url={https://doi.org/10.21595/vp.2023.23728}, DOI={10.21595/vp.2023.23728}, journal={Vibroengineering Procedia}, publisher={JVE International Ltd.}, author={Korendiy, Vitaliy and Parashchyn, Oleh and Heletiy, Volodymyr and Pasika, Viacheslav and Gurey, Volodymyr and Maherus, Nadiia}, year={2023}, month=nov, pages={35–41} }

Copy Bibtex

Copied to clipboard!

[1]V. Korendiy, O. Parashchyn, V. Heletiy, V. Pasika, V. Gurey, and N. Maherus, “Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter,” Vibroengineering Procedia, vol. 52, pp. 35–41, Nov. 2023, doi: 10.21595/vp.2023.23728.

Copy IEEE

Copied to clipboard!

Korendiy, Vitaliy, Oleh Parashchyn, Volodymyr Heletiy, Viacheslav Pasika, Volodymyr Gurey, and Nadiia Maherus. “Kinematic Analysis and Geometrical Parameters Justification of a Planetary-Type Mechanism for Actuating an Inertial Vibration Exciter.” Vibroengineering Procedia 52 (November 25, 2023): 35–41. https://doi.org/10.21595/vp.2023.23728.

Copy Chicago

Copied to clipboard!

Kinematic analysis and geometrical parameters justification of a planetary-type mechanism for actuating an inertial vibration exciter

Abstract

Highlights

1. Introduction

2. Research methodology

2.1. General design of the laboratory prototype being studied

2.2. Kinematic diagram of the planetary-type mechanism of the vibration exciter

2.3. Analytical dependencies for determining the kinematic parameters of the mechanism

3. Results and discussion

3.1. Simulating the trajectories of the unbalanced mass motion

3.2. Analyzing the speeds and accelerations of the unbalanced mass

4. Conclusions

References

Cited by

About this article

Related Articles