Numerical analysis of random vibration fatigue for a typical airborne equipment

2.1. Frequency domain method

Power spectral density function of acceleration is the main data of random vibration fatigue analysis. There are two ways to get the function, time domain analysis method and frequency domain analysis method. Time domain analysis method needs too much data, therefore we choose the frequency domain method.

The frequency domain analysis method was based on power spectral density, it had the advantages of less computation and it didn’t need cycle counting. So it was favored by many scholars. The estimation of structural fatigue life based on power spectral density function had been applied in industries such as automotive, aerospace, and machine manufacturing.

There were usually two methods to obtain power spectrum density of stress. One was the indirect method, which mainly used the finite element method to obtain the response power spectral density of local stress. Another method was direct method, strain patterns usually occurred to dangerous parts of the structure, the stress power spectral density was obtained by measured method. In this paper, the finite element method was used to obtain the response power spectral density of local stress. A dynamic model of complex structure was established, and the finite element method was used to calculate the stress power spectral density of the dangerous parts of the structure by controlling excitation inputs, applying boundary constraints, and defining structural parameters reasonably.

For a gaussian random process $x\left(t\right)$, the average value was 0, and the probability density function could be expressed as:

where, ${\sigma }_x$ was standard deviation, and it could be expressed as:

where, $G_{xx}\left(f\right)$ is unilateral PSD. The random vibration analysis of linear system could be calculated by PSD in frequency domain. When the excitation signal of linear system was Gaussian distribution, its response was also Gaussian distribution. We assumed that under the excitation of random vibration signal $x\left(t\right)$, the response of a linear system is $y\left(t\right)$. The auto-power spectrum of the system response was:

The cross-power spectrum of response and excitation was:

where, $H$ was Hermitian transformation of matrix.

2.2. Palmgren and Miner rule

Palmgren was the first person who proposed the linear hypothesis of damage accumulation of fatigue. He assumed that fatigue damage accumulation is linear. Miner proposed the calculation formula based on the linear hypothesis of damage accumulation, and formed the Palmgren-Miner rule [3].

According to Palmgren and Miner, every loading cycle could cause damage, failures would occur when the cumulative damage was equal to a certain extent D. They believed that fatigue damage can be linearly accumulated, and each stress is independent. The mathematical formulation of cumulative damage fatigue was shown in following Eq. (5):

where, $n_i$ was the number of cycles, applied at the $i$-th stress level ${\sigma }_i$, $N_i$ was the fatigue life at ${\sigma }_i$, and $k$ was the number of stress levels in the block loading spectrum. The ratio of the number of cycles at a given stress level to the fatigue life was called the cycle ratio. The equation above stated that fatigue failure would occur when the sum of the cycle ratios amounted to one.

2.3. Random vibration analysis

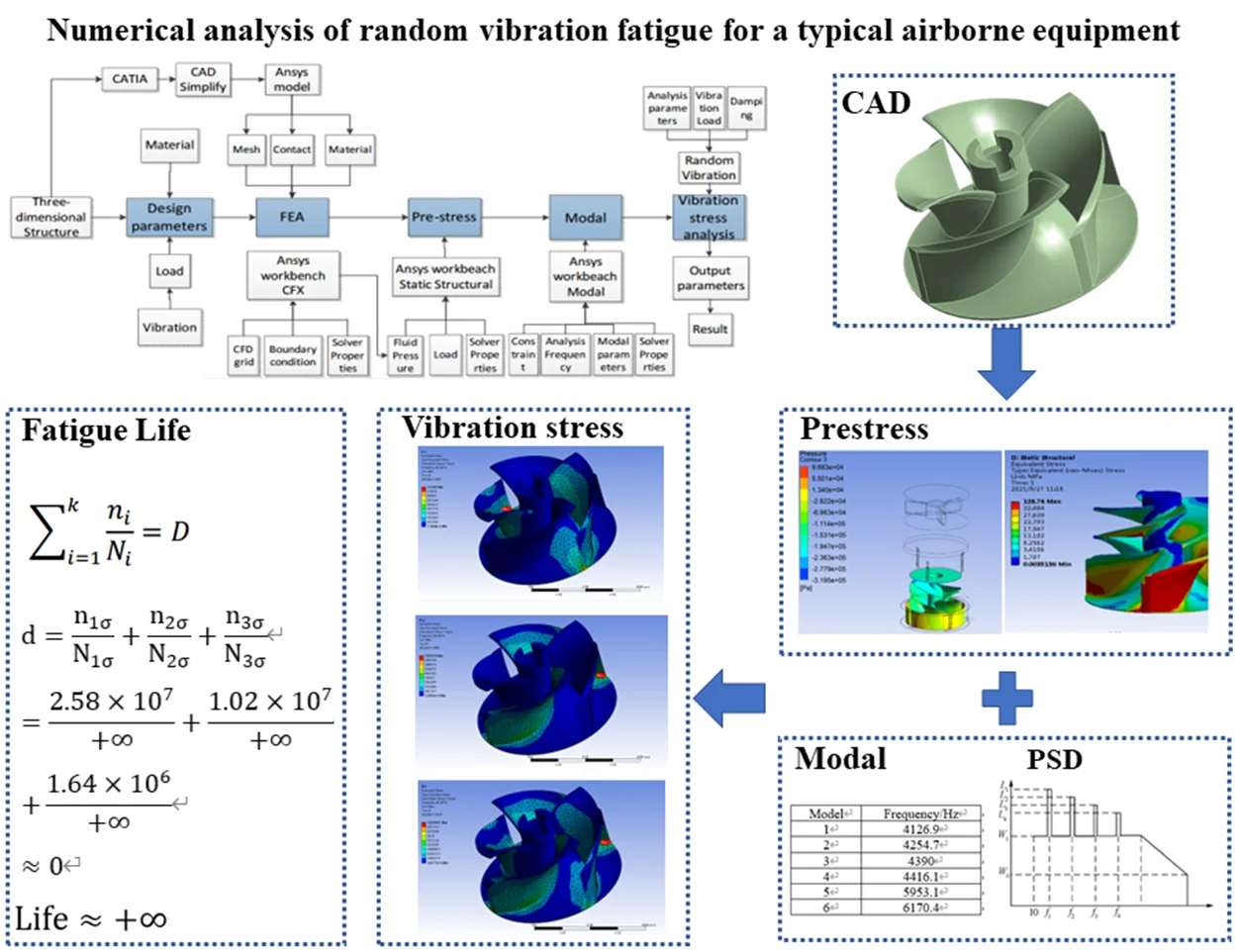
Based on the platform of ANSYS, random vibration analysis with prestress consisted of two steps. First, fluid structure coupling analysis was carried out. Then, the stress was transferred to modal analysis, and the modal with prestress was extracted, and the frequency of the vibration was analyzed. The specific analysis process was shown in Fig. 1.

Fig. 1Simulation and analysis process of random vibration with prestress

2.4. Prestress analysis

This simulation belonged to one-way fluid-structure coupling simulation. The fluid pressure on the impeller was obtained based on the platform of CFX. This fluid pressure was the input load for structural stress calculation. The fluid pressure and stress on the impeller was shown in Fig. 2.

Fig. 2The fluid pressure and stress on the impeller

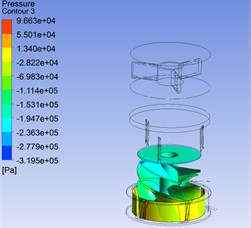
a) The fluid pressure

b) Equivalent (von-Mises) stress

2.5. Random vibration analysis

After obtaining the stress distribution of the impeller, the stress and stiffness matrix was imported into the random vibration analysis module. Modality analysis was done on the impeller with the finite element software ANSYS.

Helicopter vibration excitation included sinusoidal excitation and low-frequency random vibration excitation. Sinusoidal excitation could be equivalently converted into narrowband random vibration excitation through the following formula:

where, $G_q\left(B\right)$ was auto-power spectrum of the equivalent narrowband random vibration of sinusoidal vibration. B was equivalent narrowband bandwidth, $B=$4. $A\left(fn\right)$ was the peak value of sinusoidal vibration. $Q\left(fn\right)$ was quality factor. $fn$ was the sinusoidal vibration frequency. $\beta$ was equivalence factor,$\beta =$ 1.8.

Acceleration power spectral density could be obtained by superposition of broadband random vibration and narrowband random vibration. Through random vibration stress analysis under the random vibration excitation, stress PSD and maximum stress at dangerous parts of the impeller could be obtained.

The modal analysis results were shown in Table 1, and the corresponding mode shapes were shown in Fig. 3. The first three modes results were presented in the figure, 4126.9 Hz, 4234 Hz, 4476 Hz, outside the random vibration frequency range of the helicopter.

Fig. 3The corresponding mode shapes of fuel pump impeller

a) The first mode shape

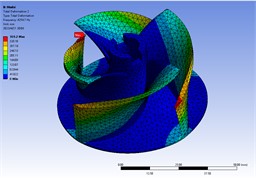
b) The second mode shape

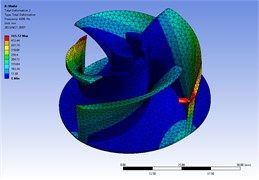
c) The third mode shape

Vibration stress response in $X$, $Y$, and $Z$ directions was shown in Fig. 4. According to the results of random vibration stress analysis, the maximum stress in the $X$ direction was 0.1165 MPa, it occurred at blade root. The maximum stress in the $Y$ direction was 0.1038 MPa, it occurred at blade root. The maximum stress in the $Z$ direction was 0.024 MPa, it occurred at blade root.

Fig. 4Vibration stress response in X, Y, Z

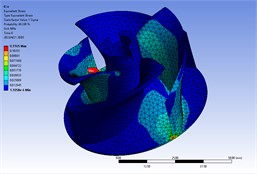
a)$X$ direction

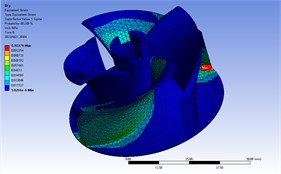
b)$Y$ direction

c)$Z$ direction