Comparative analysis of Al-SiC metal matrix composite and Al 7075 T651 alloy in the construction of CubeSat frame

2.1. Al-SiC Metal Matrix composite

Metal matrix composite (MMC) consists of reinforcing particles with a metal matrix phase. Usually, low density materials such as aluminium, magnesium, titanium are used as metal matrix. Al-SiC is a metal matrix composite which has aluminium as the metal matrix and silicon carbide as the reinforcement particle. The Al-SiC Metal Matrix Composites find their application in many industrial products due to their unique properties such as light weight, high strength, high specific modulus, high fatigue strength, high hardness, and low density. Rheo-process, compo-casting are some of the widely using processing techniques for this composite [2]. Fig. 1 shows some mechanical components manufactured using Al-SiC.

Fig. 1Some mechanical components manufactured using Al-SiC [3]

2.2. Al 7075 T651 alloy

Al 7075 T651 is an aluminum alloy, in that the primary alloying element id Zinc. It exhibits good ductility, toughness, high strength, and good resistance to fatigue. Al-7075 alloy was first used in the Mitsubishi A6M Zero fighter. This aircraft had excellent maneuverability which was achieved by the higher strength of Al 7075 compared to previously used aluminum alloys. It finds its application in gears and shafts, aircraft fittings, regulating valve parts, missile parts and worm gears etc., [4] Fig. 2 shows the chemical composition of Al 7075 T651 alloy.

Fig. 2Chemical composition Al 7075 T651 alloy

2.3. CAD modelling

The CubeSat frame is designed in CATIA V5 software. CATIA is a multi-platform software suitable for computer-aided design (CAD), computer-aided manufacturing (CAM), computer-aided engineering (CAE), PLM and 3D, developed by the French company Dassault Systèmes. The model is designed in part design section of CATIA V5. The frame is designed with the dimensions of 0.1 m in length, 0.1 m in breadth, and 0.11 m in height. The thickness of frame is 0.04 m. The final CAD is shown in Fig. 3.

2.4. Finite element analysis

Finite Element Analysis (FEA) is the simulation of a physical model using numerical mathematical equations referred to as the Finite Element Method (FEM). Engineers use FEM to reduce the production of physical prototypes and run computational analysis to optimize their designs. Partial differential equations are used to analyze most of the cases in FEM, but in some complex cases, multiple higher order equations are implemented. Finite Element Analysis is the primary computational analysis technique in industries [5]. Finite element analysis is used for predicting the reaction of a product to real forces, heat, vibration, fluid flow, and other physical effects. Its results will declare whether a product will break, wear out, or work the way it was designed [5].

ANSYS 19.2 software is used here to run and visualize the results of finite element analysis of a CubeSat’s structural frame. The CAD model was converted to IGS file and imported to ANSYS Design modeler. For predicting the effective functioning of the two materials, three types of analysis have been carried out, i.e., Static structural analysis, Modal analysis, and transient thermal analysis.

Fig. 3CAD model of CubeSat’s frame

2.4.3. Transient thermal analysis

Thermal analysis is an analytical tool in the branch of materials sciences. Accurate thermal properties of materials at fixed or varying temperature can be determined through this analysis. Transient thermal analysis determines the response of a system which undergoes fixed and varying boundary conditions over time.

The elementary equation used in the Thermal analysis:

7

$\left[C\right]\left\{\dot{T}\right\}\mathrm{}+\mathrm{}\left(\left[K_c\right]+\left[K_h\right]\right)\mathrm{}\left\{T\right\}=\mathrm{}\left\{R_t\right\}\mathrm{}+\mathrm{}\left\{R_q\right\}+\mathrm{}\left\{R_h\right\},$

where, $\left[C\right]$ is the matrix of heat capacitance, $\left[K_c\right]$ is matrix of conductive heat coefficient, $\left[K_h\right]$ is matrix of convective heat coefficient, $\left\{R_t\right\}$ is an array of heat flux across the boundary, $\left\{R_q\right\}$ is an array of heat generation, and $\left\{R_h\right\}$ is an array of convective heat flux [9].

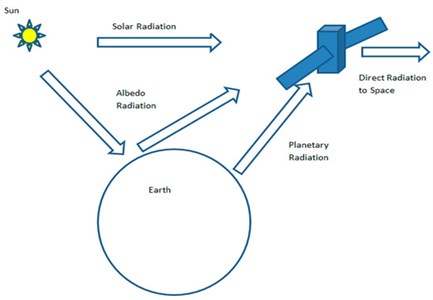
A CubeSat must work effectively in varying and hard space environment. There are three major thermal radiations which will be faced by a CubeSat:

– Radiation from the Sun,

– Albedo effect of the Earth,

– Infrared radiation from the Earth.

Satellites in the low earth orbit have to experience the temperature of range, –80 to +100 degree Celsius (approximately). In addition to this, conduction also occurs. The components of a satellite themselves emit certain amount of heat while consuming electrical energy from batteries.

The thermal load of direct solar flux acts on three faces, albedo effect acts on two faces and the earth’s infrared radiation acts on two faces. The satellite also emits radiation because of its temperature from its outer surfaces. To bear the payload properly in space, a structure should withstand maximum temperature [9]. Refer Fig. 4 for better understanding of thermal loads.

Fig. 4Thermal loading on a satellite

The equation used in the Transient thermal analysis:

8

$\left[B\right]\left\{\dot{T}\right\}+\left[K\right]\left\{T\right\}+\left[R\right]{\left\{T\right\}}^4=\left\{P\right\},$

where, $\left[K\right]$ is the matrix of conduction, $\left[R\right]$ is the matrix of radiation, $\left[B\right]$ is the matrix of heat capacity, $\left\{P\right\}$ is an array of heat loads. The solar radiation, albedo, and the infrared radiation are expressed in terms of heat flux. Heat flux ($\phi$) is the rate of heat energy transfer through a given surface ($W$), and heat flux density ($\phi$) is the heat flux per unit area (W/m²) [9].

3.1. Static structural analysis

A CubeSat’s frame has to withstand 50$g$ load ($g=$ 9.8 m/S²) in an average. Therefore, the loads are applied on the upper part of the four rails and the bottom part of the rails is fixed. Since, the bottom part is usually in contact with the P-Pod (spring-loaded deployment mechanism). The simulation is done in the case when the CubeSat’s bottom part is clamped with the P-Pod and the loads are applied on the top part ($Z$-direction). The analysis has been carried out for both the materials. i.e., Al 7075 T651 alloy and Al-SiC metal matrix [10].

Fig. 6Static structural analysis results of Al 7075 T651

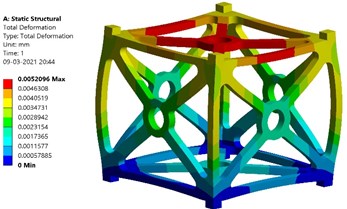
a) Total deformation – Al7075 T651

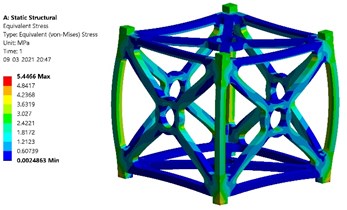
b) Equivalent stress created – Al 7075 T651

Fig. 6(a) and (b) show the simulated results for Al 7075 T651 alloy. The contours of total deformation and equivalent stress are obtained. As per the results, the maximum value of total deformation is 5.2e-6 m (0.0052 mm) and the value of maximum equivalent stress created is 5.446 MPa. The maximum yield stress of Al 7075 T651 is 503 MPa. Thus, the factor of safety is obtained as follows: 503/5.446 = 92.36.

Fig. 7(a) and (b) show the simulated results for Al-SiC metal matrix. The contours of total deformation and equivalent stress are obtained. As per the results, the maximum value of total deformation is 1.7e-6 m (0.0017 mm) and the value of maximum equivalent stress created is 5.531 MPa. The maximum yield stress of Al-SiC is 43.68 MPa. Thus, the factor of safety is obtained as follows: 43.68/5.531 = 7.90.

Fig. 7Static structural analysis results of Al-SiC

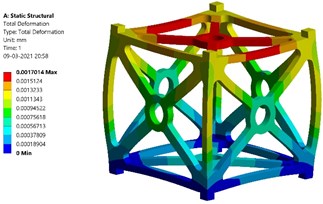
a) Total deformation – Al-SiC

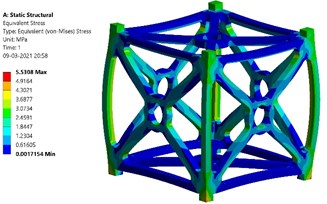
b) Equivalent stress created – Al-SiC

Comparing the results, Al 7075 T651 has higher value of factor of safety. On the other hand, Al-SiC has lesser value of total deformation.

3.2. Modal analysis

The design of the primary and secondary structures should ensure that their fundamental frequencies are satisfying the minimum values set by the launchers, by a good margin. The launching vehicle for this CubeSat is assigned as Polar Satellite Launch Vehicle (PSLV). The excitation frequency of the Polar Satellite Launch Vehicle is 100 Hz. As per the experimental data of Polar Satellite Launch Vehicle, the lowest natural frequency of the entire system must be greater than its excitation frequency (100 Hz). Modal analysis is done for first six modes and its corresponding deflections are also obtained [11].

Table 4 shows the input details applied before performing Modal analysis. Undamped natural modes of the system are obtained. Block Lanczos mode extraction method is used as it can be implemented for its quicker convergence characteristics, and also it is suitable for symmetric cases. As this model consists of only one component i.e., chassis, and no other interference of geometries, contact modelling is not applied.

Fig. 8Results of modal analysis – Al 7075 T651

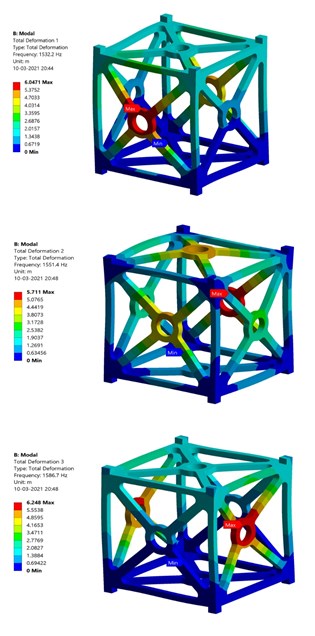
a) Al 7075 T651 – Modes 1, Mode 2, Mode 3 (Top, Middle, Bottom)

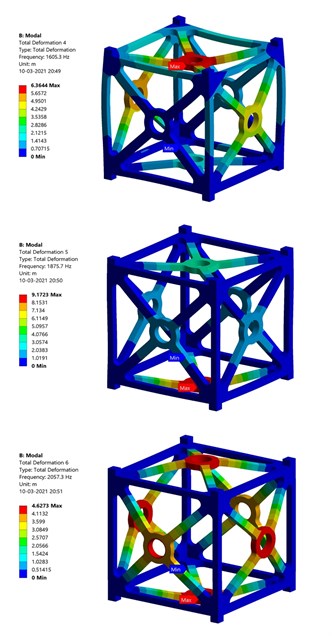
b) Al 7075 T651 – Modes 4, Mode 5, Mode 6 (Top, Middle, Bottom)

According to the specifications of launch vehicle, all the natural frequencies of both materials are greater than the excitation frequency of launch vehicle (100 Hz). Therefore, the CubeSat will not resonate easily. The first modal frequency of Al 7075 T651 is 1532.2 Hz and Al-SiC is 2620.1 Hz. Fig. 8 and Fig. 9 show the contours of Total deformation during modal analysis.

Fig. 9Results of modal analysis – Al-SiC

a) Al-SiC – Modes 1, Mode 2, Mode 3 (Top, Middle, Bottom)

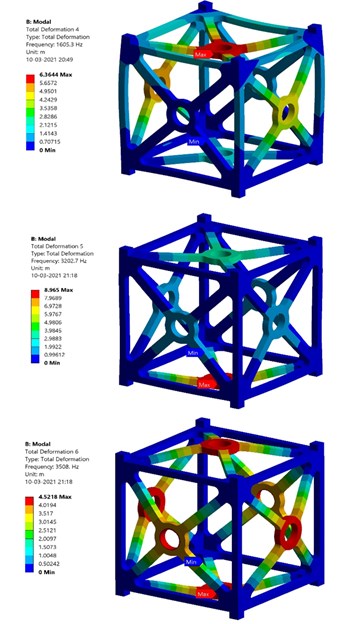
b) Al-SiC – Modes 4, Mode 5, Mode 6 (Top, Middle, Bottom)

Table 5 shows the first six natural frequencies of both Al 7075 T651 and Al-SiC. Al-SiC exhibits higher frequency in all the six modes than Al 7075 T651.

3.3. Transient thermal analysis

The CubeSat’s structure should withstand certain amount of solar radiation, infrared radiation, and albedo effect. These three external heat fluxes are applied on the six faces of the CubeSat. The internal temperature of the CubeSat is set as 298.15 K (25 °C). Table 6 shows the boundary conditions given for transient thermal analysis. Analysis is done for both worst hot and worst cold orbital conditions. At worst hot case, 35 % of the solar energy (497 W/m²) is reflected as albedo to the atmosphere by the earth. At worst cold case, 30 % of the solar energy (386.1 W/m²) is reflected as albedo to the atmosphere by the earth. The thermal loads are transferred to static structural mode to obtain the values of equivalent thermal stress [9].

The transient period is set as 30 seconds for this analysis and worst orbital conditions are applied in this analysis. Fig. 10 shows the transient thermal analysis results of Al 7075 T651 alloy. From the results, it is found that the maximum temperature which can be withstood by Al 7075 T651 alloy at worst hot case is 296.964 K (23.814 °C) and at worst cold case is 296.476 K (23.326 °C). The thermal stress produced at worst hot case is found to be 1.295 MPa. The thermal stress produced at worst cold case is found to be 1.186 MPa.

Fig. 10Transient thermal analysis results of Al 7075 T651

a) Temperature at worst hot case – Al 7075 T651

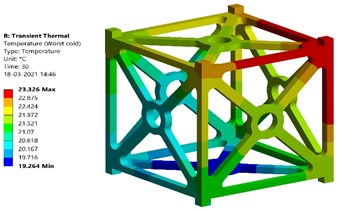
b) Temperature at worst cold case – Al 7075 T651

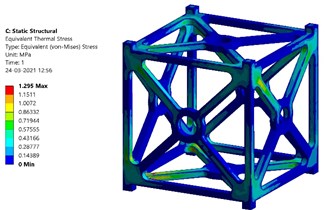
c) Thermal stress at worst hot case – Al 7075 T651

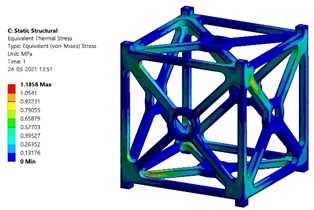
d) Thermal stress at worst cold case – Al 7075 T651

Fig. 11Magnitudes of temperature at worst hot and cold cases – Al-SiC

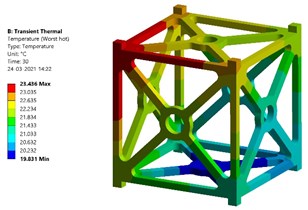
a) Temperature at worst hot case – Al-SiC

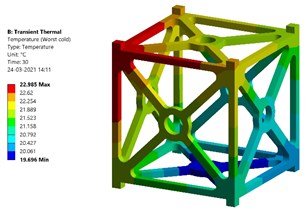
b) Temperature at worst cold case – Al-SiC

Fig. 11 and Fig. 12 show the transient thermal analysis results of Al-SiC metal matrix composite. In the case of Al-SiC composite, the maximum temperature which can be withstood at worst hot case is 296.586 K (23.436 °C) and at worst cold case is 296.135 K (22.985 °C). The thermal stress produced at worst hot case is found to be 0.60499 MPa. The thermal stress produced at worst cold case is found to be 0.55557 MPa.

The factor of safety of Al 7075 T651 based on the values of maximum thermal stress can be obtained as: At worst hot conditions, 503/1.295 = 388.41. At worst cold conditions, 503/1.295 = 424.11. The factor of safety of Al-SiC based on the values of maximum thermal stress can be obtained as: At worst hot conditions, 43.68/0.60499 = 72.199. At worst cold conditions, 43.68/0.55557 = 78.62. Comparing the numerical results, Al 7075 T651 alloy’s performance is good at worst hot condition with better factor of safety at both worst hot and cold conditions. Al-SiC composite shows better performance at worst cold condition.

Fig. 12Magnitudes of thermal stress at worst hot and cold cases – Al-SiC

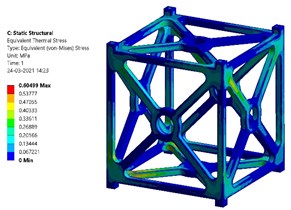
a) Thermal stress at worst hot case – Al-SiC

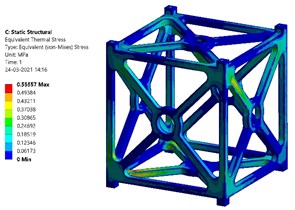
b) Thermal stress at worst cold case – Al-SiC

This work could be also validated with the experimental research carried out by Cui Yan et al. in 2008, “Multi-functional SiC/Al Composites for Aerospace Applications”, with the same range of specimen volume fraction which emphasizes the practical feasibility and manufacturing of aerospace structures using Al-SiC metal matrix composite [2].