Numerical simulation of steel tube array composite armor structure against rod projectile penetration

2.1. Basic assumptions

In this paper, the finite element numerical calculation model of projectile impact on SptAC armor structure is established, and the following assumptions and simplifications are made without affecting the calculation results: the influence of air resistance and gravity during projectile operation was ignored; the overall displacement of armor structure was not considered due to the short collision process; the steel plate, steel tube components and projectile materials in composite armor are uniform continuous medium.

2.2. Finite element model

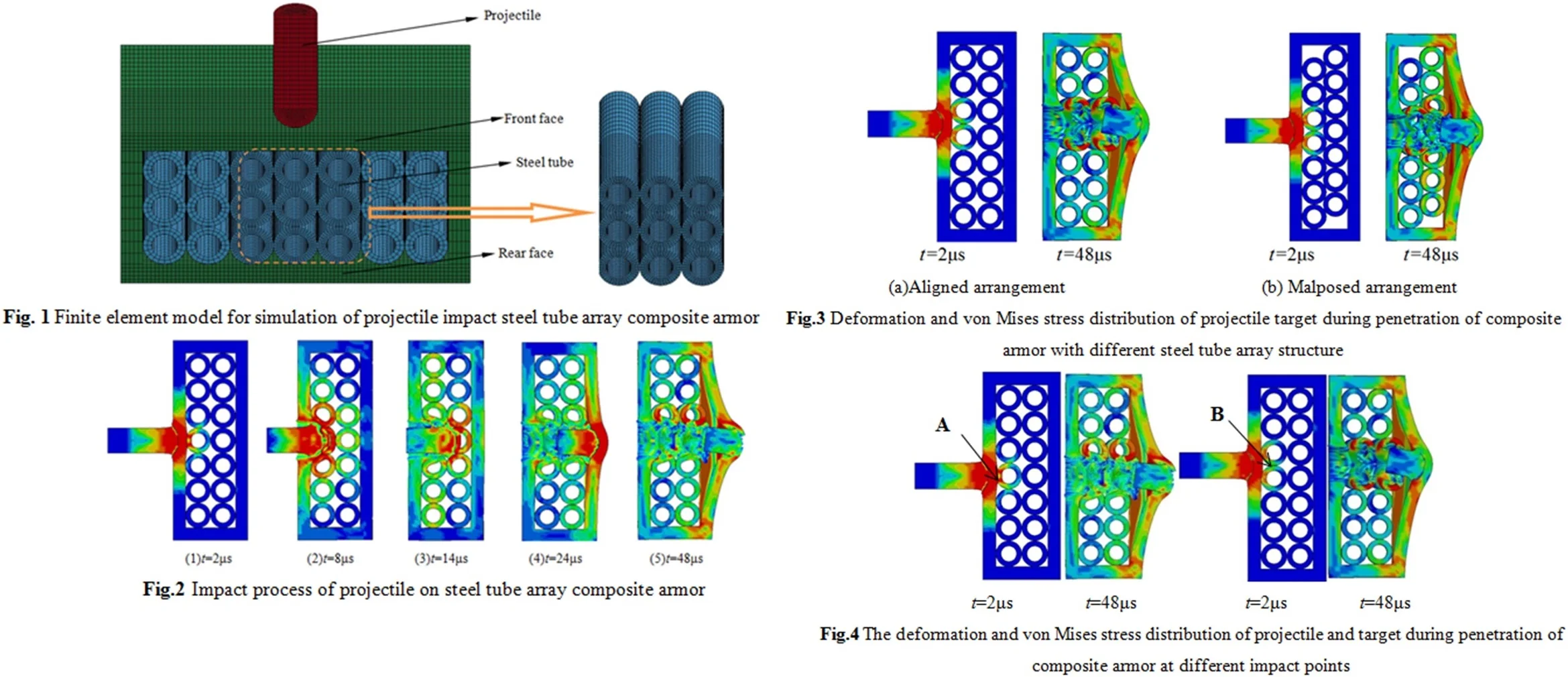
Obviously, the ballistic performance of the steel tube plate array is related to many factors, such as the material and size of the tube structure, the number of the tube, etc. In order to explore the feasibility of this kind of structure against projectile in advance, this paper fixed the size and number of tubes, and only considered the influence of tube layer number, arrangement mode and impact point position on projectile’s residual velocity and yaw. Fig. 1 shows the established finite element numerical model. There are two parts in finite element numerical model: the projectile, spherical head cylinder with a diameter of 2 mm, and slenderness ratio of 6 and a mass of 1.15 g; the armor, consist of 2 mm thickness target plate of the front, back and side face, and also the steel tube with 2 mm outer diameter and 1.2 mm inner diameter. Each layer of array is composed of seven steel tubes in contact. In view of the axisymmetric characteristics of projectile and target structure and load, a 1/2 model was established to save calculation time. Both the projectile and the target plate are made of solid 164 elements.

Fig. 1Finite element model for simulation of projectile impact steel tube array composite armor

In order to improve the calculation speed and ensure the calculation accuracy, the mesh division is relatively fine in the contact area between projectile and target. The element number of projectile target plate and steel tube array were divided into 4000, 28500 and 57600 respectively. The boundary conditions were applied to the side of the target plate to simulate the fixed condition of the target plate, and symmetrical constraints are applied on the symmetrical boundary.

2.3. Material model and parameters

When the numerical simulation of SptAC armor was carried out, the projectile and composite target plate adopt the same type of steel material, namely Q235 steel. In order to better simulate the fracture and failure of composite target under projectile impact penetration, the Johnson cook material model with failure fracture criterion was selected for both projectile and target [4]:

For von Mises yield stress model, the material yield stress is expressed as follows:

where: ${\stackrel{-}{\epsilon }}^p$ is equivalent plastic strain, ${\dot{\epsilon }}^{\mathrm{*}}$ is relative equivalent plastic strain rate, $T^{*}$ is relative temperature, $A$ is yield stress, $B$ is strain hardening, $n$ is strain hardening index, $C$ is strain rate correlation factor, $m$ is temperature correlation factor.

The expression of the fracture strain is expressed as follows:

where $D$ is the damage to a material element, $\mathrm{\Delta }{\epsilon }_y$is the increment of accumulated plastic strain, and ${\epsilon }_f$ is the accumulated plastic strain to failure under the current conditions of stress triaxiality, strain rate and temperature. Failure occurs when $D=$1. The material model parameters of Q235 steel are [5]: $\rho =$ 7.8 g/cm³, $A=$ 229.0 MPa, $B=$ 439.0 MPa, $n=$ 0.503, $C=$ 0.1, $m=$ 0.55, $D_1=$0.3, $D_2=$0.9, $D_3=$ –2.8, $D_4=$0.0, $D_5=$0.0.

3.1. Projectile penetrating progress

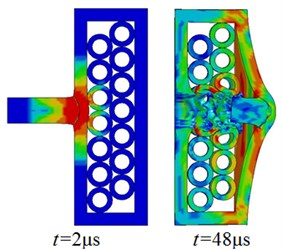
Taking the two-layer aligned steel tube array composite armor as an example, Fig. 2 shows the deformation and von Mises stress distribution of projectile and target plate at different times during the impact penetration at the speed of 1000 m/s. The results show that at the moment of contact, the stress wave propagates from the contact point of the projectile to the projectile and the panel respectively. In a short time, the stress wave propagates rapidly through the steel tube. When $t=$8 μs, the stress wave has reached the bottom of the projectile. At this time, the steel tube at the impact point has suffered severe deformation, and the steel tube side around had also undergone obvious buckling. After that, the stress wave continuously reflects and propagates back and forth in the projectile and composite target. When $t=$24 μs, the two layers of steel tube have been completely damaged, and the projectile starts to act on the back plate, which causes serious deformation of the back plate. When $t=$48 μs, the back plate had been penetrated. At the point of contact between the projectile and the composite target, the stress was always the maximum during the penetration process, the composite target plate seriously deformed, this is also the reason why the material of projectile and target at this point is easy to be penetrated and destroyed.

Fig. 2Impact process of projectile on steel tube array composite armor

a)$t=$2 μs

b)$t=$8 μs

c)$t=$14 μs

d)$t=$24 μs

e)$t=$48 μs

3.2. Influence of layers number on anti-penetration performance of SptAC armor

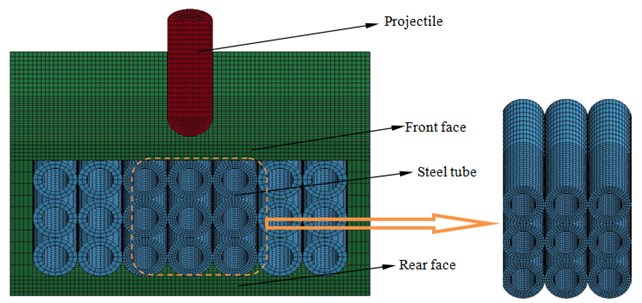
In order to study the effect of the layer number of the steel tube array on anti-penetration performance, the other parameter such as material and quality of the projectile and impact velocity were fixed. The values of the composite target plate of one layer, two layers and three layers of steel tube were calculated respectively. Fig. 3 shows the comparison of impact process of projectile on composite armor with different layers of steel tube array.

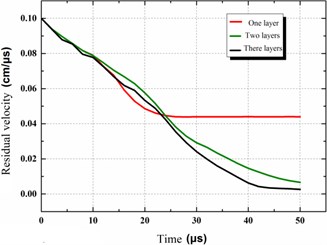
With the increase of the number of tube array layers, the anti-penetration ability of armor structure to projectile was significantly improved. On the one hand, with the increase of the number of steel tube array layers in the composite armor, the armor thickness that the projectile needs to penetrate was increased; on the other hand, when the number of steel tube array layers was large, the interaction time between the projectile and the composite armor increases, and the deformation energy absorption effect caused by the steel constraint was more significant, which makes the residual velocity of the projectile significantly reduced (as shown in Fig. 4).

Fig. 3Deformation and von Mises stress of projectile and armor with different layers at 48μs

a) One layer

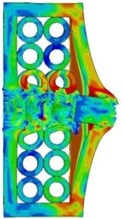
b) Double layers

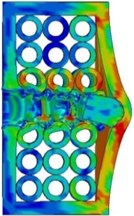
c) Three layers

Fig. 4Residual velocity curve of projectile under different layers of steel tube array

3.3. Influence of tube array relative position on anti-penetration performance of SptAC armor

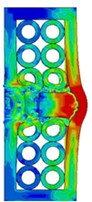
When the number of layers of steel tube array of composite armor is more than one layer, the array mode of each layer may have different forms, such as steel tube aligned arrangement and malposed arrangement between layers. Take the two-layer steel tube array composite armor as an example for simulation, as shown in Fig. 5.

The residual velocity and kinetic energy of projectile penetrating the composite armor with malposed arrangement structure decrease faster than those with aligned arrangement structure. When $t=$20 μs, the residual velocity of projectile is 554 m/s in two-layer aligned arrangement structure and 533 m/s in two-layer malposed array structure. The reason is that in the malposed array structure, each steel tube contacts with four steel tubes, while in the aligned structure, each steel tube contacts only three steel tubes. Therefore, the malposed array structure can transfer more energy to the surrounding steel tube to absorb when the projectile and target collide and deform, so as to achieve better protection performance.

3.4. Influence of projectile initial position on anti-penetration performance of SptAC armor

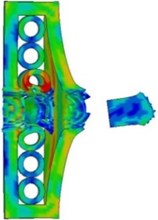
Keeps the same initial conditions as the simulation mentioned above. The impact point A (facing the center of the upper surface of the steel tube) and the impact point B (located in the middle of the adjacent steel tube) were numerically simulated (as shown in Fig. 6).

From the simulation results, for different steel tube array structures, the impact protection performance of composite armor for projectile is obviously different. When the projectile penetrates the composite armor at the impact point A, the residual velocity of the projectile is 66 m/s; when the projectile penetrates at the impact point B, the projectile velocity decreases to 0. The calculation results show that it is more difficult for the projectile to penetrate the composite target at the impact point B than at the impact point A when other conditions remain unchanged. Therefore, the dislocation array structure can transfer more energy to the surrounding steel tube to absorb when the projectile and target collide and deform, so as to achieve better protection performance.

Fig. 5Deformation and von Mises stress distribution of projectile target during penetration of composite armor with different steel tube array structure

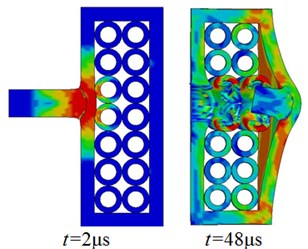
a) Aligned arrangement

b) Malposed arrangement

Fig. 6The deformation and von Mises stress distribution of projectile and target during penetration of composite armor at different impact points

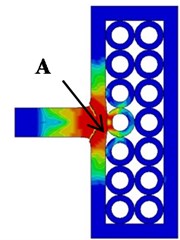
a)$t=$2 μs

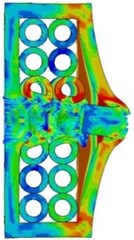
b)$t=$48 μs

c)$t=$2 μs

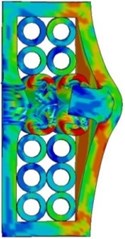
d)$t=$48 μs