Fatigue analysis of injector body based on ANSYS workbench

2.1. Physical geometry and mesh

The injector body has one fuel inlet. The fuel enters the oil chamber after passing through the oil inlet. After that the fuel divided into three parts flowing into three split ports. A physical model of the injector body is shown in Fig. 1.

The three-dimensional model of injector body is imported into ANSYS Workbench and is meshed, choosing another size for each part and the generation of face mesh with refinement on contact surface, the generated mesh is shown in Fig. 2. The statistical result is that the number of nodes is 341,962, and the number of elements is 231,381. The average elements quality is 0.79, and it is higher than 0.7; that proves that the quality of the elements is good.

Fig. 1Geometry of injector body

Fig. 2Mathematical model of injector body

2.2. Preset simulation condition

When the fuel loading and unloading instantly, the pressure will increase to 180 MPa at 7.1 s suddenly and the pressure of 180 MPa will be applied to the injector body. Then the pressure will decrease quickly, the period is 470.15 s. The Analysis Setting is made according to the period, loading the specified data to the Transient Structural module. Except the pressure of fuel, the injector body also have the bolt preload of 46500 N.

At the same time, constraints are added to the injector body model, and the Displacement is set as the type of constraints. The purpose is to limit the displacement of the injector body on some directions.

2.3. Material definition

Due to a high demand to fatigue strength of the fuel injector body, the material of injector body is proposed to be 20Cr steel, which has excellent processing properties. This grade of steel has high hardenability and wear-resistant surface after carburizing and quenching [3]. And the steel becomes mostly not prone to deformation after quenching. Some physical properties of 20Cr steel are shown in Table 1.

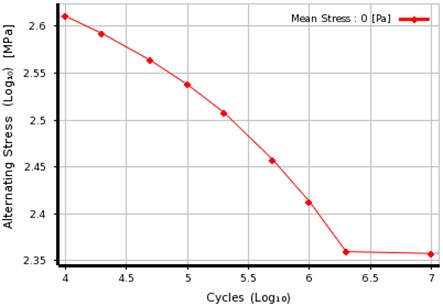
In order to get the fatigue life, it is also required to provide a S-N curve of 20Cr steel, according to experiment [4], and this S-N curve is shown in Fig. 3.

Fig. 3S-N curve of 20Cr steel

3.1. Analysis of stress

The Transient Structural is used in ANSYS Workbench to analyze the stress of the injector body provided that the fuel loads and unloads instantly. ANSYS uses the fourth strength theory to calculate the stress. The fourth strength theory is also called as the distortion energy theory. The expression is that the yield of material is caused by the change of energy density. This theory assumes that the change of energy density caused by deformation is the factor that causes material yield. This is to say, no matter what the stress state is, as long as the change of energy density caused by deformation at a point in the component reaches the fatigue limit value of the material that will produces the plastic yield. The fourth strength theory formula is:

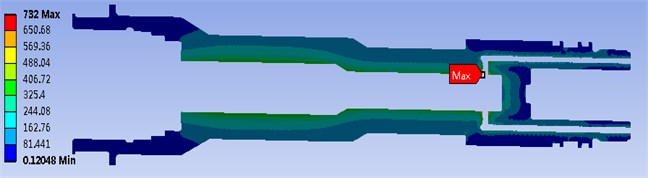
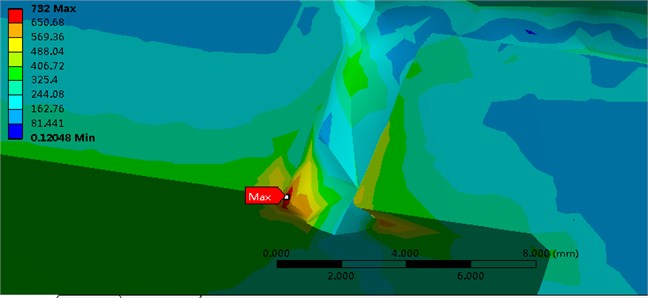
The result shows that the injector body suffers the max stress at 7.1 s, at that time the equivalent stress distribution is provided inside the injector body as shown the Fig. 4.

Fig. 4Equivalent stress distribution slice and partial detail at 7.1 s

a)

b)

The figure shows that the maximum equivalent stress value is 732 MPa and occurs at the entrances of three split ports. It can be seen the stress concentrations exist in the entrances of three split ports.

3.2. Analysis of fatigue strength

The S-N curve is a material fatigue curve which indicates the relationship between number of stress cycles and figure limit. The stress after $N$ cycles, the maximum stress cannot make the fatigue failure to the material which called as fatigue limit stress. The figure curve can be divided into two parts: one is the finite life region and the other is the infinite life region. The finite life region means that when the strain level of the components is lower or equal to the fatigue limit${\sigma }_{-1}$, the stress cycles can exceed $N_0$ that means the components will not have fatigue damage.

The relationship between the variable stress and cycle number $N$ under the stress which will lead to the fatigue damage is as follows:

This equation is called as fatigue curve equation, where ${\sigma }_{-1N}$ is the fatigue limit stress of under the finite region; where N is the cycle number fit the ${\sigma }_{-1N}$, m is the index related to the material, $C$ is the constant from experiments.

Fatigue Tool is used in ANSYS Workbench to simulate the fatigue strength of the injector body under the maximum stress. The stress concentration parts will be discussed carefully. The fatigue life of the entrances of three split ports should be discussed separately, but the partial detail figure of fatigue life is shown in Fig. 5.

Fig. 5Fatigue life partial detail figure

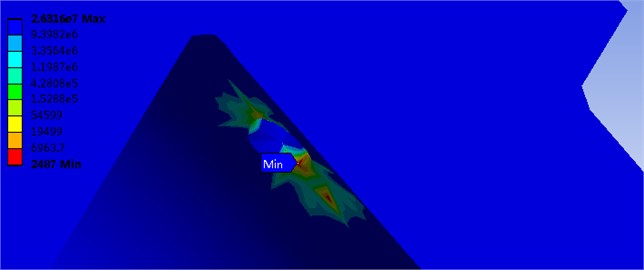
The figure shows that the minimum cycle number, which exists at the entrances of split ports, is 2487. Because the fuel loads and unloads instantly, and this always happens only during the startup of the diesel engine’s operation, the cycle number 2487 can meet the requirement. However, in order to increase the fatigue life and extend the using time, it is required to reduce the maximum stress of the stress concentration.

3.3. Structural modification

It is known from the above, although the fatigue strength can meet the requirement, the maximum value of stress concentration is large [5]. In order to reduce the stress concentration, it is required to modify the structure of the injector body.

Fig. 6Modified structure model partial detail figure

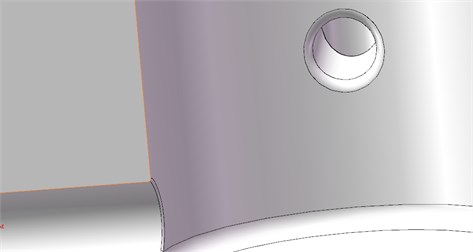
One of the methods to reduce the stress concentration consist in creating a fillet at the entrances of split ports [6], which will make the fuel inside the injector body flows more gently. So, the maximum value of stress can be reduced. This is a new method to reduce the stress concentration of the injector body. The model of the modified structure is shown in Fig. 6.

Take the radius of fillet is 0.4 mm as an example, after creating fillets, the analysis results are shown in Fig. 7.

Fig. 7Stress and fatigue strength figure after creating fillets

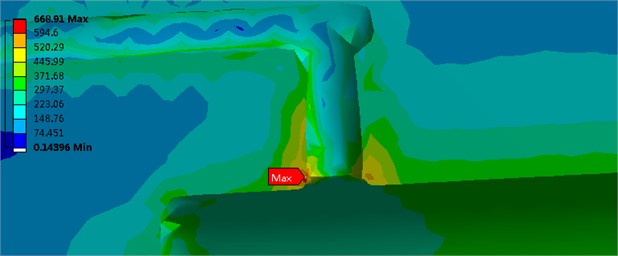
a)

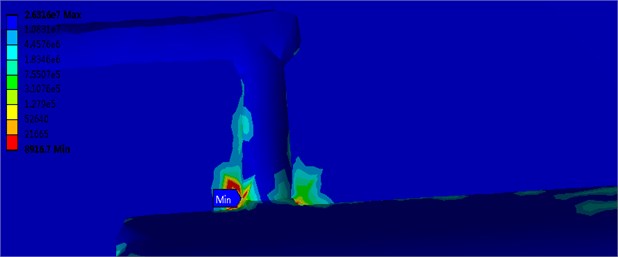
b)

As shown in the figure, after creating the fillets, the stress concentration is also available, but the maximum value of stress declines obviously, and the fatigue strength is also increasing.

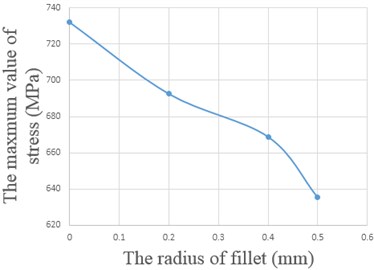
The radius of fillets has important influences on the fatigue strength of the injector body. So, if creating different radiuses of fillet to simulate the stress and the fatigue strength to prove whether different radius of fillet will affect the stress and the fatigue strength. The simulation results are shown in Table 2.

As shown in the above table, with the development of the fillet radius, the maximum value of stress decreases. However, due to the size of injector body itself the radius of fillet could not increase infinitely, so when the radius of fillet is 0.5 mm, it could reduce the maximum value of stress concentration and enhance the fatigue life well. The relationship between the radius of fillet and the maximum value of stress, and the relationship between the radius of the circular beads and the minimum fatigue life are shown in Fig. 8.

Fig. 8Relationship between radius of circular beads and stress, and fatigue life

a)

b)