Research on the influence of the normal vibration on the friction-induced vibration of the water-lubricated stern bearing

2.1. Fundamental equations

In the case of friction vibration of water-lubricated tail bearing, local contact between the stern bearing and stern shaft journal occurs. According to the analysis conclusion of Krauter and Smith [17], the movement between the stern bearing and stern shaft journal can be simplified as friction behaviour between a slat and sliding block, as shown in Fig. 1.

Fig. 1Simple model of friction-induced vibration

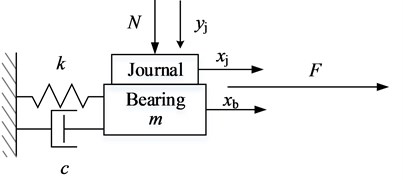
In the simple model, the journal is sliding on the surface of the bearing by the velocity of ${\dot{x}}_j$, and the relative velocity between the journal and the bearing is ${\dot{x}}_j-{\dot{x}}_b$. The dynamic friction is $F$, and the load on the bearing is $N$. The mass, stiffness and damping coefficient of the bearing is $m$, $k$ and $c$. The effect of transverse vibration is presented by the normal motion of journal $y_j$. Thus, the motion equation of the bearing slat can be expressed as:

where, the mathematical description form of dynamic friction $F$ is the problematic point in the study of friction vibration, and at present, it mainly consists of these following ways:

(1) Taylor series [5].

Supposing $F=f({\dot{x}}_j-{\dot{x}}_b)$, the right side of the Eq. (1) is expanded to the Taylor series according to the power of ${\dot{x}}_b$, and the following formula can be obtained after eliminating the high-order trace:

Generally, the motion of the bearing slat is micro-amplitude vibration. Therefore, the vibration equation of the bearing slat can be obtained without considering the minor term above the second order in Eq. (2) and the constant term irrelevant to vibration, as shown in the following equation:

The stability of friction vibration of the bearing slat can be discussed by using this equation.

(2) Polynomial.

Bannerjee et al. [18] proposed a hypothesis of quadratic polynomials, believing that the relationship between dynamic friction and sliding speed is:

where, $F_a$ is the dynamic friction force; $F_s$ is the maximum static friction force; $a$, $\beta$ is the experimental constant; $V_r$ is relative sliding speed.

(3) Empirical formula.

Frictional vibration generally occurs at lower relative velocities. Therefore, some empirical formulas of frictional force can be obtained based on coulomb's law of friction. As suggested by Smith, the friction force of water-lubricated bearing is qualitatively related to axial angular velocity [5]:

where, $N$ is the normal load of bearing; $a$ is the unit inverse velocity constant; ${\mu }_0$ and ${\mu }_1$ are respectively the static and dynamic friction coefficients; $r$ is the radius of the shaft disc; $\dot{\theta }$ is the axial angular velocity in contact with the stern bearing.

Some researchers also introduced a more simplified empirical formula [19]:

where, $N$ refers to the positive pressure between the bearing and the contact surface of the shaft; $k$ is the system stiffness; $c$ is system damping; $C_0$ is the constant related to the initial condition; $q_s$ is the slope of the relation curve between the friction coefficient and the speed measured by the bearing friction performance test; $u-\dot{x}$ is the relative velocity between contact surfaces.

In the above mathematical descriptions of the dynamic friction force $F$, the positive pressure of the sliding block on the slat remains unchanged. But in the course of friction vibration of the stern bearing, the sliding block simplified from the journal is actually in response to the lateral vibration. Thus, the displacement in the normal direction is variable, and then the positive pressure of the block on the slat is not constant. With this, referring on the above empirical formula, the motion equation of friction vibration of the stern bearing, considering the response of transverse vibration of the stern shaft, can be obtained:

where, $K_b$ is the compression stiffness of the stern bearing.

2.2. Numeric calculation

The numerical calculation is based on the performance test of a water-lubricated stern bearing conducted on the comprehensive performance test platform of the ship shafting system of Wuhan University of Technology, and the test results are shown in Table 1. The other test parameters involve: the stern shaft diameter is 185 mm; the length is 5 m; the stern bearing is 608 mm long; the pressure is 0.2 MPa; the room temperature is 30 ℃.

The approximate formula of friction coefficient can be obtained by fitting the quadratic polynomial according to the test data:

According to the above formula, the initial parameter can be obtained: $C_0$ is 0.409.

In order to simplify the calculation, the bearing slat is simplified into a unit mass block, and the system stiffness is the unit stiffness, and according to the calculation formula of damping in reference [20], the system damping is very small, then the damping can be ignored. So, $m=\text{1}$, $k=$ 1, $c=\text{0}$. Besides, supposing the bearing composition stiffness $K_b$ is also equal to 1, and the normal motion of journal $y_j$ is the stable sinusoidal periodic response, $y_j=A\mathrm{s}\mathrm{i}\mathrm{n}wt$, where $A$ is amplitude, and $w$ is response frequency, then the final equation of the mass is:

Let $x_2={\dot{x}}_b$, $x_1=x_b$, $v={\dot{x}}_j$, the Eq. (9) can be reduced to first-order differential equations:

Then, when the other initial conditions are determined, using the ODE45 function of Matlab libraries, the results of the numerical calculation could be obtained.

2.3. Experiments

The propulsion shafting experimental system at the Wuhan University of Technology has been designed and established in 2012. The test platform is mainly composed of frequency conversion motor, the decelerator, thrust bearing, propulsion shafting, the foundation and the base, also equipped with water lubrication system, oil lubrication system, hydraulic loading system and state monitoring system, which can display the friction coefficient, temperature, and pressure of tested bearings. The platform can carry out the research of propulsion shafting dynamics, shafting vibration, bearing and sealing performance test, etc. The tests of the coupled system dynamic behavior were carried out on this platform and the sensors gathering the bearing vibration and orbit of the stern shaft are shown in Fig. 2.

Fig. 2The sensors installation

a) Vibration sensors

b) Orbit sensors

The lubricating medium of this test is water, with the temperature 30 °C, the bearing pressure 0.20 MPa, the flow rate 30 L/min. After the running-in state for 50 hours, the expect experiment of lateral vibration on friction vibration was carried out. Because the shafting of the test platform is longer and the start torque is big, it is not easy to control the run-up process in the low-speed range. Therefore, this test chose to record the friction coefficient at a specific speed and the associated vibration signals in the slow run-down process.

The variation of lateral vibration was simulated by loading with bearing pressure 0.20 MPa, and no-load, because when the load changes, the response amplitude of the stern shaft journal changes accordingly, then the influence on the friction vibration can be observed.

During the test, the speed record points are that whose slope is negative in Table 1, which is 22, 27, 33, 43, 54, 87 and 141 rpm, and the corresponding transformation of the speed representation method with other shafting is shown in Table 2.

3.1. Friction-induced vibrations

When friction-induced vibration occurs, peak groups generally appear in the intermediate frequency region [21], as shown in Fig. 3, which is the vibration frequency spectrum diagram in the horizontal direction when the shaft speed is 87 r·min^-1. From this diagram, it can be seen that there were some small-peak groups in the range from 1.1 kHz to 1.6 kHz, which is response region of the friction vibration, and some apparent harmonic amplitude in the range lower than 800 Hz, which is the response region of the lateral vibration. Besides, from the corresponding time-domain diagram, as shown in Fig. 4, it can be seen that the apparent noise groups appear. Furthermore, from the orbit of shaft center, as shown in Fig. 5, it is clear that the journal began to contact with the bearing, and a jagged sharp angle appeared in the bottom-right corner of the axis trajectory, which is typical rubbing characteristics.

Fig. 3The vibration frequency spectrum diagram in the horizontal direction

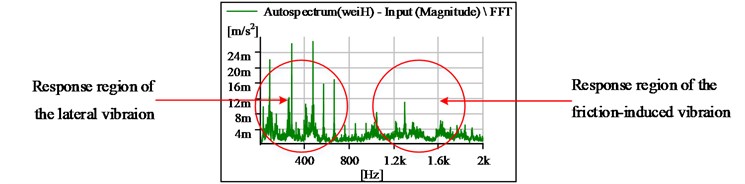
Fig. 4The time-domain spectrum

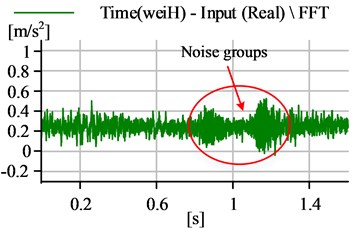
Fig. 5Orbit of shaft centre

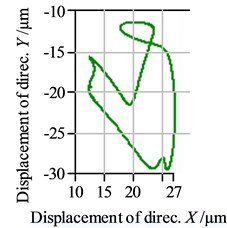
From the above analysis, it can be determined that the friction-induced vibration appeared at that point of the negative-slope area. Meanwhile, the stern bearing-shaft system is always in the state of misalignment and unbalance because of the apparent harmonics and the flat crescent-shaped axis trajectory. As the misalignment and unbalance are the main factors causing lateral vibration of the stern shaft, the motion morphology of friction-induced vibration is affected by the lateral vibration in the whole negative-slope area.

3.2. Influence of lateral vibration on the motion morphology of friction-induced vibration

Firstly, the effect of lateral vibration on dynamic friction is not considered. When the friction-induced vibration occurs, the normal motion of journal $y_j$ is set as a constant unit displacement, that is $y_j=$1. Select the speed point $v=$0.83 m/s, with $q_s$ is –0.000722545 and set the initial motion value of the vibration Eq. (10) as (0.1, 0.1), then the results of the numerical analysis within 100 s are shown in Fig. 6.

Fig. 6Motion morphology of friction-induced vibration equation without considering lateral vibration

a) Vibration displacement in horizontal direction

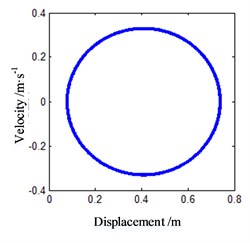
b) Phase trajectory in horizontal direction

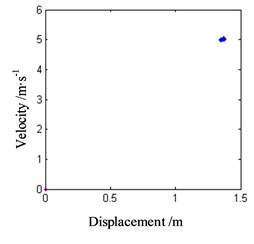
c) Poincare map in horizontal direction

From the figure, it can be seen that after 100 s passes on, the motion form of the unit mass block is still stable, and the phase point on the phase trajectory is always on the stable limit ring. The Poincare map also shows that the motion of the block belongs to the periodic motion. This indicates that the friction-induced vibration state at this speed point is stable, and the system will be in a continuous periodic vibration state and radiate frictional noise outwards.

Subsequently, the effect of lateral vibration on dynamic friction is considered. For example, the response amplitude $A$ is set as 1 m, and the response frequency $w$ is set as 10 Hz. Also, select the speed point $v=$0.83 m/s, with $q_s$ is –0.000722545, and set the initial motion value of the vibration Eq. (10) as (0.1, 0.1), then the results of the numerical analysis within 100 s are shown in Fig. 7.

Fig. 7Motion morphology of friction-induced vibration equation with A= 1 m and w= 10 Hz

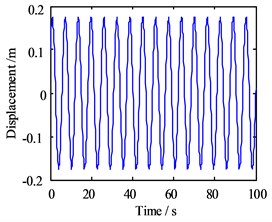
a) Vibration displacement in horizontal direction

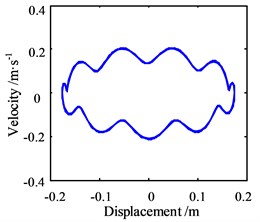
b) Phase trajectory in horizontal direction

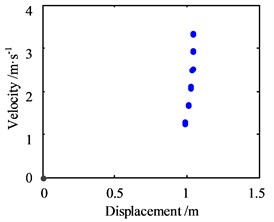
c) Poincare map in horizontal direction

Same as the motion without considering the lateral vibration response, the vibration displacement has no apparent decrease because of the minimal negative slope. Still, the phase trajectory shows that the lateral vibration periodic response of the journal indeed affects the friction-induced vibration of the unit mass block of the bearing slat, and the motion of the block becomes more complex, with the periodic effect on the trajectory. Besides, the Poincare map shows that the motion becomes the quasi-periodic state, and this phenomenon indicates that the motion is no longer stable.

For further analyzing the influence of the amplitude of lateral vibration response, the response amplitude $A$ is set as 10 m, and the other parameters are same as before, then the results of the numerical analysis within 100 s are shown in Fig. 8.

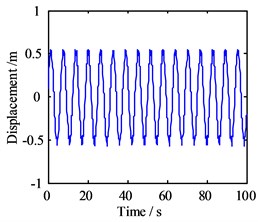
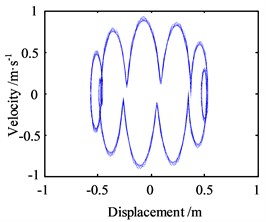
Fig. 8Motion morphology of friction-induced vibration equation with A= 10 m and w= 10 Hz

a) Vibration displacement in horizontal direction

b) Phase trajectory in horizontal direction

c) Poincare map in horizontal direction

With the amplitude $A$ increasing, the vibration displacement of the block also increases accordingly, and the phase trajectory and the Poincare map show the same form as the amplitude $A$ is 0, only with bigger value. It means that the variation of the amplitude of lateral vibration response makes the motion of friction-induced vibration of the block more complex and bigger, but no affections on the form of it.

Next, with the amplitude, $A$ still 10 m, the response frequency $w$ is changed to 100 Hz, and the other parameters are same as before, then the results of the numerical analysis within 100 s are shown in Fig. 9.

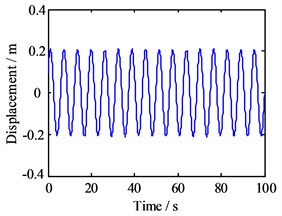
From this figure, it can be seen that the vibration displacement of the block is still stable, but the phase trajectory shows that the mass block is in a more complex motion on a loop, and the Poincare map also presents that the motion has fractal characteristics of the chaotic state. Therefore, this means that the higher frequency component of the lateral response tends to disturb the friction-induced vibration and cause it to collapse.

From the above analysis, it can be concluded that the normal vibration on the unit mass block will aggravate the amplitude of its friction-induced vibration. At the same time, it tends to periodize, and even chaos the motion of the block, and this trend will make it impossible to maintain a steady-state of friction-induced vibration. The friction-induced vibration will also not be detected anymore.

Fig. 9Motion morphology of friction-induced vibration equation with A= 10 m and w= 100 Hz

a) Vibration displacement in horizontal direction

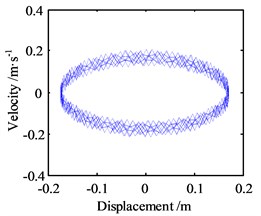
b) Phase trajectory in horizontal direction

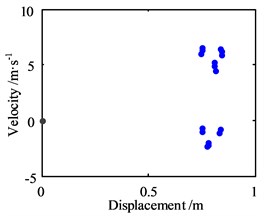
c) Poincare map in horizontal direction

3.3. Influence of lateral vibration based on the different-loading tests

When the test bench is in the condition of the different-loading, the lateral vibration of the stern shaft is different. Here is an example, which is the vibration frequency spectrum diagram in the horizontal direction with zero-load, , as shown in Fig. 10(a), and loading with bearing pressure 0.20 MPa, as shown in Fig. 10(b), when the shaft speed is 87 r·min^-1.

From the figure, it can be seen that Fig. 10(b) has more higher-frequency components and the bigger amplitude in the response region of the lateral vibration, and this means that the condition of loading can have the stronger effect than zero-load on the friction-induced vibration. Hence, from the perspective of the experiment, the effect of lateral vibration on friction-induced vibration can be observed by changing the load to get the variation of amplitude in the response region of the friction-induced vibration. The peak value of the friction region in the horizontal direction with every speed point, as listed in Table 2, was recorded under zero-load and bearing pressure 0.20 MPa. The variation curve is shown in the Fig. 11.

Fig. 10The vibration frequency spectrum diagram in the horizontal direction

a) Zero load

b) Bearing pressure 0.20 MPa

Fig. 11The variation curve of peak value in the friction region

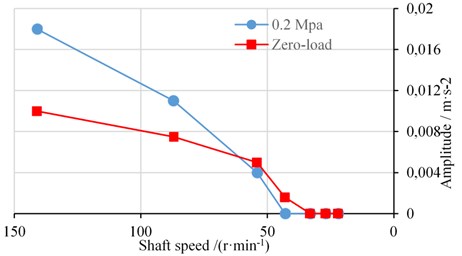
From this above variation curve, obtained by collating the test data, it can be observed that the amplitude decline rate in the friction region is relatively higher under the load of 0.2 MPa, and especially the amplitude cannot be detected when it is down to 43 rpm, while there is still data under no-load conditions. The test result indicated that the increase of load compresses the speed range where the friction-induced vibration occurs in the stern bearing-shaft system. The test result is also consistent with the above numerical analysis results that the effect of lateral vibration has the tendency of quasi-periodic and chaotic friction vibration and makes it unstable. Therefore, for the underwater vehicle, which often operates in the low-speed zone, the stern bearing-shaft system can reduce the noise intensity caused by friction-induced vibration more under load conditions.