Dynamical behavior of universal rocket transportation launching device on rough road with discrete obstacles

1.1. Theoretical background

As the most typical situations, crossing of discrete (cleats) obstacles by trailer wheels and associated with this, dynamical characteristics of vertical motion of the rocket body as well as roll motion of the trailer system when crossing a cleat and the possibility to loose stability were analyzed in the current research.

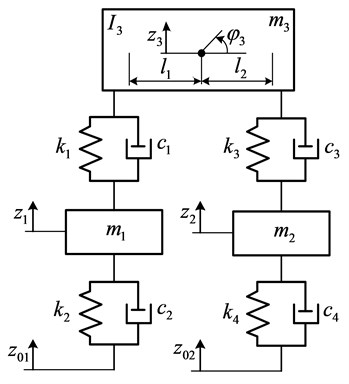
Lumped parameter half vehicle model created for the analysis of vertical dynamics is shown in Fig. 1, and roll motion characteristics of the frame of the device were analyzed with use of 2D, lumped parameter model shown in Fig. 2.

Fig. 1Half vehicle model of the universal rocket transportation launching device

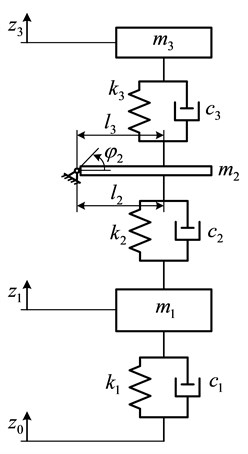
Fig. 2Perpendicular plane 2D model of the universal rocket transportation launching device

The following assumptions were made: a discrete single obstacle is crossed without losing forward velocity due to sufficient power of the towing vehicle; the trailer frame is free to pivot about hooking point to the tractor; pivot point maintains constant vertical coordinate; wheels of the trailer roll in free mode [4], i.e. traction force acts at rotation axis of the wheel, no external (driving or braking) moments are applied. Then equations of motion for half vehicle model (Fig. 1) are as follows:

where $m_1$ – unsprung mass, $m_2$ – sprung mass, $m_3$ – mass of the rocket target, $k_1$, $c_1$ – resultant stiffness and damping coefficients of the right and left tires, $k_2$, $c_2$ – stiffness and damping coefficients of the trailer suspension, $k_3$, $c_3$ – stiffness and damping coefficients of the rocket suspension, $I_2$ – total mass moment of inertia of the trailer and the rocket with respect to the pivot point of the hooking device, $l_2$ – the distance of wheel axis from pivot point, $l_3$ – distance of the frame mass center from the pivot point, $z_0$, $z_1$, $z_3$ – vertical coordinates of wheel-road contact point, wheel axis, rocket mass center accordingly, ${\phi }_2$ – angular coordinate of the unit’s frame.

For roll motion analysis of the rocket frame, mathematical model is derived:

where $m_1$ and $m_2$ are unsprung masses of right and left wheels stations accordingly, $m_2$ – total sprung mass representing the trailer frame and the rocket, $I_3$ – total mass moment of inertia of the trailer and the rocket with respect to the center of gravity, $k_2$, $c_2$ and $k_4$, $c_4$ – stiffness and damping coefficients of the right and left tires accordingly, $k_1$, $c_1$ and $k_3$, $c_3$ stiffness and damping coefficients of the wheel stations.

1.2. Simulation results and discussion

Pulse type input signals representing the process of traversing of a single discrete obstacle in the form of rectangular cleat by a wheel or traversing several cleats shifted one with respect to another along the forward motion axis are considered as dangerous excitation cases from the perspective of trailer motion stability. Following the methodology [5], enveloping properties of the tire were taken into account and its inertia properties neglected.

Fig. 3Vertical velocity of the rocket at different motion velocities of the trailer

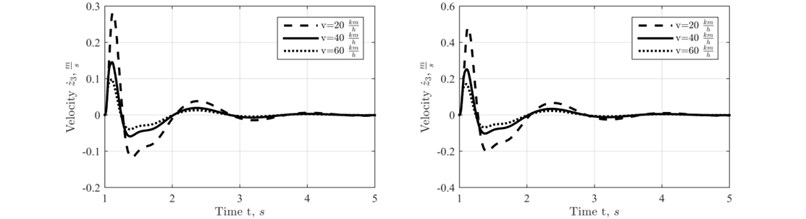
Fig. 4Vertical acceleration of the rocket at different motion velocities of the trailer

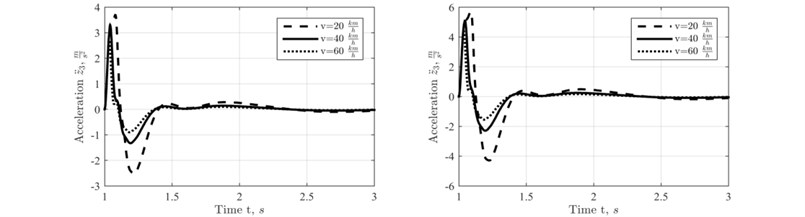
Fig. 5Angular accelerations of the trailer body at its different motion velocities

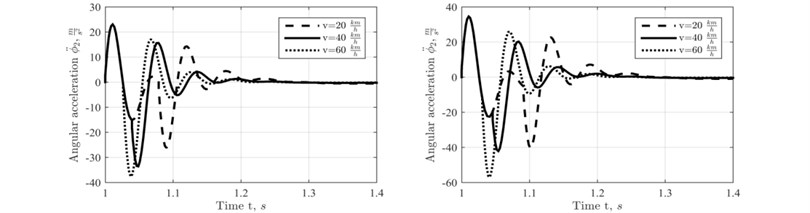
Fig. 6Vertical displacement of the rocket when traversing rectangular cleat by right wheel at different motion velocities of the trailer

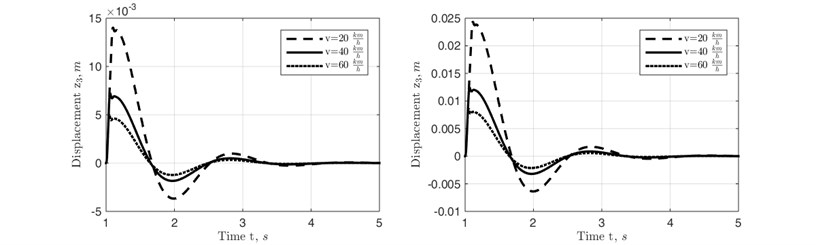
Fig. 7Angular accelerations of the frame when traversing rectangular cleat by right wheel at different motion velocities of the trailer

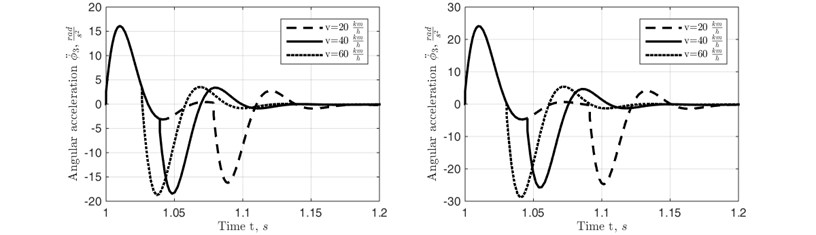
Fig. 8Angular displacement of the frame when traversing longitudinally shifted (1s shift in time axis) rectangular cleats by left and right wheels at different motion velocities of the trailer

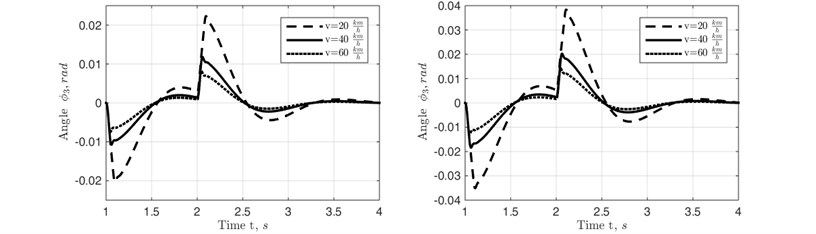
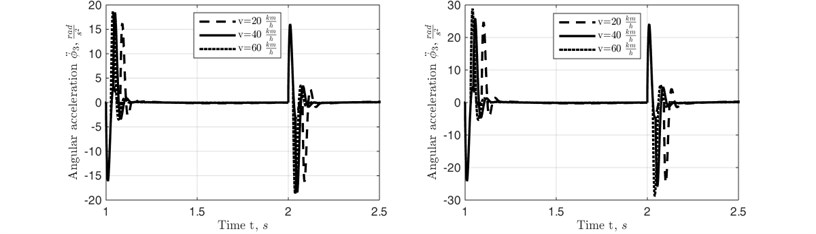
Fig. 9Angular acceleration of the frame when traversing longitudinally shifted (1s shift in time axis) rectangular cleats by left and right wheels at different motion velocities of the trailer

Simulation of the system behavior was carried out in Matlab Simulink environment. The cases of crossing different height rectangular cleats at different speeds of the unit were investigated. Simulation results of the half vehicle model are presented in Figs. 3-5.

Simulation results of perpendicular plane 2D model are presented in Figs. 6-10.

The graphs on the left column of all the figures represent the case of traversing 0,1 m height cleat, and the right column graphs represent traversing of 0,15 m height cleat.

Vertical displacement, velocity and acceleration of the rocket in transportation position were investigated with the use of half vehicle model and angular displacement, Angular displacement and angular acceleration of the frame with the attached to it rocket target in roll motion mode were investigated with the used of 2 Perpendicular plane 2D model. It is obvious from the obtained simulation results that the motion of the frame – rocket unit is oscillatory and strongly decaying. Therefore, the conclusion that single discrete obstacles (rectangular cleats) of moderate height can be crossed by the universal rocket transportation launching device at relatively high speeds of its motion can be made. Thus, the universal rocket launching device can function effectively in transportation mode moving on a ground of a training field.