The effect of dry friction forces on the process of dielectric wafer grinding

1.1. Mathematical model

In the development of the mathematical model it is assumed that the relative material removal rate is proportional to the relative velocity of the piece being ground and the polishing pad (Preston’s law [3]).

Introducing the notation:

and making a series of simple transformations, we obtain the formula for calculating the velocity $V_r$ of the point $M$ on the holder relative to the polishing pad in the form:

where:

It follows from Preston’s law [3] that the relative rate of material removal at different points of the wafers on the holder $S(r,\gamma )=S\left({\rho }_2,\gamma \right)$ is determined from the following Eq. (2):

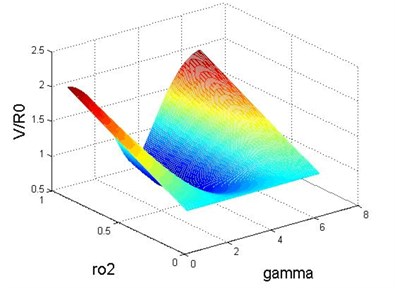
Fig. 2 shows for $f=\mathrm{}$22.7; $c=\mathrm{}$22.5; $d=\mathrm{}$3.5; $R_0=\mathrm{}$40; $b=\mathrm{}$0.4. in three-dimensional space $[gamma\left(\gamma \right),ro2\left(\rho \right),V/R_0(S\left)\right]$ the results of the calculations of the relative value of material removal on the wafers being ground depending on the coordinates of the wafer points: the angle $\gamma$, the radius $\rho$, $\omega$ at ${\omega }_0=\text{0.5}{c}^{-1}$ for the mode of the holder fixed on its axis, using the MATLAB package. Let us now consider in more detail the process of grinding in the free mode of the holder. In this case, the holder’s rotation is determined by the action of the moment $M_1$ of the frictional forces on the holder from the pad and the friction torque on the axis of the holder.

To determine ${{\rm M}}_1$, we choose a coordinate system $x\mathrm{\text{'}}y\mathrm{\text{'}}$ parallel to the system $xy$ with the origin that coincides with the center of the holder. In this system, the inertial forces acting on small parts of the holder are equal to $\mathrm{\Delta }\mathbf{F}=-\mathrm{\Delta }m{\mathbf{W}}_0$ where $\mathrm{\Delta }m$ is a part of the mass, and ${\mathbf{W}}_0$ is the acceleration of the center of the holder. These forces do not generate torque, since the holder is disk-shaped and the wafers being ground are fixed, as a rule, symmetrically without changing the position of the center of gravity that coincides with the center of the disk. Thus we conclude that the moment ${{\rm M}}_1$ is only determined by the action of the friction forces between the holder (the wafers on the holder) and the polishing pad.

A detailed study of the friction forces associated with the process of polishing is a separate large task. Therefore, to simplify, we assume that the frictional force acting on the element of the holder with the area $ds$ is equal to:

The admissibility of this representation of the frictional forces is based on the similarity of the abrasive with lubricant to a high viscosity liquid. Constant $\chi$ is proportional to its viscosity. Then we can write Eq. (4):

Fig. 2The dependence of the relative rate of material removal S on the coordinate points of the wafer

Going to the coordinate system $\xi$, $\zeta$, $\eta$ fixed relative to the holder (the axis $\xi$ is directed along $O_{4}W$, the axis $\zeta$ – along $O_{4}U$, the axes $\xi$, $\zeta$, $\eta$ form a right-handed space) and calculating the vector value $[\mathbf{r}\times {\nu }_r]$ as:

it is possible to compute the moment relative to the axis $\eta$ in the following way:

where ${\omega }_3$ is the angular speed of the holder in the coordinate system $x$ and $y$. Here, $r_0$ is the radius of the holder. Thus, in the chosen coordinate system we have:

where $J$ is the moment of inertia of the holder with the wafers. Assume further that the force of friction on the axis is the Coulomb-Amontons friction and represent the moment in the following form:

Since ${\omega }_3={\omega }_1+{\omega }_2$, where ${\omega }_2$ is the velocity of the holder relative to its axis, and ${\omega }_1$ is the angular velocity of the holder drive piece, which can be considered for the above scheme approximately equal to ${\omega }_1=A\mathrm{s}\mathrm{i}\mathrm{n}(\omega t+{\phi }_0)$, ${\omega }_0$ is the polishing pad velocity, the Eq. (6) can be rewritten as Eq. (7):

Introducing the dimensionless time $\tau =\omega t+{\phi }_0-\mathrm{\Phi }$, coordinate $x=({\omega }_2-{\omega }_0)/{\omega }_0$ and parameters $k=\lambda /\omega$, $F=A\sqrt{{\lambda }^2+{\omega }^2}/\left(\omega {\omega }_0\right)$, $a=B/\left(J{\omega }_0\omega \right)$, Eq. (7) can be rewritten as follows Eq. (8):

The phase space of Eq. (8) is two-dimensional in the coordinates ($\tau ,x$). It has a straight line $Г$ ($x=-1$), which divides the plane into three subspaces $X_{+}(x>-1)$, $X_{-}(x<-1)$ and $Г$ ($x=-1$). The motion of the image point in said subspaces is described by the Eq. (9):

Note that the motion of the image point in the subspace $Г$ ($x=-1$) takes place in time intervals ${\mathrm{\Delta }}_i\in [{\tau }_i,{\tau }_{i+1}]$ corresponding to the junction of the phase trajectories coming from the subspaces $X_{-}$, $X_{+}$.

Fig. 3 (a, b) shows the phase trajectories for different parameter values $a=$ 9 (Fig. 3(a)) and $a=$ 7 (Fig. 3(b)), respectively, at $k=$ 0.71, $F=$ 3.2.

Fig. 3Phase trajectories in the plane τ, x

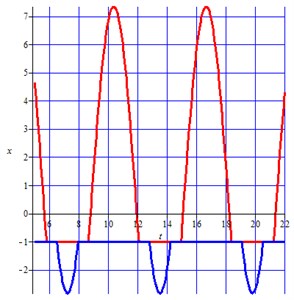
a)

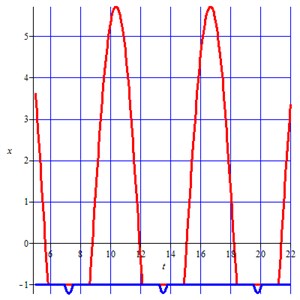
b)

What all the parameters have in common is the presence of parameter intervals ${\mathrm{\Delta }}_i$ of the holder stops, which increase with a rise of the coefficient of static friction. The rotation with the stopping of the holder begins only from some values of $a$ (see Fig. 3(b)). With the increase of the parameter $k$ the intervals are shifted to the region of the shorter times, and the oscillation amplitude decreases. In the ideal case, when we can neglect friction on the axis of the holder (${{\rm M}}_2=$ 0), it follows from Eq. (6) that with the obvious relation $(\chi r_0^4\pi /2)/\left(J{\omega }_0\right)>0$ we will obtain $\underset{t\to \mathrm{\infty }}{\mathrm{l}\mathrm{i}\mathrm{m}}{\omega }_3\left(t\right)={\omega }_0$. This means that the angular velocity of the holder ${\omega }_2$ relative to its axis is ${\omega }_2={\omega }_0-{\omega }_1\left(t\right)$. Note that for the chosen values of the parameters of the mechanism the relation ${\omega }_1=\dot{\phi }\approx 0.25\mathrm{s}\mathrm{i}\mathrm{n}(\omega t+{\phi }_0)$ holds true and hence ${\omega }_2>0$. Assuming ${{\rm M}}_2\ne 0$ and that it can be approximated by the force of friction obeying the Coulomb-Amontons law, it follows from Eq. (6) that $\underset{t\to \mathrm{\infty }}{\mathrm{l}\mathrm{i}\mathrm{m}}{\omega }_3\left(t\right)={\omega }_0-\mathrm{\Delta }\omega \text{,}$ where $\mathrm{\Delta }\omega$ is a small constant, which is equal to $\mathrm{\Delta }\omega =2{\left|M_2\right|}_{\mathrm{m}\mathrm{a}\mathrm{x}}/\left(\chi \pi r_0^4\right)$. In this case, for the steady state mode, instead of the relationship Eq. (1) we will have the following Eq. (10):