Modal and dynamic responses of the human head-neck complex for impact applications

2.1. Governing equation and finite element method

A modal analysis for the finite element head-neck model is conducted. It is reminded that carrying out a mathematical modal analysis of the head-neck system depends fundamentally on resolving the separate equation for free vibration, which can be expressed as follows:

where $\left[M\right]$, $\overrightarrow{\mathrm{\delta }}$ and $\left[K\right]$ are mass matrix, the displacement vector and rigidity matrix.

The displacement vector $\overrightarrow{\delta }$ is a function of space and time which can be written in the form:

where $\left\{\chi \right\}$ is a vector of constants providing the mode’s eigenform, whilst $\varphi \left(t\right)$ is a function illustrating the mode amplitude’s sinusoidal temporal tendency.

The following equation is fulfilled by the temporal part:

and has got the following solution:

The following equation is fulfilled by the spatial part:

The system solution consequently depends on finding the eigenvectors $\left\{{\xi }_r\right\}$ in a way corresponding to the eigenvalues ${\omega }_r=2\pi f_r$, where $f_r$ symbolizes the natural frequencies. The spacial matrix, or the eigenfrequency matrix, is defined using the following quotient:

where $\left\{{\chi }_r\right\}$ is the shape vector being connected with the eigenpulse, ${\omega }_r$ symbolizes the modal deformation in a similar way called mode shape.

2.2. Development of the 3D finite element head-neck model (FEHM)

The FEHM was developed by means of the semi-automatic method of different tissue types’ segmentation in the human head-neck complex, followed by the problem domain’s discretization into number of finite elements. In the meantime, relying on the problem’s nature, material properties need to be assigned to every finite element and boundary conditions need to be applied to the finite element model ahead of its applications in numerical simulations.

In the present work, the human skull’s geometric information is acquired from 600 axial pictures with resolution of 512 by 512 pixels, from a series of a 50th percentile volunteer’s CT and MRI scan information. A global threshold scope of Hounsfield units is selected to segment the skull’s denser bony structure from the soft tissues. Region that grows algorithm is used to filter the floating pixels or “noise” which do not join up to the skull bones when cavity fill techniques are adopted to close the small cavities in the body. The segmented skull’s connectivity is further upgraded by manual in both 3D and 2D. All the internal details, which were frequently disregarded in earlier studies, such as frontal, maxillary sinuses and sphenoidal as well as the inferior, senior and middle turbinates, are in a similar way modeled.

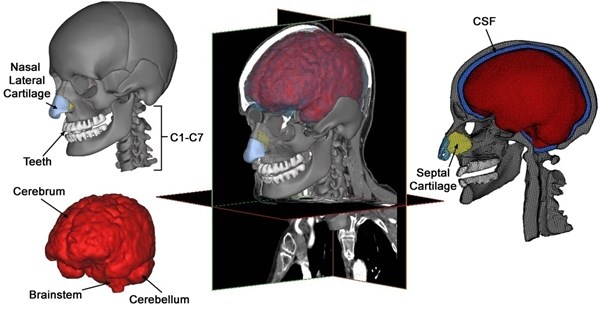
Brain segmentation is crucial for modeling head injury models’ precise brain geometry. The tools for automatic segmentation of soft tissues from CT imaging are not extremely accurate and difficult for brain tissues, although these tools are available utilizing default option threshold scope for “muscle tissue”. Consequently, MRI information of another healthy patient likewise acquired from the scanned database, is mapped and scaled to CT scan information for brain segmentation. The brain is initially segmented into just the cerebellum and cerebrum without gray and white matters’ further differentiation. Afterwards, so as to simulate the strain distribution and stress in a better way, separated representations of the ventricular structure as well as gray and white gray matters are implemented. Ventricular structures as well as the gray and white matters of the skull-brain tissues can be segmented from MRI pictures, utilizing the segmentation approach that is built by Dale et al. [18] and afterwards by Fischl et al. [19] with less manual edition. The model is composed of the cranial skull with elaborate facial bone attributes, cervical vertebrae, teeth, nasal lateral cartilages, nasal septal cartilage; brain components such as cerebellum and cerebrum and the cerebrospinal fluid separating the brain and the skull (Fig. 1).

Though meshes in normal size are most desired in modal and dynamic responses analysis, this is not potential in our numerical simulation of such complicated human head-neck model. Mesh refinement is needed by areas such as those of special interest and of elaborated attributes. An adaptive semi-automatic meshing technique is then utilized to optimize between element quality and computation efficiency. Further mesh reparation is executed to get ready for 3D mesh generation. In spite of the cavity-dominant skull’s complex geometry, it is possible to hexahedrally mesh model utilizing isomorphism technique [20], bringing about a model of 403176 hexahedral elements and 483711 nodes.

Fig. 1Various components of a subject-specific model of the human head-neck segmented from CT and MRI data

2.3. Validation of the 3D finite element head-neck model

The former section introduces details in the description and development of FEHMs. Proper validations in the viewpoint of the models’ intended uses, however, ought to be executed before their dynamic applications. A numerical model’s experimental validation, ahead of its applications, is significant and required in deciding the degrees to which a model accurately predicts the physical phenomena of the real-world. Only when this is accomplished, it does represent a powerful tool with which to correlate the biomechanical parameters that are involved in the head injury. Before analyzing the numerical results obtained with the finite element method, the reliability of the numerical results themselves could be guaranteed.

The finite element model of the human head-neck complex was validated against intracranial pressure data of Nahum et al. [21]’s cadaver experiments, with some that was validated against extra pressure history information for Trosseille et al. [22]’s long duration impact. Further, the FEHM was validated against relative displacement information between brain and skull supplied by Hardy et al. [23]. These validations involve impact force, the intracranial pressure and intracranial acceleration response. The FEHM demonstrated good agreement with post mortem human subjects’ information according to the injury prediction and biomechanical response in validation test. Apart from very good estimations in terms of injuries and biomechanical response, the model illustrated stability at severe loading circumstances. Overall results that were acquired in the validation showed improved biofidelity that was in relation to former finite element models.

2.4. Frequency spectrum of the human head-neck complex

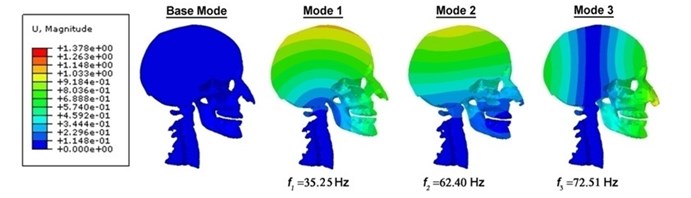
By performing modal dynamic analyses of three-dimensional mathematical model of the head-neck complex which is introduced in the former part with 25 frequency spectrums in Abaqus, related modal and an inexplicit finite element code figures are acquired in the frequency domain between 35 Hz and 360 Hz (Table 1). Resonant frequencies which were computed under the condition of the damped and undamped free vibration are listed in Table 1, and with individual fundamental natural frequencies of 35.25 Hz, 34.50 Hz, 34.23 Hz and 33.38 Hz. Additionally, there is a fluctuation within a narrow range in the inherent frequencies under the damped vibration circumstances and undamped ones, which are given in Table 1. In the 25 modal shapes, the head-neck complex’s five diverse, distinguishable mode shapes are demonstrated: a posterior-anterior flexion-extension mode about the neck of 35.25 Hz, a sideward inflexion mode of 62.40 Hz, a co-axial rotational mode of 72.51 Hz, an S-shaped posterior-anterior mode of 221.59 Hz and eventually a perpendicular translational mode of 254.04 Hz. The nasal sideward cartilages’ transverse flexion and the horizontal movement of the “mastication” or “mandible” mode which predominate after the head-neck complex’s fundamental modes in the twenty-five modals are likewise discovered. The modal shapes under the undamped vibration circumstances are the same as that under the damped ones, aside from the difference in biomechanical properties like displacement, Mises stresses of skull as well as intracranial pressure (Table 2). Table 2 also suggests the positions of vertex intracranial pressure and vertex Mises stresses of skull that is corresponding to each mode.

3.1. Comparison of fundamental frequency

The fundamental natural frequency which has been acquired from modal analysis and compared with those that were found in reported documents is one of the significant dynamic characteristics, Because of the difference in the sections involved, the reported fundamental frequencies, nevertheless, differ widely across literatures; from the few thousands for skull-only studies to the few hundred hertz in studies accounting for the brain.

In the present work, Meyer et al. [16]’s finite element study is selected for the modal responses’ comparison as it is the only study that involves the head-neck system similar to the present finite element head-neck model. In the free vibration circumstance, our computed fundamental frequency is 35.25 Hz when Meyer et al. [16] reported that the fundamental frequency is 3.01 Hz. This discrepancy in the fundamental frequency arises between the two finite element head-neck models, probably owning to the distinction in material properties and the approach in modeling, with the former factor being more likely to result in the discrepancy. With the prioritized focus on the neck injury, the head and all the cervical vertebrae of Meyer et al. [16]’s finite element model were modeled as rigid bodies, with all the individuals’ masses and inertial moment taken into consideration. Only the intervertebral discs were modeled as deformable bodies. Conversely, all the components in our finite element head-neck complex are modeled as deformable bodies with the capability to disperse outside energy by means of deformation. Besides, unlike the rigid head of Meyer et al. [16]’s finite element model, our finite element head model comprises skull, subarachnoid space, brain tissues as well as cartilages. By having the various kinds of different components in the head model, particularly with viscous intracranial content’s lumped mass, it is expected that the fundamental frequency of the multi-components system will be lower than that of a one-component system as extra natural frequencies appear on the one-component system’s frequency spectrum.

In conclusion, our computed fundamental frequency of 35.25 Hz is reasonably close to the reported scope of 20 to 30 Hz in previous studies. Though the present value is higher than the few hertz as reported by Meyer et al. [16] and lower than the analytic head-neck complex’s few hundred hertz, it is still considered to fall within a reasonable range ever since the consensus on this dynamic characteristic stays debatable.

3.2. Effect of damping on resonant frequencies and biomechanical responses

Most former studies revealed that the modal dynamic analysis of the human head-neck complex adopted traditional frequency extraction approach and disregarded the influence of damping factor. As has been noted, a few damping factors are introduced in the matrix eigenvalue and eigenvector analysis. As the value of damping factor extends, it is expected that resonant frequencies will be lower. A considerable fluctuation of natural frequencies is noticed under the damped vibration circumstances and undamped ones. In particular, several brain tissues’ dynamic responses exist at the same time in the lower natural frequencies. Since every single component resonates by itself in the medium modes, these distinguish under damped cases and undamped ones fall down. Nevertheless, with the number of resonance modes grows greatly, the distinguish appears to be expanded by the growth of damping factor, in all probability owning to the complex combination and addition of shearing, bending and torsional modes in the brain tissues.

With regard to the influence of the biomechanical properties such as intracranial pressure (ICP) and Mises stresses of skull, damping factor in a similar way seems to have substantial influence on its significances with special resonance modes, as shown in Table 2. That damping factors are introduced in the matrix eigenvalue and eigenvector analysis has greatly reduced maximal Mises stresses of the skull and the maximal ICP, of up to 74.1 % and 84.8 % individually, in particular modes, as shown in Table 2 and Figs. 3-4. These modes with the maximal deviation in vertex skull stresses and ICP between the damped models and undamped are discovered and displayed in Fig. 3 and Fig. 4. It can be found that vertex ICP changes greatly in the principle few modals while the human head-neck either rotates or flexes. The jaw or mandible opens greatly of the continuous modals such as 14th, 18th, 21st, 22nd or 23rd, therefore making the cranium have a relative movement to the brain. The skull-brain relative motion, as previously, brings about gradient variation of ICP [25]. The existence of damping factor will impede the skull-brain movement and bring the ICP down subsequently. In a similar way we notice that the skull head’s vertex stress deviates greatly where the human neck undergoes the maximal Mises stresses. Owning to the lack of muscle tissues and ligaments the “over-stiff” neck in the head-neck model can bring about much higher vertex Mises stresses of the undamped finite element model. Nevertheless, these modal and dynamic responses are reduced under the damped circumstances as a result of the connection of the stiffness matrix and the damping matrix. Therefore, it is of great significant to identify the suitable damping factor in reckoning modal and dynamic responses. Likewise, it ought to be noticed that this damped influence turns strong while damping factor exceeds the value of 0.2, this implies that greater damping factor will not affect the results of dynamic analyses. As increasing damping factor to the value exceeds 0.2 is discovered to produce magnifying influence on lowering frequencies of higher order modals and eliminating effects in reducing vertex modal dynamic features. Consequently, it comes to a conclusion that there is a reverse proportionality between damping factor effect on resonance frequencies and on biomechanical responses.

Fig. 3Contour plots of biomechanical responses of human finite element head-neck model, indicating their vertex values at special important modes

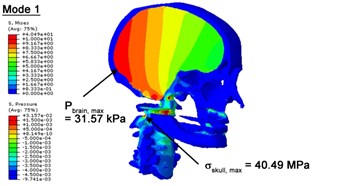
a) 1st mode

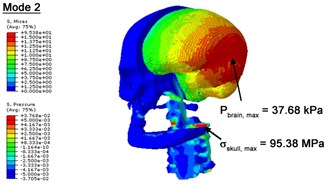
b) 2nd mode

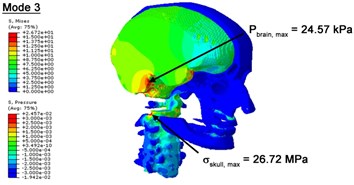
c) 3rd mode

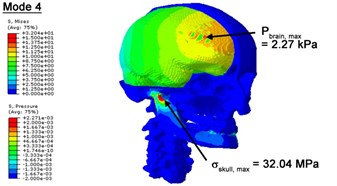
d) 4th mode

3.3. Limitations

The flexibility of the neck, which are highly dependent of the elasticity of the neck ligaments, muscles and intervertebral discs, may not be accurately simulated in present work on account of the lack of the neck ligaments, muscles and intervertebral discs in our model. As a matter of fact, the cervical vertebrae are modeled as deformable bodies, with master-slave restriction between each pair. However, similar to other numerical simulation, the present study made presumption and this accustomed simplification in finite element modeling because its prioritized focal point is to inquire into the effect of damping utilizing complex harmonic eigenvalue modal analysis in which vectors of the computed mode shapes are always not the absolute movement but the arbitrarily relative one. Furthermore, the model provides a reasonable estimation of the mode shapes and natural frequencies, comparable to those in the literature. It, however, shall be emphasized that a more elaborated neck model’s incorporation would be required in the future study for a more comprehensive and precise analyses of neck dynamics.

Fig. 4Contour plots of biomechanical responses of human finite element head-neck model, indicating their vertex values at special important modes

a) 5th mode

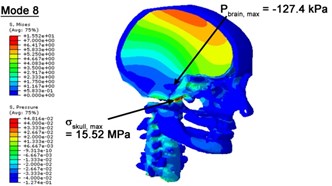
b) 8th mode

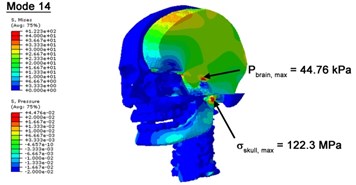
c) 14th mode

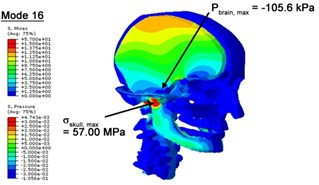
d) 16th mode

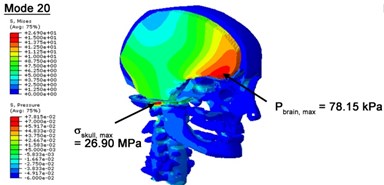
e) 20th mode

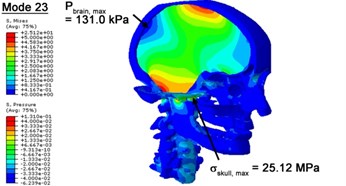
f) 23rd mode