Abstract
The effectiveness of single-leg crossties that were anchored by heads in reinforced concrete columns was assessed. Seven reinforced concrete columns were tested under reversed cyclic loading with a 10 % axial load of the nominal axial capacity of the columns. Four columns were designed to fail in a flexural mode, and three columns were designed to fail in a shear mode. The main variable was the anchorage type of crossties: conventional crossties that were anchored with 135° and 90° hooks, crossties that were anchored with one-side head and one-side 180° hook, and crossties that were anchored with double heads. The test results indicate that the hysteretic behavior of the columns with crossties that were anchored by double heads or one-side head was similar or superior to the columns with conventional crossties anchored by hooks in terms of ductility and energy dissipation. After the cover concrete spalled, the 90° hooks inevitably opened and the column longitudinal bars buckled. However, the heads could delay the buckling of the column bars and the columns could maintain their capacities until 8 % drift ratio for the columns that were designed to fail in a flexural mode. For the columns that were designed to fail in a shear mode, all columns showed similar behaviors and had identical strengths. The columns with the headed crossties had smaller crack widths than the columns with conventional crossties because the headed crossties well confined the core concrete under severe shear deformation. The test results show that headed crossties can effectively confine the column bars and core concrete of the columns: therefore, the ductility and energy dissipation capacity of the columns were improved.
1. Introduction
Transverse reinforcement in reinforced concrete (RC) columns provides confinement to the core concrete, prevents premature buckling of the longitudinal reinforcing bars, and serves as shear reinforcement [1]. Under seismic loading, the spalling of the cover concrete can be extensive and the strength and ductility of RC columns significantly depend on the effectiveness of the transverse reinforcement on the three roles.
The use of headed bars as crossties offers many advantages. Heads that are properly connected and adequately sized can enable the development of tensile strength of the bar at the bar extremities, unlike crossties with standard 90° and 135° hooks. Using headed reinforcing bars reduces reinforcement congestion and can make fabricating the reinforcing cages easier. In addition, the contribution of the head size and rigid head-to-bar connection can increase the reinforcement’s confining effect [1-3]. However, the cost of the headed shear reinforcement is obviously higher than that of the crossties with standard hooks. This disadvantage may be mitigated by using crossties with one-side head and one-side 180° hook. The 180° hook has the same anchorage capacity to the head because the 180° hook does not open under reversed cyclic loading. The crossties with one-side head and one-side 180° hook can be fabricated as easily as the crossties with double heads. Moreover, the 180° hook can reduce the interference of hook’s tail on the column bars. Until now, the structural behavior of the crossties with one-side head and one-side 180° hook has not been reported.
Although there are a number of experimental studies on columns with hoops, ties, and crossties, there are only a few experimental results on columns [1, 4] with headed reinforcement. Moreover, only double headed bars were used in the previous studies [1, 3, 4], and the effectiveness of headed bars was investigated in columns or walls that were subjected to monotonic axial loading [1, 3] or in columns that were designed to fail in a flexural mode subjected to cyclic loading [4]. The reported study in this paper is aimed at investigating the confining effect of double or one-side headed bars that serve as crossties in RC columns, which are subjected to cyclic lateral loading with a constant axial load. In addition, the columns were designed to fail in two types of flexural and shear modes to clearly assess the shear resistance of headed bars.
2. Test program
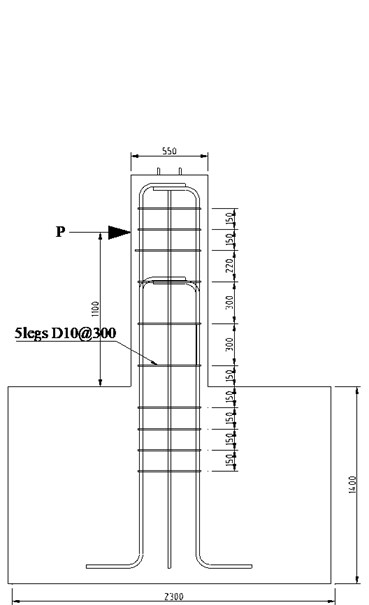
Seven reinforced concrete columns were tested under reversed cyclic loading with a 10 % axial load of the nominal axial capacity of the columns. Four columns were designed to fail in a flexural mode (hereafter flexural tests), and three columns were designed to fail in a shear mode (hereafter shear tests). All columns had a cross section of 800×550 mm as shown in Fig. 1, and the distances between the critical points and the loading points were 2,200 mm and 1,100 mm for the flexural tests and shear tests, respectively, as shown in Fig. 2. To access the effectiveness of crossties, the number of crossties was designed to be more than the legs of a hoop and, therefore, at least three crossties were required in a layer. The sectional dimension was determined to place three crossties in a layer. The aspect ratios of columns are 4 and 2 for the flexural tests and shear tests, respectively. The aspect ratios and vertical spacing of transverse reinforcement were determined to ensure the intended failure mode of flexure or shear.
Fig. 1Column sections of the flexural tests
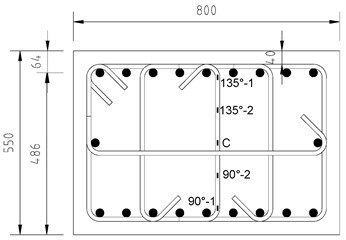
a) FC
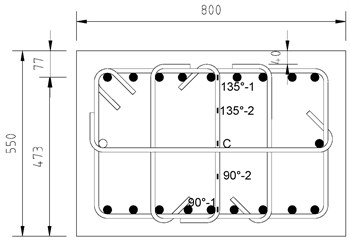
b) FC-1
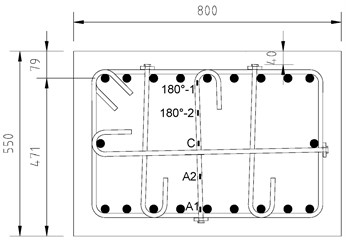
c) FMC

d) FM
The main variable was the anchorage type of crossties as shown in Fig. 1: FC and SC had conventional crossties that were anchored with 135° and 90° hooks; FC-1 had identical conventional crossties as FC and SC, but the hooks enclosed the hoops instead of enclosing the longitudinal bars; FM and SM had double headed crossties that replaced conventional crossties, and the heads enclosed the hoops; and the crossties of FMC and SMC were anchored by one-side head and one-side 180° hook. In the specimen nomenclature, F and S represent the flexure and shear tests, respectively; C indicates a conventional anchorage using standard hooks; and M indicates a mechanical anchorage using heads. The flexural tests consist of four specimens of FC, FC-1, FMC, and FM and the shear tests consist of three specimens of SC, SMC, and SM. There is a notably small difference between FC and FC-1 that the conventional hooks enclose either the longitudinal bars or the hoops. This difference may affect the effectiveness of confining the core concrete and preventing buckling of the longitudinal bars; therefore, only FC-1 was included in the flexural tests.
Fig. 2Elevations of the specimens

a) Flexural tests
b) Shear tests
The headed shear reinforcement conformed to ASTM A970/A970M-15[5] and had circular heads. The diameters of the heads were 35 and 40 mm for 10 and 13 mm-diameter bars, respectively, so that the net bearing area of the heads should be almost 9Ab according to 20.5 of ACI 318-14 [6]. The thicknesses of the heads were 6 and 8 mm for 10 and 13 mm-diameter bars, respectively. The attachment of the heads to the re-bar was accomplished through threads conforming to 1.1.3 of ASTM A970-15/A970M-15.
Twenty longitudinal bars of 22 mm-diameter (ρ= 1.76 %) were placed for the flexural tests as shown in Fig. 1 and twenty longitudinal bars of 25 mm-diameter (ρ= 2.30 %) were placed for the shear tests. The measured yield strengths of 22 mm and 25 mm bars were 412 and 409 MPa, respectively. As transverse reinforcement, five legs of 13 mm-diameter bars were placed at 140 mm vertical spacing in the flexural tests and five legs of 10 mm-diameter bars were placed at 300 mm vertical spacing in the shear tests as shown in Figs. 1 and 2. The measured yield strength of the 10 mm and 13 mm bars were 509 and 388 MPa, respectively. The wide vertical spacing of the shear tests was designed for the intended shear failure. The specimens conformed to ACI 318-14 [6] except the vertical spacing of transverse reinforcement of the shear tests. The compressive strength of the concrete was 30.6 MPa on the test date.
The clear cover was 40 mm which was measured to the head according to 20.6.1.3 and R25.4.4.1 of ACI 318-14. Due to the head, the effective depths were changed as shown in Fig. 1. The effective depth of FMC and FM was reduced to 471 mm and the difference is 3.09 % compared with 486 mm of FC. Consequently, the flexural strengths of the columns were also changed which were summarized in Table 1. The design strength of FMC and FM is 403 kN which is 97.6 % of the design strength of FC. The difference of the design strength is only 2.4 % which is less than the difference of the effective depth because of the existence of axial force.
Fig. 3Test setup for the flexural tests (In side view, the strong frames are invisible for clarity)
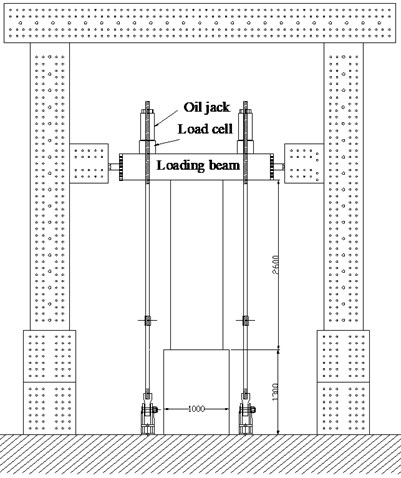
a) Front view

b) Side view
Fig. 4Loading sequence
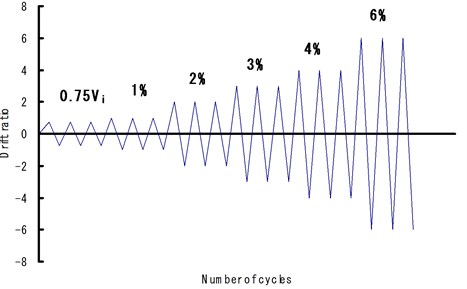
Reversed cyclic loading was applied to the specimens with a 10 % axial load of the nominal axial capacity of the columns as shown in Fig. 3. The lateral cyclic load was applied under displacement control with three reversed cycles at each specified drift ratio in accordance with ACI 374.1-05 [7] as shown in Fig. 4. The lateral load was applied to the weak axis of the columns considering the actuator capacity of 2,000 kN. The test measurements included the applied actuator load and column deflection at the loading point. The strains on the crossties were measured and the measuring points were marked in the column sections as shown in Fig. 1.
3. Results of flexure tests
3.1. Overall behavior
All specimens showed typical flexural behaviors such as the sequence of [flexural cracking]-[yielding of longitudinal bars]-[spalling of cover concrete]-[buckling of compression longitudinal bars]. In all specimens, the initial flexural cracks occurred at the 0.5 % drift ratio. The longitudinal bars in tension yielded and diagonal shear cracks occurred at the 1 % drift ratio. The lateral load at the 4 % drift ratio was the maximum for all specimens. After the maximum load, the concrete covers began to be damaged and the buckling of longitudinal bars began in the compression side, which caused the strength degradation of the specimens. The strength degradation of FC progressed more rapidly than that of FM because the 90° hooks opened toward the concrete cover and the compression bars of FC buckled earlier than those of FM. Fig. 5 shows the crack patterns of FC and FM specimens at the 6 % drift ratio. Because the 90° hooks of FC opend, the cover concrete of FC severely spalled and the crack width was wider than FM. Failure was defined as 15 % reduction of the maximum load. The specimen FC failed at 6 % drift ratio, whereas the specimen FM failed at 8 % drift ratio. The other specimens of FC-1 and FMC behaved similarly to FC.
Fig. 5Crack patterns of FC and FM at 6 % drift ratio

a) FC at 1st ‒6 %

b) FC at 2nd +6 %
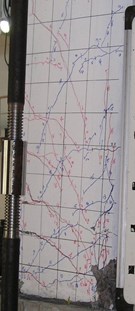
c) FM at 1st ‒6 %

d) FM at 2nd +6%
Fig. 6Buckling of the longitudinal reinforcing bars
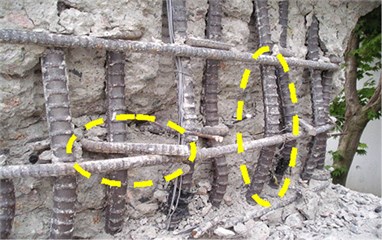
a) FC

b) FM
The buckled longitudinal bars were shown in Fig. 6 where the cover concrete was intentionally removed. For FC, the 90° hooks obviously opened as shown in Fig. 6(a) and they could not prevent the longitudinal bars from buckling. The buckling length of the longitudinal bars increased twice and the load resistance capacity rapidly decreased. However, the heads in FM could effectively enclose the hoops until the ultimate state as shown in Fig. 6(b) and the buckling length of the initial value was maintained until the ultimate state. This result made the different behaviors between FC and FM.
3.2. Relationship between load and displacement
The relationships between lateral load and lateral displacement are shown in Fig. 7. All specimens showed a typical flexural behavior; in particular, the yielding and maximum loads were notably similar for all specimens because the specimens conformed to the seismic-design requirements of ACI 318-14. No significant pinching was observed until failure because of the flexural behavior.
Fig. 7Relationships between lateral load and drift of the flexural tests

a) FC
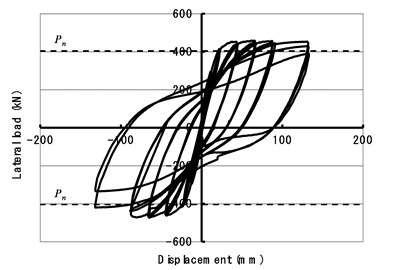
b) FC-1
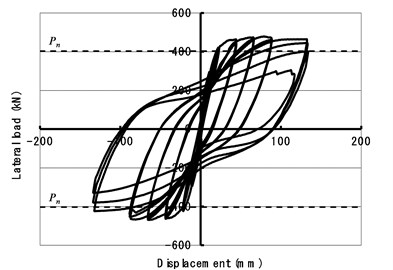
c) FMC
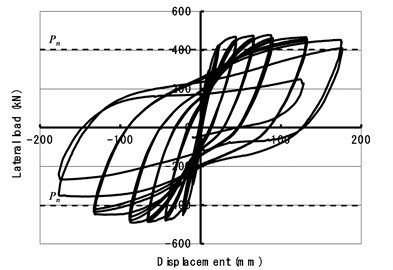
d) FM
Table 1Summary of the test results
Specimens | Pye, kN | Pyt, kN | Pmax, kN | Pn, kN | Pmax/Pn | ∆, mm | , mm | ||
Flexural test | FC | 434.1 | 393.9 | 471.4 | 413.0 | 1.20 | 25.8 | 131.8 | 5.11 |
FC-1 | 412.6 | 393.9 | 458.6 | 404.0 | 1.16 | 21.9 | 132.8 | 6.06 | |
FMC | 411.6 | 393.9 | 479.2 | 403.0 | 1.22 | 22.6 | 133.8 | 5.92 | |
FM | 423.4 | 393.9 | 478.2 | 403.0 | 1.21 | 21.5 | 176.2 | 8.20 | |
Shear test | SC | 1,129.9 | 965.3 | 1,136.8 | 967.4 | 1.18 | 20.3 | 33.0 | 1.62 |
SMC | 1,004.5 | 965.3 | 1,146.6 | 966.4 | 1.19 | 16.8 | 33.1 | 1.97 | |
SM | 1,057.4 | 965.3 | 1,102.5 | 966.4 | 1.14 | 15.2 | 33.4 | 2.20 | |
* and are measured and calculated yield loads, respectively, is a measured maximum load, is a nominal strength based on actual material properties, and and are lateral displacements at yield load and failure load, respectively. | |||||||||
There is little difference between specimens up to approximately 130 mm (6 % drift ratio) in the hysteretic curves. FC failed at the 1st cycle of −6 % drift ratio; FC-1 maintained the load until the complete 2nd cycle of 6 % drift ratio; FMC maintained the load until the complete 3rd cycle of 6 % drift ratio; and FM maintained the load until the complete 2nd cycle of 8 % drift ratio. The measured and calculated strengths and the measured yield and ultimate displacements of all specimens are summarized in Table 1. The ductility factor, which is defined as the ratio of ultimate displacement () to yield displacement (), of FM is much higher than that of FC as shown in Table 1. Although the anchorage types of crossties did not affect the load-carrying capacity, they affected the ductility of the columns. Considering the excellent observed behavior of FM and FMC, it is concluded that the heads were more effective as anchorages of the crossties than the conventional hooks in RC columns.
The envelope curves of FC and FM are shown in Fig. 8. The flexural cracking, bar yielding, and maximum loads are almost identical. However, the longitudinal bars in FC buckled before those in FM did because the 90° hooks opened. The buckled longitudinal bars made the transverse reinforcement yielded; finally, the columns lost the load resistance.
3.3. Energy dissipation comparison
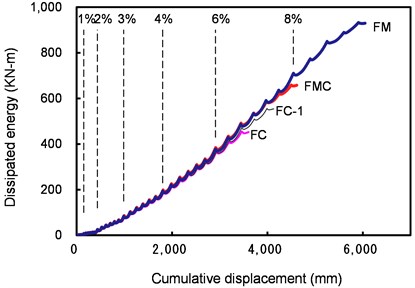
The cumulative displacement-dissipated energy curves are shown in Fig. 9. The -axis represents the cumulative displacement that the specimen experienced, whereas the -axis represents the dissipated energy. Until the 1st cycle of 6 % drift ratio (approximately 3000 mm cumulative displacement), all specimens had identical energy dissipation capacities. Although FC failed at the 1st cycle of 6 % drift ratio, FM maintained the load-resisting capacity until the 8 % drift ratio; therefore, FM dissipated more energy as shown in Fig. 9. The energy dissipation capacities of FMC and FM show again that the heads can effectively confine the core concrete and prevent the longitudinal bars from buckling.
Fig. 8Envelope curves of the relationship between lateral load and drift of FC and FM specimens
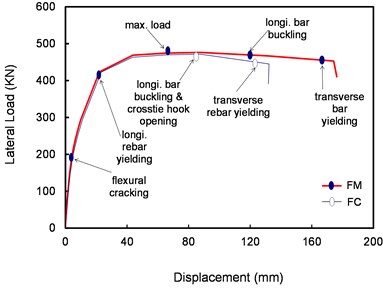
Fig. 9Relations between energy dissipation and cumulative displacement of the flexural tests
4. Results of shear tests
4.1. Overall behavior
The SC, SMC and SM specimens are columns with low volumetric ratios of transverse bars, which were designed to fail in a shear mode. The behaviors of all columns are notably similar except the crack patterns. The initial flexural cracks occurred at the first loading. Diagonal shear cracks began at the 0.5 % drift ratio. Although the columns were designed to fail in shear, the longitudinal bars yielded. Immediately after the longitudinal bars yielded, the maximum lateral load was developed at the 2.0 % drift ratio, which was followed by diagonal cracks propagating to the top of the column and the decrease in strength. At the 3 % drift ratio, the specimens significantly lost the load resistance. The shear failure mode dominated in all shear specimens.
Fig. 10 shows the crack patterns at 1.5 % and 3.0 % drift ratios. While many cracks with small widths are widely spread in SM, a few large cracks are concentrated in SC. It is considered that the headed bars confined the core concrete so effectively that only small cracks occurred at the overall column face.
4.2. Relationship between load and displacement
The relationships between lateral load and lateral displacement of the shear tests are shown in Fig. 11. The load-displacement relations indicate that all specimens showed a limited ductility such that all columns immediately failed after the maximum loads were reached. The yielding and maximum loads were notably similar for all specimens as shown in Table 1. Because of shear cracks, significant pinching was observed from the first loading.
Fig. 10Crack patterns of shear tests at the 1st cycles of 1.5 % and 3.0 % drift ratios
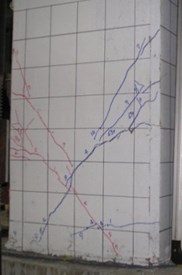
a) SC at 1.5 %

b) SMC at 1.5 %
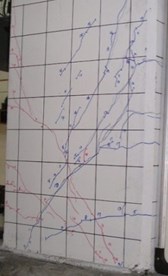
c) SM at 1.5 %
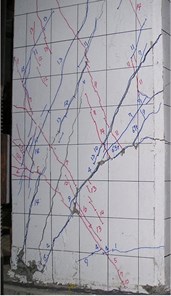
d) SC at 3.0 %

e) SMC at 3.0 %

f) SM at 3.0 %
All specimens failed at the first cycle of −3 % drift ratio. All specimens had almost identical yield and maximum strengths. After the longitudinal bars yielded, the maximum flexural strengths were developed. Shear failure was dominant in all columns but the specimens had higher flexural strengths than the nominal flexural strength by at least 14 % as shown in Table 1. The ductility factors () are 1.62 and 2.20 for SC and SM, respectively. Considering the small values of the ductility factors, there is no meaningful difference in ductility between SC and SM.
The envelope curves of the shear tests are shown in Fig. 12. The flexural cracking, diagonal cracking, bar yielding, maximum, and failure loads are almost identical. The envelope curves show that the double headed bars and one-side headed bars can be effective alternatives to the standard hooked bars as crossties in RC columns.
Fig. 11Relationships between lateral load and drift of the shear tests
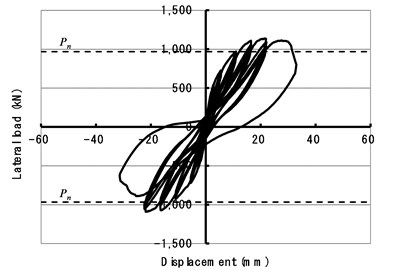
a) SC
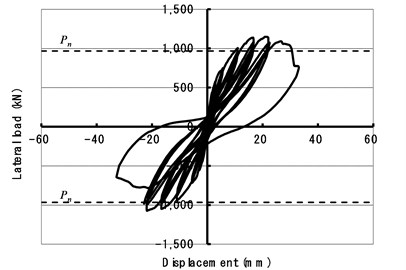
b) SMC
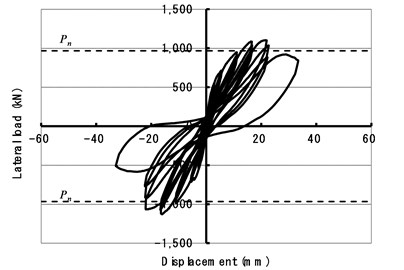
c) SM
Fig. 12Envelope curves of the relationship between lateral load and drift of the shear tests
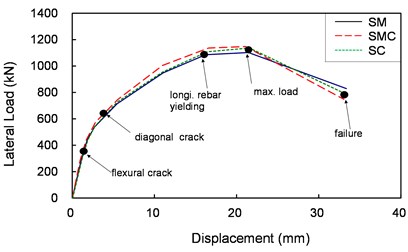
Fig. 13Relations between energy dissipation and cumulative displacement of the flexural tests
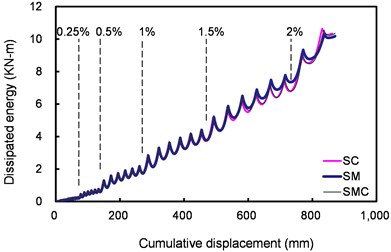
4.3. Energy dissipation comparison
The cumulative displacement-dissipated energy curves of shear tests are shown in Fig. 13. Because all specimens show similar hysteretic behaviors, the dissipated energies of all specimens were almost identical.
4.4. Strains of transverse reinforcement
Fig. 14 shows the strains that were measured at the crossties. The measuring points are indicated in Fig. 1. The -axis represents the cumulative displacement that the specimen experienced and the -axis represents the strains. The crossties of SC with conventional hooks only yielded at the center, so the strains at both ends near the 90° and 135° hooks were relatively low. However, the crossties of SMC and SM yielded at both ends and the center as shown Fig. 14(b) and (c) at the 0.5 % drift ratio. The measured strains show that the heads are more effective to develop the design strength of crossties than the conventional hooks. In addition, the crack patterns can be explained with the measured strains, such that the large cracks that are observed in SC can be attributed to the strain distribution.
Fig. 14Relations of the strain of a crosstie and cumulative displacement of the shear tests
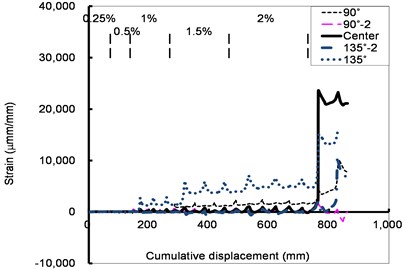
a) SC
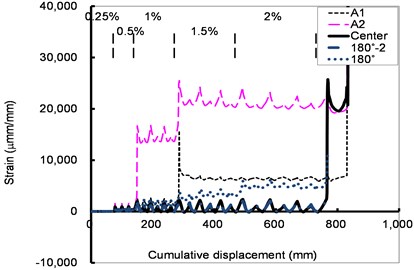
b) SMC
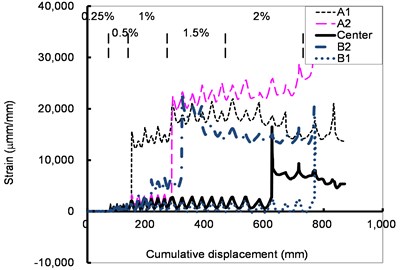
c) SM
5. Conclusions
Based on the experimental investigation on crossties that are anchored by heads in reinforced concrete columns, the following conclusions can be drawn:
1. The columns with double or one-sided headed shear reinforcement exhibit superior behavior in terms of ductility and energy dissipation capacity compared to those with conventional crossties.
2. The columns with headed shear reinforcement have approximately identical flexural and shear strengths as the columns with conventional crossties.
3. The column with double headed shear reinforcement that was designed to fail in a flexural mode maintained its flexural capacity until 8 % drift ratio, whereas the column with conventional crossties failed at 6 % drift ratio. After the cover concrete spalled, the 90° hooks inevitably opened and the column longitudinal bars buckled. However, the heads could delay the buckling of the column bars up to 8 % drift ratio.
4. Whether the hooks of crossties enclose the longitudinal bars or the hoops did not affect the strength and ductility of columns.
5. The crossties with one-side head and one-side 180° hook exhibit almost similar behavior to the crossties with double heads. The crossties with one-side head and one-side 180° hook can be a good alternative to the crossties with double heads.
6. For the columns that were designed to fail in a shear mode, the columns with the headed crossties had smaller crack widths than the columns with the conventional crossties because the headed crosstie well confined the core concrete under severe shear deformation.
References
-
Mitchell D., Cook W. D., Liu J. Confinement of columns and wall boundary elements using headed bars. Journal of Structural Engineering, Vol. 140, Issue 3, 2014.
-
Ghali A., Youakim S. A. Headed studs in concrete: state of the art. ACI Structural Journal, Vol. 102, Issue 5, 2005, p. 657-666.
-
Dilger W. H., Ghali A. Double-head studs as ties in concrete. Concrete International, Vol. 19, 6, p. 59-66.
-
Youakim S. A., Ghali A. Ductility of concrete columns with double-head stud. ACI Structural Journal, Vol. 99, Issue 4, 2002, p. 480-487.
-
ASTM A970/A970M-15, Standard Specification for Headed Steel Bars for Concrete Reinforcement. ASTM International, 2015.
-
Building Code Requirements for Structural Concrete (ACI 318M-14) and Commentary (ACI 318RM-14). American Concrete Institute Committee 318, 2014.
-
Acceptance Criteria for Moment Frames Based on Structural Testing and Commentary. American Concrete Institute Committee 374, 2005.
-
ASMT A970-15: Standard Specification for Headed Steel Bars for Concrete Reinforcement. American Society of Testing and Materials, 2015.
About this article
This research was supported by the Basic Science Research Program through the National Research Foundation of Korea (NRF) funded by the Ministry of Education, Science and Technology (No. 2011-0013828). The authors wish to thank Boo Won B.M.S. Co. Ltd., Korea, for providing the headed bars used in the tests.
