Numerical simulation of buried pipeline subjected to blast seismic waves

2.1. Finite element model

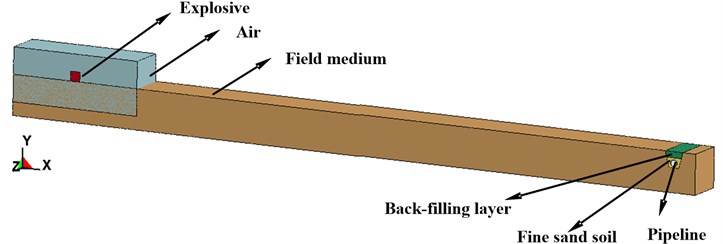
In the present paper, the mechanical performance of buried pipelines subjected to blast wave is studied numerically using nonlinear finite element code LS-DYNA. Considering the symmetries of the geometrical model, to save computation time, a half of the system was modeled as shown in Fig. 1. The ALE model involves six different material types. Eulerian meshes were generated for the explosive and the air. On the other hand, the Lagrangian meshes were used to model the field medium, the back-filling layer, the fine sand soil and buried pipelines. The model was constructed with Solid 164 solid element and g-cm-us unit. The material of the pipeline is X70, with a diameter 1219 mm and wall thickness 26.4 mm. The explosive used in the analysis was emulsion explosive. The distance between the pipeline and the explosion center was around 50 m. The finite element model is shown in Fig. 1.

Fig. 1Finite element model

2.2. Material behavior

The explosive charge was modeled using the high explosive burn material model and the Jones-Wilkin-Lee (JWL) equation of state (EOS). The JWL equation of state defines the pressure as a function of the relative volume, $V$ and initial energy per volume, $E$, such that:

where $A_1$, $R_1$, $B_1$, $R_2$ and $\omega$ are material constants, $P$ is the pressure, $V$ is the relative volume of detonation product and $E$ is the specific energy with an initial value of $E_0$. The emulsion explosive C-J parameters and the JWL equation of state parameters: ${\rho }_e=$0.6 g/cm³, $D=$6.93 km/s, $p_{CJ}=$1.5 GPa, $A_1=$132.8 GPa, $B_1=$0.439 GPa, $R_1=$5.3, $R_2=$1.2, $\omega =$0.21, $E_0=$3.2×10⁹ J/m³, respectively.

Material Type 9 of LS-DYNA (*MAT_NULL) is used to model the behavior of the air. Air mass density ${\rho }_0$ and initial internal energy $e_0$ are 1.29 kg/m³ and 0.25 J/cm³, respectively.

There are three different kinds of soils used in the analysis. In order to simulate the behavior of soil in this research, a plastic hardening model was used based on the soil properties. The yield stress and the strain rate has the following relationship:

where $C$, $P$ are the Cowper-Symonds strain rate parameters, respectively; $E_y$ is Young modulus; ${\sigma }_0$ is the initial yield stress; $\dot{\epsilon }$ is applied strain rates; $E_p$ is the plastic hardening modulus. The parameters of the model can be seen in [8].

The X70 pipeline material has been modeled using the Johnson-Cook (JC) constitutive relation and the Gruneisen equation of state. The JC model accounts for isotropic hardening, strain rate sensitivity and thermal softening. The model defines the yield stress ${\sigma }_y$ as:

where $A$, $B$, $C$, $n$ and $m$ are the material parameters determined by experiments. ${\dot{\epsilon }}^{\mathrm{*}}$ is the dimensionless effective strain rate for and $T^{*}$ is the homologous temperature. The parameters of the X70 pipeline can be seen in [9].

2.3. Finite element formation

The damage of the buried pipeline depends mostly upon the stress state. So the analysis of stress distribution can be helpful to the vibration analysis. The wave propagation progress and vibration of the pipeline are studied through numerical simulation.

Fig. 4 shows the stress distribution of buried pipeline at different times after explosion. From the figure we can see, the blast waves arrive to buried pipeline at $t=$ 3.3 ms. Then shock waves propagate to the end side of the pipeline. At the time $t=$ 11.9 ms, the stress waves travel through the entire pipeline. At this time, the bottom earth generates a resistance to prevent the vibration of the pipeline and produce the stress concentration at the bottom face of the pipeline. With the vibration of the pipeline, the stress concentration area keeps decreasing. At $t=$ 70 ms, there is little stress left on the surface of the pipeline.

Fig. 2Stress distribution of buried pipeline at different times

a)$t=$ 3.3 ms

b)$t=$ 8.4 ms

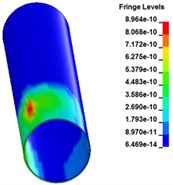
c)$t=$ 9.4 ms

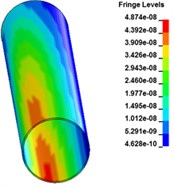
d)$t=$ 11.9 ms

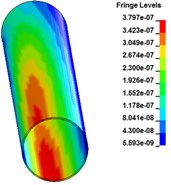
e)$t=$ 12.3 ms

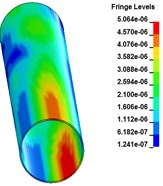
f)$t=$ 14.8 ms

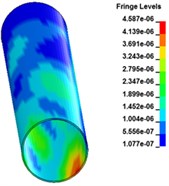
g)$t=$ 30 ms

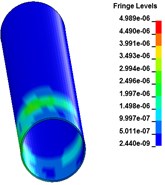
h)$t=$ 70 ms

3.1. Velocity analysis

After explosion, the middle points of the pipeline are closest to the explosion center. The vibration of the middle points of the pipeline need to be observed intensively. So we choose four points on fracture surface, namely A, B, C and D to analysis the vibration. The positions of the points are shown in Fig. 3. The velocity of the four points are analyzed.

Fig. 3The cross section of the pipeline

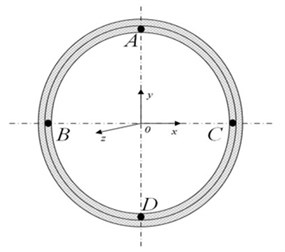
Fig. 4 shows the velocity-time curves of the four points at the cross section. The distance from the explosion center is 50 m, and the buried depth 1.2 m. From the figures we can see, when the stress wave propagates to point A, the body wave contribution most to the vibration velocity in the $x$ direction and $y$ direction. The amplitude and scope are almost the same in the two directions. The peak velocities of point A and B are 1.17 cm/s and 1.98 cm/s in the reverse $x$ direction. The vibration velocity in the $z$ direction is small and can be ignored. On the other hand, the peak velocities of point C and D are 0.69 cm/s and 2.13 cm/s in the reverse y direction. The velocity in the $z$ direction can also be ignored.

Fig. 4Velocity-time curves of the points at the cross section

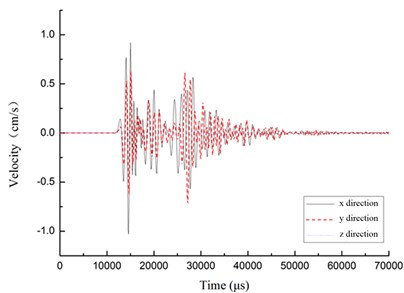
a) Point A

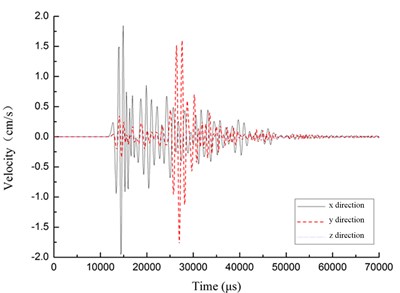
b) Point B

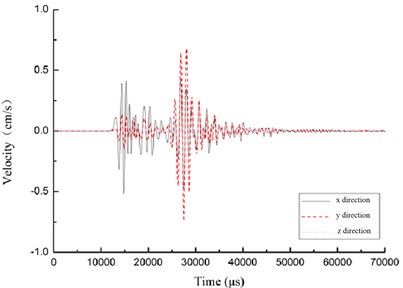
c) Point C

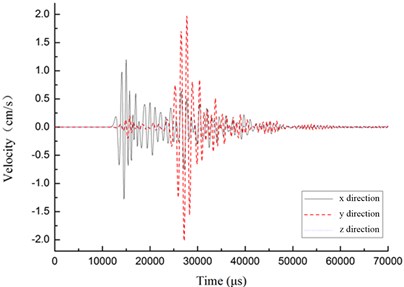
d) Point D

Fig. 5Peak velocity curves in radial direction

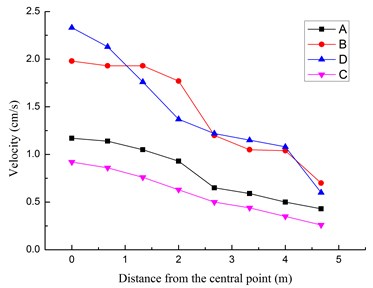
Fig. 6Peak velocity curves in circumferential direction

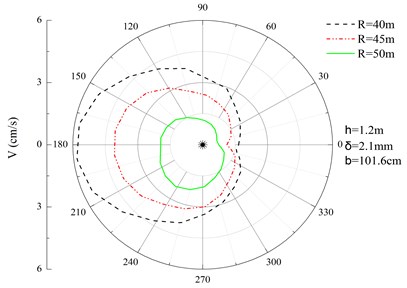
Fig. 5 shows the radial peak velocity curves of four points. The figure shows that with the increase of the distance to the end of the pipeline, the peak velocity keeps decreasing. At the distance of 4.7 m from the central point, point C has the smallest velocity 0.26 cm/s of the four points, while point B and D have almost the same velocity values. Fig. 6 shows the distribution of peak velocity in circumferential direction at the cross section. From the figure we can see, with the increase of the distance from the explosion center, the peak velocity shows downtrend. The largest velocity appears at the left side of the pipeline and the smallest velocity appears at the right side.

3.2. Influence of buried depth

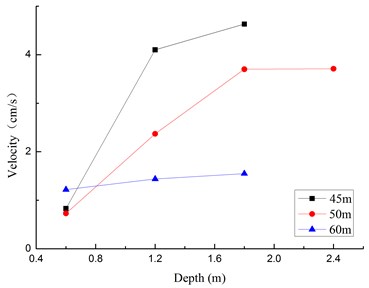
Figs. 7-9 show the peak velocity curves at the middle point of the pipelines with the distance from the explosion center 45 m, 50 m and 60 m, respectively. From the figures we can see that circumferential vibration velocity increase with the increase of buried depth. The differences of the peak velocities among different depth decrease with the increase of the explosion distance. At the distance of 60 m, the peak velocities among different depth become almost the same.

Fig. 10 shows the relationship between circumferential vibration velocity and the buried depth. From the figure we can see that the vibration velocity decrease with the increase of the distance from the explosion center. At the buried depth of 0.6 m, the peak velocity change little with the increase of the depth.

Fig. 7Peak velocity curves at the distance of 45 m

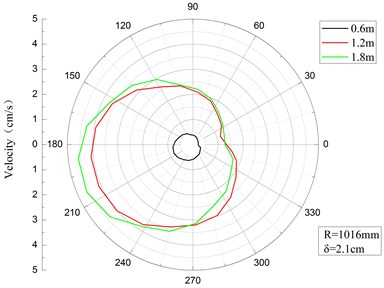
Fig. 8Peak velocity curves at the distance of 50 m

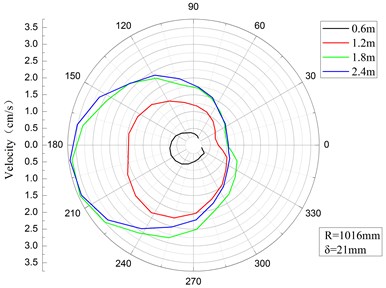
Fig. 9Peak velocity curves at the distance of 60 m

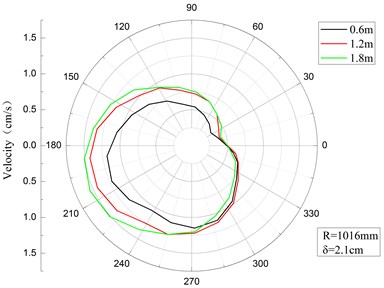
Fig. 10Relationship between peak velocity curves and buried depth