Application and measurement of underwater acoustic reciprocity transfer functions with impulse sound sources

2.1. Reciprocity principle

For the transmission of structure vibration and radiation noise, the reciprocally relation shows that when keeping the surrounding constant and driving some exciting force ($f_1$) on the underwater structural objects, the ratio between the far-field pressure ($p_2$) and this exciting force equals to the ratio between the vibration velocity ($v_1$) at this point on structure due to a far-field source and the source volume velocity ($q_2$):

The pressure $p_2$, exciting force $f_1$ and response velocity $v_1$ in Eq. (1) can be measured directly, but the volume velocity, $q_2$ need to be calculated. The Eq. (2) for calculating the source volume velocity in free field is:

where $q\left(\omega \right)$ and $p\mathrm{\text{'}}\left(r,\omega \right)$ are the source volume velocity and the sound pressure at a distance of $r$ from the source center when the circular frequency is $\omega$. ${\rho }_0$ is the density of the medium.

For a single structural actuating point and a single radiation noise measuring point, the reciprocity transfer function can be expressed as:

2.2. Impulse source and reverberation

If the outer sound source is an impulse sound source, the sound pressure $P\mathrm{\text{'}}$ around the sound source in time domain is an impulse signal, shown as:

where, $P_0$ is the amplitude of the impulse signal, $t_0$ is the arriving time of the impulse to the volume velocity measuring point. The relation of $P\mathrm{\text{'}}$ and $p\mathrm{\text{'}}$ can be shown as:

When the sound source is in a reverberant field, the reflective signals and scatter signals are superposed on the measuring results. Recently study showed the reverberant influences of $p_2$ and $v_1$ in Eq. (1) can be counteracted against each other [7]. So just need to consider about the influences on $P\mathrm{\text{'}}$:

where, $P_k$ are the signals amplitudes of reflection and scatter in sound field, and $t_k$ are the transfer time of these signals.

The sound path length of reflective signals and scatter signals is larger than the direct signals’, so $t_0<t_1$, $t_2$,…, $t_n$ . For convenient of this discussion, define $t_1\le t_2\le \dots t_n$ , and the minimum time gap is:

Now define a time gate [8]: $\in \left(0,\mathrm{\Delta }t\right)$ . When the volume velocity sound pressures are measured, just record the signals in the time gate $\left[t_0,t_0+T\right]$. The signals beyond $t_0+T$ are rejected, thereby the reverberant field influences are eliminated.

3.1. Experimental conditions

The above theory and method was validated with an experiment in a lake. The experimental structure is a double-layer cylindrical shell model, and the length of it is 2.05 m. The diameter of its outer shell is 1.78 m, and the thickness of it is 2 mm; the diameter of its inner shell is 1.46 m, and the thickness of it is 8 mm. An actuator is installed in the model. A force sensor and an accelerometer are placed at the contact point of the actuator.

Fig. 1The double-layer cylindrical shell model

The underwater impulse sound source used in this experiment is an electric spark impulse source, and an electromagnetic sound source is also used in this experiment.

Based on the theoretical analysis above, the experiment was performed according to the following steps:

(1) The model was suspended under water, and the underwater impulse source was fixed to the boat in the inlet, 5.7 m apart from the model; hydrophones were placed at four symmetrical locations around the source, each 2 m apart from it.

(2) The impulse was taken with the source; then the sound pressure response around the source and the acceleration response in the model were measured.

(3) The impulse source was withdrawn; an electromagnetic sound source was placed at the location and started with a frequency ranging from 80 Hz to 750 Hz. Likewise, measured the sound pressure response around the source and the acceleration response in the model.

(4) The electromagnetic source was withdrawn, and a hydrophone was placed at the location.

(5) The actuator was started in the model with a frequency ranging from 80 Hz to 750 Hz. The actuating force and the response radiation sound pressure were measured using the hydrophone mentioned in step (4).

3.2. Experimental results

Firstly, demonstrate the effect of time-gate operation to the experiment results. For the convenience of discussion, define the original sound pressure signals around the impulse sound source as original sound pressure signals. Likewise, define the sound pressure signals though time gates as revised sound pressure signals. As shown in Fig. 2, (a) is the original sound pressure signal in time domain; (b) is the revised sound pressure signal in time domain.

Secondly, likewise for the convenience of discussion, define the transfer functions which are obtained with the original sound pressure as original impulse-based reciprocity transfer functions (OIRTF); define the transfer functions which are obtained with the revised sound pressure as revised impulse-based reciprocity transfer functions (RIRTF); define the transfer functions, which are obtained with the measuring results of electromagnetic sound source and eliminated the reverberant influences with traditional methods, as steady-source-based reciprocity transfer functions (SRTF).

Fig. 2The sound pressure signal in time domain

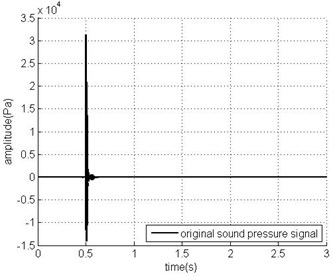
a) Original signal

b) Revised signal

Fig. 3The reciprocity transfer functions from the radiation noise measuring point to the actuator

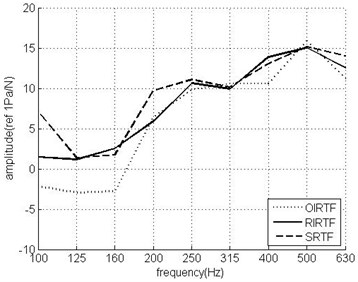
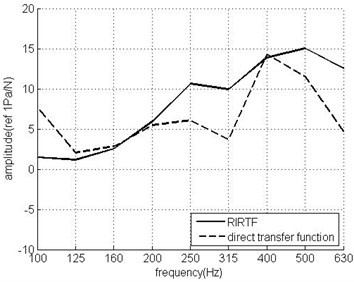
Fig. 4The RIRTF and the direct transfer function of the actuator

As shown in Fig. 3, it is found that in most frequency bands, compared with OIRTF, RIRTF is more close to SRTF. Especially in low frequency bands, the effect of the revisal is significant.

So, with data processing, the impulse-based transfer function measurements can achieve the similar accuracy as the measurements with a steady source. Moreover, for an impulse sound source, the actuating time is short, and the actuating frequency band is wide. Compared with the steady-source-based measuring time, the impulse-based measuring time is reduced and the efficiency is increased.

Lastly, compare the transfer functions of impulse-based reciprocity and direct measurement. As shown in Fig. 4, the error of RIRTF is over 3 dB in some frequency bands, the complex measuring environment of shallow water perhaps contributes to it.