Driver – ECO-car system: design and computer simulation of dynamics

1. Introduction

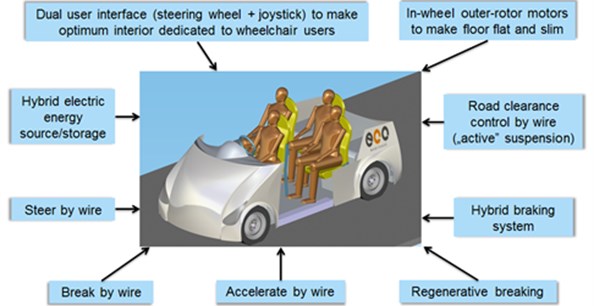
This paper presents a new concept of electric car for four passengers (both for able-bodied and disabled users). The term “adjustments for the disabled” means the vehicle allows disabled people in hand-powered wheelchairs to be able to drive the car (incl. getting into it) as well as transporting a passenger in an electric wheelchair. In the presented version of the car, the disabled are understood as those with disabilities of the lower limbs. The car has a top speed of 50 km/h and is intended for use in enclosed areas where conventional vehicle traffic is limited or forbidden. It is designed to function as part of a car rental system such as Park and Ride schemes. Therefore, such infrastructure problems such as rental and battery-charging systems, etc. must be addressed. Design problems of the electrical system are shown in papers [1, 2]. This article focuses on selected issues related to these technical aspects. Fig. 1 [3] illustrates some of the solutions used in the car’s design.

Fig. 1General characteristics of the presented car

Due to this paper’s limited scope, it will focus on the following issues:

– Vehicle’s computer simulation (regarding a disable driver).

– Ergonomic analysis.

Computer simulations, as mentioned previously, include an analysis of the dynamic properties of the system: the driver-car, during moose test. Thus, the system is analyzed comprehensively. The system takes into account the human factor and car dynamics.

Fig. 2Visualisation of ECO-car functionality: a) general vehicle structure, b) possibilities of entering to the vehicle, c), d) a placement of disabled person (driver) and location of four people (including one disabled person), e), f) entering of a manual wheelchair and an electric wheelchair through the rear ramp, g), h) locating one passenger in the electric wheelchair and the remaining three non-disabled passengers

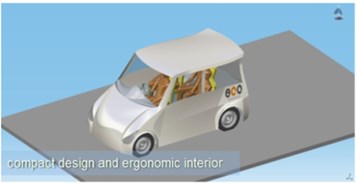
a)

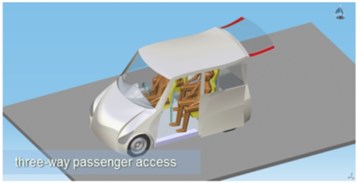
b)

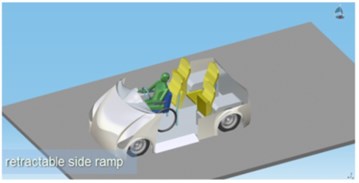
c)

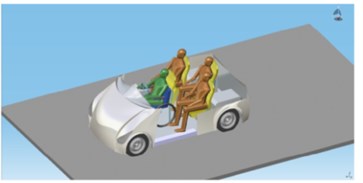
d)

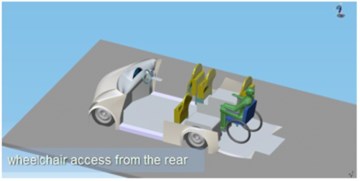
e)

f)

g)

h)

2. ECO-car functionality and versatility

The notion of being “human-friendly and specifically tailored to meet the needs of the people” has been developed based on the assumption which radically differs from the design “philosophy” in the current means of transport. The vehicles should be “universal,” with construction allowing easy and independent handling both for fit persons and persons with disabilities, without extra-special equipment [4]. The means of transport used today call for individual adjustments to meet the needs of persons with disabilities. The different approach is based on the fact that European societies are facing increasing rates of persons with disabilities and seniors; hence improvement of their mobility in a broad sense becomes critically important in the social and economic context. As it was mentioned a person with limited mobility will drive from the vantage position of their own wheelchair. This factor necessitated adequate space for boarding and positioning the wheelchair within the cabin. The vehicle is also equipped with a special securing system to prevent the wheelchair from moving around inside the cabin. Allowing wheelchair users to be drivers of the vehicle required a specific system for folding and maneuvering the front seat when necessary. The mechanism and accompanying microprocessor control system is an important innovation for the authors of this paper. A wheelchair can enter the vehicle either from the rear (with the aid of a special ramp) or from either side of the cabin. Using an air suspension system the car’s floor plate is lowered to 12 cm above the ground which allows easy and trouble-free access according to tests conducted with wheelchair users and who are further assisted with ergonomically located handles. For electric wheelchair users, access to the cabin is only through the rear of the car. Fig. 2 presents the full access functionality of the vehicle.

A characteristic feature that distinguishes the ECO-Car’s design from that of typical passenger cars is seat height. When designing the cabin space the needs of users of reduced height (C₅ women in the driver’s seat and C₉₅ men sitting in the highest wheelchairs) were fully taken into account. In accordance with the legal standards, the height of the wheelchair ranges from 40 cm to 52 cm. The height of the driver’s seat is set to 40 cm resulting in maximum difference in seat and wheelchair height of 12 cm. The cabin is designed in such a way that the driver’s seat has no longitudinal adjustment and due to its height, neither any vertical adjustment. Both a wheelchair-user driver and an able-bodied driver in the driver’s seat occupy the same area in the cabin’s spatial structure. This is also shown in the dashboard design which, with both longitudinal and vertical adjustment features, can be set to facilitate drivers of all sizes.

The Fig. 3 shows the location of the driver‘s wheelchair and the dashboard.

Fig. 3Location of a driver’s active wheelchair and moveable dashboard with multifunction steering wheel

a)

b)

Another issue is the construction of wheelchairs with low backs. When travelling by car, and especially when driving requires the head and back to be supported. What the wheelchair does not provide for the disabled driver, must be accommodated another way, and in the case of the ECO-Car, the backrest from the folded driver’s seat is used. It also comes with seatbelts which can be used to secure a wheelchair.

This additional use required the backrest to be specially designed to enable the wheelchair to move and be accurately aligned despite the wheelchair’s projecting shelf and handles. Fig. 4 shows the position of the driver in a wheelchair, supported with the folded seat for a non-disabled driver.

The important element of of the ECO-car is the circular multifunctional steering wheel (Fig. 3) that allows the driving of the car with the upper limbs only. The steering wheel is one of the elements of the ‘drive by wire’ system that was used in the ECO-car. This means that the traditional mechanical connections (between the steering wheel and the wheel turning mechanism or between the brake pedal and the wheel braking mechanism) were replaced by using electrical or electro-mechanical systems for performing various vehicle functions. This solution is a fundamental part of the design inter alia due to allow the parameterization of the steering system (e.g. an analysis of “steering system sensitivity” – i.e. the variability in the ratio of the steering wheel rotation to the corresponding turning angle of the turning wheels).

Fig. 4Location of a driver in an active wheelchair

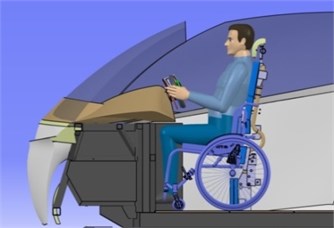
a) In the sagittal plane

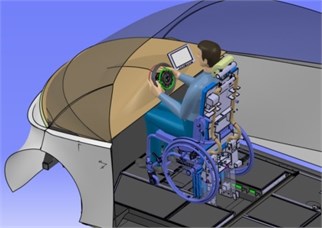
b) The backrest from the folded driver’s seat

Preliminary tests on laboratory model of the steer-by-wire carried out with the participation of people with reduced mobility of the lower limbs showed the presence of local vibration on the steering wheel. The multifunction steering interface is an essential element in the ECO-car (which connects all control functions including acceleration and deceleration), accordingly, the local vibrations are more dangerous for the process than in a traditional car, in which the control is done by the lower and upper limbs. However, it is known that drivers with lower limb disabilities may have minimal difficulty in gripping the steering wheel. Therefore, simulation studies should determine whether it is reasonable to limit the turning range of the car’s steering wheel. It is assumed that a disabled driver will not be able to turn the steering wheel with the same speed as an able-bodied driver.

3. Analysis methods of the steering velocity of front wheels in the double lane change maneuver

This chapter presents simulation studies of the driver-vehicle-road system and the research will address the double lane change maneuver (“moose test”). In the steering process, the steer-by-wire and break-by-wire systems that were installed in our car are of key importance (Fig. 1). Recent studies by other research centers provide evidence that this solution is particularly useful for people with disabilities [5]. Key solutions based on automatic control systems used in SBW systems were presented in [6, 7]. In this system, the steering angle ratio defined as the ratio of steering wheel angle to front wheel steering angle can be adjusted in order to achieve the desired handling characteristics.

Research in the driving process task is divided into the kinematics and dynamics analysis. The purpose of testing the vehicle’s kinematics, prior to testing the its dynamics, is to estimate the minimum velocity values in turning the steering wheel, resulting in a positive test result. The dynamics simulation studies are more important and in the case of our car, their purpose is to select a suitable structure for the electronic differential system of the drive motors as well as either examining the need to use an anti-roll bar with McPherson strut suspension [8] or the steering force needed to turn the wheels. Currently, the “moose test” conditions are standardized in most countries but as an example it could be the German standard [9]. In literature one can also find publications containing the results of experimental studies on the analysis of the selected driver behavior during the “moose test”, i.e. an analysis of the angular speed of the steering wheel [10].

However, the authors of this paper are unaware of any publications describing the results of driving tests carried out for disabled drivers. However, it is known that drivers with lower limb disabilities may have minimal difficulty in gripping the steering wheel. Therefore, simulation studies should determine whether it is reasonable to limit the turning range of the car’s steering wheel. Limiting the turning range results in a lower angular speed in the steering wheel required in the “moose test”. It is assumed that a disabled driver will not be able to turn the steering wheel with the same speed as an able-bodied driver.

Kinematics studies can be performed with relevant and well-known assumptions that simplify the description of the car’s movement, where the Ackermann’s formula is used. With these assumptions, the analysis of the movement trajectory is purely geometrical and helps to objectively determine the minimum required steering velocity of the wheels in the “moose test”. However, the results obtained do not provide a complete picture of the course of the car’s trajectory. For this vehicle the calculations were performed assuming the parameters: wheelbase $L=$2.33 m the distance of the gravitational centre of the vehicle’s body from the centre of the front wheel axis LPA = 1.521 m, maximum steering angle of the front wheels $a_{max}=$ 35°/s. Road dimensions for the lane change manoeuver correspond with the initial phase of the passing in the Moose Test: AC = 13.5 m and CD = 4 m [9, 10]. The calculation results of the kinematics analysis are shown in Fig. 5.

The dotted line DD’ consists of two arcs with $R$ as a constant radius and $E$ as the midpoint of the two curves. The curvature radius is $R=$ 16.7 m. The black line describes the trajectory of the vehicle’s gravity-point centre achieved when the turning velocity is constant and the ride does not include a movement phase with a constant steering angle. The flow diagram of the steering angle is shown in Fig. 5(b). The resulting required minimum velocity of turning the front wheel is $u_w=$ 54°/s. It should be noted that under both model-test and real test drive conditions it will be very difficult for the prototype vehicle to perform a lane-changing manoeuver in a way that the manoeuver is perfectly aligned with the lane axis. A number of factors are attributed to this, including driver behaviour, vehicle drift as well as how the steer-by-wire system works. Any deviation in the final manoeuver from its assumed perfect alignment status results in a change in the velocity of the steering wheels. This may also contribute to a negative assessment of the manoeuver performed.

Fig. 5The calculation results of kinematics of changing lanes maneuver at the lowest turning velocity of the front wheels: a) trajectory, b) turning angle

a)

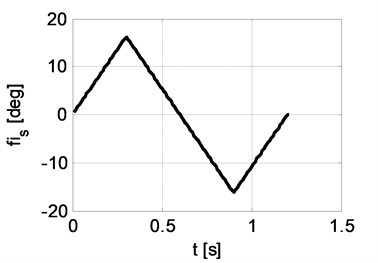
b)

In order to retain this paper’s more manageable scope, the emphasis here is on the control aspect of the human driver and its subsequent conceptual or computer-based modelling. The function of the steering angle adjuster is performed by the “driver model” and its structure is shown in Fig. 6. The input value is a set value of the vehicle wheels’ steering angle, resulting from an analysis of the geometric manoeuver (Fig. 5). The adjustable steering angle relative to the chassis is subject to a time delay due to complicated human reactions, which according to various authors could include; the length of time of the signal’s reception or a decision making time lapse coupled with the motor’s reaction time length [11]. In the presented model, the decision-making duration is described indirectly as the distance travelled from the time of passing through the set point of beginning the manoeuver until the beginning of the reaction (therefore, there is no time delay in the structure of the driver model). Whereas the time of motility (turning the steering wheel through a certain angle) is related to the maximum steering wheel turning velocity ${\upsilon }_k$. According to studies [2] the velocity of turning the steering wheel for the tests carried out in driving simulators produces values of 300-1200°/s ranges, when the test drive only achieves 100-300°/s. This limitation may be included in the Rate Limiter block. An inertial element (block of First Order Inertia) describes the dynamics of the steer-by-wire system. The Saturation block describes the design limitations of the adjustable steering angle of vehicle’s front wheels. The PID controller block is used for modelling the driver’s reaction to any deviation in the car’s trajectory from the desired “perfect” trajectory – Fig. 6. The task of the block is to steer the vehicle back to the desired trajectory.

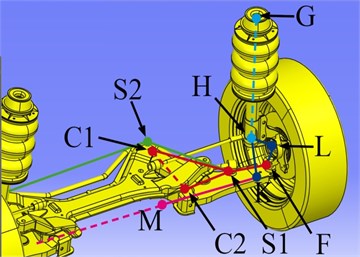
The dynamic model of the examined object consists of partial models: the mechanical system, the electronic differential system for controlling the rotational velocity of the drive wheels, steer-by-wire system to change the driving direction and the steering system (the model described above). The mechanical system model is developed in accordance with the principles of MBS [12]. The model has been developed in a Matlab-SimMechanics environment MBS car suspension simulation models can also be built in Adams environment, as shown in [13]. The structure of the model is described with Fig. 7. Fig. 7(b) with indicated: C1-C2-S1-F – rocker arm (red), H-L-F-K – wheel carrier (blue), G, H – McPherson strut (light blue), M-K – steering gear bar (magenta). S1-S2 – anti-roll bar (green line).

Fig. 6The model structure of the steering system Vehicle dynamics simulation during the double lane change maneuver – “moose test”

Fig. 7Model structure of a) system of rigid bodies of a car model, b) McPherson strut suspension

a)

b)

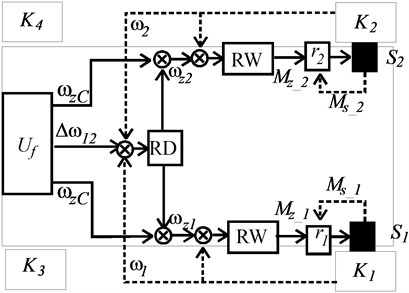
To present the dynamic properties of the tyres the TNO Delft Tyre package has been used [14] with the tyre parameters defined with the library file “TNO_car205_60R15.tir”. The TNO mathematical model describing the wheel slippage relative to the road used the mathematical formulas presented in [15]. The solution of installing an electronic differential of propulsion motors [16], greatly affects the dynamic properties of the vehicle. Preliminary studies of dynamics simulations of the car model have shown that it is worth taking into account the following structures of motor regulatory systems: operating on speed difference for speed control or with the regulator of an average velocity in point A of the front axle of the car. The structures of the regulating systems are shown in Fig. 8 with the infliction of a velocity difference. In the control system shown in Fig. 8 [17], the motor dynamics is described with the first-order inertial element assuming that the time constant of the engine response is $T_{inertia{l}_s}=$5 ms. Visual simulation of the car model driving along the “moose test” route is shown in Fig. 9. The vehicle is estimated to be travelling at a constant driving velocity $v_{sA}=$ 50 km/hr, with the propulsion system operating. Simulation results are presented in Fig. 10.

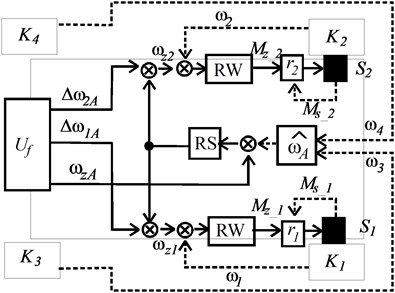
Fig. 8Block diagrams of regulating systems with velocity setting considered for applications in the designed ECO car: a) with the infliction of a velocity difference, b) with the regulator of an average velocity in point A of the front axle of the car. Marked: RW – velocity control regulator, RD – velocity difference regulator, RS – average velocity regulator, S1, S2 – electric traction motors, r1, r2 – regulators of traction motors, ωzA – the preset angular velocity of the replacement wheel at the point A of the front axle center, Δω1A, Δω2A – the preset difference of angular velocity between the replacement wheel and the first wheel, and the second wheel, ωz1, ωz2 – preset angular velocity of motors, Δω12 – preset velocity difference

a)

b)

Fig. 9Visualization of simulation. The positions of the car for the moments 0, 1, 2, 3, 4, 5 s

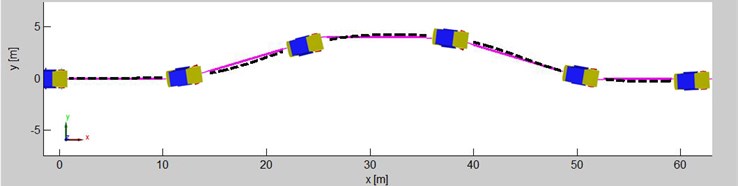
Fig. 10The results of driving simulation in the “moose test”. Front wheel steering angles of the driver model: Input (“reference”) and output (“resulting”)

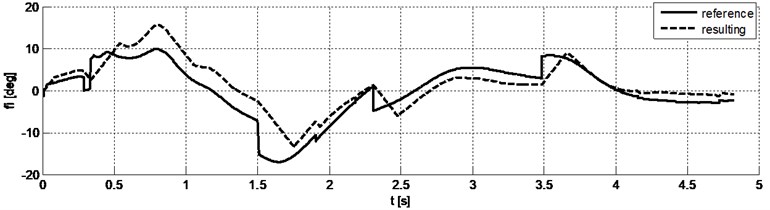
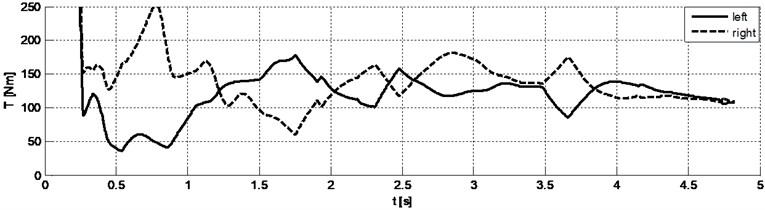
Fig. 11 shows the deflection angle of vehicle’s chassis. The yaw angle is the resultant angle in the drifting of the vehicle’s centre of gravity relative to the $OZ$ axis of the local chassis system. In order to demonstrate the workflow of the McPherson strut suspension, which is shown in Fig. 7, a deflection angle of rocker arms and a steering angle of the stabiliser have been determined. Fig. 12 shows the simulation test results of the variables. At the start of the simulation a galloping motion of the car model occurs, which is related to the inaccurate specified initial conditions of the simulation. It can also be observed that when the car makes a turn, the control arm deflections are compensated by a twist of the compliant element of the anti-roll bar. Fig. 13 shows the applied torque of the propulsion system traction motors $M_{s1}$ and $M_{s2}$, resulting from the operation of the adjustment system (Fig. 10). As a result of this adjustment, the outer wheels are driven by a torque higher than the internal wheels (the first turn is to the left).

Fig. 11The results of driving simulation in the “moose test”. Tilt angle of body chassis in the local coordinate system: roll – yaw – pitch

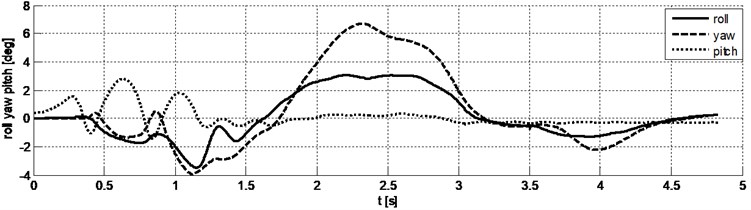
Fig. 12The results of driving simulation in the “moose test”. The deflection angle of control arms and the twist angle of the anti-roll bar

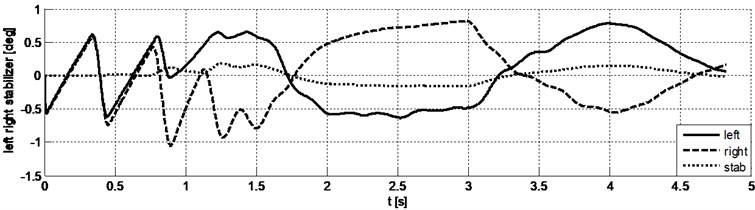
Fig. 13The results of driving simulation in the “moose test”. Motor torques

4. Analysis of the required steering velocity of the front wheels in the moose test

The above simulation results show that the vehicle is able to pass the “moose test”. As stated above, it is assumed that the driver may, to some degree, have difficulties gripping the steering wheel, thus the analysis results of the turning velocity of the wheels should provide an answer to the question of how to adjust the maximum turning range of the steering wheel in the vehicle.

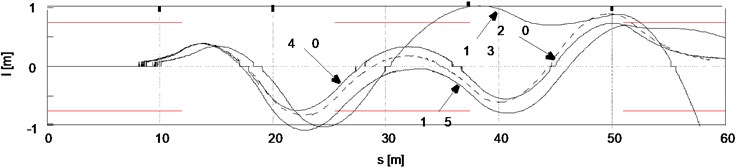
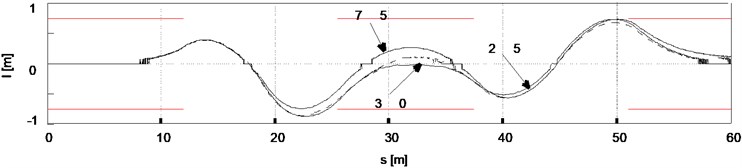
Fig. 14 shows the vehicle’s trajectory simulation results of the dynamics model. The “13” line indicates the distance of the vehicle’s central gravitational point from the road axis, in the case of limitation to the maximum velocity of turning the front wheels to the value ${\upsilon }_s=$13°/s. In this case it failed to keep the vehicle within the required distance range from the road axis. The “15” line shows the velocity ${\upsilon }_s=$ 15°/s, line “20” – ${\upsilon }_s=$ 15°/s, line “40” – ${\upsilon }_s=$40°/s. The results show that for a steering velocity of at least ${\upsilon }_s=$ 40°/s is required the positive test performance. For a maximum steering angle of the front wheels ${\alpha }_{max}=$ 35°/s, to be achieved through a 1.25 rotation of the steering wheel, the wheel would have to be turned at a velocity of at least ${\upsilon }_k=$514°/s, and for a 0.5 rotation, just 206°/s.

Fig. 14The results of driving simulation in the “moose test”. Distance of the gravity center point of the car model from the road axis, for different preset values of the maximum speed of turning the steering wheel

a)

b)

5. Conclusions

This paper presents the transient vibration caused by lane change maneuver (called: moose test). The object of analysis was the innovative design of the ECO-car equipped with, among others steer-by-wire system, the interface which consisted of multifunction steering wheel adapted for people with and without disabilities. Article demonstrated that the velocity of rotation of this wheel has extremely significant impact on the correctness of this type of maneuver. Computer simulation experiment required including it in the simulation model, the model of man. Preliminary results are very promising and the conclusions from them were used in the actual construction of ECO-car.