Structural dynamic response analysis on structure under tsunami bore impact

2.1. Experimental setup

The experiments were carried out in a 16.6 m long, 0.6 m wide, and 0.45 m deep wave tank, as shown in Fig. 1. A single bore was generated by lifting a 6.4 mm thick stainless steel gate, which initially separated a thin layer of water from the impoundment behind the gate. The downstream water depth was maintained at 20 mm. A 120×120 mm square column were used in the present experiments, it has two sides parallel to the tank side walls and consequently the other two perpendicular. To analyze tsunami bore on the square column generated from different impoundment depth $H$, nine cases including 100, 125, 150, 175, 200, 225, 250, 275, 300 mm were considered in the experiment respectively.

Fig. 1The experiment setup (unit: mm)

a) Plane layout

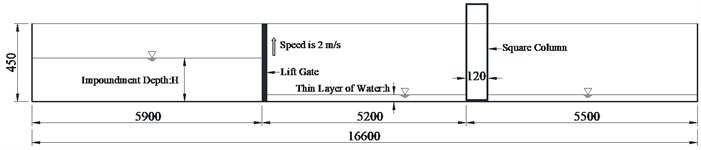
b) Elevation layout

2.2. Development of 3D numerical model of tsunami bore and analysis of effectiveness

On the basis of experiment, a 3D wave numerical model of structure under impact of tsunami bore was established according to the experiment of impact of long dam-beak bore onto squared column in Herry Yeh et al. [12], shown in Fig. 2. The boundary conditions were set as follows: non-slip solid wall boundary condition was applied to upstream, two sidewalls, and the bottom of the tank. On this boundary, the flux of the fluid was zero and only tangential stress existed. The top of the tank was free surface boundary and the downstream was set as outflow boundary.

Fig. 23D model for tsunami bore

With the above 3D tsunami bore model, this study conducted numerical simulation of interaction of the tsunami bore and the column with 9 different impoundment depths $H$. Table 1 summarizes the comparison of the maximum horizontal impact force between the experiment and simulation. From Table 1 it can be seen that the maximum error of the simulation was 12.87 %, which occurred with $H=$100 mm. The second maximum error was 8.70 %, which occurred with $H=$300 mm. The remaining 7 simulations had errors smaller than 5 %. It indicated that the numerical simulation in this study was relatively accurate. Fig. 3 shows the comparison of time history curves of horizontal impact force onto the column due to the tsunami bore between experimental and simulation result, with different impoundment depths. In Fig. 3, the vertical coordinate is the maximum horizontal impact force acting on the column, the horizontal coordinate is the impact duration, and $H$ Similarly, from the comparison of the time history curves shown in Fig. 3, the overall trend of experiment and simulation was consistent, which reflected the loading process of the column under the impact of the tsunami bore.

Fig. 3Comparison of time history curves of horizontal impact force on the column between experiment and simulation with different impoundment depths

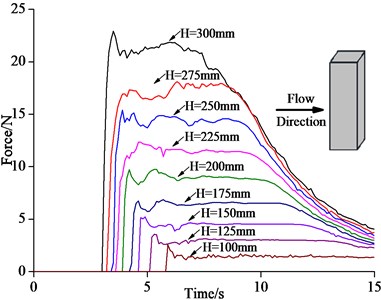
a) Simulation curves

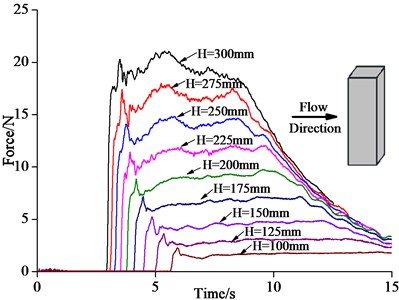
b) Experiment curves

3.1. The impact model of tsunami bore onto structure

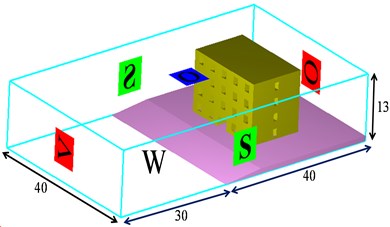
It was assumed that a coastal reinforced concrete frame structure was under the impact of a tsunami bore in this study. The layout of the structure is shown in Fig. 4. This frame structure had 4 floors with the height of 3 m at each floor and 3 spans of 5 m, 3 m, and 5 m, respectively. The cross section of each column was 400 mm×400 mm. The cross section of each beam was 250 mm×450 mm and thickness of the slab was 100 mm. Fig. 5 shows the detailed sketch of 3D tsunami bore model and boundary condition. Considering that the tsunami waves had considerable velocity before approaching the shore and the waves demonstrated a breaking feature, the inlet of the model was set as velocity boundary V. Symmetry boundary S was set at the left- and right-hand side of the computational region of the model. The bottom of the model was solid wall boundary condition W, where the normal velocity of the fluid at this boundary was 0, which did not allow the fluid to flow in or out. The top and the downstream of the model were applied with outflow boundary O, which allowed the fluid to flow freely.

Fig. 4Layout of the structure (unit: mm)

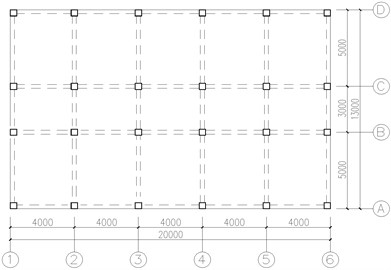
Fig. 53D tsunami bore model of the structure and boundary conditions (unit: m)

3.2. Simulation result and analysis

Table 2 lists the case conditions of simulation and maximum horizontal impact force on the structure. It can be seen in Table 2 that with the increase of wave height and inlet boundary velocity, the maximum horizontal impact force acting on the structure by the tsunami bore demonstrated a significant increasing trend. When the wave height reached 3 m, 4 m, and 5 m, the maximum horizontal impact forces were 3.1, 5.2, and 7.6 times than that at wave height of 2 m. Clearly, the key impact factors of impact force of tsunami bore on the structure were wave height and impact velocity.

The time history curve of horizontal force acting on the structure by the tsunami bore was obtained through simulation with 4 different wave heights, shown in Fig. 6. With the increase of the wave height, during the period from the initial impact time of the tsunami waves on the structure to 10 seconds later, the horizontal impact force acting on the structure by the waves increased rapidly at the initial stage, followed by an oscillation process with unsteady force. The dynamic effect of the force was very clear. It was mainly attributed to the strong turbulent phenomenon that was generated during the impact process of the tsunami bore on the structure, which induced unsteady forcing on the structure. Meanwhile, the reflection of the waves could also affect the forced state of the structure. During 10 to 20 seconds after the initial impact, the horizontal force tended to be steady. At this time, the horizontal force acting on the structure was primarily dynamic water pressure force.

Fig. 6Time history curves of horizontal forces on the structure by tsunami

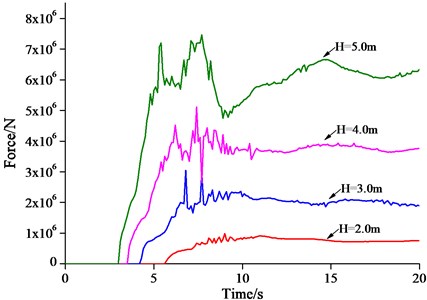
Fig. 7Impact on the structure by the tsunami bore with wave height H= 3 m

a)$T=$ 4.4 s

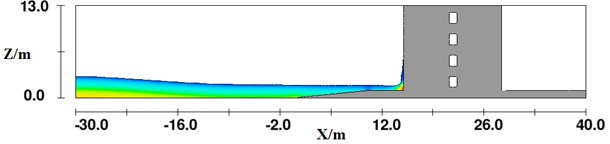
b)$T=$ 4.9 s

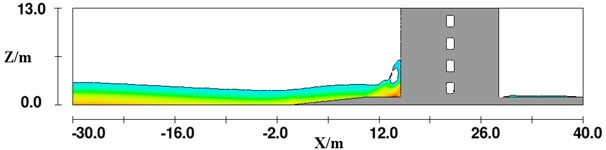
c)$T=$ 6.8 s

Fig. 7 shows a series of structure under impact of the tsunami bore with wave height $H=$3 m. Fig. 7(a) shows the scenario at $T=$ 4.4 s after the initial impact between fluid and the structure. In this figure, it can be seen that the fluid started to impact the bottom of the structure and had not climbed along the structure. Fig. 7(b) shows the relative location of the fluid and the structure at impact time $T$ of 4.9 s. It is clearly demonstrated that the fluid started to climb along the structure at this moment. When the impact time lasted to $T=$ 6.8 s, shown in Fig. 7(c), the fluid reached the maximum elevation on the structure, where the reflection phenomenon occurred. Consequently, the impact force on the structure by the fluid reached the maximum value.

4.1. Static response of the reinforced concrete frame under the impact of tsunami bore

The concrete grade of the frame is C30, the axial compressive strength is 20.1 MPa, the passion ratio is 0.2, elastic modulus is 3.0×10⁴ MPa and density is 2400 kg/m³. HRB400 is adopted for the longitudinal load-bearing bar and stirrups, the standard yield strength is 400 MPa, the passion ratio is 0.3, elastic modulus is 2.0×10⁵ MPa and the density is 7850 kg/m³.

Fig. 8Finite element model of the frame structure

Fig. 9Local fiber reinforced steel REINF264

The 3D finite element model was created for the frame with the finite element software ANSYS, shown in Fig. 8. Beam-column element was selected as BEAM 188 [13] in the analysis of structure, which is suitable for analysis of slender, medium and long beams and columns. SHELL63 [13] element was selected for the slab element of the structure, which had bending and membrane characteristics. In this simulation, multilinear kinematic hardening model was used to simulate the elastoplasticity of the concrete. On this basis, the concrete constitutive relation proposed by Hongestad [14] is employed to realize the simulation. To precisely simulate the relative positioning between the steel and concrete, this paper employed separate model. This model used strengthened fiber reinforced element REINF264 [13] to simulate the steel bars in structural beams and columns. Bilinear kinematic hardening model was used to simulate the mechanical characteristics of elastoplasticity of the fiber reinforced steel. REINF264 element was formed on the basis of existing solid base element with built-in of beam column element. The same base element could specify multiple strengthened fiber reinforced element at the same or different directions, which could accurately reflect the relative position of the steel bar and concrete. Partial fiber reinforced element is shown in Fig. 9.

In the static analysis, the maximum value of the horizontal force in the time history curve of the impact force on the structure due to the tsunami bore can be treated as the static load of the structure that was acted on the frame. The maximum displacement along the direction of the tsunami impact was calculated under the maximum load in the simulation. Table 3 gives the maximum horizontal displacement along the direction of the waves at the structure top under different case conditions. In Table 3, it can be known that the maximum horizontal displacement at the structure top increased with the increase of maximum horizontal impact force.

4.2. Dynamic analysis of the reinforced concrete frame under the impact of tsunami bore

Structural transient dynamic analysis (sometimes called time-history analysis) was usually used to determine the dynamic response of the structure with the time dependent various load such as impact load and shock load. It can be used this type of analysis to determine the time-varying displacement, velocity, acceleration, and force in a structure as it responds to any combination of static, transient, and harmonic loads. Due to strong dynamic effect of impact of the tsunami bore on the structure, the time between the initial impact and the occurrence of maximum impact was very short. Therefore, transient dynamic analysis of the structure under impact of the tsunami was necessary.

Modal analysis is mainly used to determine the vibration characteristics of structure, such as the structure natural frequency and vibration mode. The structure natural frequency and vibration mode are the important parameters in the design of structure under dynamic loading and also the foundation of other kinds of dynamic analysis. On many occasions, the modal analysis plays a decisive role. The multi degree of freedom system does not have the same number of natural frequency as the DOF, but also has the corresponding style of vibration mode, expressed as the equation of motion in the form of a matrix:

where $\left\{\ddot{\mathbf{u}}\right\}$ is the acceleration vector, $\left\{\mathbf{u}\right\}$ is the displacement vector, $\left[\mathbf{M}\right]$ is the mass matrix, $\left[\mathbf{C}\right]$ is the damp matrix and $\left[\mathbf{K}\right]$ is the stiffness matrix.

From the Eq. (1), the characteristic equation could be derived:

Accordingly, It has characteristics of $n\text{:}$ natural frequency ${\omega }_i$ and vibration mode $\{\varphi {\}}_i$ ($i=$1, 2, 3,…, $n$), they form a complete set of modal, which is used to represent dynamic vibration characteristics of the structure.

To determine the vibration characteristics of the structure, ANSYS was used to perform modal analysis for the frame. The finite element model of the modal analysis is shown in Fig. 8. The first two order mode of the frame are shown in Fig. 10, which the deformation of the structure are mainly in translation. The calculation results of the first eight vibration modes of natural frequency are summarized in Table 4.

Fig. 10First two order mode of the frame

a) First order mode

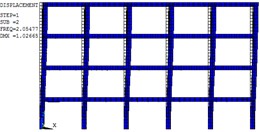
b) Second order mode

In the structural dynamic analysis, the basic equation of motion solved by a transient dynamic analysis is:

where $\left[\mathbf{C}\right]$ is the structural damp matrix, $\left\{\dot{\mathbf{u}}\right\}$ is the nodal velocity vector and $\left\{\mathbf{F}\right(t\left)\right\}$ is the load vector.

It is necessary to determine the damping. In this paper, Rayleigh damping was used, following:

where $\alpha$ is mass damping coefficient, $\beta$ is stiff damping coefficient. The two coefficients can be obtained from Eq. (5) and (6), respectively:

where ${\omega }_i$ and ${\omega }_j$ are $i$th and $j$th natural frequency of the structure. ${\xi }_i$ and ${\xi }_j$ are the damping ratio of $i$th and $j$th vibration mode. In this paper, the damping ratio of the reinforced concrete was set to be 0.05 and corresponding vibration modes were selected as the first and second mode of the structure.

Fig. 11Time history curve of displacement of structure top

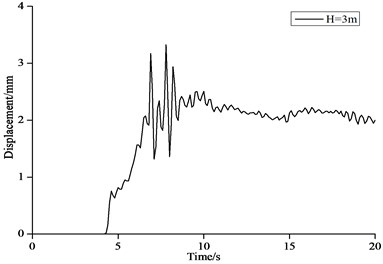
a)$H=$3 m

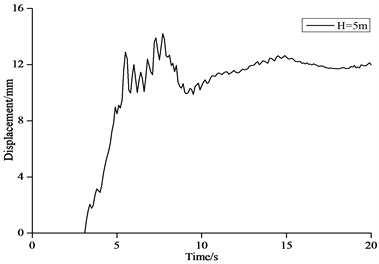
b)$H=$ 5 m

Structural transient dynamic analysis includes full method, reduced method, and mode superposition method. This paper applied full method to perform the dynamic response analysis, i.e., using complete system matrices to calculate the transient dynamic response of the structure. It could include all kinds of structural non-linear deformations. In the transient dynamic calculation, initial condition of the structure should be determined firstly. Both initial displacement and velocity were zero before the impact of tsunami waves on the structure. Hence only the corresponding gravitational load was acted on the structure.

Fig. 11 shows the time history curves of horizontal displacement of the structure top with wave height of 3 m and 5 m. Fig. 12 shows the time history curves of horizontal velocity of the structure top with wave height of 3 m and 5 m. Fig. 13 shows the time history curves of horizontal acceleration of the structure top with wave height of 3 m and 5 m.

In Fig. 11-13 it can be seen that the dynamic impact effects of the tsunami bore on the structure were very different at different impact time. From 0 to 5 s, the structure start to vibrate from the stationary state, while the displacement, velocity and acceleration were small. From 5 to 10 s, the displacement, velocity and acceleration at the structure top reached the maximum values. From 10 to 20 s, the vibration of the structure decayed rapidly until close to the initial stage. This time of period belonged to the last stage of the forced vibration of the structure. The displacement of the structure tended to be stable, while the velocity and acceleration were close to 0.

Fig. 12Time history curve of velocity of structure top

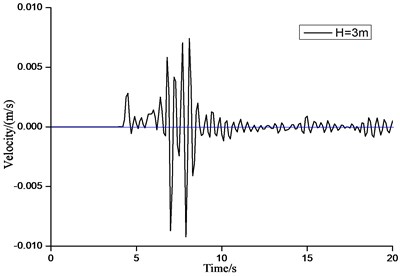
a)$H=$ 3 m

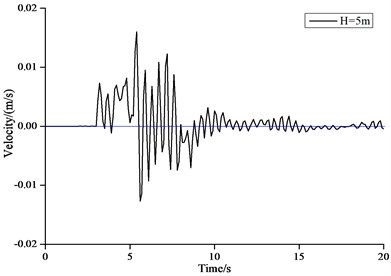
b)$H=$ 5 m

Fig. 13Time history curve of acceleration of structure top

a)$H=$ 3 m

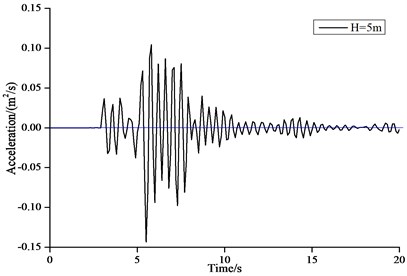
b)$H=$ 5 m

Table 5 gives the maximum dynamic displacement of the reinforced concrete frame and the dynamic magnification factor of the structure under the impact of the tsunami bore. Structural dynamic magnification factor indicated the ratio between the maximum dynamics displacement value of the structure under the varying load with respect to time and the static displacement value under the static force that was equal to the maximum value of the varying load. Table 5 indicated that the dynamic displacement of the structure was larger than the corresponding static displacement. Because the lasting time of the maximum impact force on the structure due to the tsunami bore was short, the impact force reached the maximum value and decreased rapidly. Therefore, the dynamic magnification factor of the structure was relatively small, where the maximum value of 1.147 occurred when the tsunami wave height was 5 m. It indicated that the impact effect of the tsunami bore on the structure had certain magnification effect. In the tsunami disaster regions, design of the anti-tsunami structures could take conservative dynamic magnification factor of 1.2.

Generally speaking, in order to reduce the tsunami wave impact forces acting on the structure, taking into consideration the structural feature of buildings, houses with structural forms which could resist tsunami impact should be constructed. When constructing houses in areas exposed to tsunami hazard, the engineering prevention and control measures should be taken, for example, upstream walls should not be arranged excessively at the bottom of the house, and the bottom layer should be designed into the open and transparent type, which can help to shunt tsunami waves, to reduce the impact of tsunami wave on the structure. Due to the tsunami wave propagation velocity is very high, so decrease the speed of tsunami waves can effectively reduce the tsunami impact force acting on the structure, therefore, it is can be set up seawall in the tsunami potential coastal area to decrease the speed of tsunami waves. Moreover, the tsunami impact force is local structural force which just only affect the bottom components of the structure, so that in anti-tsunami design, the strength of bottom components should be strengthened to improve the load-bearing ability of the structure.