A study of influencing parameters on conductor galloping for transmission lines

1. Introduction

It is very known that conductor galloping of the transmission line is a classical self-excited aeroelastic oscillation phenomenon due to wind action [1]. This motion is characterized by low frequencies (about 0.1-3 Hz) and large amplitudes (about 20~300 times conductor diameter) with a single or a few loops of standing waves per span [2, 3]. Damages caused by conductor galloping can be equally dramatic and very costly with broken conductors and fittings, damaged tower components and even whole towers [4-6]. As a result, the consequential economic and social costs of power loss to whole areas can also be very considerable. So the phenomenon of conductor galloping has been gaining more and more attention from the mechanism to the anti-galloping method all over the world [3].

Conductor galloping has been widely studied since the 1930s [7, 8]. A vertical galloping mechanism was firstly proposed by Den Hartog [7]. Whereafter, several other mechanisms, such as torsinal galloping mechanism [9], torsional feedback mechanism and nonlinear instability [10, 11], were also proposed based on the different emphases and phenomenon interpretations. Although the conductor galloping has been studied extensively, hitherto it is still not full understood because of its nonlinearity and complex influence factors. And it has been realized through field observations and tests that the factors influencing conductor galloping characteristics are so complicated that they include not only weather conditions and structural factors of transmission lines, but also many other random factors. Based on the theoretical and experimental points of view, the main factors may include ice accretion type and shape, wind velocity and direction, span lengths, conductor self-damping, attachment type between conductor and tower, number of sub-conductors and their arrangement, torsional frequency and stiffness, operating tension, sub-conductor spacing, spacer properties, retrofit devices, and so on [3].

Some investigations have been performed to determine the non-dimensional factors and provide the best candidates for galloping analysis. Lilien and Dubois [12] proposed four comprehensive dimensionless parameters due to galloping characteristic analysis for bundle conductors. The CIGRE work group of conductor galloping summarized the proposed dimensionless parameters of single and bundle conductors [3]. Other researches mainly focus on the conductor galloping characteristics or the single physical parameter [13-18]. So, in-depth analysis for the influencing factors to the conductor galloping is also lacking from the existing literatures. The existing studies on factors influencing conductor galloping are still not satisfactory.

So it is significant to study the factors influencing the conductor galloping, especially for the development of effective anti-galloping methods as the galloping mechanism has not been solved completely. In this paper, three important dimensionless parameters, damping ratio, reduced wind velocity and reduced mass ratio, are proposed by dimensionless analysis based on the conductor galloping model. The galloping equivalent amplitude is derived by solving the nonlinear galloping equation whose aerodynamic force is obtained by polynomial fitting by using the average method. And the formula to calculate the galloping critical wind velocity is also presented. Based on three typical eccentric ice accretion shapes, D shape, U shape and airfoil shape, the influence of dimensionless parameters to the galloping equivalent amplitude is also studied. The results obtained in this study have an important guidance to the anti-galloping design for transmission lines.

2. Galloping analysis model

Based on the differential equation of motion for one DOF system, there is:

where $m$ is the mass per unit length, $c_y$ is the damping coefficient, $k_y$ is the stiffness coefficient, $\ddot{y}$, $\dot{y}$, $y$, $F_y$ are the acceleration, velocity, displacement and force vector in $y$ direction respectively. Assume that the axial deformation is neglected. Fig. 1 gives a schematic diagram of an iced conductor and shows definitions of the aerodynamic forces.

Fig. 1Galloping analysis model of an iced conductor

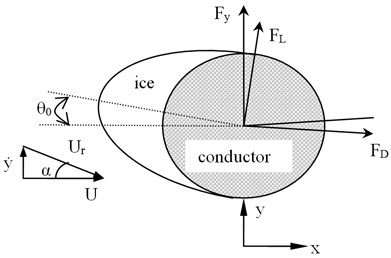
Wind velocity is known as $U$, and the relative wind velocity of the vibrant conductor is $U_r=\sqrt{{\dot{y}}^2+U^2}$. $\alpha$ is the modification of the angle of attack introduced by the vertical velocity $\dot{y}$. And considering $\alpha$ as a relatively small amount, the angle of wind attack can be calculated as:

Based on the quasi-steady assumption [3], the dynamic angle of attack $\theta$ is defined as:

where ${\theta }_0$ is the initial static off-set angle of the iced conductor. And the aerodynamic forces can be expressed as follow:

where $\rho$ is the mass density of air, $D$ is the reference length of the iced cross section which is generally substituted by the conductor diameter, and $C_D$, $C_L$ is the drag and lift coefficients of the profile respectively.

By considering the angle $\alpha$, the aerodynamic force on $Y$ direction is obtained as:

where $C_y=C_L\text{cos}\alpha -C_D\text{sin}\alpha \approx C_L-C_D\alpha$.

Generally, the properties of lift and drag coefficients $C_L$ and $C_D$ mainly depend on the ice shape, ice angle, and ice thickness etc. And they can be obtained by the wind tunnel test. In order to establish the aerodynamic model conveniently, the force coefficient $C_y$ is usually expressed as a polynomial function with respect to the dynamic angle of attack $\theta$ based on the quasi-steady theory, and the polynomial curve can be given in the form as:

where $b_k$ is the fitting coefficient and $N$ is the order of the polynomial.

Substituting Eq. (3), (5) and (6) into Eq. (1), there is:

By introducing dimensionless displacement $u=\frac{y}{D}$ and dimensionless time $\tau ={\omega }_{y}t$ in which ${\omega }_y$ is vertical natural frequency ${\omega }_y=\sqrt{\frac{k_y}{m}}$, three dimensionless parameters are defined from Eq. (7) as follow:

damping ratio ${\xi }_y=\frac{c_y}{2m{\omega }_y}$,

reduced wind velocity $U_y=\frac{U}{{\omega }_{y}D}$,

and mass ratio ${\mu }_y=\frac{\rho D^2}{2m}$.

Then by dimensionless analysis, Eq. (7) becomes:

where ${\left(\right)}^{\text{'}}=\frac{d}{d\tau }$.

Assuming that $k_{\omega }=\frac{\omega }{{\omega }_y}$ as the dimensionless frequency, Eq. (8) can be rewritten as:

where $\epsilon f(u,u^{\text{'}})=\left(k_{\omega }^2-1\right)u-2{\xi }_y{u}^{\text{'}}+{\mu }_y\left(U_y^2+{u^{\text{'}}}^2\right)\sum _{k=0}^N{b}_k{\left({\theta }_0-\frac{u^{\text{'}}}{U_y}\right)}^k\text{,}$ and $\epsilon$ is an expression of mirror quantity.

Eq. (9) can be solved by using the average method. So assuming the solution of Eq. (9) is:

where $a$ is amplitude, and $\varphi =k_{\omega }\tau +\phi$.

Substituting Eq. (10) into Eq. (9), we can obtain:

where $a$ and $\varphi$ are the slow variable functions with respect to $\tau$. So the right items of the Eq. (11) are averaged in one cycle, and we can obtain:

Using ${\int }_0^T\text{cos}\varphi {\text{sin}}^k\varphi d\varphi =0$, ${\int }_0^T{\text{cos}}^2\varphi d\varphi =\frac{T}{2}$, and $\left\{\begin{array}{ll}{\int }_0^T{\mathrm{s}\mathrm{i}\mathrm{n}}^k\varphi d\varphi =0,& k=2n-1,\mathrm{}\mathrm{}\mathrm{}\mathrm{}\left(n=\mathrm{1,2},3,\dots \right),\\ {\int }_0^T{\mathrm{s}\mathrm{i}\mathrm{n}}^k\varphi d\varphi =\frac{C_n^{2n}}{2^{2n}}T,& k=2n,\left(n=\mathrm{1,2},3,\dots \right),\end{array}\right.$

Eq. (12) becomes:

Considering the steady state solution (periodic solution) of Eq. (13), amplitude $a$ does not change with respect to time. So Eq. (13) becomes:

From the first expression of Eq. (14), it presents a high order equation with respect to the amplitude variable $a$. And the order of the equation depends on the order of the fitting polynomial. Solving Eq. (14) by using $N=3$ and $N=5$, we can get the following results.

(1) When the curve of $C_y$ in Eq. (6) is fitted by cubic polynomial, namely $N=3$, a forth order equation with respect to $a$ can be derived from Eq. (14). And an analytic solution can be obtained as follows:

(2) When the curve of $C_y$ in Eq. (6) is fitted by quintic polynomial, namely $N=5$, a 6th order equation with respect to $a$ can be derived from Eq. (14). And an analytic solution can be also obtained from Eq. (14) as follows:

where the expressions:

$A_1=35b_5{\mu }_y,$

$A_2=\left(40\left(b_3+b_5\right){\mu }_y+400b_5{\mu }_y{\theta }_0^2+160b_4{\mu }_y{\theta }_0\right)U_y^2,$

$A_3={48\left(b_1+b_3\right){\mu }_y+240b_5{\mu }_y{\theta }_0^4+192b_4{\mu }_y{\theta }_0^3+\left(144b_3+480b_5\right){\mu }_y{\theta }_0^2+\left(96b_2+192b_4\right){\mu }_y{\theta }_{0}U}_y^4,$

$A_4=128{\xi }_y{U}_y^5+\left(64b_1{\mu }_y+320b_5{\mu }_y{\theta }_0^4+256b_4{\mu }_y{\theta }_0^3+192b_3{\mu }_y{\theta }_0^2+128b_2{\mu }_y{\theta }_0\right)U_y^6,$

$B_1=\frac{2A_2^3}{27A_1^3}-\frac{A_2{A}_3}{3A_1^2}+\frac{A_4}{A_1},$

$B_2=\frac{A_3}{A_1}-\frac{A_2^2}{3A_1^2},$

$h_i=\left\{\begin{array}{l}{h}_1=1,\\ h_{\mathrm{2,3}}=\frac{-1\pm \sqrt{3}i}{2}.\end{array}\right.$

(3) When the curve of $C_y$ in Eq. (6) is fitted by seven order polynomial or above, namly $N>$5, it is difficult to obtain an analytic solution from Eq. (14). But it can be solved by numerical methods.

3. Influencing parameters analysis

From Eq. (14), three dimensionless dominant parameters, damping ratio, reduced wind speed and mass ratio, can be studied. And the influence to the galloping amplitude can be also obtained by solving Eq. (14). In this study, as an example, the expression (15) is used to analyze the influence of these parameters to the galloping amplitude. In the practical transmission lines, the parameter ${\mu }_y$ has small variation to a given conductor type because ${\mu }_y$ is mainly related to the structural characteristics of the conductor. So other two parameters, ${\xi }_y$ and $U_y$, are mainly considered in the following analysis.

According to field observations, three common ice shapes covered on the conductor surface, U shape, D shape and airfoil shape, are introduced in this study. Fig. 2 shows the schematic diagrams of the three eccentric ice accretions on the conductor respectively, in which $S$ is ice thickness.

Fig. 2Schematic diagrams of three typical iced conductors

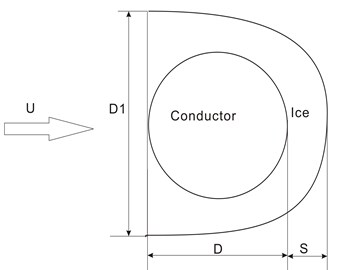
(a) D-shape iced conductor

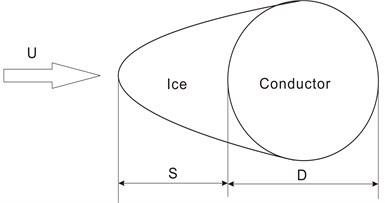
(b) U-shape iced conductor

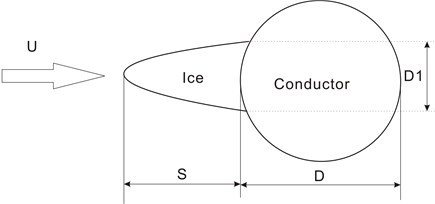
(c) Aerofoil-shape iced conductor

Field observations and tests show that the characteristic timescale of conductor galloping is much larger than the characteristic timescale of the flow, and the vortex shedding frequency is much higher than the frequency of galloping [22]. So the quasi-steady assumption is used wildly to obtain the aerodynamic forces.

The validity of quasi-steady theory has been tested based on the existing studies. The curves of aerodynamic force coefficients in this study are obtained by the wind tunnel tests according to the stationary iced conductors at different angles of incidences.

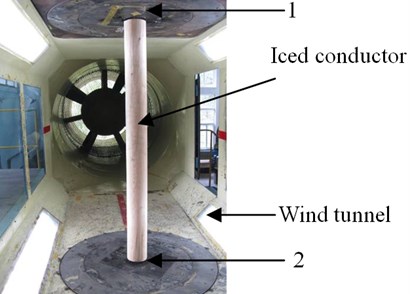
The size of our wind tunnel is 1.4 m×1.4 m×2.8 m for width, height and length. It is a close loop with an open section for the testing area. And it allows the maximum wind speed of 68 m/s and the minimum steady wind speed is about 10 m/s. The turbulence intensity is not more than 0.14 %. Fig. 3 gives a typical photo for the U-shape iced conductor in the tunnel test. The sample is fixed by two steel plates at the two endpoints 1 and 2 in Fig. 3, which can make the sample rotation in 360° range of angle of attack. Dynamomenters used to measure the aerodynamic coefficients are set at 1.

Fig. 3A typical photo in the tunnel test

As an example, the experimental data for U-shape, D-shape and airfoil-shape iced conductors with $S=$6 mm and $D=$26.8 mm are presented in Fig. 2. The conductor mass of unit length is 1.35 kg/m. The quasisteady measured coefficients of $C_y$ as functions of the angle of attack are shown in Fig. 4. And the fitting curves by using the cubic polynomial are also shown in Fig. 4.

Fig. 4Quasi-steady aerodynamic force coefficients measured in the wind tunnel and fitting curves by 3th order polynomial

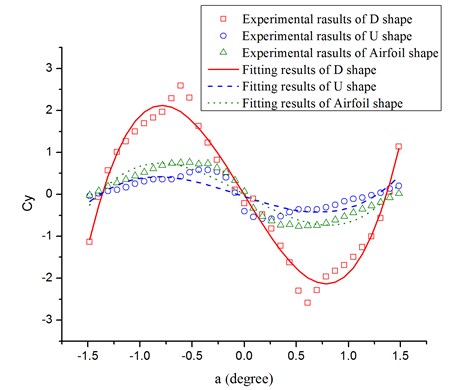
Fig. 5A typical time history and phase diagram on galloping for the D-shape iced conductor

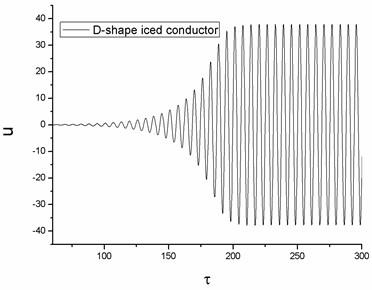
(a) response curve

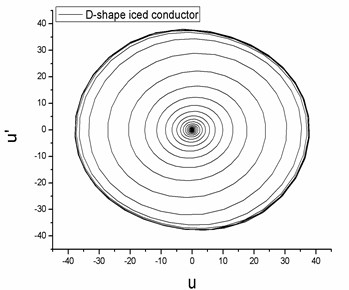
(b) phase diagram

3.2. Critical wind velocity of conductor galloping

In order to obtain a simplified expression, suppose that the initial static offset angle ${\theta }_0=$0. To make sure that the amplitude $a$ has only real roots from Eq. (15), the right item in Eq. (15) must equal to or greater than zero, then we can obtain:

17

$\left\{\begin{array}{l}-3(b_1+b_3)\pm \sqrt{9{\left(b_1+b_3\right)}^2-40b_1{b}_3-\frac{80b_3{\xi }_y}{{\mu }_y{U}_y}}\ge 0,\\ 9{(b_1+b_3)}^2-40b_1{b}_3-\frac{80b_3{\xi }_y}{{\mu }_y{U}_y}\ge 0.\end{array}\right.$

In fact, from Eq. (17), two stability conditions are presented to excite the conductor galloping phenomenon.

Therefore, the critical wind velocity of the conductor galloping can be derived from Eq. (17), and we can obtain:

18

$\left\{\begin{array}{ll}{U}_{cy1}=-\frac{2{\xi }_y}{b_1{\mu }_y},& \text{(I)}\text{,}\\ U_{cy2}=\frac{80b_3{\xi }_y}{{\mu }_y(9{(b_1+b_3)}^2-40b_1{b}_3)},& \text{(II)}\text{.}\end{array}\right.$

From Eq. (18), the critical wind velocity (condition I) $U_{cy1}$ coincides with the well-known Den Hartog criterion. And the critical wind velocity (condition II) $U_{cy2}$ is a new criterion which is more complex than $U_{cy1}$. It is obvious that $U_{cy1}$ is only relative to the first-order coefficient $b_1$ of the fitting polynomial in Eq. (6). But $U_{cy2}$ is relative to both the first-order and the third-order coefficients, $b_1$ and $b_3$. Due to ${(b_1+b_3)}^2\ge 0$, there is the expression $U_{cy2}\le U_{cy1}$. And the equality $U_{cy2}=U_{cy1}$ will be set up when $b_1+b_3=0$. So there will have much lower critical wind velocity when the third-order coefficient of the fitting polynomial is also considered.

Submitting the fitting data presented in Fig. (4) into Eq. (18), the galloping critical wind velocities are obtained with respect to the damping ratio for different ice-shape conductors. The results are shown in Fig. (6). From Fig. (6), the critical wind velocity of conductor galloping increases as the damping ratio increases. And the magnitude of the critical wind velocity calculated by $U_{cy2}$ is lower than by $U_{cy1}$. For D-shape iced conductor (Fig. 6(a)), the critical wind velocity decreases about 8.3 % by $U_{cy2}$ than by $U_{cy1}$. And for U-shape iced conductor (Fig. 6(b)) and aerofoil-shape iced conductor (Fig. 6(c)), it decreases about 7.1 % and 9.5 %, respectively. The lower critical wind velocity denotes the easier conditions to excite conductor galloping, which need to improve the exiting anti-galloping design for transmission lines.

Fig. 7 shows a contrast on the galloping critical wind velocity with the given three ice-shape conductors. From the results, it is obvious that ice shape presents a significant influence to galloping occurrence. The D-shape iced conductor is more liable to galloping phenomenon than the other two ice shapes, which coincide with the results by field observations and tests. In the same conditions, the U-shape iced conductor has the maximum galloping critical wind velocity. Actually, the U shape and the airfoil shape are two more familiar eccentric ice accretions on conductors than the D shape. But the D-shape iced conductor is mostly applied in galloping tests and simulations just because it is much easier to excite conductor galloping phenomenon.

Fig. 6Galloping critical wind velocity vs. damping ratio for the three ice-shape conductors

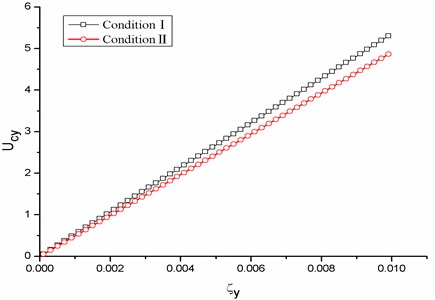
(a) D-shape iced conductor

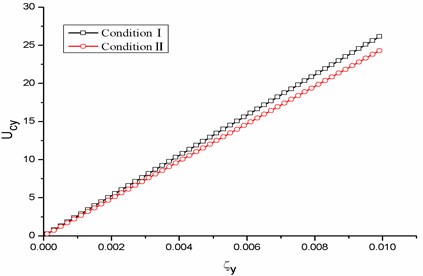
(b) U-shape iced conductor

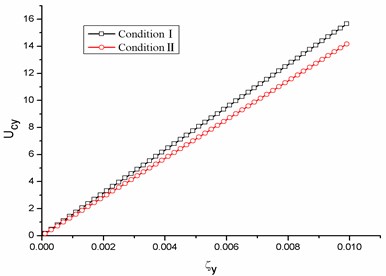
(c) Aerofoil-shape iced conductor

Fig. 7Contrast on galloping critical wind velocity with the three ice-shape conductors

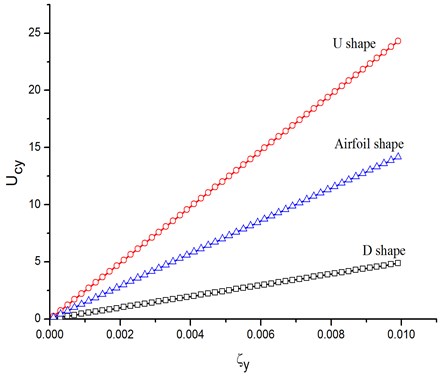
Fig. 8Galloping equivalent amplitude vs. damping ratio and reduced wind velocity for the three ice-shape conductors

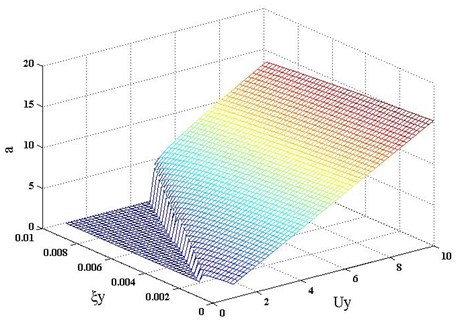
(a) D-shape iced conductor

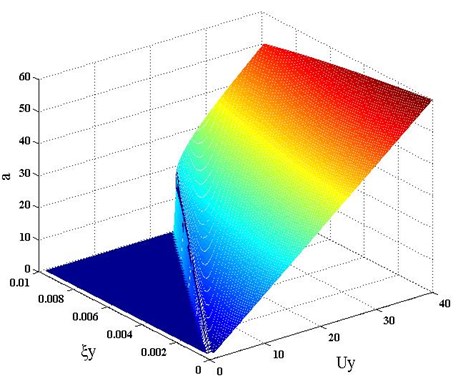
(b) U-shape iced conductor

(c) Aerofoil-shape iced conductor

4. Conclusions

To analyze the factors influencing conductor galloping for the transmission lines, three dimensionless parameters, reduced wind velocity, damping ratio and reduced mass ratio are proposed based on the conductor galloping model. The expression of galloping equivalent amplitude is derived and the analytic solutions are obtained when the aerodynamic curve is fitted by cubic and quintic polynomials respectively. Then the influence of dimensionless parameters to the conductor galloping is obtained by using the aerodynamic forces of three typical iced conductors with U-shape, D-shape and airfoil shape. A new more accurate formula to calculate the galloping critical wind velocity is proposed and the influence of the reduced wind velocity and the damping ratio to the galloping equivalent amplitude is studied. And from the results, we can obtain the following conclusions.

(1) Reduced wind velocity $U_y$ and damping ratio ${\xi }_y$ are two important parameters to affect the conductor galloping characteristics besides the aerodynamic parameters. The study on the two parameters can provide the convenience for the anti-galloping design of analyzing and selecting effective design conditions and structural parameters.

(2) When the third-order coefficient of the fitting polynomial for the aerodynamic curve is also considered, the galloping critical wind velocity is much lower than the magnitude calculated by Den Hartog criterion.

(3) In the same conditions, the galloping critical reduced wind velocity has the relation, $U_{Cy-D}<U_{Cy-Airfoil}<U_{Cy-U}$, for the three typical eccentric iced conductors with D-shape, U-shape and airfoil shape.

(4) The reduced wind velocity has a much obvious influence to the galloping equivalent amplitude. The amplitude increases as the wind velocity increases, which show an approximate linear relationship.

(5) The influence of damping ratio to the galloping equivalent amplitude is limited due to the small self-damping. But the influence to the critical wind velocity is conspicuous, and the critical wind velocity increases as the damping ratio increases.