Modelling of the ground motion of the maximum probable earthquake and its impact on buildings, Vilnius city

3.1. Seismic properties of the geological section

There are several deep wells reaching (or nearly reaching) the crystalline basement in Vilnius area (wells Vilnius-1, Jačionys-299, A. Paneriai, Šiaudinė-707) that provide quite good knowledge on the geological section of the whole sedimentary cover and underlying basement rocks. The sedimentary cover is of about 500 m thick here. It is composed by (from top to bottom) Quaternary, Lower Cretaceous, Middle Devonian, Lower Silurian, Ordovician, Lower Cambrian, and Ediacarian (Vendian) sediments of different composition (Fig. 2). The Quaternary section is composed of alternating sandy and clayey lithologies. Carbonaceous lithologies (marlstone, limestone, and dolomite) predominate in the upper part of the Pre-Quaternary section, while terrigenous sediments (shale, sandstone, conglomerate) compose the lower part of the Pre-Quaternary sedimentary cover. The basement is represented by highly metamorphosed crystalline rocks, mainly granulites.

Vilnius city is characterised by dissected landscape composed of marginal moraine with overlying and intercalating sandy/clayey deposits. Due to intense urbanisation a large part of the city soil is composed of the technosoil [20].

Fig. 2Composite geological section of Vilnius area

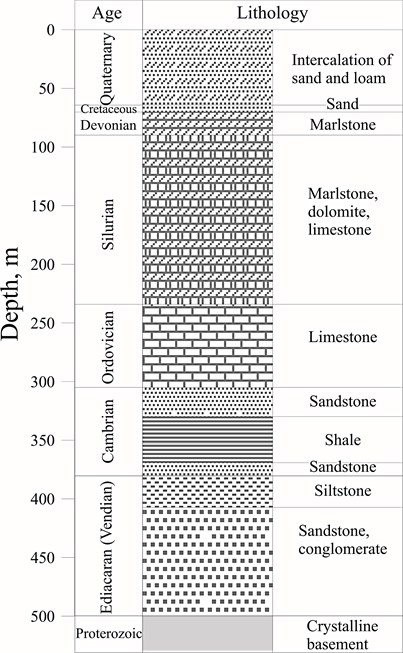
The impact of two marginal types of the soil, i.e. technosoil/sandy soil and moraine (sandy and clayey loam), were modelled to evaluate the seismic ground motion. According to measurements of Ignalina NPP area sandy soil and technosoil are characterised by lower s-wave velocities compared to undisturbed moraine clayey lithologies that inevitably influence the seismic reaction of the ground. The model parameters of the two soil types are presented in Table 1. The soil of Vilnius city is characterised by highly variable composition owing to complex nature of Quaternary sedimentation and urbanisation that also results in variations of seismic properties. For the first approach the two end-member conditions were assumed in the present study.

According to seismic velocity measurements of Ignalina NPP area [13] s-wave velocities generally increase with depth. In the model, s-wave velocities of top interval of 20 m thick range from 150 m/s to 300 m/s for technosoil/sandy soil and from 230 m/s to 380 m/s for moraine soil. Also, the rock densities were measured in shallow and deep wells of Ignalina NPP area and were used in the present study (Table 1).

The s-wave velocities increase with depth owing mainly to rock compaction. The lowest values are measured in the Quaternary section, while they are considerably higher in the Pre-Quaternary succession (Table 2). There are significant variations in velocities against the general depth-related trend that are accounted to lithological variations. The highest velocities in excess of 2000 m/s are measured in Ordovician carbonaceous (limestones and dolomites) layer, while most of the Pre-Quaternary layers have velocities within the range of 1500-2000 m/s. Seismic s-wave velocities dramatically increase to more than 3000 m/s in the crystalline basement underlying the sedimentary cover.

3.2. Free-field ground response spectrum

As described above, in the Step 1 the ground response spectrum was calculated for the crystalline basement using seismic attenuation equation developed by [21]:

here $R$ is taken as a closest distance to the surface projection of the fault (1 km), $M$ is the earthquake magnitude ($M=$ 5.0), $C_n$ are frequency related regression coefficients as presented in [21] (see Appendix 1).

This seismic attenuation function was derived based on abundant seismic instrumental registrations of shallow earthquakes of moderate magnitude from the exposed hard rock regions of USA characterised by similar to Lithuania geological setting.

The spectrum calculated for the earthquake of magnitude $M=$ 5.0 is presented in Fig. 3. The peak ground acceleration (PGA) is calculated 0.43 m/s² (for outcrop conditions). The maximum spectral acceleration values were obtained for frequency domain 10-50 Hz with a peak at 30-40 Hz. Predominance of high frequencies is typical for hard rock type ground [22]. The in-situ PGA value is lower (0.33 m/s²) due to overburden.

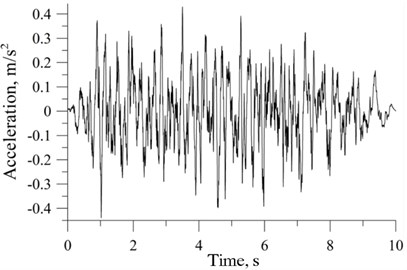
Based on the calculated free-field ground response spectrum the synthetic accelerogram was modelled for the basement rocks as the initial seismic signal (Step 2). The seismic events are of different duration that primarily depends on the magnitude of the earthquake [23]. The enveloping function of the synthetic accelerogram was applied as that recommended by ASCE 4-98 [24], i.e. duration of the ground shaking 10 s with 1 s of rise phase and 3 s of decay phase for an earthquake of magnitude $M=$ 5.0 [24]. The damping value was assumed 5 %. The modelled time history is presented in Fig. 4.

The seismic wave when passing through the overlying sediments transforms, essentially in the uppermost part of the section that accounts mainly to drastic deceleration of s-wave velocities (Fig. 5), which leads to amplification of a wave acceleration (amplitude, velocity) due to energy conservation. This transformation was evaluated in Step 3. The transformation of s-waves passing through the sedimentary cover was alternatively calculated using (1) programme EERA2000 (Equivalent Linear Earthquake Response Analysis) that implements the concepts of equivalent linear earthquake site response analysis and (2) NERA non-linear site response analysis program that is based on non-linear stress-strain relationships developed by [25, 26]. Seismic observations show that the linear models are in better agreement to registered weak seismic motions, while strong motions (acceleration > 1.5 m/s²) are more accurately modelled assuming non-linear soil behaviour [27]. The hypothetical Vilnius earthquake is classified as a moderate seismic event; therefore both models should be taken into consideration.

Fig. 3Free-field ground response spectra calculated for hard-rock basement, earthquake M= 5.0, hypocentral depth 15 km

Fig. 4Synthetic accelerogram modelled for hard-rock basement, earthquake M= 5.0, hypocentral depth 15 m

The wave transformation depends on the seismic properties of the geological layers. Apart of seismic wave velocities, the shear modulus is one of the basic parameters [28]. This parameter was modelled using EERA2000 programme (Fig. 6).

The peak ground acceleration (PGA) only little changes across the depth interval of Palaeozoic and Ediacarian (Vendian) rocks (Fig. 5). The most considerable increase in PGA values occurs in the Quaternary succession, essentially in its uppermost part.

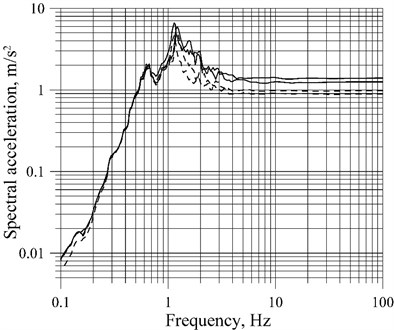
Four free-field ground response spectra were derived from the initial seismic signal passing from the crystalline basement to the surface of two different soil types (Fig. 7). The linear and non-linear models were applied for comparative analysis. The modelled surface spectra (Fig. 7) are considerably different from the basement spectrum (Fig. 2). Assuming linear soil behaviour PGA increases to 1.25 m/s² (maximum relative velocity 0.08 m/s, maximum relative displacement 0.009 m, maximum stress 5.2 kPa) and 1.40 m/s² for moraine and sandy soils, respectively. The non-linear modelling shows somewhat lower PGA values 1.02 m/s² for moraine soil and 1.21 m/s² for sandy soil.

Fig. 5Profiles of maximum shear modulus and s-wave velocities of sedimentary cover, moraine soil, Vilnius area

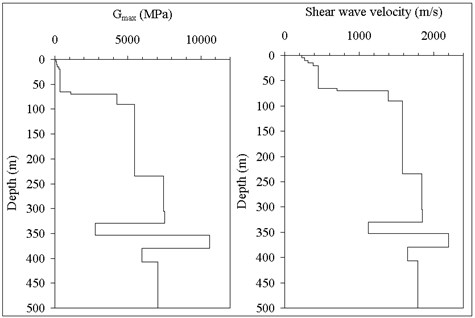
Fig. 6PGA depth profile of the sedimentary cover (moraine soil type of uppermost part of the section), Vilnius area

The shape of surface spectra is considerably different from the basement spectrum, showing shifting of maximum spectral acceleration values from high frequencies (peak 40 Hz) to low frequencies (Fig. 7). In the linear model the maximum spectral acceleration values are identified in the frequency domain 1-2 Hz with a peak value at 1.1-1.2 Hz. The non-linear modelling obtains maximum spectral acceleration values at frequencies 2-5 Hz with a peak value at 3-4 Hz. Shifting from high-frequency dominated to low-frequency dominated ground shaking is characteristic for transition from hard-rock to soft-soil [29].

The acceleration (velocity, amplitude) decreases with depth owing to overburden and increasing s-wave velocities. Therefore the foundation embedment depth is an important parameter in seismic risk analysis. A comparison of free-field ground response spectra and response spectra at the depth of 5 m is presented in Fig. 8. PGA values for the depth of 5 m were calculated as low as 0.89 m/s² and 0.98 m/s² for, respectively, moraine and sandy soils (linear models), which are much lower than acceleration for free field conditions. No discernable changes are recognised in the shape of response spectra.

Fig. 7Free field ground response spectra modelled for moraine and sandy soils. Bold lines correspond to linear soil behaviour models, dashed lines correspond to non-linear models

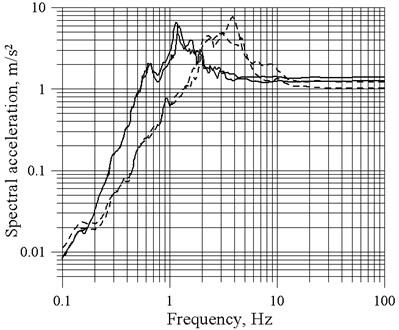
Fig. 8Free field ground response spectra compared to spectra inside the layer at the depth of 5 m (linear soil behaviour model). Bold lines are the same as in Fig. 7, dashed lines show spectra calculated at depth 5 m (sand/technosoil and moraine soils, linear soil behaviour models)

3.3. Discussion

The most important (for buildings reaction) parameters of earthquake ground motions are shaking duration, acceleration (amplitude of displacement and velocity) and frequency of the ground motion.

Modelling of the seismic ground motion of the most probable maximum earthquake of Vilnius area compatible to Kaliningrad earthquake in 2004 infers PGA values as high as 1.25-1.4 m/s² (non-linear model 1.02-1.21 m/s²). These values correspond to macroseismic intensity $I_o=$ 7 (MSK-64) [30, 31], e.g. minor damages of buildings can be expected. This intensity estimate is in concert to epicentral intensity $I_o=$ 6.5-7 defined by macroseismic observations of the Kaliningrad earthquake [32]. The modelled free-field ground response spectrum is dominated by low frequencies in the range of 1-2 Hz for linear soil behaviour, while 2-5 Hz for non-linear stress-strain relationship. Predominance of low frequencies is primarily related to low seismic velocities of soil lithologies. The half-kilometre thick sedimentary cover of type A (rock, Eurocode 8 [33]) only little affects the seismic signal passing upwards from the basements, PGA values are in the range of 0.34-0.45 m/s² (overburden conditions). The main seismic wave modification takes place in the uppermost part of the Quaternary succession classified as soil of type B and C (soil and soft soil, Eurocode 8).

In terms of the seismic risk there is a correlation between the seismic frequencies and building height via the shaking resonance. Different buildings may respond in a different way to the same earthquake ground motion depending on its characteristics. Taller buildings have longer natural periods compared to low buildings (Table 3), also the building construction is of importance [34-36].

Vilnius free-field seismic ground response spectra are dominated by low frequencies in the range of 1-5 Hz. It implies that local earthquake will most strongly affect 2-10 store buildings.