Study on standard response spectrum parameters of special long-period ground movements

3.1. Acceleration response spectrum

Fig. 1 demonstrates a comparison of acceleration response spectra under three types of earthquake action. The shapes of seismic acceleration spectra under NFPL long-period and common ground movements are close to each other. That is to say, they both experienced three stages: rapid growth → rapid decrease → slow decrease. The increase and decrease of seismic acceleration spectrum under FFH long-period ground movements are relatively slow. When the fundamental period of a SDOF system is beyond 8 s, the acceleration spectrum basically remains unchanged. On the whole, the seismic acceleration spectrum under NFPL long-period ground movements is higher than those under common and FFH long-period ground movements. The peak of acceleration time-history under FFH long-period ground movements attenuates rapidly with the increase of distance, which leads to its low acceleration spectrum. The peaks of the normalized acceleration spectra under common, NFPL and FFH long-period ground movements are close to each other, and they are 2.377, 2.370 and 2.339 respectively. However, the fundamental periods of a SDOF system corresponding to spectrum peaks have significant differences. The normalized acceleration spectrum under common ground movements reaches the peak at 0.18 s, while those under NFPL and FFH long-period ground movements reach the peaks at 0.52 s and 1.48 s respectively. In addition, the normalized acceleration spectra under long-period ground movements are relatively dispersed throughout the fundamental periods, and they are not concentrated in the period range of $T<$1 s as that under common ground movements. When $T$ is less than 0.42 s, the normalized acceleration spectra under long-period ground movements are smaller than that under common ground movements. When $T$ is beyond 0.56 s, spectra values under long-period ground movements are larger than that under common ground movements.

3.2. Velocity response spectrum

Fig. 2 illustrates a comparison of velocity response spectra under three types of earthquake action. The shapes of seismic velocity spectra under long-period and common ground movements are close to each other. That is to say, they all experienced two stages: rapid growth → slow decrease. On the whole, the seismic velocity spectrum under NFPL long-period ground movements is higher than those under common and FFH long-period ground movements. The peak of acceleration time-history under FFH long-period ground movements attenuates rapidly with the increase of distance, which leads to the low integral velocity spectrum. When $T$ is beyond 1.0 s, seismic velocity spectrum under NFPL long-period ground movements is about 2 times of that under common ground movements, while the spectrum under FFH long-period ground movements is only half of that under common ground movements. When $T$ is less than 0.8 s, the normalized velocity spectra under long-period ground movements are similar to that under common ground movements. When $T$ is beyond 0.8 s, spectra values under long-period ground movements are larger than that under common ground movements. The fundamental periods corresponding to the peaks of velocity spectra under long-period ground movements are obviously larger than that under common ground movements. The normalized velocity spectrum under common ground movements reaches the peak at 0.88 s, while those under NFPL and FFH long-period ground movements reach the peaks at 6.14 s and 6.36 s respectively.

Fig. 1Acceleration response spectra

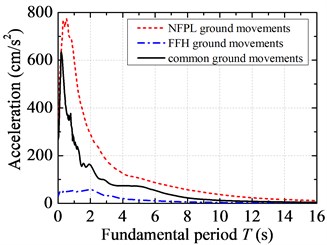
a) Seismic acceleration spectra

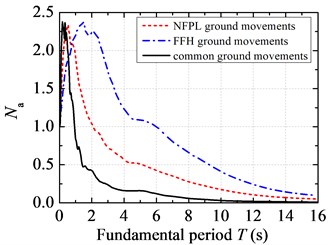
b) Normalized acceleration spectra

Fig. 2Velocity response spectra

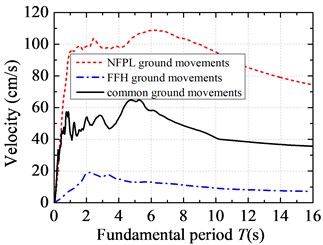
a) Seismic velocity spectra

b) Normalized velocity spectra

3.3. Displacement response spectrum

Fig. 3 demonstrates a comparison of displacement response spectra under three types of earthquake action. The shapes of seismic displacement spectra under NFPL long-period ground movements and common ground movements are close to each other. That is to say, they both experienced two stages: rapid growth → slow decrease. The seismic displacement spectrum under FFH long-period ground movements changes slightly with the increase of fundamental period. It increases slowly when $T$ is less than 4.0 s and remains unchanged after 4.0 s. The peak of acceleration time-history under FFH long-period ground movements attenuates rapidly with the increase of distance, which leads to a low value of quadratic-integral displacement spectrum. On the whole, the seismic displacement spectrum under NFPL long-period ground movements is higher than those under common and FFH long-period ground movements. When $T$ is less than 8.88 s, seismic displacement spectrum under NFPL long-period ground movements increases rapidly with the increase of fundamental period. And it is about 2.8 and 9.9 times of those under common and FFH long-period ground movements after 8.88 s. When $T$ is less than 0.56 s, the normalized displacement spectra under long-period ground movements are similar to that under common ground movements. When $T$ is beyond 0.56 s, the spectra under long-period ground movements are larger than that under common ground movements. The normalized displacement spectrum under FFH long-period ground movements is larger than that under NFPL long-period ground movements. The normalized displacement spectrum under FFH long-period ground movements increases rapidly when $T$ is less than 8.82 s and decreases rapidly after 8.82 s. When fundamental period is 8.82 s, the spectrum value under FFH long-period ground movements reaches the peak of 1.093. And it is about 12.1 and 2.4 times of those under common and NFPL long-period ground movements.

Fig. 3Displacement response spectra

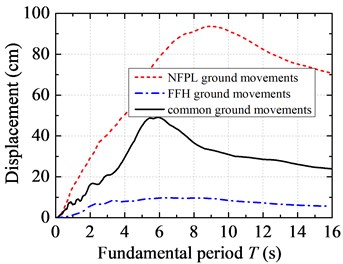
a) Seismic displacement spectra

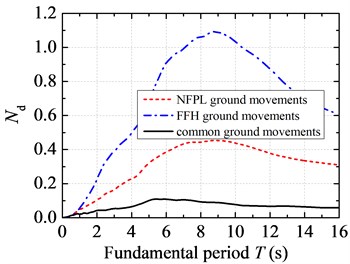
b) Normalized displacement spectra

4.1. Standard response spectra of long-period ground movements

The acceleration response spectrum of actual earthquake records is smoothed and normalized to the form of code design spectrum, and this process is called the calibration of acceleration response spectrum. Currently, the least-square and minimization method for fitting and calibrating response spectrum is the most widely used in the seismic design of buildings. The standard deviation between the fitted response spectrum calibrated by the least-square and minimization method and the original response spectrum is the lowest. And the shape of the fitted spectrum is very similar to that of original spectrum. Therefore, the least-square and minimization method can be employed to calibrate the normalized acceleration spectra about two types of long-period ground movements.

Zhou et al., who employed the earthquake influence coefficient as the evaluation index of design spectra curves, evaluated the reliability of long-period segments of seismic design spectra [24]. The significant difference between this paper and the reference is that the dynamic magnification factor is used here for the standard response spectrum of long-period ground movements. Both of them present similar four-segment curves. According to the curve shape of the current code design spectrum usually applied in China [25], the calibrated standard response spectrum by the segmented least-square and minimization method is composed of four segments, which can be shortened as:

where, ${\beta }_{\mathrm{m}\mathrm{a}\mathrm{x}}$ is the maximum value of dynamic magnification factor (i.e. platform segment height), and platform value is generally within 2-3; $T_g$ is the characteristic period (i.e. the period in the second inflection point), which can be determined by site condition, earthquake magnitude and epicentral distance. According to the influence of earthquake magnitude and epicentral distance, the design earthquake is divided into three groups in China's specification. So, the characteristic period can be determined by site classifications and design earthquake groups.

The least-square and minimization method is a mathematical optimization technique, and it finds the best function match of the data by minimizing the sum of squares of errors. The least-square and minimization method can be used to easily obtain the unknown data, and minimize the sum of squares of errors between the obtained data and the actual data. In this paper, by searching for the best two characteristic parameters (platform value and characteristic period), the sum of squares of errors between the fitted response spectrum and the actually normalized acceleration spectrum is minimized. Further, the calibrated standard response spectra under NFPL and FFH long-period ground movements as shown in Fig. 4 and Fig. 5 can be determined:

where, $S_i$ is the fitted spectrum; ${\stackrel{-}{S}}_i$ is the statistical average spectrum. ${\beta }_{\mathrm{m}\mathrm{a}\mathrm{x}}$ and $T_g$ are automatically processed by minimizing $Q$. That is, the solution of Eq. (3) needs to be satisfied:

In this paper, the earthquake records in the C and D site classifications of NFPL long-period ground movements and C, D and E site classifications of FFH long-period ground movements are selected for the next study regardless of magnitude and distance. And the C, D and E site classifications in NEHRP correspond to the II, Ⅲ and IV site classifications in China’s code, respectively. The seismic design spectrum are extended to 10 s in the long-period segment according to the existed stretching trend of the current code. At the same time, the average normalized acceleration spectra of long-period ground motion records are drawn with corresponding fitted spectra in the same coordinate system.

4.1.1. Standard response spectra of NFPL long-period ground movements

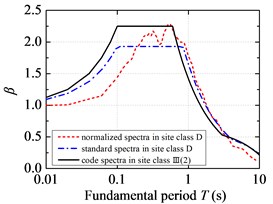
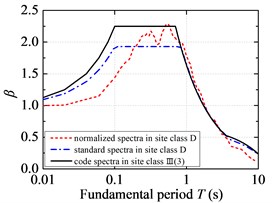
Fig. 4 illustrates the comparison of standard response spectra of NFPL long-period ground movements with the current code design spectra. On the whole, the code design spectrum overestimates the structural seismic response in the short period under NFPL long-period ground movements, while it underestimates the structural seismic response in the long period under NFPL long-period ground movements. However, the maximum value of structural fundamental period in the current code only reaches 6 s. It is suggested that the fundamental period of a SDOF system should be extended to consider the influence on long-period structures acted by a long-period earthquake. By comparing the standard response spectra in the C site classification of NFPL long-period ground movements with the seismic design spectra in the II site classification, the gaps between the fitted platform values of standard response spectra and those of code design spectra are very low, yet the fitted characteristic periods are greatly different from those of the current code. When the same are compared in the D site classification of NFPL long-period ground movements with the seismic design spectra in the Ⅲ site classification, the fitted platform values of standard response spectra are greatly different from those of code design spectra, yet the gaps between the fitted characteristic periods and those of the current code are very low. When the fundamental period of a SDOF system is beyond 0.8 s, the code design spectrum seriously underestimates the structural seismic response under NFPL long-period ground movements in the first design earthquake group, while it slightly underestimates the structural response under NFPL long-period ground movements in the third design earthquake group.

Fig. 4Standard response spectra of NFPL long-period ground movements and code design spectra: a) the first design earthquake group; b) the second design earthquake group; c) the third design earthquake group

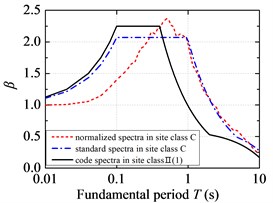
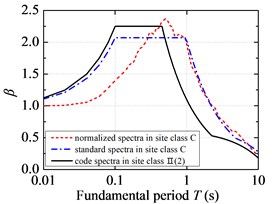
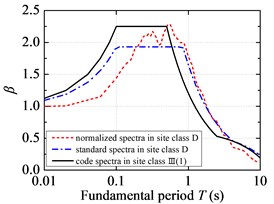
a)

b)

c)

4.1.2. Standard response spectra of FFH long-period ground movements

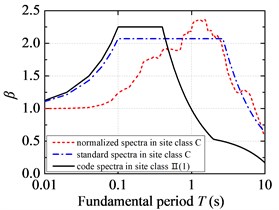
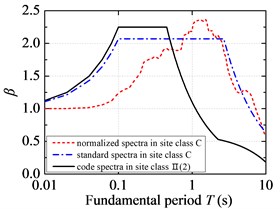
Fig. 5 demonstrates the comparison of standard response spectra of FFH long-period ground movements with the current code design spectra. The standard response spectra of FFH long-period ground movements are less than the current code design spectra in the short period. Considering the code design is based on a certain low probability of exceedance, so it is reasonable to design short-period structures by adopting the current code for seismic design of buildings. However, the standard response spectra of FFH long-period ground movements are larger than the current code design spectrum in the medium-long and long period, and the corresponding probability of exceedance is relatively high from the probability point of view. So it is unsafe to design structures with medium-long and long fundamental period if the current code for seismic design of buildings is adopted. For FFH long-period ground movements with long-distance propagation, it contains more long-period components due to the filtering effect of low-frequency amplification and high-frequency attenuation. The period corresponding to the inflection point in the peak region of standard response spectra is shifted to the long-period direction, and it is suggested that the long-period part of standard response spectra should not be segmented and the attenuation index should be always taken as 0.9. The fitted characteristic periods of standard response spectra under FFH long-period ground movements are much larger than that of the current code design spectrum. The fitted platform values of standard response spectra in the C and D site classifications are around 2.05, which is lower than that of the current code design spectrum. However, the fitted platform values in the E site classification of FFH long-period ground movements are slightly higher than specification due to the soft-soil filtering effect.

Fig. 5Standard response spectra of FFH long-period ground movements and code design spectra: a) the first design earthquake group; b) the second design earthquake group; c) the third design earthquake group

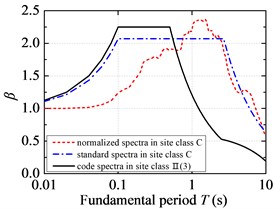
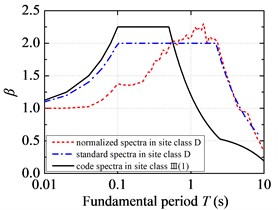
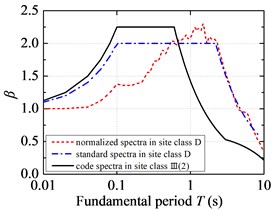
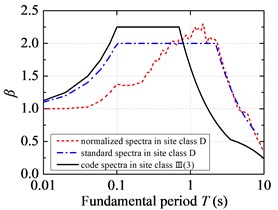
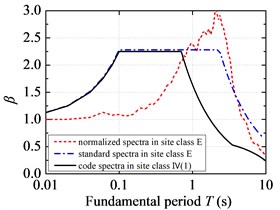
a)

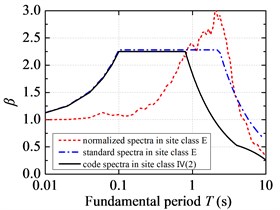
b)

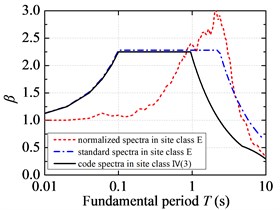
c)

4.2. Comparison on characteristic parameters of response spectra

The platform value and characteristic period are two important characteristic parameters of seismic design spectrum. The platform value can reflect the amplitude intensity of earthquake action, while the characteristic period can reflect the spectrum characteristic of earthquake action. The platform value is related to a variety of factors, and the site condition is one of the most important factors.

Table 4 illustrates the characteristic parameters of platform value and characteristic period about the standard response spectra in various site classifications under long-period ground movements. Table 5 illustrates the characteristic parameters of platform value and characteristic period about the code design spectra in various site classifications and design earthquake groups.