The analysis of the destabilizing motion of a hyperbolic cooling tower during demolition blasting

2.1. Theory of tensile-compression elastic-plastic constitutive model

The tensile-compression elastic-plastic model, which possesses the characteristics of element size independence and offers the advantages of customizable principal models, demonstrates a superior ability to accurately depict the mechanical properties of concrete materials when compared with other concrete material models in the DYNA material library [14]. The Von-Mises yield criterion serves as the assessment standard for material failure within this model:

where: $J_2$ is the second bias stress tensor invariant; ${\sigma }_{yield}^{}$ is the yield stress; ${\epsilon }_{eff}^p$ is the effective plastic strain; $E_p$ is the plastic hardening modulus.

The yield stress and effective plastic strain in the two states of tensile and compressive are represented by the two load curves defined by this model, $f_t\left(p\right)$ and $f_c\left(p\right)$, respectively. It is chosen whether to follow the compression curve or the tensile curve when these two pressure values, pt and pc, are exceeded. The weighted average of the two curves is utilized if the pressure is in the range between these two values. This can be said in the following way:

where: $f_t\left(p\right)$ is the tensile yield stress and effective plastic strain curve; $f_c\left(p\right)$ is the compressive yield stress and effective plastic strain curve; $P_t$ is the tensile stress cut-off value; $P_c$ is the compressive stress cut-off value; $s$ is the weighted average; $d^e$ is the damage variable related to plastic strain; $d^p$ is the damage variable related to pressure.

2.2. Parameters of the tensile-compression elastic-plastic constitutive model

The tensile-compression elastic-plastic model has 22 parameters, as shown in Table 1.

In the table: MID is the material number; RO is the material density; E is the material modulus of elasticity; PR is the Poisson’s ratio; C and P are the strain rate parameters; FAIL is the material failure parameter; TDEL is the minimum time step parameter; LCIDC is the compressive load curve; LCIDT is the tensile load curve; LCSRC is the compressive strain rate curve; LCSRT is the tensile strain rate curve. SRFLAG is the rate effect algorithm; LCFAIL is the material failure curve; EC is the material compressive modulus of elasticity; RPCT is the mean stress parameter; PC is the compressive mean stress; PT is the tensile mean stress; PCUTC is the compressive stress truncation; PCUTT is the tensile stress truncation; PCUTF is the stress truncation activation parameter; K is the bulk modulus.

For using the model for numerical simulation computations, a minimum of eight parameters (RO, E, PR, FAIL, LCID, LCIDT, LCSRC, LCSRT) must be established, with the system default values being utilized for the remaining parameters.

2.3. Verification of the applicability of the tensile-compression elastic-plastic model

For the numerical calculations of the reliability research, the displacement controlled element loading method is utilized with the C30 concrete model and a positive hexahedral solid element with a side length of 150 mm. The Reinforced Concrete Code is used to determine the material characteristics. Uniaxial tensile, uniaxial compression, triaxial compression, and element size effect tests were done on the single element, respectively. In addition, the element was subjected to strain rate testing for uniaxial compression and tensile at strain rates of 10^-5S^-1 and 10^-2S^-1, respectively. Fig. 2 displays the model testing' outcomes.

Fig. 1Test element geometry model and finite element model

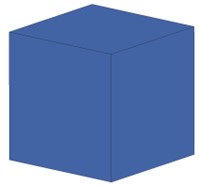
As seen in Fig. 2, the tensile-compression elastic-plastic material model can simulate the mechanical properties of concrete materials under a variety of circumferential pressure conditions, including uniaxial tension, uniaxial compression, triaxial compression, and different circumferential pressure conditions. It also has the advantage of being independent of the unit size effect, which can make it better applied to the numerical calculation of demolition of concrete-like building structures.

Fig. 2tensile-compression elastic-plastic model test

a) Uniaxial tensile stress-strain curve

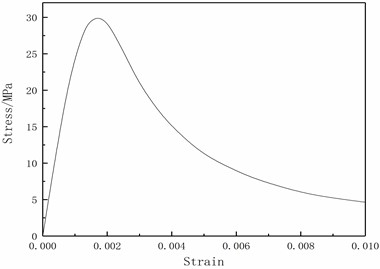
b) Uniaxial compression stress-strain curve

c) Triaxial compression stress-strain curve

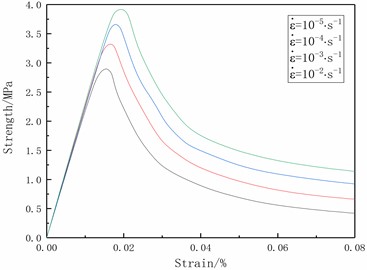
d) Strain rate effect under tension

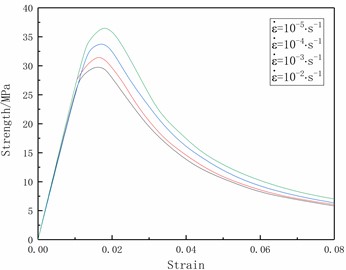
e) Strain rate effect under compression

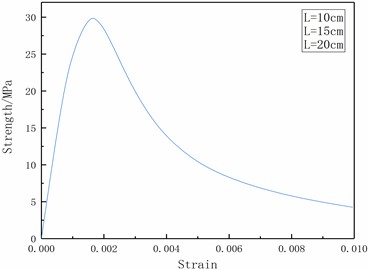
f) Compression dimensional effect on the element

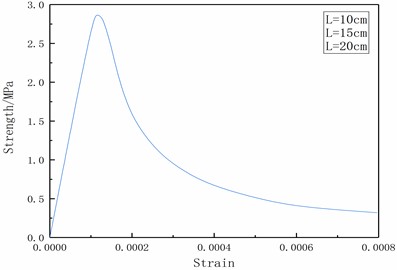
g) Tensile dimensional effect on the element

3.1. Numerical modeling

3.1.1. Project overview

A power plant's double-curved cooling tower required to be destroyed by blasting in order to comply with the national “low carbon emission reduction” development policy. The cooling tower has a total of 36 pairs of herringbone columns with a height of 4.8 m, spacing of 5.36 m, and cross-sectional dimensions of 0.4 m×0.4 m. It is a double-curved, thin-walled reinforced concrete structure with a height of 75 m, concrete grade C30, a top radius of 18.38 m, and a bottom radius of 30.76 m. Herringbone columns are reinforced at a rate of 0.48 %, and tower walls at a rate of 0.63 %. Fig. 3 depicts the double-curved cooling tower that needs to be dismantled.

Fig. 3Double curve cooling tower to be dismantled

3.1.2. Blasting design program

The double curve cooling tower blasting demolition program was created in accordance with the cooling tower design drawings, the cooling tower blasting demolition location, and the surrounding environment. Table 3 lists the relevant parameters.

Table 3Incision design parameters

Incision elements	Incision parameters
Incision shape	Composite incision
Incision angle	220°
Incision height	17.3 m
Time Difference Settings	8 explosive areas 4 segments

Table 4Cooling tower herringbone column blasting parameters

Geometric size / m	Hole depth / m	Hole distance / m	Hole layout	Single hole dosage / g	Number of holes	Total Explosives / kg
0.40×0.40	0.27	0.3	Single row	70	748	52.36

Fig. 4Cooling tower cutout time difference parameters

3.1.3. Numerical model building

In order to establish a reasonable numerical model of double-curved cooling tower demolition, herringbone column, and tower wall models are established respectively, and the keyword *CONSTRAINED- NODAL-RIGID-BODY is used to treat the nodes of the connection surface as nodal rigid bodies at the interface between the herringbone column and the ring beam, and the transfer of forces and displacements in the two regions is achieved by controlling the coordination of the displacements and corners of these nodal rigid bodies.

The literature [15] is cited to consider the role of reinforcement rate, and the parameters of the herringbone column and tower wall are finally determined as shown in Table 5 and Table 6, respectively. The density of the herringbone column, ring beam, and tower wall is 2400 kg/m³ and Poisson’s ratio is 0.2. A rigid body model is used for the ground. Automatic single-sided contact is used between the herringbone column and the tower wall and the ground.

Table 5Concrete material parameters for herringbone columns

RO	E	PR	FAIL
2400 kg/m3	31.02GPa	0.20	0.02
LCIDC		LCIDT
0.0000	3.03MPa	0.00	1.20 MPa
1.47×10-3	33.73 MPa	9.00×10-5	2.57 MPa
3.69×10-3	30.02 MPa	1.16×10-4	2.90 MPa
6.35×10-3	24.65 MPa	1.86×10-4	1.71 MPa
8.96×10-3	20.75 MPa	2.36×10-4	1.23 MPa
1.15×10-2	17.97 MPa	2.86×10-4	0.96 MPa
1.41×10-2	15.90 MPa	3.36×10-4	0.79 MPa
1.66×10-2	14.31 MPa	3.86×10-4	0.67 MPa
1.91×10-2	13.05 MPa	4.36×10-4	0.59 MPa
2.16×10-2	12.03 MPa	4.86×10-4	0.53 MPa
2.40×10-2	11.17 MPa	5.36×10-4	0.48 MPa
2.65×10-2	10.45 MPa	9.86×10-4	0.28 MPa

Table 6Concrete material parameters of tower wall

RO	E	PR	FAIL
2400 kg/m3	31.22 GPa	0.20	0.02
LCIDC		LCIDT
0.00	2.97 MPa	0.00	0.30 MPa
5.18×10-4	13.82 MPa	2.14×10-7	1.49 MPa
8.58×10-4	22.35 MPa	1.05×10-6	2.07 MPa
1.21×10-3	26.92 MPa	4.33×10-6	2.57 MPa
1.43×10-3	29.21 MPa	1.41×10-5	2.88 MPa
2.80×10-3	34.70 MPa	1.67×10-4	2.02 MPa
3.77×10-3	33.62 MPa	2.01×10-4	1.48 MPa
5.99×10-3	31.12 MPa	2.46×10-4	1.16 MPa
6.72×10-3	27.54 MPa	3.06×10-4	0.87 MPa
1.04×10-2	21.74 MPa	3.76×10-4	0.69 MPa
1.99×10-2	14.39 MPa	5.54×10-4	0.47 MPa

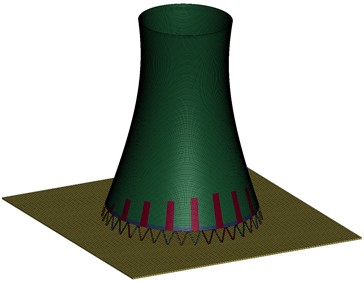
According to the above modeling ideas and parameter selection to establish the numerical simulation calculation model of the hyperbolic cooling tower, as shown in Fig. 5.

Fig. 5Calculation model of hyperbolic cooling tower

3.2. Verification of natural vibration frequency of the cooling tower

In order to verify the reasonableness of the numerical simulation model, the modal analysis of the established numerical simulation model is carried out implicitly using the solver, and the first 5 orders of modalities of the model are extracted as shown in Table 7, and the three views of the 1st order vibration pattern are shown in Fig. 6.

The minimum natural vibration frequency of a hyperbolic cooling tower can be calculated using the following empirical formula [15]:

where: $h_{\mathrm{m}\mathrm{i}\mathrm{n}}$ is the minimum cylinder wall thickness; $D_{\mathrm{m}\mathrm{i}\mathrm{n}}$ is the minimum cylinder body diameter.

According to the design parameters of the double-curved cooling tower, the natural vibration frequency of the cooling tower can be calculated from the empirical formula as 1.31 Hz. Literature [16] found that if only the effect of the minimum natural vibration frequency of the cooling tower is considered, the increase in the calculated natural vibration frequency should be less than 10 %, and the natural vibration frequency calculated in this model meets this requirement, and it can be approximated that this calculation model can better reflect the mass distribution and stiffness characteristics of the cooling tower.

Fig. 6Three views of the 1st-order vibration pattern of the hyperbolic cooling tower

a) Three views of 1st order vibration pattern

b) 1st order vibration $XY$ axis view

c) 1st order vibration $YZ$ axis view

d) 1st order vibration type $XZ$ axis view

3.3. Calculation simulation verification

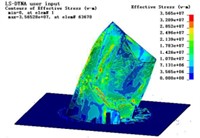
According to the actual blasting construction plan for the double-curved cooling tower calculation model for incision setting and gravity loading, double-curved cooling tower blasting and demolition of the actual collapse process and numerical calculation of the collapse process are shown in Fig. 7.

To quantitatively reflect the destabilization motion of the double-curved cooling tower in the demolition and blasting, the distances and relative deformations of the actual collapse process and the numerical calculation process of the cooling tower at $t=$1.6 s, $t=$3.3 s, and $t=$5.0 s, respectively, are shown in the following table.

Fig. 7 and Table 8 can be used to analyze the actual collapse process and numerically calculate the collapse process of the demolition of the double-curve cooling tower. The reserved herringbone column begins to falter at $t=$ 1.6 s because it is unable to support the weight of the top construction. The cooling tower's real collapse process had a vertical relative deformation of 2.7 % for the higher displacement observation point, while the numerical computation process had a vertical relative deformation of 3.3 %.

The real vertical relative deformation of the cooling tower collapse process for the lower displacement observation point is 0.36 %, whereas the vertical relative deformation of the numerical calculation process is 7.2 %. At $t=$ 2.0 s, following the incision’s closure, the tower wall exhibits plastic deformation, the tops of the tower wall columns on either side of the unloading tank show damage, and under the influence of the touchdown reaction force, there is a loss of bearing capacity due to in-plane deformation. As a result, the obvious phenomenon of flexural deformation occurs in the middle of the sidewall, and the stable structure of the double curve is damaged. The cooling tower is still collapsing at time $t=$3.3 s. Both the vertical relative deformation of the actual collapse process and the vertical relative deformation of the cooling tower’s numerical computation process are 2.7 % for the upper displacement observation point. The actual cooling tower collapse process vertical relative deformation for the lower displacement observation point is 2 %, while the numerical calculation process vertical relative deformation is 3.6 %. At time $t=$4.0 seconds, the cooling tower’s flexural deformation line extends to the top of the tower, and the tower wall starts to exhibit the initial folding phenomenon. Both the vertical relative deformation of the cooling tower’s actual collapse and the vertical relative deformation of the numerical computation process are 3.3 % at time $t=$5.0 s for the highest displacement observation point. The cooling tower’s real collapse process had a vertical relative deformation of 11.23 % for the lowest displacement observation point, while the numerical computation process had a vertical relative deformation of 16.5 %. During time $t=$6.0 s, the rear wall’s centre and the directional window’s location experienced fracture damage, leading to the formation of a “inverted V-shaped” retained part. At time $t=$8.0 s, the cooling tower’s fracture and decomposition during the collapse process are complete, resulting in the formation of a burst pile that is only a little bit larger than the bottom diameter.

Fig. 7Actual collapse process and numerical calculation process of double-curved cooling tower

a)$\mathrm{t}=$ 0 s

b)$\mathrm{t}=\mathrm{}$1.6 s

c)$\mathrm{t}=\mathrm{}$2 s

d)$\mathrm{t}=\mathrm{}$3.3 s

e)$\mathrm{t}=\mathrm{}$4 s

f)$\mathrm{t}=\mathrm{}$5 s

g)$\mathrm{t}=\mathrm{}$6 s

h)$\mathrm{t}=\mathrm{}$8 s

Due to the incision formation process of the numerical calculation model using the element deletion method, which completely eliminated the support of the incision area, in addition, did not consider the air resistance, cooling tower construction quality and weathering degree of influence, the collapse process of the cooling tower numerical calculation is slightly faster than the actual collapse process, from the incision formation, incision closure, tower wall deflection touching the ground, the deformation of the tower wall flexion, to the crushing and disintegration of the tower wall, The numerical calculation process of the cooling tower and the actual collapse process did not achieve complete morphological similarity, but the actual collapse process of the cooling tower exhibited a high level of agreement with the numerical calculation results in terms of both collapse time and collapse morphology. This serves as validation for the applicability of the model in simulating concrete-type building structures.

4.1. Layout of measurement points

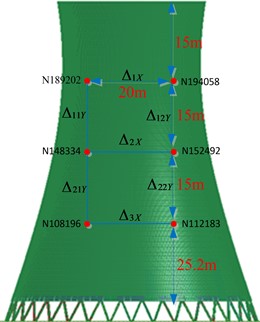
The height direction of the cooling tower is divided into 15 m intervals, where a profile with two nodes is captured. The horizontal distance between these nodes is set at 20 m to ensure a comprehensive and systematic analysis of the buckling deformation during the collapse process. The upper profile consists of nodes N189202 and N194058, the middle profile includes nodes N148334 and N152492, while the lower profile is composed of nodes N108196 and N112183. The horizontal distances are assigned values of ${\mathrm{\Delta }}_{1X}$, ${\mathrm{\Delta }}_{2X}$, and ${\mathrm{\Delta }}_{3X}$ for the node pairs N189202-N194058, N148334-N152492, and N108196-N112183 respectively. The vertical distance between nodes N189202 and N148334 is denoted as ${\mathrm{\Delta }}_{11Y}$,, the vertical distance between nodes N194058 and N152492 is denoted as ${\mathrm{\Delta }}_{12Y}$, the vertical distance between nodes N108196 and N148334 is denoted as ${\mathrm{\Delta }}_{21Y}$, and the vertical distance between nodes N112183 and N152492 is denoted as ${\mathrm{\Delta }}_{22Y}$. The schematic representation in Fig. 9 illustrates the observation locations on the tower, enabling analysis of variations in horizontal distance at the same height and vertical distance at different heights over time.

Fig. 8Cooling tower displacement observation point schematic diagram

4.2. The study of deformation in hyperbolic cooling tower

The flexural deformation in the cylinder during the cooling tower collapse process is appropriately induced by the out-of-plane disturbance. The Fig. 9 illustrates the temporal evolution of $X$-$Y$ coordinates for each measurement point in accordance with the collapse movement of hyperbolic cooling tower.

The distance and relative deformation in the $X$-direction and $Y$-direction of each measurement point at the times of $t=$1.6 s, $t=$3.3 s, and $t=$5.0 s were computed as shown in Table 9 to quantitatively analyze the destabilizing motion of the hyperbolic cooling tower during demolition blasting.

The data presented in Table 9 demonstrates the gradual inclination of the cylinder due to gravitational forces. At $t=$1.6 s, it is observed that there is no relative $X$ deformation in the upper section, while the middle and lower sections experience a compression deformation of 1 % $X$ and 1.5 % $X$ respectively. In the $Y$ direction, there was no comparable deformation observed in the upper left or right cross-sections. The lower right cross-section exhibited negligible relative $Y$-direction deformation, whereas the lower left cross-section experienced a compressive deformation of 0.6 %. At $t=$3.3 s, the upper cross-section experiences a 5.5 % deformation in the $X$-direction, while the middle cross-section undergoes a 0.5 % deformation in the same direction. The lower cross-section also deforms by 2 % in the $X$-direction. In terms of $Y$-direction deformations, the top left section exhibits a compressive deformation of 2 %, whereas there is no relative deformation observed in the upper right section. On the other hand, the bottom left cross-section experiences a tensile deformation of 7.3 %, and finally, the lower right cross-section shows a compressive deformation of 4.7 % in the Y direction. At $t=$5 s, the upper section exhibits a tensile deformation of 10.5 % in the $X$ direction, while the middle segment experiences a 5.5 % tensile deformation in the same direction. Conversely, the lower section undergoes a compressive deformation of 5 % in the $X$ direction. The upper left cross-section is subjected to a compression of 9.3 % in the $Y$ direction, whereas the upper right cross-section experiences a compression of 1.3 % in that same direction. Similarly, the bottom left area is compressed by 14.6 % in the $Y$ direction, while the lower right section is compressed by 16 %.

Fig. 9Cooling tower measurement point X-Y direction coordinate time curve

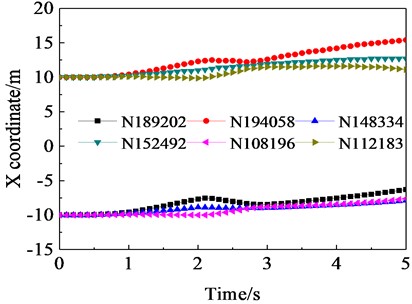
b)$X$ direction coordinate time curve

a)$Y$ direction coordinate time curve

The instability and collapse of the cooling tower indicate that the in-plane deformation caused by out-of-plane perturbation destabilizes both the horizontal and vertical directions of the side wall. Additionally, it can be observed that tensile perturbations primarily affect the upper and middle sections of the cylinder, while compressive perturbations mainly impact the vertical section. The upper section is undergoing tensile deformation in the horizontal direction, with the intensity of deformation gradually increasing. The middle section initially experiences compression and then transitions to tensile deformation. Lastly, the lower section undergoes a process of compression-tension-compression deformation. The upper left section underwent compression, with the deformation gradually intensifying in the vertical direction. The upper right section experienced a smaller compression deformation. The lower left section went through compression-extension-compression deformation. Lastly, the compression process of the lower right section gradually intensified. The upper part experienced the most severe deformation, followed by the middle section, and the lower section had the least severe deformation. The bottom right section exhibited the most serious distortion, while the lower left section showed the second most serious deformation. The upper left section displayed a smaller degree of deformation, and finally, the upper right section had the smallest amount of deformation.