Controlled experimental research and model design of double-layer high-frequency vibrating screen machine

2.1. Establishment of the mechanical model

Fig. 1 is a mechanical model of the plane motion double-layer high frequency vibrating screen system, which is composed of the upper screen body $m_1$, lower screen body $m_2$, and two eccentric rotors $m_{}$.

Fig. 1Mechanical model of double-layer high frequency vibrating screen

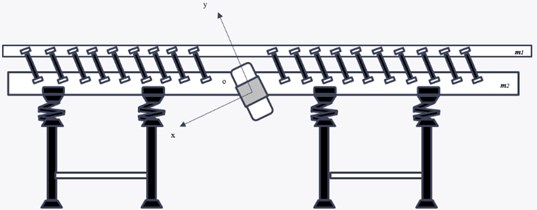
Fig. 2Schematic diagram of eccentric block rotation in the exciter (motor)

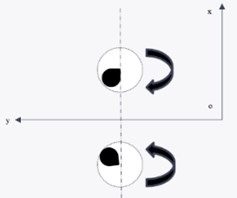
In Fig. 1, two motors of the same specification do synchronous reverse motion on the lower insulator. The structure of the eccentric block inside the motor is shown in Fig. 2. To ensure the normal operation of the vibrating screen machine, the eccentricity and mass of the eccentric block in the motor are equal, respectively. The coordinate system shown in the figure is established, and the $Y$-$Y$ axis is the axis of symmetry passing through the mass center of the vibrating screen. According to the force analysis, the instantaneous centrifugal inertia force generated by the rotation of the eccentric block is always in the opposite direction along the $x$-$x$ direction, offsetting each other. In the direction of the $y$-$y$ axis, the forces are superposed with each other, forming a single direction excitation force.

Under the motion of the eccentric block of the motor, the magnitude of the exciting force changes periodically, which realizes the reciprocating linear motion of the vibrating screen. When the vibrating screen operates at any time $t$, the calculation formula of its exciting force value is as follows:

where: $P_y$ – exciting force in the $Y$ direction; $P$ – maximum exciting force; $m$ – the sum of eccentric Block Mass on Vibrator; $r$ – rotation radius of eccentric block; $\omega$ – rotation angular velocity of exciter.

The amplitude and frequency of the motor’s exciting force are the vibration sources that make the double-layer or even three-layer vibrating screen produce forced vibration. According to the transmission speed and screening quality requirements of the tobacco leaf, the motor speed can be adjusted and the horizontal movement speed of the vibrating screen can be controlled. Can stimulate the vibration of the vibrating machine with a larger amplitude according to the design requirements.

2.2. Analysis of motion characteristics

2.2.2. Analysis of the influence law of the rigidity and quality of the double-layer screen body

If the system is composed of $n$ particles, let the mass of the $i$th particle $m_i$, the radius $r_i$, the active force $F_i$ acting on the particle, the restraining reaction $F_{Ni}$, and the inertial force $F_{gi}$, which can be obtained by using the principle of virtual work:

5

${\sum }_{i=1}^n(F_i+F_{Ni}+F_{gi})\cdot \delta r_i=0.$

Then the general equation of dynamics under ideal constraint is: ${\sum }_{i=1}^n(F_i+F_{gi})\cdot \delta r_i=0$ Among them, the virtual work of the active force is: ${\sum }_{i=1}^n{F}_i\cdot \delta r_i={\sum }_{i=1}^N{Q}_i\delta q_i$, where: $Q_i$ –generalized force, $\delta q_i$ – generalized hypocrisy.

The virtual work of inertia force is:

6

${\sum }_{i=1}^n{F}_{gi}\cdot \delta r_i=-{\sum }_{i=1}^N\left[\frac{d}{dt}\left(\frac{\partial T}{\partial \dot{q}}\right)-\frac{\partial T}{\partial q_i}\right]\delta q_i,$

where: $T$ – kinetic energy of the system. By substituting the virtual work of active force and inertia force into Eq. (5), the Lagrange equation of non-conservative system can be obtained as follows:

7

$\frac{d}{dt}\left(\frac{\partial T}{\partial {\dot{q}}_k}\right)-\frac{\partial T}{\partial q_k}+\frac{\partial U}{\partial q_k}=Q_k,k=\mathrm{1,2},\dots ,N.$

The motion of the vibrating screen can be regarded as a steady forced vibration composed of mass elastic-element-damping, in which mass refers to the weight of the vibrating screen equipment, elastic element refers to the damping spring device between the vibrating screen and the main building of the equipment, and damping includes spring damping and air damping. According to the theory of mechanical vibration, this kind of dynamic system can be regarded as forced vibration in an independent coordinate system.

The establishment of the differential equation of motion of the dual-mass high-frequency vibrating screen.

Fig. 3The simplified model of the dual-mass high-frequency vibrating screen

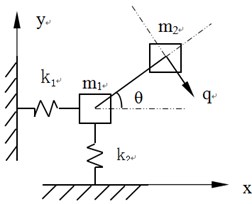
In this paper, the above-mentioned dual-mass high-frequency vibrating screen is regarded as a vibration system with three degrees of freedom, and its model is established as shown in Fig. 3:

$\gamma =\frac{Ml}{EI},\gamma =\frac{q}{l},M=\frac{qEI}{l^2},U=\frac{M^{2}l}{2EI}.$

Take $M$ into $U$ to get:

$U=\frac{M^{2}l}{2EI}=\frac{q^2{E}^2{I}^{2}l}{2l^{4}EI}=\frac{q^{2}EI}{2l^3},T=\frac{1}{2}{m}_1{\dot{x}}^2+\frac{1}{2}{m}_1{\dot{y}}^2+\frac{1}{2}{m}_2{\dot{q}}^2,$
$Q_x=2m_{0}r{\omega }^2\mathrm{s}\mathrm{i}\mathrm{n}\omega t\mathrm{s}\mathrm{i}\mathrm{n}{\beta }_0,Q_y=2m_{0}r{\omega }^2\mathrm{s}\mathrm{i}\mathrm{n}\omega t\mathrm{c}\mathrm{o}\mathrm{s}{\beta }_0,Q_q=0,$

where: $m_0$ – the mass of the eccentric block of the motor; $r$ – rotation radius of the eccentric block; $\omega$ – excitation frequency; ${\beta }_0$ – angle between motor and horizontal plane:

8

$\begin{array}{l}V=\frac{1}{2}{k}_1{x}^2+\frac{1}{2}{k}_2{y}^2+\frac{1}{2}\cdot \frac{EI}{l^3}\cdot {\left(q+y\mathrm{c}\mathrm{o}\mathrm{s}\theta -x\mathrm{s}\mathrm{i}\mathrm{n}\theta \right)}^2\\ =\frac{1}{2}{k}_1{x}^2+\frac{1}{2}{k}_2{y}^2+\frac{1}{2}\cdot \frac{EI}{l^3}\cdot (q^2+2qy\mathrm{c}\mathrm{o}\mathrm{s}\theta -2qx\mathrm{s}\mathrm{i}\mathrm{n}\theta +qy\mathrm{c}\mathrm{o}\mathrm{s}\theta +y^2\mathrm{c}\mathrm{o}{\mathrm{s}}^2\theta \\ -2xy\mathrm{s}\mathrm{i}\mathrm{n}\theta \mathrm{c}\mathrm{o}\mathrm{s}\theta -qx\mathrm{s}\mathrm{i}\mathrm{n}\theta -xy\mathrm{s}\mathrm{i}\mathrm{n}\theta \mathrm{c}\mathrm{o}\mathrm{s}\theta +x^2\mathrm{s}\mathrm{i}{\mathrm{n}}^2\theta )\\ =\frac{1}{2}{k}_1{x}^2+\frac{1}{2}{k}_2{y}^2+\frac{1}{2}{k}_3{q}^2+k_{3}qy\mathrm{c}\mathrm{o}\mathrm{s}\theta -k_{3}qx\mathrm{s}\mathrm{i}\mathrm{n}\theta +\frac{1}{2}{k}_3{y}^2\mathrm{c}\mathrm{o}{\mathrm{s}}^2\theta \\ -k_{3}xy\mathrm{s}\mathrm{i}\mathrm{n}\theta \mathrm{c}\mathrm{o}\mathrm{s}\theta +\frac{1}{2}{k}_3{x}^2\mathrm{s}\mathrm{i}{\mathrm{n}}^2\theta .\end{array}$

By substituting $T$ and $V$ into Eq. (7), the differential equations of motion of the dual-mass high-frequency vibrating screen can be obtained:

9

$\left\{\begin{array}{l}{m}_1\ddot{x}+\left(k_1+k_3\mathrm{s}\mathrm{i}{\mathrm{n}}^2\theta \right)x-k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta \mathrm{c}\mathrm{o}\mathrm{s}\theta \cdot y-k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta \cdot q=2m_{0}r{\omega }^2\mathrm{c}\mathrm{o}\mathrm{s}\omega t\mathrm{s}\mathrm{i}\mathrm{n}{\beta }_0,\\ m_1\ddot{y}-k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta \mathrm{c}\mathrm{o}\mathrm{s}\theta \cdot x+\left(k_2+K_3\mathrm{c}\mathrm{o}{\mathrm{s}}^2\theta \right)y+k_3\mathrm{c}\mathrm{o}\mathrm{s}\theta \cdot q=2m_{0}r{\omega }^2\mathrm{c}\mathrm{o}\mathrm{s}\omega t\mathrm{c}\mathrm{o}\mathrm{s}\beta ,\\ m_2\ddot{q}-k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta \cdot x+k_3\mathrm{c}\mathrm{o}\mathrm{s}\theta \cdot y+k_{3}q=0.\end{array}\right.$

The specific characteristic equation can be obtained as follows:

10

$\left|\begin{array}{ccc}{k}_1+k_3\mathrm{s}\mathrm{i}{\mathrm{n}}^2\theta -m_1{\omega }^2& -k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta \mathrm{c}\mathrm{o}\mathrm{s}\theta & -k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta \\ -k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta \mathrm{c}\mathrm{o}\mathrm{s}\theta & k_2+k_3\mathrm{c}\mathrm{o}{\mathrm{s}}^2\theta -m_1{\omega }^2& k_3\mathrm{c}\mathrm{o}\mathrm{s}\theta \\ -k_3\mathrm{s}\mathrm{i}\mathrm{n}\theta & k_3\mathrm{c}\mathrm{o}\mathrm{s}\theta & k_3-m_2{\omega }^2\end{array}\right|=0.$

Among them, $k_1=$ 320000 N/m, $k_2=$ 1480000 N/m, $\theta =$55°, $m_1=$ 870 kg, $m_2=$ 300.

According to the test results, the rigidity of the spring pieces can be obtained $k_3=$ 34000×38 = 1292000 N/m.

By substituting the data, the stiffness matrix and mass matrix can be obtained as:

$K=1.0e+006*\left[\begin{array}{ccc}0.4067& -0.0607& -0.1058\\ -0.0607& 1.5225& 0.0741\\ -0.1058& 0.0741& 0.1292\end{array}\right],M=\left[\begin{array}{lll}870& & \\ & 870& \\ & & 300\end{array}\right].$

Matlab is used to solve the problem, and the first to third order natural frequencies are obtained as shown in Table 1.

Table 1Natural frequency of the dual-mass high-frequency vibrating screen

${\omega }_1$	${\omega }_2$	${\omega }_3$
17.1214	38.9880	75.7656

The excitation frequency of the vibrating screen is 78.54 rad/s. From Table 1, it can be seen that the third-order natural frequency of the vibrating screen is lower than the excitation frequency, so the resonance of the high-frequency vibrating screen will not occur under the normal working frequency. However, in the process of starting and braking, the motor will pass through the resonance area of the vibrating screen, which makes the screen body shake greatly in the process of starting and braking. Therefore, we must carry out transient analysis of the screen body starting the process and study the stress changes of each part of the screen body in the starting process.

2.2.3. Change curve of natural frequency of vibrating screen with various physical parameters

As shown in Fig. 4, it is a regular curve of the natural frequency of each order of the high-frequency vibrating screen changing with the stiffness $k_3$, that is, a regular curve of the natural frequency of each order with the number of upper-layer spring pieces. It can be seen from the figure that when $k_3$ increased from 1.02×e6 N/m to 2.04×e6 N/m, the number of spring pieces increased from 30 groups to 60 groups (each group has two spring pieces), the first and second natural frequencies of the high-frequency vibrating screen change little with K3, while the third natural frequency increases from 69.8 rad/s to 97.85 rad/s with K3. When the stiffness K3 reaches 1.31×e6 N/m, the third-order natural frequency reaches 78.33 rad/s, that is, changing the number of upper spring pieces has little effect on the low-order frequency of the vibrating screen system. Therefore, the amplitude and horizontal movement speed of the upper sieve can be controlled by changing the number of spring pieces by reasonable design, and the screening efficiency can be improved according to the tobacco feeding situation. when the number of spring piece groups is equal to 40 groups, resonance will occur at this time so that the mechanical equipment can work normally.

Fig. 4Relation curve between natural frequency and rigidity K3 of vibrating screen

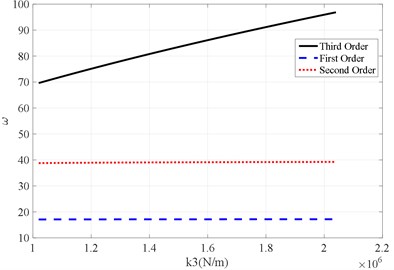
Fig. 5Relation curve between the natural frequency of vibrating screen and number of elastic supports

As shown in Fig. 5, when the stiffness $k_3$ is unchanged, the stiffness $k_1$ and $k_2$ are changed, that is, the number of elastic supports of ROSTA. It can be seen that the number of ROSTA elastic supports has little effect on the third-order natural frequency and the natural frequency increases as the number of elastic supports increases. When the number of elastic supports reaches 10, the screen body will resonate. The change of the number of lower spring pieces will affect the low-order natural frequency of the system. Considering the influence of the quality of the lower screen body, a reasonable design can effectively reduce the large lateral vibration of the vibrating screen when the machine is switched on and off.

As shown in Fig. 6, when the stiffness does not change, changing the magnitude of the vibration mass m1, that is, changing the mass of the intermediate frame, the range of the third-order natural frequency changes little, but it decreases as the mass of the intermediate frame increases. When $m_1=$ 816 kg, the screen body generates resonance. From calculation and analysis, the mass of the lower sieve body is reasonable between 600 and 1000 kg. The change of the lower sieve body mass has little effect on the vibration characteristics of the system, but if its mass is too small, it will cause a larger side directional vibration of the vibrating machine which will affect system stability.

Fig. 6Relation curve between the natural frequency of the vibrating screen and the vibration mass m1

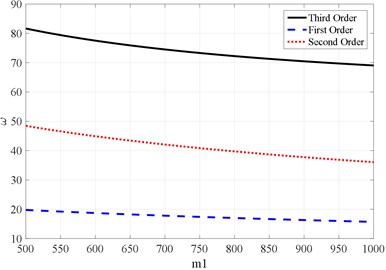
As shown in Fig. 7, when the stiffness K1, K2, K3, and the vibration mass $m_1$ are unchanged, changing the magnitude of the vibration mass $m_2$, that is, changing the mass of the upper screen body component, has a greater impact on the third-order natural frequency. The third-order natural frequency decreases with the increases in the mass of the upper screen body. When the vibration mass of the upper screen body is about 293.4 kg, resonance will occur. By changing the size of $m_2$, the occurrence of the third-order natural vibration of the system can be controlled, resulting in higher frequency and larger amplitude vibration.

Fig. 7Relation curve between the natural frequency of the vibrating screen and the vibration mass m2

3.1. Measurement of motion characteristics

The DASP instrument was used to measure a type of high-frequency double-mass vibrating screen in the laboratory. Select two points on the lower sieve body, named point 1 and point 2; select two points on the upper sieve body, named point 5 and point 6. The arrangement of measuring points is shown in Fig. 9. The time-domain waveform analysis diagram of these four points measured by the DASP instrument is shown in Fig. 9. Where Fig. 9(a) shows the motion waveform of four points in the horizontal direction and Fig. 9(b) represents the motion waveform of four points in the vertical direction.

Fig. 9Horizontal and vertical measuring point layout of double-layer vibrating screen

a) Sensor level measuring point

b) Sensor vertical measuring point

From the waveform diagram, it can be concluded that the amplitude of the points above the lower sieve body of the dual-mass high-frequency vibrating screen is much smaller than the point amplitude of the upper sieve body in the horizontal and vertical directions.

According to the actual situation, the mass of the upper sieve of the dual-mass high-frequency vibrating screen we measured is 200 Kg; the mass of the lower sieve is 976 Kg (including the motor), and the motor that provides the exciting force for the entire vibrating screen is located in the lower sieve, which makes the mass of the lower sieve larger than the mass of the upper sieve. According to the momentum conservation and the derived Eq. (4) obtained in the first part, it is found that the momentum conservation relationship is satisfied when the measured waveform amplitude and the vibration quality of the upper and lower tanks are brought into the momentum conservation Eq. (4) for calculation, which verifies the correctness of the theoretical research in the first part.

Fig. 10Time-domain waveform comparison of sieve measurement points above and below

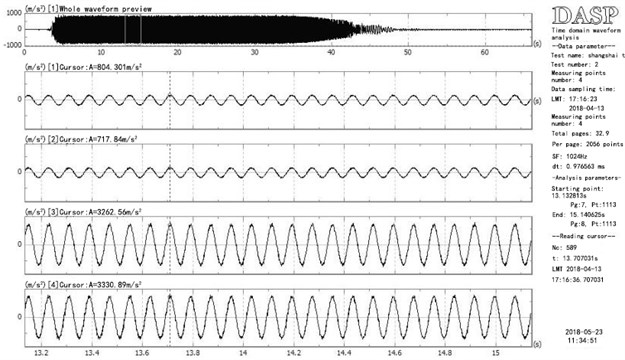
a) Horizontal amplitude of measuring points

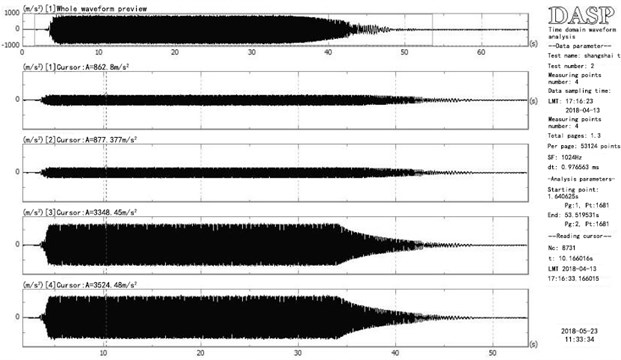
b) Vertical amplitude of measuring points

The waveform diagram of Fig. 10 is sorted out and summarized to obtain a correspondence table between test points and amplitudes, as shown in Table 2.

According to the table of horizontal and vertical amplitudes of the working state, it can be concluded that the amplitudes of the points on the upper and lower sieve bodies have different positive and negative amplitudes at the same time, which means that the motion is reversed, which probably meets the law of conservation of momentum.

The four corners of the lower sieve body are selected as test points, and the displacement waveform in the horizontal direction is measured, as shown in Fig. 11.

Fig. 11Time-domain waveform diagram of the corner points of the lower sieve

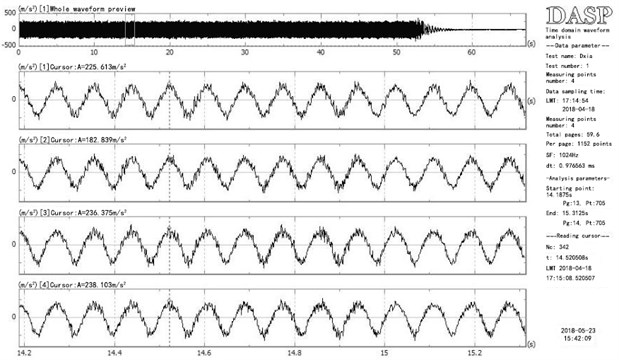
From Fig. 11, we can see that the motion of the sieve body is periodic, and the vibration amplitudes of the four corner points are relatively similar, and the vibration periods are consistent, indicating that the relative vibration of the upper and lower grooves can be simplified into their respective rigid body vibration.

3.2. Effect of spring plate number on motion characteristics

The different number of spring leaves influences the motion characteristics of the screen body of the vibrating screen. Therefore, it is an important research direction of this paper to make the motion of the screen body controllable by changing the number of spring leaves.

To ensure the safe and stable operation of the vibrating screen, we change the number of spring leaf groups used by the vibrating screen and use the DASP to measure the motion waveform of the vibrating screen under different spring leaf groups to obtain the required research data.

To ensure the normal and safe operation of the vibrating screen, we have set 20 sets of spring pieces, 19 sets of spring pieces, and 18 sets of spring pieces, and measured the movement of the points on the upper and sieve bodies in three cases, and obtained the corresponding relationship. The four test points above the lower sieve body are named point 1, point 2, point 3, and point 4; the four points above the upper sieve body are point 5, point 6, point 7, and point 8. The movement of these 8 points in horizontal and vertical directions was measured by the DASP instrument. Take the motion waveform diagram of each point under the normal operation of 20 groups of springs as an example, as shown in Fig. 12.

Fig. 12(a) shows the movement of four measuring points on the lower sieve body under normal operation of 20 sets of spring pieces, and Fig. 12(b) shows the movement of four measuring points on the upper sieve body. After the motion stabilized, the amplitude of the 8 points in the horizontal direction was tested. In the same way, the horizontal motion of 8 points in the two cases of 19 sets of spring pieces and 18 sets of spring pieces is obtained, and the 1, 2, 3, and 4 points are located in the lower trough; the 5, 6, 7, and 8 points are located upper trough. Table 3 lists the comparison of eight points of motion of the vibrating screen in normal operation under three different numbers of spring sheets, which are expressed by amplitude.

Fig. 12Comparison of vibration of testing points of the vibrating screen machine with different number of spring pieces

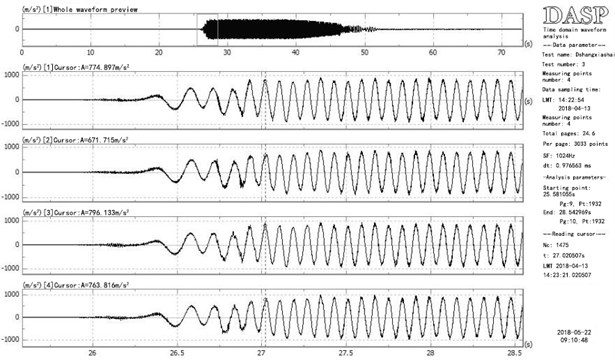
a)

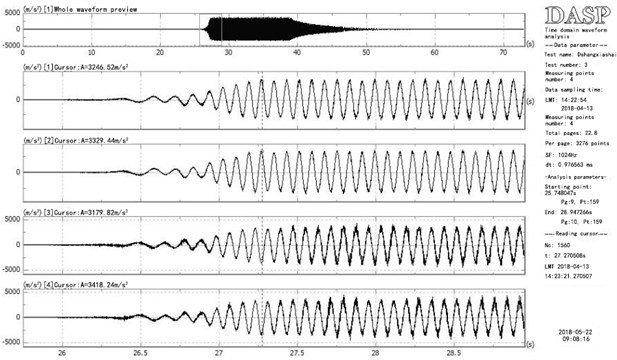
b)

From Table 3, it can be concluded that for the points on the lower sieve body, the number of spring sheets has little effect on the points above. Considering the unavoidable errors in the actual operation, the amplitude of 19 groups of spring sheets is larger than that of 18 groups of spring sheets; the amplitude of three groups of spring sheets will fluctuate due to the specific position of the test point. As the number of upper spring pieces decreases, its rigidity also decreases. The amplitude of the upper screen body of the 18 groups of spring pieces is higher than that of the other two groups, and the third-order natural frequency of the system decreases, which is closer to the operating frequency of the motor 12.5 Hz, so the amplitude is larger.

3.3. Rigid body modal test and parameter analysis of double-layer vibrating screen machine

Fig. 13 shows the frequency response curves of the sensors located at the upper and lower troughs. The sensors are arranged horizontally and the hammer is excited horizontally. The horizontal vibration frequencies of the upper and lower vibrating screens are shown in Table 4 below. The measured natural frequency values are consistent. The 20, 19, and 18 sets of spring sheets are tested in the same way. The natural frequencies are shown in the Table 4.

Fig. 13Comparison of vibration at test points of the vibrating screen with different numbers of spring blades

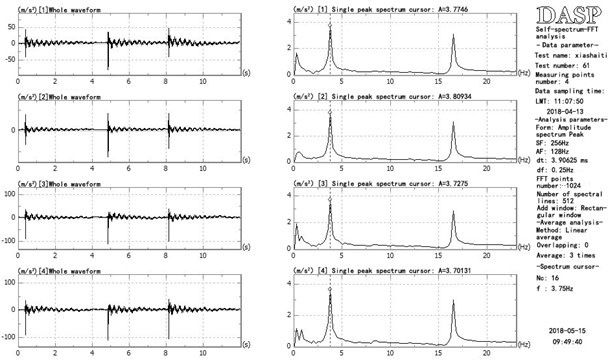
a) Lower trough

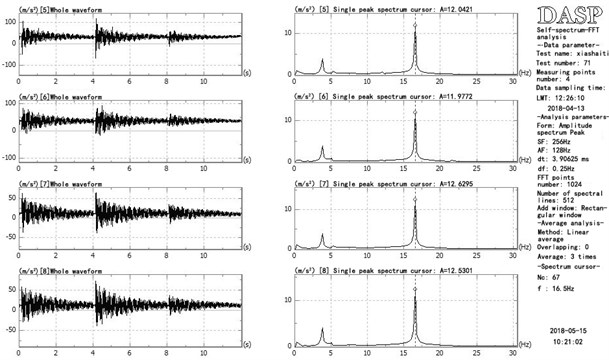
b) Upper trough

The vibration amplitude along the vertical direction of the spring plate corresponding to the position of the connecting upper and lower grooves is studied. As shown in Fig. 14, the position of the upper groove is 38.39 m/s², and the acceleration of the position of the lower groove is 6.96 m/s², which is a 5.5:1 relationship. The mass ratio of the upper and lower grooves is about 1:5, so the vertical direction along the spring plate also basically meets the law of momentum conservation.

For the lower sieve body, it can be seen from the measured data that the change of the number of spring plate groups has a greater impact on the motion amplitude of the upper sieve body, and with the increase of the number of spring plate groups, the amplitude of the measuring points on the upper sieve body as a whole has a decreasing trend. With the increase in the number of spring plates and the increase of the overall stiffness, the vibration amplitude will decrease. Increasing or decreasing the number of spring plate has a slight effect on the vibration frequency of the upper and lower tanks.

Fig. 14Comparison of vibration of test points at the upper and lower grooves in the direction of the vertical spring leaf

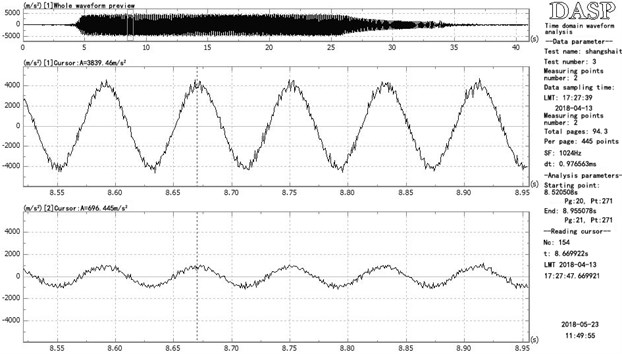
a) Amplitude graph

b) Measuring point position

3.4. Vibrating screen modal test

12 piezoelectric acceleration sensors and a force hammer were used to attain the rigid body modal of the double-layer vibrating screen by the MIMO hammering method, as shown in Fig. 15.

The frequency of the motor in normal operation is 12.5 Hz. The modal testing frequency of the elastomer in the lower groove of the motor is shown in Table 5.

Because the upper groove body will produce a strong wind whistle when the machine is running, it is necessary to carry out an in-plane modal analysis. The results show that the local relative stiffness of the design is small, warping, and twisting vibration modes will occur. The modal analysis is shown in Fig. 16, The overall stiffness of the upper groove body needs to be increased or strengthened locally. The practice has proven that the follow-up has played an optimization and improvement effect on the design of the vibrating screen in Hangzhou Tobacco Factory. In addition to the relative reverse motion during operation, the upper and lower grooves are shown in Fig. 17(a), and there is relative torsional vibration outside the plane, as shown in Fig. 17(b), but the amplitude is small, and its influence should be considered in the structural design and selection of the spring sheets.

Fig. 15Modal test of the vibrating screen

Fig. 16Modal of upper groove of the vibrating screen

a) Distortion

b) Warpage

Fig. 17Rigid body mode of the upper and lower trough of vibrating screen

a) Horizontal rubbing

b) Relative torsion

The picture below shows a vibrating screen machine placed on the second floor of an industrial plant in Hangzhou. It is required to minimize the vibration of the floor when the machine is working. Therefore, we control the mass ratio of $m_1$ and $m_2$, reasonable choosing elastic support K1 and adjusting the number of spring pieces to control K2 according to the law of influence of the above parameters on the vibrating screen system to make the machine can operate normally at 12.33 Hz while greatly reducing the vibration to the floor.

Fig. 18Test site pictures

Fig. 19The vertical displacement and velocity curve of the upper tank (normal work maximum displacement 360 um, maximum speed 26.8 mm/s; switch machine maximum displacement 754 um, maximum speed 30.2 mm/s)

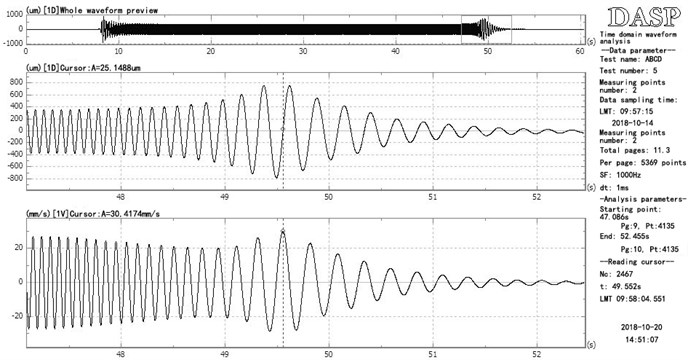
Fig. 20The vertical displacement and speed curve of the lower support (normal working maximum displacement 2 um, maximum speed 0.162 mm/s; switch machine maximum displacement 2.35 um, maximum speed 0.12 mm/s gradually decrease)

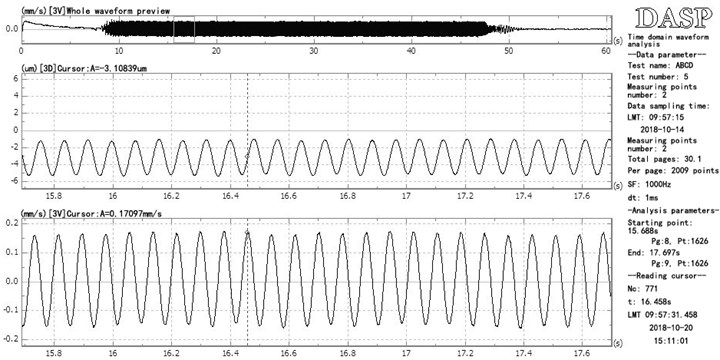
When the double-layer vibrating screen is working normally, by comparing the maximum displacement and velocity amplitude between the upper and lower screen bodies of the vibrating screen and the floor, it can be found that the design requirements are well met.

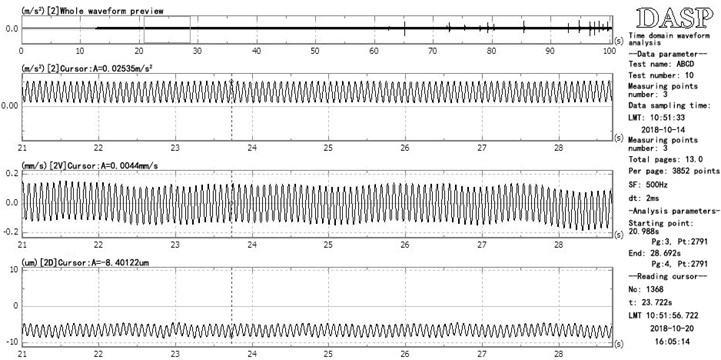
Fig. 21The machine is running normally, the acceleration, velocity, and displacement curve of the ground position (normal work, the maximum acceleration is 0.0119 mm/s, the maximum speed is 0.162 mm/s, the maximum displacement is 2.2 um)