Multiple root resonance of tandem mill

2.1. Definition of multiple-root resonance

According to the vibration theory [11], when the excitation frequency is included by the resonance region of the system, the resonance will occur. When there are two close natural frequencies ${\omega }_1$ and ${\omega }_2$ in a system, the resonance region of each natural frequency is described by the neighbourhood as follows:

where ${\omega }_i$ is the natural frequency of the system, and ${\delta }_i$ is the neighbourhood radius of the natural frequency ${\omega }_i$, which is positive. When the excitation frequency $\omega$ is included by the resonance region $U\left({\omega }_i,{\delta }_i\right)$ of a natural frequency of the system, the resonance will occur.

It is assumed that the two adjacent natural frequencies are ${\omega }_1$ and ${\omega }_2$ and ${\omega }_1<{\omega }_2$. The neighbourhood of the two adjacent frequencies is shown in Eq. (1). Then, we have the intersection of these regions:

where $U\left(\stackrel{-}{\delta }\right)$ is the intersection of two natural frequency resonance regions. If the excitation frequency $\omega \in U\left(\stackrel{-}{\delta }\right)$, the two resonance frequencies will be excited, and two natural modes will simultaneously occur. If two resonance frequencies are similar or equal, the corresponding natural modes are identical. In the case of $\omega \in U\left(\stackrel{-}{\delta }\right)$, it is known from the principle of linear superposition that the system will vibrate more violently than the resonance with a single natural mode. The vibration, where two or more natural modes are excited by the same source, is called as a “multiple-root resonance”, and the neighborhood intersection $U\left(\stackrel{-}{\delta }\right)$ is called as the “multiple-root region”.

If ${\omega }_1\ne {\omega }_2$, but their values are similar, they are called as a pair of “sub-multiple roots”, and $U\left(\stackrel{-}{\delta }\right)$ is called as the “sub-multiple-root region”. If ${\omega }_1={\omega }_2$, the characteristic equation has multiple roots in the analytic solution, which are called as the “complete-multiple-root” here, and $U\left(\stackrel{-}{\delta }\right)$ is called as the “complete-multiple-root region”. This paper only studies the sub-multiple-root.

The definition of the “multiple-root region” of multiple frequencies is similar.

2.2. Vibration mode of “multiple-root resonance”

This section proves that when the two natural frequencies are similar enough by their value, their vibration modes are consistent. The matrix in the dynamic equation for the multi-DOF vibration system is as follows [11]:

where $\mathbf{K}$ is the stiffness matrix; $\mathbf{M}$ is the mass matrix; $\mathbf{u}$ is the eigenvector. When $\mathbf{F}=\mathbf{K}-{\omega }^2\mathbf{M}$ is substituted, Eq. (3) is transformed to:

where $u_i$ ($i=$1, 2, 3, 4) is the element of $\mathbf{u}$; $f_{ij}\left(\omega \right)$ is the element of matrix $\mathbf{F}$, and:

where $k_{ij}$, and $m_{ij}$ are the corresponding elements of matrices $\mathbf{K}$ and $\mathbf{M}$; Eq. (4) has a nonzero solution, and matrix $\mathbf{F}$ is not a full-rank matrix. According to the elementary transformation of matrix [12], matrix $\mathbf{F}$ can be transformed into a trapezoidal matrix $\stackrel{-}{\mathbf{F}}$:

where ${\stackrel{-}{f}}_{ij}\left(\omega \right)$ is the element of the transformed matrix $\stackrel{-}{\mathbf{F}}$. For the convenience of discussion, it is assumed that the system does not have an analytic multiple root; so that only the $n$th row of the trapezoidal matrix $\stackrel{-}{\mathbf{F}}$ is zero, and the first rows of Eqs. (4) and (5) are equal. The second lines of $\stackrel{-}{\mathbf{F}}$ are:

where ${\stackrel{-}{ROW}}_i$ and ${ROW}_i$ are elements of each row of $\stackrel{-}{\mathbf{F}}$ and $\mathbf{F}$.

Every element, $f_{ij}\left(\omega \right)$, in the matrix $\mathbf{F}$ has a quadratic continuous function for $\omega$. From Eq. (6), we know that the operation of $\mathbf{F}$ is a simple operation of addition, subtraction, multiplication and division in the trapezoidal transformation process. Therefore, according to the theorem of continuous function [13], it is known that ${\stackrel{-}{f}}_{ij}\left(\omega \right)$ is a continuous function, so the following can be obtained:

The linear equations of Eq. (5) are:

According to the final equation of Eq. (8), the following can be obtained:

where $r_{n-1}$ is the vibration mode. With substitution $u_n=\text{1}$ and iteration, the corresponding mode ${\mathit{r}}^{\left(i\right)}$ of natural frequency ${\omega }_i$ is as follows:

Suppose that the system has two natural frequencies: ${\omega }_1$, ${\omega }_2$, and ${\omega }_2={\omega }_1+\sigma$, where $\sigma$ is infinitesimal. Their modes are:

With Eqs. (7) and (9), the following can be obtained:

Similarly, it can be proven that $r_i^{\left(1\right)}=r_i^{\left(2\right)}$. Therefore, when the natural frequencies, ${\omega }_1$ and ${\omega }_2$, are similar by their value (the difference between them is tiny enough), the following can be obtained:

Thus, when the system has two natural frequencies with very similar values, the mode shapes are identical. And if two natural frequencies are induced by an excitation frequency, the multiple-root resonance occurs, and the superposition of the identical mode shapes amplifies the vibration.

2.3. Dynamics study of multiple-root vibration

In this section, the “device-foundation” dynamic model is introduced to study the multiple-root vibration. This model is shown in Fig. 1.

Fig. 1Dynamic model of “device-foundation” system

It is assumed that the foundation does not swing. For Fig. 1, the dynamic equations are as follows:

The characteristic equation of Eq. (14) is:

The changing mass and stiffness have a great significance in discussing the “multiple-root”.

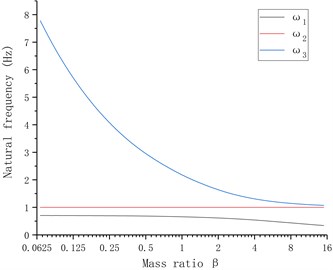
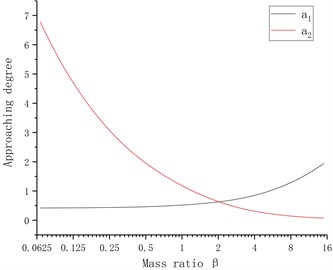
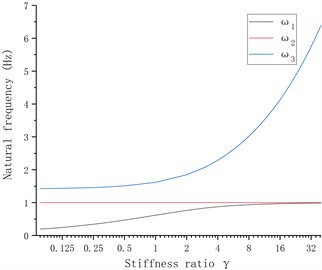
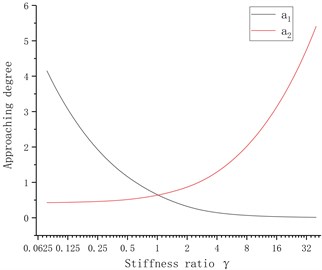
Thus the mass ratio $\beta$ is considered as a variable, and $m_1=m_3=$ 1 kg, $k_1=k_2=k_3=$ 1 N/m, $m_2=\beta m_1$ is substituted, the multiple-root natural frequency of the equipment when the mass ratio changes is discussed; and then the stiffness ratio $\gamma$ is considered as a variable, and substituted $m_1=m_3=$ 1 kg, $k_1=k_3=$ 1 N/m, $m_2=2m_1$, $k_2=\gamma k_1$, then the “multiple-root” of the system is discussed when the stiffness varies. The relationships among the mass, stiffness and natural frequency are shown in Fig. 2, where $a_1$ is the approaching degree between ${\omega }_1$ and ${\omega }_2$; $a_2$ is the approaching degree between ${\omega }_2$ and ${\omega }_3$, and $a_i=({\omega }_{max}-{\omega }_{min})/{\omega }_{min}$ is defined. Fig. 2 shows that when the mass ratio $\beta$ increases, the 2nd-order natural frequency and 3rd-order natural frequency continue approaching, and when $\beta >\text{11}$, the approaching factor $a_2<\text{0.1}$, when the stiffness ratio $\gamma$ increases, the 1st-order natural frequency ${\omega }_1$ and 2nd-order natural frequency ${\omega }_2$ are gradually approaching one another, and when $\gamma =\text{40}$, the approaching factor $a_1=$ 0.013, and will continue to decrease with the increase in stiffness ratio.

Fig. 2Relationship among mass ratio, stiffness ratio and root natural frequency

3.1. Dynamics analysis of tandem mill

According to Fig. 3, a simplified diagram of the tandem mill is shown in Fig. 4, where $x_i$ ($i=\mathrm{}$1, 2, 3, 4, 5) is the DOF of each mill, and $x_6$ is the DOF of the foundation; $q_i$ ($i=\mathrm{}$1, 2, 3, 4, 5) is the DOF of each connect region, and $q_3=x_6$; $m_i$ ($i=\mathrm{}$1, 2, 3, 4, 5) is the quality of each stand; $m_6$ is the quality of the foundation; $I$ is the moment of inertia of the foundation; $k_1$ is the connection stiffness of each mill and foundation; $k_2$ is the stiffness of the foundation and earth; a micro-pendulum motion around the center of the foundation is considered in the system, and $\theta$ is the pendulum angle; $F$ is the harmonic excitation, and $F=F_0\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)$, $F_0$ is the amplitude; $a$ is the distance between two stands. There are seven DOFs in the system, and vector $\mathbf{x}$ is defined as the DOFs of the system:

The dynamics equations of Fig. 4 are:

The mass matrix $\mathbf{M}$ is:

The stiffness matrix $\mathbf{K}$ is:

The characteristic equation is:

From the literature [14] and field measurement, we have:$k_1=$ 4.152×10⁹ N/m, $k_2=$ 2.36×10⁷ N/m. The inertia of the foundation is:

where $h$ is the height of the foundation, and $a$ is the length between two stands. From the design, it is possible to obtain $h=$9.6 m, $a=$5.5 m. Because the quality of each stand of the tandem mill approaches 444000 kg according to the field data, it is supposed $m_1=m_2=m_3=m_4=m_5=$ 444000 kg. The mass of the foundation is about 20 times that of the rolling mill according to the field data, thus, it is supposed as $m_6=$8880000 kg.

Fig. 4Simplified diagram of tandem mill

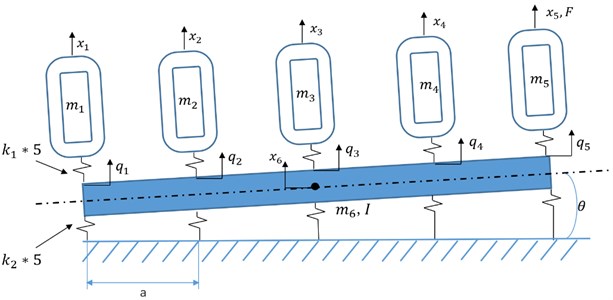
3.2. Multiple-root resonance of tandem mill

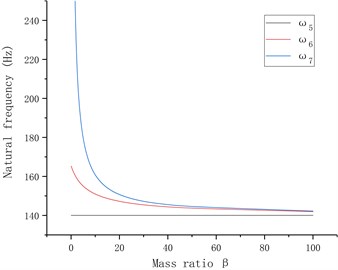
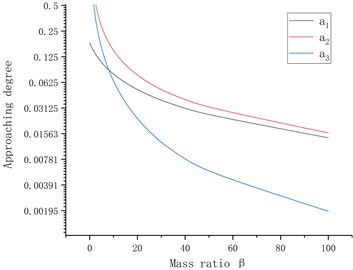
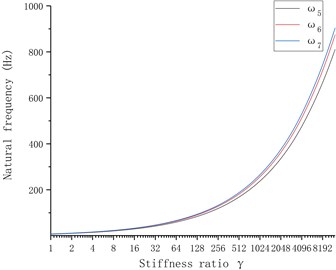
When the mass of the foundation, $m_6$, is considered as a variable, $m_6=\beta m_1$ ($\beta$ is the mass ratio) is defined and $k_1$, $k_2$, $m_1$, $m_2$, $m_3$, $m_4$, $m_5$ and $m_6$ are substituted to Eq. (20); when the stiffness is considered as a variable, $k_1=\gamma k_2$ ($\gamma$ is the stiffness ratio) is defined, and the above parameters are substituted to Eq. (20). The relationship among $\beta$, $\gamma$ and the natural frequencies of the system are obtained by numerical calculation as shown in Fig. 5, where $a_1$ is the approaching degree between ${\omega }_5$ and ${\omega }_6$; $a_2$ is the approaching degree between ${\omega }_5$ and ${\omega }_7$; $a_3$ is the approaching degree between ${\omega }_6$ and ${\omega }_7$, and the equation is defined as follows: $a_i=({\omega }_{max}-{\omega }_{min})/{\omega }_{min}$. Fig. 5 shows that when $\beta$ is considered as a variable, the 6th- and 7th-order frequencies of the mill gradually approach and converge to the 5th-order frequency, and the approaching degree of the 6th and 7th-order frequencies, $a_3$, is minimal; when $\gamma$ is considered as a variable, the proximity factor has little variation, thus the mass ratio $\beta$ is only considered.

The foundation mass of the tandem mill in one steel factory is 20 times of that of a single mill ($\beta =\text{20}$). Then, the 6th- and 7th-order natural frequencies are 147 Hz and 150 Hz, and the proximity factor between them is 2.4 %. It is easy to form the multiple-root region and initiate the multiple-root resonance.

Fig. 5Relationship among natural frequency, mass ratio and stiffness ratio of rolling mill

3.3. Modal analysis of multiple-root resonance

The modal analysis of the tandem mill is performed with ANSYS workbench. The results are shown in Fig. 6.

Fig. 6 shows that the tandem mill has two natural frequencies with similar value: 142.93 Hz and 144.33 Hz, and the corresponding vibration modes are different but have large vertical components: the maximum vibration amplitudes occur on F5 and F4, respectively. Therefore, if the tandem mill is excited with a frequency approach to 142 Hz and 144 Hz, two natural modes will be simultaneously excited and initiate the multiple-root resonance.

3.4. Field test of multiple-root resonance of tandem mill

The beat vibration is the superposition of two or more signals with similar frequencies and amplitudes, and these signals are characterized with strong-weak cycles, therefore, the spectrum of the beat vibration shows two or more dominant frequencies approach each other. Many studies show that, one of these dominant frequencies in the beat vibration is the excitation frequency. However, in the study of tandem mill vibration, it is found that the two dominant frequencies approach each other due to that they are both natural frequencies, and make a pair of “sub-multiple-root”. To verify that the “sub-multiple-root” exists in the tandem mill, the mill vibration was tested before and after the foundation modification which reduced the mass of the whole foundation and turned the mass ratio of the foundation and the single mill from 20 to 17.5. Magnetoelectric vibration velocity transducers and data collector were used in the field test, and the parameters of the sensor and collector are shown in Table 1 and Table 2, respectively.

Fig. 7 shows the test position in field.

Fig. 6Mode of continuous rolling mill

Fig. 7Field test site and testing instruments

The vibrations of F4 and F5 before and after the reconstruction of foundation are shown in Fig. 8 and Fig. 9, respectively.

Fig. 8 shows that both F4 and F5 stands have an obvious double-beat vibration with the frequencies of 142 Hz and 144 Hz, which are almost identical to the two natural frequencies in the simulation in section 3.3. The excitation frequency is 138 Hz, which approaches two dominant frequencies of F5 stand. From Fig. 8, it can be seen that F5 stand has a larger vibration than F4.

Fig. 9 shows that after the foundation reconstruction, F4 and F5 stands have one dominant frequency of 132 Hz. The excitation frequency of 138 Hz remains in the spectrum of F5.

Fig. 8Vibration spectrum before foundation reconstruction

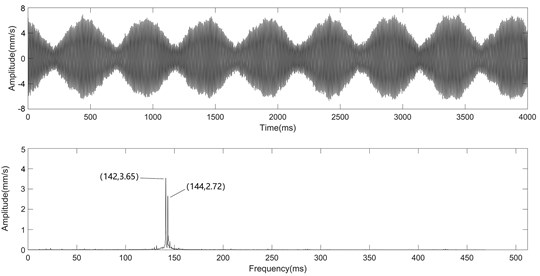
a) Vibration spectra of F4 stand

b) Vibration spectra of F5 stand

Fig. 9Vibration spectrum after foundation reconstruction

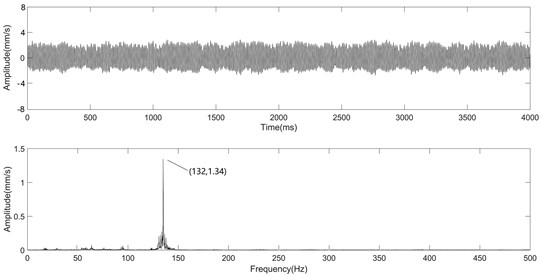
a) Vibration spectra of F4 stand

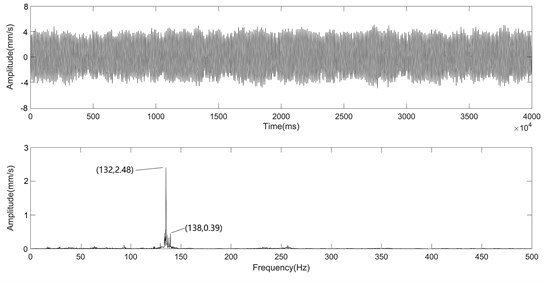
b) Vibration spectra of F5 stand

Comparing the time domain waveforms of Fig. 8 and Fig. 9, it can be seen that the multiple-root resonance of the mill is much more violent than that of the mill with the resonance as one dominant frequency. After the foundation reconstruction, the mass ratio is changed, and the gap between the two natural frequencies, which are a pair of “sub-multiple-root” before the reconstruction, widens. Then, only one natural mode is induced by the excitation, thus, the vibration is reduced.