Dynamics modeling and experimental modal analysis of bolt loosening for lightning rod

2.1. Model of bolts

The material of the fastening bolt in FBSU is carbon steel, type Q235, elastic modulus is 200 GPa, Poisson ratio is 0.3, density is 7850 kg/m³, and the finite element model of bolts is built by ANSYS software. In order to get close to the actual condition and obtain higher precision, the paper adopted the solid bolt model to establish the contact unit on the horizontal contact surface of the upper and lower flanges, the bolt head and the contact surface of the nut and the flange, which can transmit the tensile, bending and thermal loads. Establishing a threaded model can be very troublesome for pre-processing and solving. Therefore, when modeling a bolt finite element, the thread is simplified and the equivalent cylindrical area is taken. The bolt pre-loading method is directly added to the cylindrical surface of the bolt with the bolt pretension command.

2.2. Virtual material method

The virtual material method is equating the joint interface with a layer of virtual material, as shown in Fig. 1, but in fact this material does not exist, the mechanical properties of the joint interface is simulated by changing the density, Poisson ratio and elastic modulus of this layer of virtual material.

Fig. 1Equivalent layer of virtual material

The virtual material method can establish the dynamic model by the elastic modulus, density and Poisson ratio of the two connecting members, as well as the surface roughness and the bolt pre-tightening force. The elastic modulus of the virtual material layer can be calculated by the Eq. (1) [29]:

The Poisson ratio can be calculated by the Eq. (2):

The density can be calculated by the Eq. (3):

where $D$ represents the fractal dimension; $\psi$ represents the domain extension factor for microcontact size distribution only associated with $D$; $E^{\mathrm{\text{'}}}$ represents the equivalent elastic modulus; $G$ represents the fractal roughness parameter; $a_L$ represents the maximum contact area of the micro-protrusion; $a_C$ represents the critical contact area of the micro-protrusion; ${\mu }^{\mathrm{\text{'}}}$ represents the equivalent Poisson ratio; $E^{*}$ represents the dimensionless virtual material elastic modulus; $G_x^{\mathrm{*}}$ represents the dimensionless virtual material shear modulus; ${\rho }_1$, ${\rho }_2$ represents the density of the two connected materials; $l_1$, $l_2$ represents the thickness of two connect members. $D$ and $G$ are determined by $R_{a1}$ and $R_{a2}$ respectively. $R_{a1}$ and $R_{a2}$ are the surface roughness of surface 1 and 2.

2.3. Complete finite element model of FBSU

The ANSYS software is used to build the complete finite element model of FBSU. The solid model consists of four parts: upper and lower bodies, fastening bolts and virtual material layers. There are virtual material layers locate in joint interface between bodies and bolts, also in joint interface between upper and lower bodies. The material of the upper and lower bodies is Q235B, the elastic modulus is 200 GPa, the Poisson ratio is 0.3, and the density is 7850 kg/m³. The upper and lower bodies are locked by 24 positionally symmetrical fastening bolts, and a pre-tightening force of 25562 N is applied to each fastening bolt. The elastic modulus of the virtual material layers is 216.6 GPa and the Poisson ratio is 0.28. The overall finite element model is shown in Fig. 2(a). The finite element model after meshing is shown in Fig. 2(b).

Fig. 2Overall finite element model and meshing of FBSU: a) overall finite element model, b) meshing

a)

b)

2.4. Simulation results of finite element model

Fig. 3 shows the first 8 non rigid body modes of FBSU under no restraint. When processing the corresponding vibration mode diagram, since the flange connection structure analyzed is symmetrical, the natural frequency and vibration mode of every two orders are the same, and only one type of vibration mode is listed. It can be seen from Fig. 3 that the corresponding vibration modes are different at different natural frequencies, and the resulting low-order mode has a larger deformation at the flange bolt connection. Numerical modal analysis of FBSU was performed. Setting the contact relationship and adding the constraint condition, the pre-load force on the 24 bolts is 25562 N, and the first 14 natural frequencies when the FBSU model is unconstrained are shown in Table 1.

Fig. 3Vibration modes of FBSU

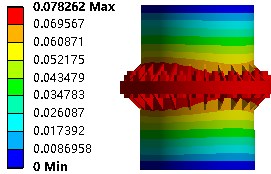
a) Mode 7 and 8

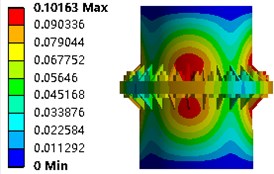
b) Mode 9 and 10

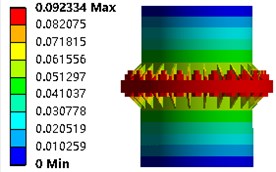
c) Mode 11 and 12

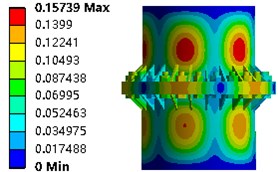
d) Mode 13 and 14

3.1. Basic Principles of experimental modal analysis

Experimental modal analysis is a process in which the response of the system to excitation is measured experimentally after excitation. The digital signal processing is used to obtain the frequency response function, and then the non-parametric model of the forced vibration system is obtained. Parameter identification yields the modal parameters of the system, which is an inverse process of the finite element modal analysis. According to the modal theory, any two frequency response functions are different from each other, each frequency response function is extremely sensitive to the resonant frequency of the structure, and a high peak response is generated at each natural frequency, and the frequency response function is different. The superposition of each other makes the resonance peak more obvious. Therefore, by analyzing the fitting curve of the frequency response function, the various modal frequency values of the experimental structure can be identified. When dealing with practical engineering vibration problems, the comprehensive use of computational modal analysis and experimental modal analysis can provide a sufficient basis for the overall design and inspection of mechanical system structures.

3.2. Experimental setup

The experimental modal analysis was carried out on an experimental size model. The material of the test model is Q235B, the elastic modulus is 200 GPa, the Poisson ratio is 0.3, the density was 7850 kg/m³, and the upper and lower flange steel pipes were connected by 24 M30 bolts. A fixed torque wrench controls the applied pre-load.

In this test, the LMS Test. Lab modal test system, which integrates data acquisition and signal processing, was used to perform modal test on the test model. Fig. 4(a) is an experimental equipment diagram, through which the modal test is performed to obtain the natural frequency and damping ratio. Eight measuring points are arranged both in the upper and lower directions in the figure. In order to obtain the overall dynamic characteristics, the measuring points were evenly arranged on the steel rod. According to the LMS experimental modal analysis process, the model of the measuring point is built in the LMS software, as shown in Fig. 4(b), the test model is excited by a steel hammer. The hand hammer consists of hammerhead, hammer cap, grip and force sensor. Since the test model is too heavy to meet the ideal state of suspension, it was placed on a soft foam during the test to reduce the influence of boundary conditions.

Fig. 4Experimental setup and measuring point model: a) experimental setup, b) measuring point model

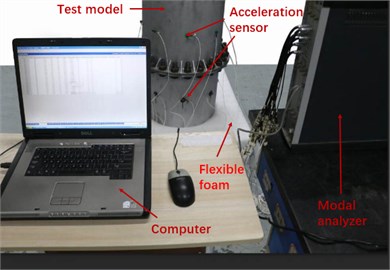
a)

b)

3.3. Verification of finite element model

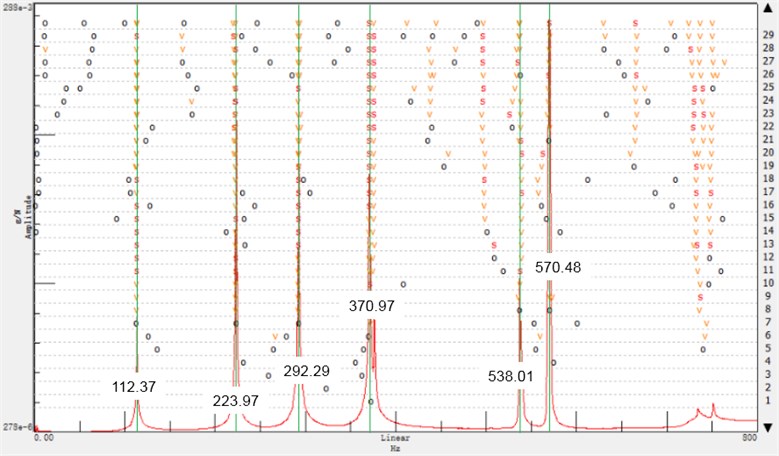
Fig. 5 shows a frequency response function and its corresponding response steady state diagram obtained by processing the vibration signal using Time MDOF method. In Fig. 5, the continues line is the frequency response function, the y label on the right is modal order, ‘v’ means that the mode vector is stable, ‘s’ means that the frequency, damping and mode vectors are stable, ‘o’ means that the pole is unstable. Since the support mode adopts soft foam, the influence of the rigid body modal frequency can be neglected. According to the modal steady state diagram, the corresponding modal frequency is selected, and the experimental modal frequency (EMF) and damping ratio (DR) of 7-14 modes are obtained after verification as shown in Table 2.

Fig. 5Response steady state diagram of FBSU by experiment

It can be seen from the data that there are errors between the simulation results and the experimental results of FBSU, according to the relative error Eq. (4):

where $x_1$ is the simulation results calculated by ANSYS software, and $x_0$ is the experimental results obtained in modal test. The calculated result is shown in Table 3. As can be seen from Table 3, the total error of the test frequency and the average percentage of the simulation frequency is substantially within 15 %.

Modal Assurance Criterion (MAC) is a good tool to evaluate the intersection angle of modal vector space which can be implemented in the LMS system, the frequency dependence of each order can be used to verify the quality of the modal experiment, as shown in Fig. 6. It can be seen from the MAC histogram of Fig. 6 that the values on the diagonal of the MAC matrix are all 1, indicating that the correlation of each mode is 100 %. And the values on the non-diagonal are far less than 1, it shows that the correlation of each order mode is small and relatively independent, which can also prove that the test data is true and effective. Therefore, it can be explained that the virtual material method is effective for the modeling of the flange bolt joint interface, and the measuring point model can effectively obtain the vibration signal of FBSU.

Fig. 6MAC histogram of natural frequencies

4.1. Verification of finite element model

In the FBSU, after the bolt is loosened, the contact stress between the joint interface will change, and the change of the contact stress will result in changing the connection stiffness, which will affect the stiffness distribution and natural frequency of the whole system. The finite element modeling of FBSU needs to be able to effectively simulate the tightness of the bolt. When the connecting bolt set is loose, the pre-load of some partial bolts becomes zero. As the vibration of the whole structure continues to occur, the distribution force of the bolt joints caused by the loosening of some bolts is uneven, which will accelerate the loosening of the adjacent bolts, thereby causing more bolt loosening.

Therefore, the following seven kinds of cases are designed to simulate the early partial bolt loosening of FBSU: Case 1, All 24 bolts are fully tightened (pre-load is rated); Case 2, in a clockwise order, 1 bolt pre-load is reduced to zero, and the other 23 Tightened; Case 3, in the clockwise order, 4 bolts pre-load is reduced to zero, the other 20 are tightened; Case 4, in clockwise order, 8 bolts pre-load is reduced to zero, the other 16 are tightened; Case 5, in clockwise order, 12 bolts The pre-load is reduced to zero, the other 12 are tightened; Case 6, in 16 clockwise, the pre-load of the 16 bolts is reduced to zero, and the remaining 8 are tightened; Case 7, in the clockwise order, the pre-load of the 20 bolts is reduced to zero, and the other 4 Tighten. The different working cases are shown in Fig. 7, where red indicates loosened bolts. For different working cases, the general pre-load is different. The elastic modulus and Poisson ratio of the virtual material used in the processing of the joint interfaces are changed accordingly. The specific parameters are shown in Table 4.

Fig. 7Different working cases of FBSU

a) Case 1

b) Case 2

c) Case 3

d) Case 4

e) Case 5

f) Case 6

g) Case 7

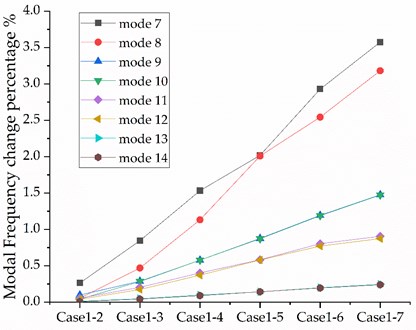
The modal frequencies are obtained by simulation under different working cases. No fixed constraints are added during simulation. The first 6 modes are rigid body free mode, which are not considered. Consider the frequency of non-rigid modal parameters from 7-14 modes. Fig. 8 shows the extent of frequency change of each mode frequency compared to the normal state under different loosened cases, as the bolts loosen, the frequency of high-order modes changes significantly less than the low-order modes.

Fig. 8The variation extent of modal frequency

4.2. Experimental modal analysis of FBSU under different working cases

The experimental modal test of FBSU under different working cases is carried out. The test model, experimental equipment and sensor arrangement are the same as those in Fig. 4(a). The total number of bolts on the test model is 24, and 20 of them are designed to be loosed during the test therefore numbered as shown in Fig. 9. Case 1, all the bolts are tightened. The same pre-tightening force is applied to each bolt with the force wrench. Case 2, the bolt numbered 1 is loose, Case 3, bolts numbered 1-4 are loose. Case 4, bolts numbered 1-8 are loose. Case 5, bolts numbered 1-12 are loose. Case 6, bolts numbered 1-16 are loose. Case 7, bolts numbered 1-20 are loose.

Fig. 9Numbering of bolts on FBSU

The comprehensive frequency response function of the working case 1 was obtained in the former test. Here, the modal test is started from the working case 2, and Fig. 10 is the comprehensive frequency response function of different working cases. Fig. 10 shows that the frequency response function of the test points under different working conditions has obvious difference, and the modal frequency decreases with the increase of the number of loosened bolts, indicating that loose bolts will cause changes in the overall structural modal parameters. The modal frequency was analyzed from the frequency response diagram. Fig. 11 illustrates the modal frequency variation diagram measured under different working cases in simulation and experiment. It can be seen from Fig. 11 that the trend of the natural frequency in the experiment is basically the same as the simulation, but the magnitude of the decrease is larger than the natural frequency of FBSU in the simulation, the obvious difference between the adjacent modal frequencies of experiment in the figure is likely to be caused by the fact that the experimental model is not strictly symmetrical.

Fig. 10Comprehensive frequency response function under different working cases

a) Case 2

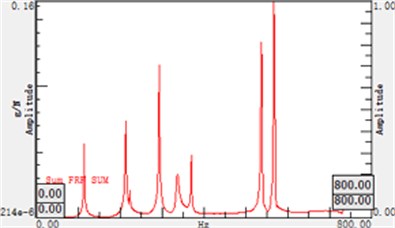
b) Case 3

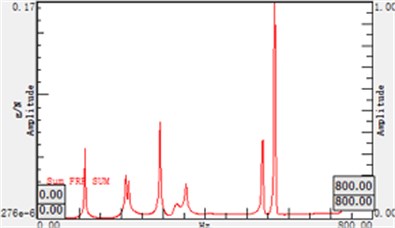
c) Case 4

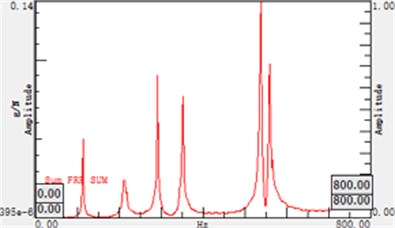
d) Case 5

e) Case 6

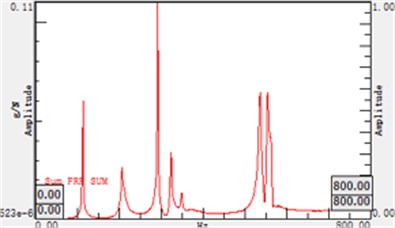
f) Case 7

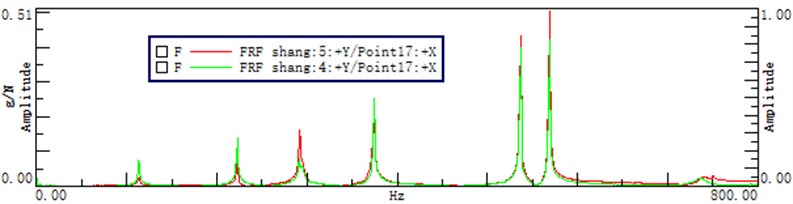
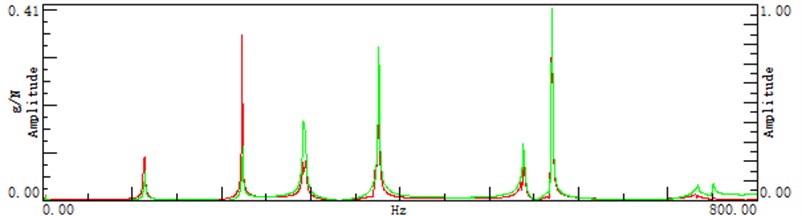
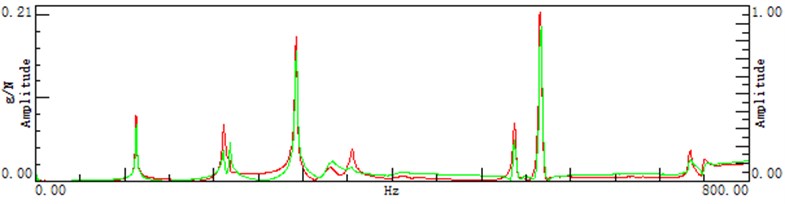
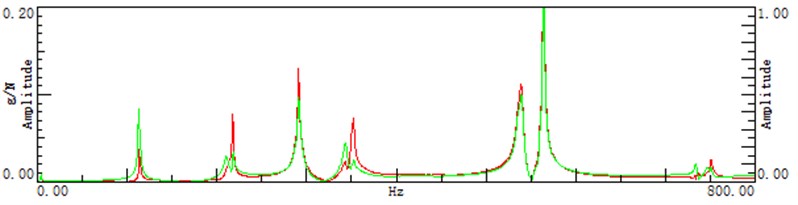
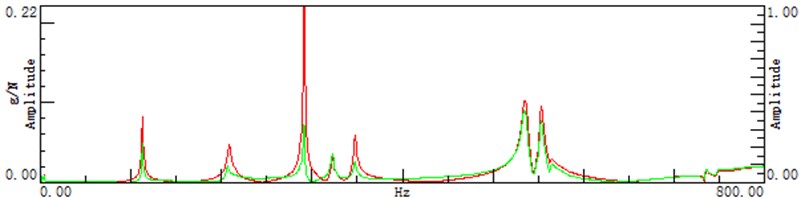
After the excitation at the same position, the frequency response of a certain measuring point will show different response spectrum under different working cases. Therefore, according to this law, the relationship between the natural frequency and the bolt loosening can be further explored, and the same measuring point can be used for different working cases. For the bolt numbered 1, it is tightened in case 1, and it is untightened in case 2 to case 7. The measuring point No. 4 is adjacent to the bolt numbered 1, and the measuring point No. 5 is adjacent to the bolt that has tightened. The response of the measuring point No. 4 and the measuring point No. 5 under different working cases is shown in Fig. 12. The green line in the figure represents the response of measuring point No. 4, and the red line represents the response of measuring point No. 5.

Fig. 12 shows that as the number of loosening bolts increase, the frequency response of measuring point No. 4 and No. 5 changes correspondingly. Fig. 12(a) shows the frequency response with no bolt loose, by comparing the case 1 and case 2-7, we can see that the amplitude of fifth peak decreases significantly both at measuring point No. 4 and No. 5, the amplitude of sixth peak also decreases obviously, the amplitude of second peak and fourth peak shows abrupt change in case 2 and case 4 respectively. The variation of amplitude is likely to become the basis to judge the loose condition of bolts. By comparing the response of different measuring point, we may figure out the approximate position where the bolt is loose. Fig. 12 shows the different variation of response at measuring point No. 4 and measuring point No. 5. When the loosening of bolts occurs, the amplitude of resonance peak at measuring point No. 5 shows more saltation than it at measuring point No. 4.

Fig. 11Frequency variation diagram under different working cases in the simulation and experiment

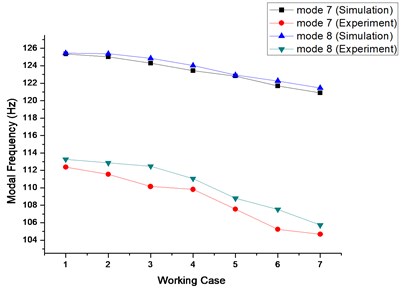
a) Mode 7 and mode 8

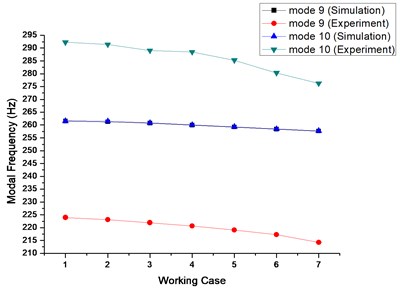
b) Mode 9 and mode 10

c) Mode 11 and mode 12

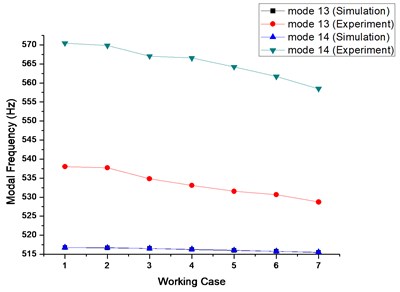
d) Mode 13 and mode 14

Fig. 12Frequency response of measuring point No. 4 and measuring point No. 5 under different working cases