Dynamic analysis of impact on needle valve assembly

2.1. Material definition

Needle valve assembly consists of needle valve and needle body. The material of these two parts is alloy with different processes and can be defined in the software with several of material properties which is shown in Table 1.

Fig. 1Part of the model for finite element model (section)

2.2. Mesh

In order to ensure the accuracy of the calculation, the needle body and the needle valve are sliced and hexahedron mesh is generated for each part. The method of generating the grid uses the grid generation function of Ansys [6].

The size of the mesh requires stress wave theory as a reference. The velocity of the tress wave in materials is calculated by the properties of materials [7]. The equation is:

where $C_d$ is the velocity of the stress wave, $E$ is Young’s modulus and $\rho$ is the density of materials.

According to the material properties, the velocity of the stress wave in this calculate is:

The time of the impact is 0.01 ms according to the measured velocity curve, so the minimum value of the size of mesh $L_{min}$ is:

After choosing different size for each part and the generation of face mesh with refinement on contact surface, the generated mesh is shown in Fig. 2. The statistical result is that the number of nodes is 521743 and the number of elements is 578271.

2.3. Boundary conditions

For transient analysis, the boundary conditions only need to define the impact velocity of the needle valve and support of valve body. The velocity of the needle valve is 1600 mm/s whose direction is vertical down to the needle body. As for the support, chose to fix the needle body.

Fig. 2The mesh model of the impacted part of needle valve assembly

3.1. Equivalent stress (von-mises) curve of the impact surface

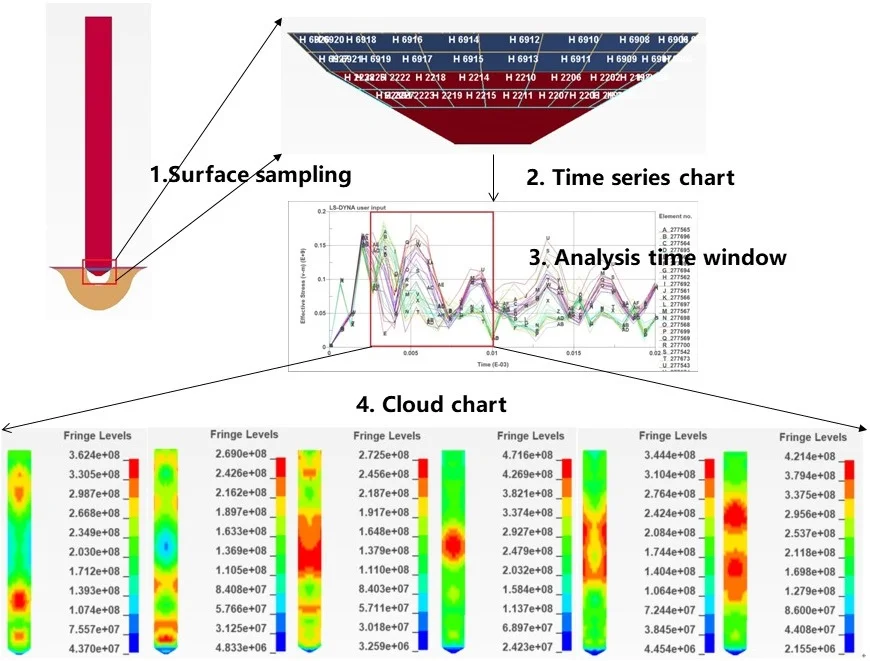
Select the sampling elements on the impact surface of the needle valve and the needle body respectively, which is shown in Fig. 3. After selecting the elements, export the time series diagram of corresponding equivalent stress with the post-processing software like Fig. 4 and Fig. 5. According to the measured velocity curve of needle valve, the impact will occur within 0.01 ms. So the focus of the analysis is on this period and the rest of time series diagram which is in the range of 0.01 ms to 0.02 ms is used to observe the follow-up trends and the condition of its convergence.

Fig. 3Sampling elements on the surface of impact

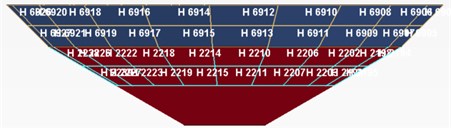
Fig. 4Needle body’s time series diagram of equivalent stress

Fig. 5Needle valve’s time series diagram of equivalent stress

Observing the results from this two time series diagram of equivalent stress within 0.01 ms, there are two large stress fluctuations at 0.0025 ms and 0.008 ms on the impact surface of the needle body. The maximum equivalent stress is about 460 MPa at the time around 0.008 ms. Then the equivalent stress shows the trend of continuous oscillation within the time period after 0.01 ms and the maximum value of this period is decreasing gradually.

On the other hand, one large stress fluctuation occurs at 0.0025 ms on the impact surface of needle valve, whose maximum equivalent stress is about 180 MPa. Then the series diagram of equivalent stress is flattened after some small oscillations.

Analyzing this two time series diagrams of equivalent stress, the entire 0.02 ms time period can be divided into the following phases as is shown in Table 2. In the phase 1, equivalent stress increase gradually. A large stress fluctuation occurred around 0.0025 ms in both needle valve and needle body. It means that this moment which impact energy reaches the climax is around the 0.0025 ms during the period of impact. After this moment, the status of the impact is in phase 2, equivalent stress decrease because of the consumption of the energy, and the influence of the impact is became weaken gradually. In phase 3, the needle valve and valve body is already separated and the concussion of equivalent stress shows that the impact will spread by the form of waves from the impact surface. However, the specific situation is different in needle valve and needle body because of the different boundary conditions in the finite element model and the difference of the material physical parameters and component quality.

3.2. Cloud chart of equivalent stress (von-mises)

Because the output of results is 30 frames set by the solving parameter of finite element model, the cloud chart need to be chosen with larger equivalent stress for stress analysis and strength checking. According to the series diagram of equivalent stress on the surface of the impact, choose the cloud chart during the period from 0.0025 ms to 0.01 ms to analyze.

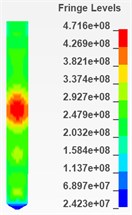
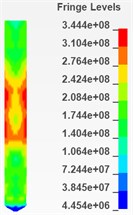
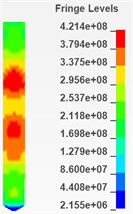
As is shown in Fig. 6 and Fig. 7, there are 6 cloud charts of valve body and 6 of the needle valve.

According to the respective equivalent stress cloud charts, it is found that the equivalent stress of the impacted part of the needle body is less than 573 MPa during the period from 0.0025 ms to 0.005 ms and this period is considered to be more affected by the impact. Therefore, it can be determined that during the impact process, the maximum equivalent stress of the needle body is 573 MPa. Similarly, the maximum equivalent stress of the needle valve is 471 MPa during the period from 0.0025 ms to 0.005 ms and it can also be determined that during the impact process, the maximum equivalent stress of the needle valve is 471 MPa.

Fig. 6Cloud chart of impacted part of needle body from 0.0025ms to 0.005ms (top view)

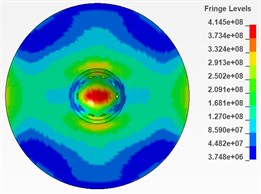
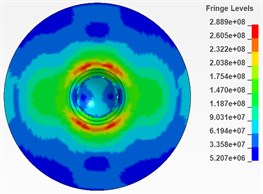
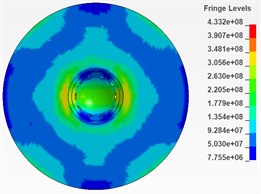
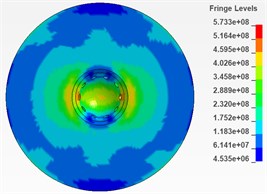
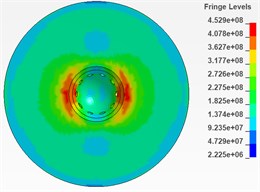
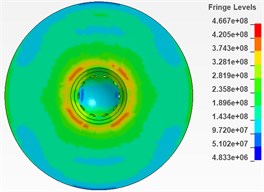
Fig. 7Cloud chart of impacted part of needle valve from 0.0025ms to 0.005ms (section)

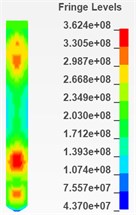
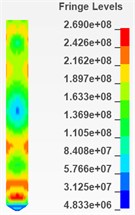
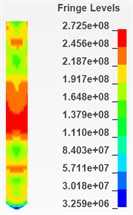
This analysis uses the fourth strength theory for strength checking which will compare the value of equivalent stress multiplied by safety factor with the yield strength. According to the material property, the yield strength of both the needle valve and needle body material is 950 MPa. Under the premise of the value of the safe factor equals 1.5, the comparison formula is as follows. Both the needle valve and needle body are satisfied the requirement of strength: