Mathematical modeling for estimation of acoustic radiation from clamped free tapered annular circular plate having different parabolically varying thickness

Chatterjee, Abhijeet; Ranjan, Vinayak; Azam, Mohammad Sikandar

doi:10.21595/vp.2018.19826

Vibroengineering Procedia

Browse Procedia

Published: 13 December 2018

Check for updates

Mathematical modeling for estimation of acoustic radiation from clamped free tapered annular circular plate having different parabolically varying thickness

Abhijeet Chatterjee¹

Vinayak Ranjan²

Mohammad Sikandar Azam³

^{1, 3}Indian Institute of Technology (ISM), Dhanbad, India

²Bennett University, Greater Noida, India

Corresponding Author:

Abhijeet Chatterjee

Cite the article Download PDF

Downloads 1389

CrossRef Citations 3

Abstract

In this study, a comparison of sound radiation characteristic of a clamped free annular circular plate with different parabolically varying thickness with different taper ratios 0.25, 0.50 and 0.75 with different excitation locations are analyzed by keeping the mass of the plate constant. Rayleigh integral is applied to determine the acoustic behavior of the plate. The same problem is also solved by FEM to draw the comparison. It is observed that excitation locations and modes have a significant effect on sound power level in comparison to stiffness variation due to different taper ratios. Further, the variation of peak sound power level is investigated with different taper ratio with different excitation locations. Finally, the design options for peak sound power are suggested for different excitation locations with different taper ratios.

1. Introduction

In engineering applications, annular circular tapered plates with different combinations of arbitrarily varying thickness are widely used in structural components i.e. diaphragms and deck plates in launch vehicles, diaphragms of turbines, telephone industry, aircrafts/missiles, naval structures, constructions of ships, automobiles and other vehicles etc. Several researchers have examined the acoustic response of circular or annular plate with uniform or tapered thickness. Lee and Singh [1] used the thin and thick plate theories to determine the sound radiation from out-of-plane modes of uniform thickness annular circular plate. Levine and Leppington [2] analyzed the sound power generation of a circular plate of uniform thickness using exact integral representation. Rdzanek and Engel [3] determined the acoustic power output of a clamped annular plate using asymptotic formula. Wodtke and Lamancusa [4] minimized the acoustic power of circular plates of uniform thickness using the damping layer placement. Wanyama [5] studied the acoustic radiation from linearly-varying circular plates. Lee and Singh [6] used the flexural and radial modes of a thick annular plate to determine the self and mutual radiation. Lee and Singh [7] investigated the annular disk acoustic radiation using structural modes through analytical formulations. Rdzanek et al. [8] investigated the sound radiation and sound power of a planar annular membrane for axially-symmetric free vibrations. Hasegawa and Yosioka [9] determined the acoustic radiation force used on the solid elastic sphere. Lee and Singh [10] used a simplified disk brake rotor to investigate the acoustic radiation by a semi-analytical method.

2. Mathematical formulation

2.1. Plate free vibration

The natural frequency and modes shape of the plate is given by Eq. (1):

1

([k] - ω^{2} [M]) ψ_{m n} = 0,

where [ $k$ ] is the stiffness matrix and [ $M$ ] is the mass matrix while $ψ_{m n}$ is the mode shape of structure and $ω$ is the corresponding natural frequency of the plate in rad/sec. The non-dimensional frequency parameter $λ^{2}$ is given by Eq. (2):

2

λ^{2} = ω a^{2} \sqrt{ρ h / D},

where $D$ is the flexure rigidity $D = E h^{3} / 12 (1 - υ^{2})$ , $E$ is Young’s modulus of elasticity, $υ$ is Poisson’s ratio, $h$ is thickness of the plate and $ρ$ is density of plate.

Fig. 1Acoustic radiation due to the vibration modes of unbaffled plate in Z direction enclosed in a sphere

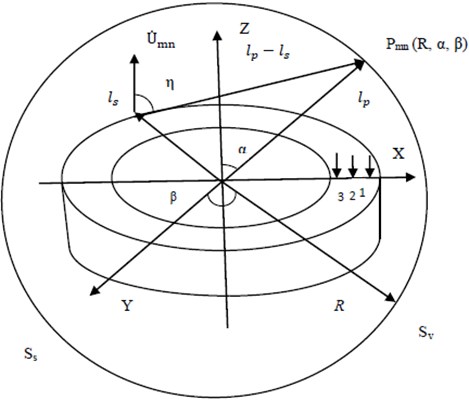
2.2. Analytical and numerical solution for acoustic radiation from annular circular plate

The structure sound radiation obtained by Rayleigh integral [1] is given by Eq. (3):

3

P (l_{p}) = \int_{S_{s}} (\frac{\partial q}{\partial f} (l_{p}, l_{s}) P (l_{p}) - \frac{\partial P}{\partial f} (l_{s}) q (l_{p}, l_{s})) d s (l_{s}),

where, $P$ is the sound pressure amplitude, $S_{s}$ is the surface of the sound source, $q$ is the Green function in free field, $l_{s}$ and $l_{p}$ is the position vectors of source and receiver and $f$ , is the surface normal vector at $l_{s}$ . If, $ρ_{0}$ is the mass density of air, $c_{0}$ is the speed of sound in air, $B$ is the corresponding acoustic wave number, and $\dot{U}$ and $\dot{u}$ are the corresponding vibratory velocity amplitude and spatial dependent vibratory velocity amplitude in the $z$ direction at $l_{s}$ . The sound pressure radiated from non-planar source in far and free field environment based on plane wave approximation can be expressed by Eq. (4):

4

P (l_{p}) = \frac{ρ_{0} c_{0} B}{4 π} \int_{S_{s}} \frac{e^{i B |l_{p} - l_{s}| \dot{U}} (l_{s})}{|l_{p} - l_{s}|} (1 + c o s η) d S .

The modal sound pressure $P_{m n}$ for an annular plate with ( $m$ , $n$ )th mode is obtained from simplifying Eq. (4) with Hankel transform and is expressed by Eqs. (5), (6):

5

P_{m n} (R, α, β) = \frac{ρ_{0} c_{0} B e^{i B_{m n} R_{d}}}{2 R_{d}} c o s n β {(- i)}^{n + 1} A_{n} [\overset{}{\dot{u} (l)}] (1 + c o s η),

6

A_{f} [\overset{}{\dot{u} (l)}] = \int_{0}^{\infty} \dot{u} (l) J_{n} (B_{l} l) l d l, B l = B s i n θ; R_{d} = |l_{p} - l_{s}|,

where $J_{n}$ is Bessel function of order $n$ , ( $α$ , $β$ ) are the cone and azimuthal angles of the observation positions, respectively, $η$ is the angle between the surface normal vector and the vector from source position to receiver position, and $A$ is the Hankel transform. According to the far field condition, $R_{d}$ in the denominator is approximated by $R$ where $R = |l_{p}|$ is considered to be radius of the sphere. If $D_{m n}$ is acoustic intensity and $S_{v}$ is control surface then the modal sound power $S_{m n}$ for the ( $m$ , $n$ )th mode [1, 6] from the far-field is given by Eq. (7):

7

S_{m n} = {(D_{m n} S_{v})}_{s} = \frac{1}{2} \int_{0}^{2 π} \int_{0}^{π} \frac{P_{m n}^{2}}{ρ_{0} c_{0}} R^{2} s i n α d α d β .

The radiation efficiency $σ_{m n}$ of the plate [1] is given by Eq. (8):

8

σ_{m n} = \frac{S_{m n}}{|{\dot{u}}_{m n}^{2}| t s}, |{\dot{u}}_{m n}^{2}| t s = \frac{1}{2 π (a^{2} - b^{2})} \int_{b}^{a} \int_{0}^{2 π} {\dot{U}}^{2} d φ d l,

where, ${|{\dot{u}}_{m n}^{2}|}_{t s}$ is the spatially average r.m.s velocity for two normal surfaces. Considering the plate thickness ( $h$ ) effect, the sum of sound radiations [6] from two normal surfaces of the plate at ( $Z =$ 0.5 $h$ and –0.5 $h$ ) will represent the modal sound power which can be given by Eqs. (9)-(11):

9

P_{m n} (R, α, β) = (1 + c o s α) P_{m n}^{s} (R, α, β) + (1 - c o s α) P_{m n}^{o} (R, α, β),

10

P_{m n}^{s} (R, α, β) = \frac{ρ_{0} c_{0} B_{m n} e^{i B_{m n} R}}{2 R} e^{- i B_{m n} (\frac{h}{2}) c o s α} c o s n β (- i)^{n + 1} A_{n} [\overset{}{\dot{U} (l)}],

11

P_{m n}^{o} (R, α, β) = \frac{ρ_{0} c_{0} B_{m n} e^{i B_{m n} R}}{2 R} e^{- i B_{m n} (\frac{h}{2}) c o s α} c o s n (β + ϕ) (- i)^{n + 1} A_{n} [\overset{}{\dot{U} (l)}],

where, the corresponding acoustic wave number of the ( $m$ , $n$ )th mode is represented by $B_{m, n}$ , $s$ and $o$ in Eq. (10), (11) represent source side and opposite to source side. For numerical analysis, the plate is modeled in ANSYS with Plane 185 with 8 brick node element. FLUID 30 and FLUID130 elements are used to create the acoustic medium environment around the plate. The numbers of elements and nodes come out to be 11351 and 3091 respectively. A force of 1 N is applied at 1, 2 and 3 as shown in Fig. 1. The position chosen are 0.0069 m, 0.0345 m and 0.0621 m for these excitation locations. The air density $ρ_{0} =$ 1.21 kg/m³ and at 20 °C, the speed of sound $c_{0}$ of air is 343 m/s. The structural damping coefficient of the plate is assumed as 0.01.

2.3. Thickness variation of the plate

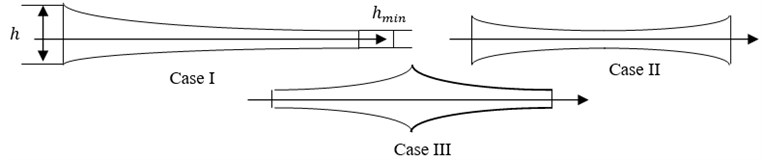
A thick annular circular plate with different parabolically varying thickness is considered for analysis and is reported in Fig. 2. The thickness of the plate is varied in radial direction by keeping the total mass of the plate constant. The plate thickness at radial direction is given by $h_{x} = h [1 - T_{x} {\{f (x)\}}^{n}]$ , where $h$ is considered to be maximum plate thickness and $f (x)$ is considered to be an arbitrary function of ordinate $x$ . Where:

12

f (x) = \{\begin{array}{l} 0, x = b, \\ 1, x = a, \\ \frac{x - b}{a - b}, b < x < a . \end{array}

The taper parameter or taper ratio ( $T_{x}$ ) is given by Eq. (13):

13

T_{x} = (1 - \frac{h_{m i n}}{h}) .

The equations for Case I (parabolically decreasing thickness), Case II plate (parabolically decreasing-increasing) and Case III plate (parabolically increasing-decreasing) thickness variation of (Fig. 2) are given by Eq. (14)-(16):

14

h_{x} = h \{1 - T_{x} {(\frac{x - b}{a - b})}^{n}\},

15

h_{x} = h \{1 - T_{x} {(1 - a b s (1 - 2 \frac{(x - b)}{(a - b)}))}^{n}\},

16

h_{x} = h \{1 - T_{x} a b s {(1 - 2 \frac{(x - b)}{(a - b)})}^{n}\},

where, $n =$ 2 for parabolic profile.

The total volume of the plate is kept constant and is given by Eq. (17):

17

Volume = π (a^{2} - b^{2}) h = \int_{b}^{a} (a^{2} - b^{2}) h_{x} d x .

Fig. 2Plate with different parabolic varying thickness variation

3. Results and discussions

3.1. Validation of modal frequency

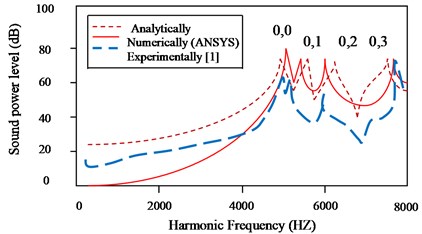
For validation of modal frequency of thick annular isotropic plate, the published result of Lee et al. [1] is taken as reported in Table 2. From Table 2 it is clear that the results obtained in this paper matches well with the published results [1]. For acoustic power calculation the computed analytical, numerical and experimental results [1] are considered as reported in Fig. 3. From Fig. 3, a well and good agreement of computed acoustic results obtained analytically, numerically with published experimental results [1] is seen and matches well with each other.

Table 1The specification and the material properties of the annular circular plate

Dimension of the plate	Isotropic annular circular plate
Outer radius $a$ (m)	0.1515
Inner radius $b$ (m)	0.0825
Radii ratio, $b$ / $a$	0.54
Thickness ratio, $h$ / $a$	0.21
Density, $ρ$ (kg/m³)	7905.9
Young’s modulus, $E$ (GPa)	218
Poisson’s ratio, $υ$	0.305

Table 2Comparison and validation of frequency parameter λ2 of isotropic annular circular unloaded plate for taper ratio, Tx= 0.00 obtained in present work with that of Lee et al. [1]

Plate	Mode	Non dimensional frequency parameter, $λ^{2}$
Plate	Mode	H. Lee et al. [1]	Present work
Uniform unloaded plate $b / a =$ 0.54 $h / a =$ 0.21	(0, 0)	13.61	13.4929
	(0, 1)	13.63	13.4946
	(0, 2)	14.28	14.1185
	(0, 3)	16.81	16.6681

Fig. 3Analytical, experimental and numerical comparison of sound power level of unloaded plate having uniform thickness for taper ratio Tx= 0.00

3.2. Acoustic response solution of parabolic tapered plate for different taper ratios

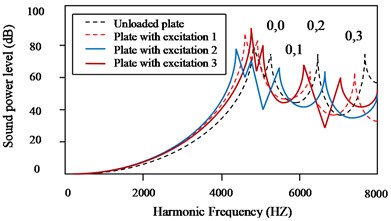
The sound power level (dB, reference = 10^-12 watts) of parabolic tapered annular circular plate at different excitation locations are investigated by applying concentrated load under time-varying harmonic excitations. The frequency range of 0-8000 Hz is taken. From Figs. 4(a)-(c) it is observed that for all cases of thickness variation and for excitation frequency up to 1000 Hz, effect of different excitation locations and stiffness variation due to taper ratio $T_{x} =$ 0.75 do not have significant effect on sound paper radiation for clamped-free boundary condition.

Fig. 4Comparison of sound power level (dB) for: a) Case I plate, b) Case II plate, c) Case III plate having different parabolic thickness variation for different excitation locations for taper ratio Tx= 0.7

Comparison of sound power level (dB) for: a) Case I plate, b) Case II plate, c) Case III plate having different parabolic thickness variation for different excitation locations for taper ratio Tx= 0.7

a) Case I plate (parabolic decreasing)

b) Case II plate (parabolic decreasing-increasing)

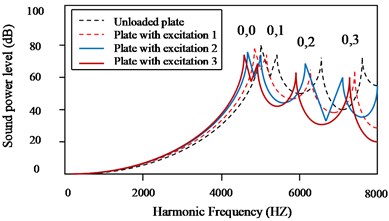
c) Case III plate (parabolic increasing-decreasing)

3.3. Peak sound power level variation with different taper ratio for all excitation locations

Peak sound power level for plate having different parabolic varying thickness for different taper ratio is shown in Figs. 5(a)-(c). Peak sound power level for all excitation locations is reported at first peak which corresponds to (0, 0) mode of the plate. It is clear that for Case I and Case II plates under excitation location 1 and 2 peak sound power level is maximum as well as minimum at $T_{x} =$ 0.75 and for excitation locations 1 and 2 for Case III plate, peak is always minimum at $T_{x} =$ 0.75. But under excitation 3 i.e. towards mid position, a variation of peak sound power level is observed for all cases of thickness variation. For Case I plate toward mid excitation, peak is minimum at $T_{x} =$ 0.25 and maximum at $T_{x} =$ 0.50. Similarly, for Case II plate, at mid excitation, highest peak sound power level is obtained at $T_{x} =$ 0.75 and minimum at $T_{x} =$ 0.50. Further, for Case III plate, under mid excitation, the lowest peak sound power level is obtained at $T_{x} =$ 0.75 and maximum at $T_{x} =$ 0.50. It is thus quite obvious that excitation location has significant impact on peak sound power level. Further excitation locations with different taper ratio provide us design options for peak sound power level. As for example, for peak sound power reduction, taper ratio $T_{x} =$ 0.75 with excitation location 2 for Case I and II plate and $T_{x} =$ 0.75 with mid excitation location for Case III plate may be the option. Similarly, for sound power actuation, taper ratio $T_{x} =$ 0.75 with excitation location 1 for Case I plate towards outer radius and $T_{x} =$ 0.75 for mid excitation location for Case II plate may be the another form of solution.

Fig. 5Peak sound power level (dB) for: a) Case I, b) Case II, c) Case III plate having different parabolic thickness variation

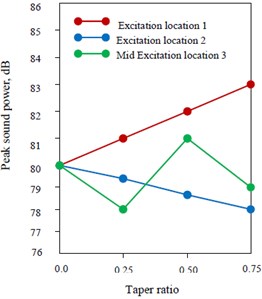
a)

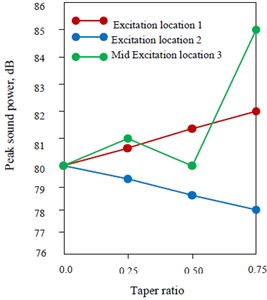
b)

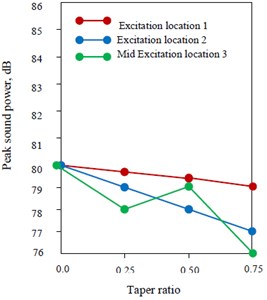
c)

4. Conclusions

The sound radiation behavior of a parabolic tapered annular circular plate in air medium with different taper ratio is analyzed. It is observed that excitation locations and modes variation have significant impact on sound power level in comparison to the stiffness variation due to taper ratio. It is further investigated that peak sound power level either decreases or increases purely depends on excitation locations and taper ratios. For excitation location 1 peak sound power level increases with taper ratios while for excitation location 2 it decreases with taper ratios for all cases of thickness variations. But for mid excitation, peak sound power level is seen to be fluctuated between maximum and minimum for all cases of thickness variations. Further, excitation locations with different taper ratio provide us design options for peak sound power level. For peak sound power reduction, taper ratio $T_{x} =$ 0.75 with excitation location 2 for Case I and II plate and $T_{x} =$ 0.75 with mid excitation location for Case III plate may be the option. Similarly, for sound power actuation, taper ratio $T_{x} =$ 0.75 with excitation location 1 for Case I plate towards outer radius and $T_{x} =$ 0.75 for mid excitation location for Case II plate may be the other solution.

References

Lee H., Singh R. Acoustic radiation from out-of-plane modes of an annular disk using thin and thick plate theories. Journal of Sound and Vibration, Vol. 282, Issues 1-2, 2005, p. 313-339.

Publisher
Levine H., Leppington F. G. A note on the acoustic power output of a circular plate. Journal of Sound and Vibration, Vol. 121, Issue 5, 1988, p. 269-275.

Publisher
Rdzanek Jr W. P., Engel Z. Asymptotic formula for the acoustic power output of a clamped annular plate. Applied Acoustics, Vol. 60, Issue 5, 2000, p. 29-43.

Publisher
Wodtke H. W., Lamancusa J. S. Sound power minimization of circular plates through damping layer placement. Journal of Sound and Vibration, Vol. 215, Issue 5, 1998, p. 1145-1163.

Publisher
Wanyama W. Analytical Investigation of the Acoustic Radiation from Linearly-Varying Circular Plates. Ph.D. Thesis, Texas Tech University, 2000.

Search CrossRef
Lee H., Singh R. Self and mutual radiation from flexural and radial modes of a thick annular disk. Journal of Sound and Vibration, Vol. 286, Issues 4-5, 2005, p. 1032-1040.

Publisher
Lee M. R., Singh R. Analytical formulations for annular disk sound radiation using structural modes. Journal of the Acoustical Society of America, Vol. 95, Issue 6, 1994, p. 3311-3323.

Publisher
Rdzanek W. J., Rdzanek Jr W. P. The real acoustic power of a planar annular membrane radiation for axially-symmetric free vibrations. Archives of Acoustics, Vol. 22, Issue 4, 1997, p. 455-462.

Search CrossRef
Hasegawa T., Yosioka K. Acoustic radiation force on a solid elastic sphere. Journal Acoustical Society of America, Vol. 46, Issue 5, 1969, p. 139-143.

Publisher
Lee H., Singh R. Determination of sound radiation from a simplified disk brake rotor using a semi-analytical method. Noise Control Engineering Journal, Vol. 52, Issue 5, 2000, p. 225-238.

Publisher

Cited by

Analysis of sound radiation from a vibrating elastically supported annular plate using compatibility layer and radial polynomials

Wojciech P. Rdzanek | Jerzy Wiciak | Marek Pawelczyk

(2022)

Experimental and Numerical Investigation of Sound Radiation from Thin Metal Plates with Different Thickness Values of Free Layer Damping Layers

İlhan Yılmaz | Ersen Arslan | Kadir Çavdar

(2021)

Sound radiation by a vibrating annular plate using radial polynomials and spectral mapping

Wojciech P. Rdzanek | Krzysztof Szemela

(2019)

About this article

Received

05 March 2018

Accepted

20 March 2018

Published

13 December 2018

SUBJECTS

Acoustics, noise control and engineering applications

DOI

https://doi.org/10.21595/vp.2018.19826

Keywords

thick annular plate

excitation location

taper ratio

sounds power level

This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Previous article in issue Previous Next article in issue Next

Research article

2021 01 08

Minimizing the radiated sound power from vibrating plates by using in-plane functionally graded materials

Nabeel Alshabatat, Koorosh Naghshineh

Research article

2020 04 02

Numerical investigation of sound transmission loss of circular plates embedded with acoustic black hole

Xiaofei Du, Jianrun Zhang

Research article

2017 09 30

Numerical optimization for acoustic performance of the micro-perforated plate and its application in high-speed trains

Yang Li, Chen Guo

Research article

2016 12 31

A Unified method for vibration analysis of moderately thick annular, circular plates and their sector counterparts subjected to arbitrary boundary conditions

Fazl e Ahad, Dongyan Shi, Anees Ur Rehman, Hafiz M. Waqas

A. Chatterjee, V. Ranjan, and M. S. Azam, “Mathematical modeling for estimation of acoustic radiation from clamped free tapered annular circular plate having different parabolically varying thickness,” Vibroengineering PROCEDIA, Vol. 21, pp. 161–167, Dec. 2018, https://doi.org/10.21595/vp.2018.19826

Copy Extrica

Copied to clipboard!

TY  - JOUR
DO  - 10.21595/vp.2018.19826
UR  - https://doi.org/10.21595/vp.2018.19826
TI  - Mathematical modeling for estimation of acoustic radiation from clamped free tapered annular circular plate having different parabolically varying thickness
T2  - Vibroengineering PROCEDIA
AU  - Chatterjee, Abhijeet
AU  - Ranjan, Vinayak
AU  - Azam, Mohammad Sikandar
PY  - 2018
DA  - 2018/12/13
PB  - JVE International Ltd.
SP  - 161-167
VL  - 21
SN  - 2345-0533
SN  - 2538-8479
ER  - 

Copy Ris

Copied to clipboard!

@article{Chatterjee_2018,
	doi = {10.21595/vp.2018.19826},
	url = {https://doi.org/10.21595/vp.2018.19826},
	year = 2018,
	month = {dec},
	publisher = {{JVE} International Ltd.},
	volume = {21},
	pages = {161--167},
	author = {Abhijeet Chatterjee and Vinayak Ranjan and Mohammad Sikandar Azam},
	title = {Mathematical modeling for estimation of acoustic radiation from clamped free tapered annular circular plate having different parabolically varying thickness},
	journal = {Vibroengineering {PROCEDIA}}
}

Copy Bibtex

Copied to clipboard!

[1]A. Chatterjee, V. Ranjan, and M. S. Azam, “Mathematical modeling for estimation of acoustic radiation from clamped free tapered annular circular plate having different parabolically varying thickness,” Vibroengineering PROCEDIA, vol. 21, pp. 161–167, Dec. 2018, doi: 10.21595/vp.2018.19826.

Copy IEEE

Copied to clipboard!

Chatterjee, Abhijeet, Vinayak Ranjan, and Mohammad Sikandar Azam. “Mathematical Modeling for Estimation of Acoustic Radiation from Clamped Free Tapered Annular Circular Plate Having Different Parabolically Varying Thickness.” Vibroengineering PROCEDIA 21 (December 13, 2018): 161–67. https://doi.org/10.21595/vp.2018.19826.

Copy Chicago

Copied to clipboard!

Mathematical modeling for estimation of acoustic radiation from clamped free tapered annular circular plate having different parabolically varying thickness

Abstract

1. Introduction

2. Mathematical formulation

2.1. Plate free vibration

2.2. Analytical and numerical solution for acoustic radiation from annular circular plate

2.3. Thickness variation of the plate

3. Results and discussions

3.1. Validation of modal frequency

3.2. Acoustic response solution of parabolic tapered plate for different taper ratios

3.3. Peak sound power level variation with different taper ratio for all excitation locations

4. Conclusions

References

Cited by

About this article

Related Articles