Seismic responses and dynamic characteristics of boom tower crane basing on measured strong earthquake excitation

3.1. Element types and material properties

Boom tower crane is mainly comprised of boom, cab, A-frame, balance arm with counterweight, mast, trolley mechanism, slewing mechanism and hoisting mechanism, etc. According to the structural characteristics of tower crane, mast sections, boom, A-frame, the balance arm were modelled by Beam188 element. Slewing mechanism was simulated by Shell63 element. Counterweight of balance arm, cab, electric machinery and hook of trolley or hoisting mechanism were simplified to be lumped-mass and Mass21 element was used. The luffing and hoist cables were considered as pure tensile members, which can be modelled with Link180 element. The main unit parameters of boom and mast were shown in Table 1.

3.2. Basic assumptions

In order to provide an accurate and realistic result in dynamic analyses of crane system, this paper adopted simplified finite-element models. The final model quality, which was equivalent to the real tower crane, was determined by adjusting the density and elastic modulus of element. The connection between mast sections including ear-plate joint, pins and bolts were not considered in the model, and were regarded as rigid connections simply.

The mast of tower crane could be braced at various elevations of the inner concrete core with tie-in struts. The models of tower crane were analyzed separately without considering the building-crane interaction. The type, level, direction and position of load applied on tower crane model were defined in accordance with the real load of an actual tower crane when operating. Meanwhile, all the stresses and strains of the structural members were in linear elastic range and Hookes law was applied.

4.1. Dynamic characteristics results

The subspace iteration method was applied to analyse the structural modal characteristics. First five natural frequencies and mode shapes of tower crane under different working conditions were obtained, as shown in Table 3, which included the deformation characteristics of corresponding modes. Furthermore, five kinds of vibration patterns were labelled with Type-A to Type-E, according to deformation characteristics respectively. Because the first few modes are usually important in structural dynamics, Fig. 3-Fig. 5 showed the vibration-distortion of the first, third and fifth mode of crane models. Fig. 6 showed the changes of natural frequencies of tower crane at different jib angles.

Fig. 2Finite-element models of boom tower crane: a) working condition one, b) working condition two, c) working condition three

a) WC-1

b) WC-2

c) WC-3

Fig. 3Vibration pattern of working condition one (WC-1): a) first mode, b) third mode, c) fifth mode

a)

b)

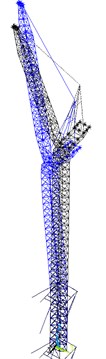
c)

Fig. 4Vibration pattern of working condition two (WC-2): a) first mode, b) third mode, c) fifth mode

a)

b)

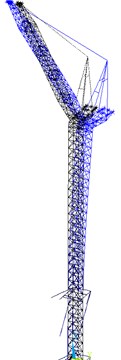
c)

Fig. 5Vibration pattern of working condition three (WC-3): a) first mode, b) third mode, c) fifth mode

a)

b)

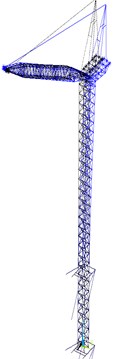
c)

Fig. 6Effect of jib angles on natural frequencies of mode types

As can be seen from above, it was found that:

(1) Table 3 showed that the change of jib angle had different effects on the natural frequencies. With the decreasing jib angle from WC-1 to WC-3, the natural frequencies of the first, fourth and fifth mode increased. But the second mode decreased and the third mode changes little.

(2) The overall deformation characteristics of the first five vibration modes under different working conditions were basically identical. And the corresponding modal orders changed, in addition to the fifth vibration mode that remains unchanged.

(3) Fig. 6 showed that some mode types were insensitive to the change of jib angle, while others change significantly depending on the mode shapes. For example, the Type-E mode was actually related to complex coupled boom deformations and less dependent on the jib angle, as in Figs. 3(c)-5(c).

(4) The main vibration modes of crane model were mainly characterized by the vibration of boom and coupled structural deformations of the mast, such as cantilever deformation, twisting or sway in Fig. 3-Fig. 5. From WC-1 to WC-3 with jib angle decreasing, the modal order of Type-A and Type-B vibration modes exchanged with each other, which indicated that Type-B vibration mode replaced Type-A as the dominant vibration pattern gradually. The natural frequency of Type-A vibration mode increased while Type-B vibration mode decreased. It is indicated that the stiffness of mast resisting to sway becomes larger, while the stiffness of boom resisting to swing becomes smaller. A broken boom occurred more easily than tower crane overturning.

4.2. Measurement of dynamic characteristics

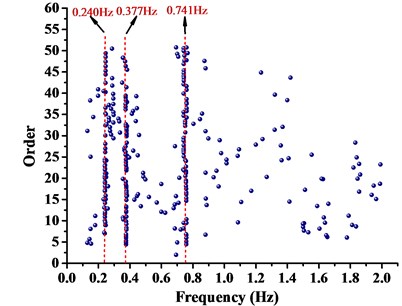
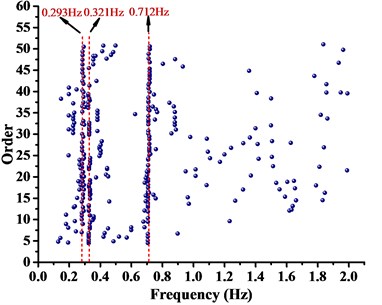
Environment random vibration stimulating method was applied to test structural dynamic characteristics, and acceleration transducer of ICP type was used to carry out data measurement. Based on engineering experience and numerical simulation, the sampling frequency was 100.0 Hz and sampling instant was 16.0 min. According to theoretical analysis and site construction condition, acceleration transducer should be arranged in location with large structural response. ZSL1250 boom tower crane and location of acceleration transducer was shown in Fig. 7. The directions of the acceleration transducer were consistent with the finite-element models of boom tower crane. Acceleration transducers were respectively arranged on the top of A-frame, in the jib head and in the middle of tower and jib. SSI method was chosen to distinguish low-end natural vibration frequencies of boom tower crane and analysis results are shown in Fig. 8.

Fig. 7ZSL1250 Boom tower crane and location of sensors

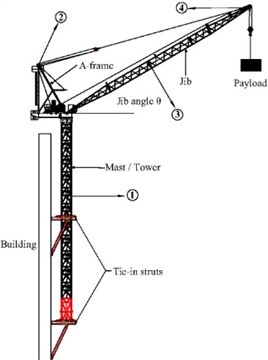
From Fig. 8, it can be seen that natural vibration frequencies of boom tower crane were distinct and accurate. The first three natural vibration frequencies of WC-1, WC-2 and WC-3 were demonstrated and compared in Table 4. It indicated that the dynamic properties of the first steps by theory essentially agreed with that by practical test. Finite-element models were established with high accuracy. Therefore, the analysis method was applicable, and the results were convincible.

Fig. 8Modal identification with SSI: a) working condition one, b) working condition two, c) working condition three

a) WC-1

b) WC-2

c) WC-3

5.1. Ludian seismic wave

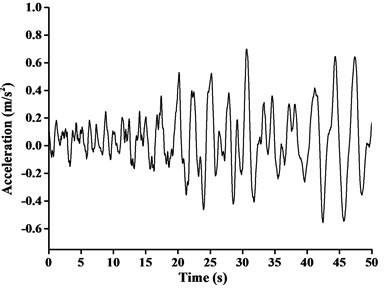
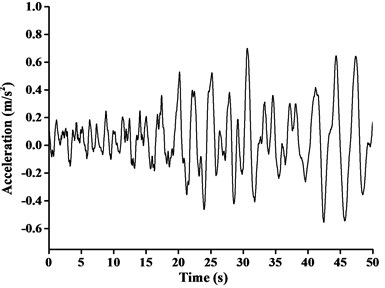
In this paper, construction site of boom tower crane was situated in Kunming, Yunnan province of China. Consequently, Ludian Ms6.5 earthquake acceleration record, which was the measured strongest and nearest ground motion record, was considered to be three-direction uniform seismic excitation. The peak ground acceleration of $x$-direction, $y$-direction and $z$-direction of Ludian earthquake ground motion was 2.66 m/s², 2.85 m/s² and 1.09 m/s² respectively. The time interval between recorded data was 0.04 s, and the total number of data points recorded was 1250 and the duration was 50.0 s. The max value of ground acceleration applied in time-history analysis is required to be 0.7 m/s² when the anti-seismic fortification grade is Ms8.0 [1]. Based on this provision, the seismic ground acceleration curves adjusted by equal proportion scaling (the scaling factor as 0.7/2.66, 0.7/2.85 and 0.7/1.09 respectively) in different directions were shown in Fig. 9, the directions of the seismic was consistent with the finite-element models of boom tower crane.

From the dynamic analysis, the effect of horizontal seismic force at least in two main axis directions of the building should be discussed. For those specific structures with unsymmetrical mass and stiffness distribution, it is also vital to investigate torsional effect under bi-directional horizontal earthquake ground motion at the same time. Moreover, the influence of vertical earthquake action on long-span structures, long cantilever structures or high-rise buildings needs to be focused [18]. For accurate calculation and comparison, the uniform excitation input of adjusted three-dimensional Ludian seismic wave was introduced in time-history analysis of this paper. The combination values of tri-directional components of earthquake ground acceleration were assumed to be 1.00, 0.85, 0.65, respectively in horizontal component ($x$ and $y$-directions) and vertical component ($z$-direction).

Fig. 9Ludian seismic ground acceleration curve in three-direction: a) x-direction, b) y-direction, c) z-direction

a)

b)

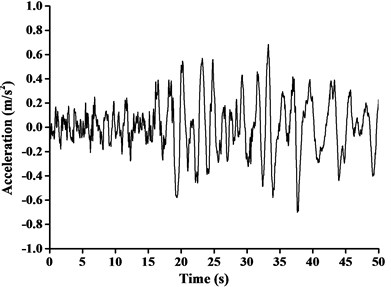
c)

5.2. EI Centro seismic wave

Differing from three-dimensional seismic excitation input above, EI Centro earthquake acceleration record (with a 3.42 m/s² peak ground acceleration) was considered in order to study the effect of one-dimensional seismic excitation on dynamic characteristics of boom tower crane comparative analysis. According to code for seismic design, EI Centro seismic ground acceleration curve was adjusted in the way described above, as shown in Fig. 10 (with a scaling factor 0.7/3.42). The time interval between recorded data, total number of data points recorded, and duration were the same as previously stated. The $x$-direction, $y$-direction and $z$-direction were separately selected as the single direction of seismic wave input from WC-1 to WC-3. Then the dynamic response analysis results of crucial nodes or elements of crane model at any time of excitation input can be obtained.

6.1. Dynamic response under Ludian earthquake excitation

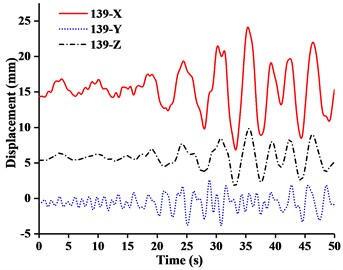
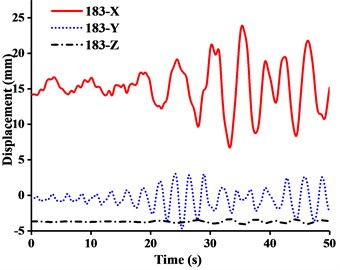
The aim of multi-dimensional input Ludian seismic wave was to evaluate the overall movement of tower crane under simulation of real seismic process. By comparing the time-history analysis result of crane models under WC-1, WC-2 and WC-3, the main concern was concentrated on the displacements of four nodes of important structural members, including No. 216 node (the jib head), No. 139 node (the end of balance arm), No. 183 node (the mast top), and No. 215 node (the A-frame top). The displacement-time history curves of four nodes under three-dimensional Ludian seismic wave were shown in Figs. 11-13. Fig. 14 showed the stress nephograms of crane models when the max unidirectional displacement of nodes occurred.

Fig. 10EI Centro seismic ground acceleration curve

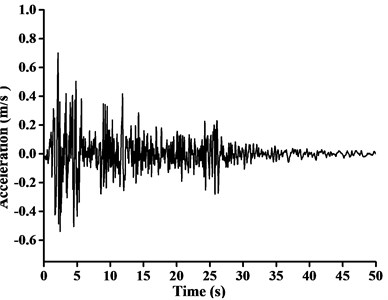
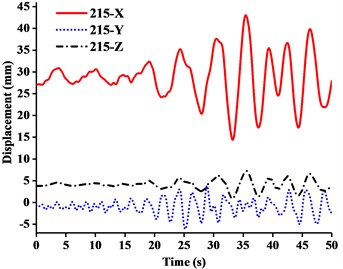
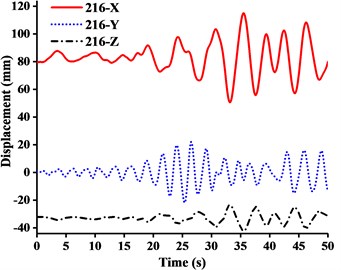
Fig. 11Displacement-time curves of WC-1 under three-dimensional Ludian seismic wave: a) No. 139 node, b) No. 183 node, c) No. 215 node, d) No. 216 node

a)

b)

c)

d)

It can be seen from the Figs. 11-14 that:

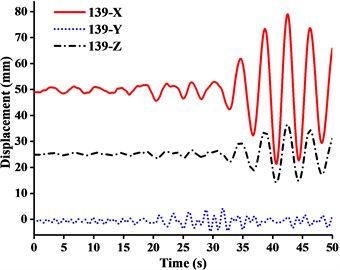
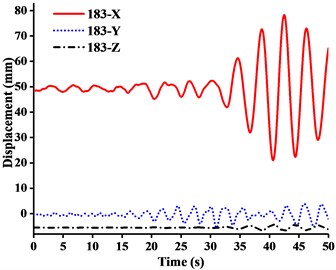
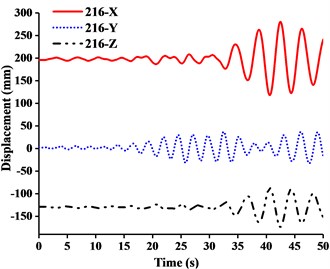
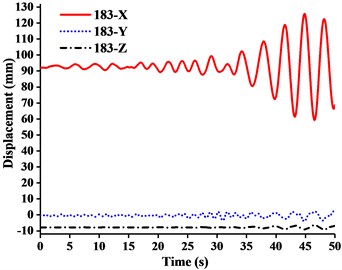
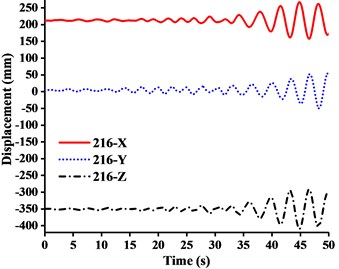
(1) Figs. 11-13 showed that the nodal displacements oscillated about and approach the static values due to the seismic excitation. The initial displacements of four nodes in $x$-direction and z-direction was not zero under self-gravity. Due to the static action of payload on the jib head, the displacement of jib head was always the maximum at any time of seismic duration. The maximum amplitude of dynamic response of nodal displacements was greater than the static displacement. Taking No. 216 node of WC-2 in Fig. 12(d) as an example, the dynamic amplification factors of displacement are 1.43, 16.3 and 1.35, respectively in $x$, $y$ and $z$-direction (vertical direction). Therefore, the earthquake excitation had a significant influence on boom tower crane under fully loaded working conditions.

(2) From Figs. 11-13, it was found that the displacement of $y$-direction was always closed to zero under all working conditions. The nodal displacements were concentrated in $x$-direction or $z$-direction. In comparison, the relative displacement in $y$-direction was negligible. The oscillation wave crest had significantly hysteresis action with jib angle decreasing. Figs. 12 and 13 showed that when the earthquake continued to about 35.0 s, the amplitude got larger drastically in the last 15.0 s. From the point of view of structure form, it is indicated that the heavy weight or the payload is equal to a damper because of its inertia. Once it absorbs a certain amount of earthquake energy, the jib and tower are going to absorb seismic energy directly, which leads to a sudden stronger vibration consequently. Tower crane may be damaged without warning.

(3) From Fig. 11(d) and Fig. 13(d), we can know that although there was a 75.0 % decrease in the payload, the peak response of the displacement of crane model increased by about 2.6 times on the contrary. It is indicated that the effect of structural form changes is far greater than that of the payload under seismic excitation. The smaller jib angle is, the greater destructive effect of earthquake on working tower crane becomes sharply.

Fig. 12Displacement-time curves of WC-2 under three-dimensional Ludian seismic wave: a) No. 139 node, b) No. 183 node, c) No. 215 node, d) No. 216 node

a)

b)

c)

d)

6.2. Dynamic response under EI Centro earthquake excitation

In order to study dynamic response characteristics and failure modes of tower crane under unidirectional earthquake excitation, the results of dynamic response of No. 216 and No. 183 node and stresses of crane model were presented in Table 5. It showed that under fully loaded working conditions, the displacement responses of crane models in $x$ and $z$-direction were mainly controlled by $x$-directional seismic excitation, while the displacement response in $y$-direction was controlled by $y$-directional seismic excitation. The displacement responses were concentrated at the jib head or mast top. Although the amplitude of key nodal displacements became larger from WC-1 to WC-3 under $z$-direction seismic excitation, it was still far less than the displacement induced by the other two directions. Therefore, the effect of $z$-directional earthquake excitation on tower crane was unremarkable.

Fig. 13Displacement-time curves of WC-3 under three-dimensional Ludian seismic wave: a) No. 139 node, b) No. 183 node, c) No. 215 node, d) No. 216 node

a)

b)

c)

d)

For further investigation on the failure modes or weak parts caused by $x$ or $y$-directional seismic excitation, the dynamic responses of the displacement at the jib head (No. 216 node) and the mast top (No.183 node) under $x$ or $y$-directional seismic wave were described in Figs. 15 and 16.

The following were summarized from Table 5, Fig. 15 and Fig. 16.

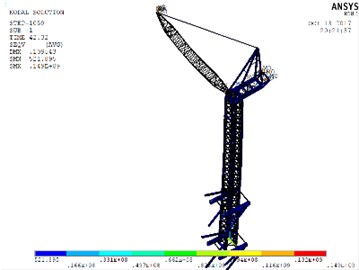
(1) Table 5 showed that the response of stress peak of crane model generated by $x$, $y$, or $z$-direction seismic waves gradually decreases. Under different working conditions, the displacement responses of crane models in $x$ and $z$-direction were mainly controlled by $x$-direction seismic waves, while the displacement response in $y$-direction was controlled by $y$-direction seismic waves. The tip displacement of jib was always the largest at any time of seismic duration. Because of the capacity in the tip under $x$-direction seismic excitation, the earthquake would produce inertial acceleration on the lift and caused a cantilever beam load that was far greater than required capacity. And there was also a great vibration of jib from up and down at the same time. Therefore, the $x$-direction seismic wave had the greatest destructive effect on tower crane. It was most likely to cause a broken jib under working conditions.

(2) Fig. 15(a) showed that when under $x$-direction seismic excitation with working condition one (WC-1), the $x$-direction displacement of the jib head was about 3.5 times the $z$-direction displacement. The deformation mode of tower crane was analogous to the cantilever deformation of beam, the end of which was fixed on the ground. The bending stiffness of mast generated by standard mast sections made a major contribution to resisting overturning in $xoz$ plane. Meanwhile the movement of the boom at small angle tended to be independent on the overall structural deformation. Therefore, when the boom was at large or small angle, the tower crane was inclined to be the global or the boom motion system.

(3) Fig. 15 showed that from WC-1 to WC-3 under $x$-direction seismic wave, the $z$-direction displacement of the jib head exceeded the $x$-direction displacement eventually and got close to the $x$-direction displacement of the mast top. It was shown that the movement of jib tended to be independent on the overall structural deformation, instead of being a part of the cantilever deformation of mast.

Fig. 14Stress nephograms of models when the max unidirectional displacement of nodes occurs: a) WC-1, b) WC-2, c) WC-3

a)

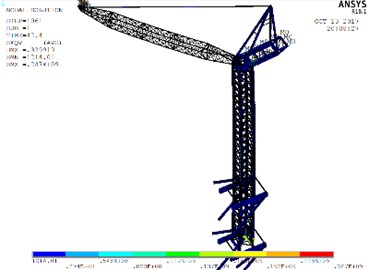
b)

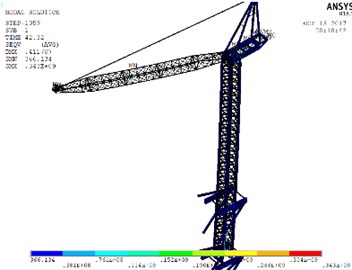
c)

Fig. 15Displacement-time curves under x-direction seismic excitation: a) WC-1, b) WC-2, c) WC-3

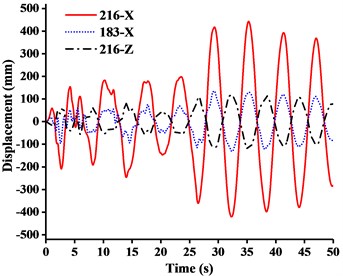
a)

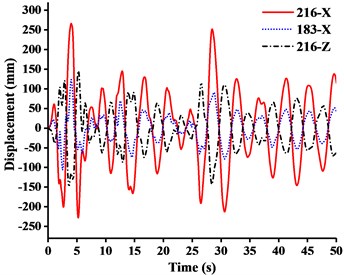
b)

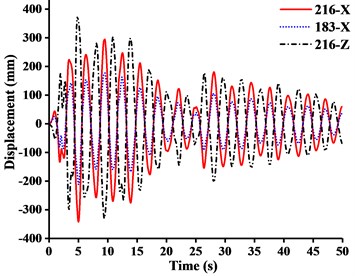
c)

Fig. 16Displacement-time curves under y-direction seismic excitation: a) WC-1, b) WC-2, c) WC-3

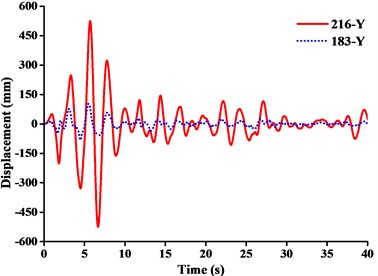
a)

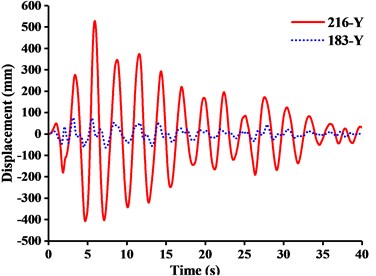
b)

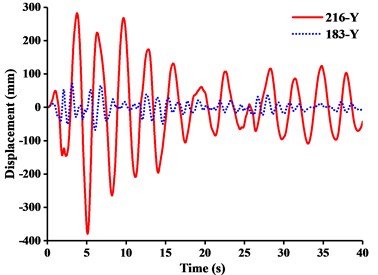
c)