Effects of axial movements of the ends and aspect ratio of laminated composite beams on their non-dimensional natural frequencies

1. Introduction

Composite have been used in engineering structures over the last four decades or so. They could be seen in a variety of applications as in craft wings and fuselage, satellites helicopter blades, wind turbines boats and vessels, tubes and tanks etc. Their advantages over traditional materials are widely recognized and these are high strength to weight ratio, and their properties which can tailored according to need. Other advantages include high stiffness, high fatigue and corrosion resistance, good friction characteristics, and ease of fabrication. They are made of fiber such as glass, carbon, boron, etc. embedded in matrix or suitable resin that act as binding material. The increasing use of composites has been required a good understanding of composite mechanics and their behavior. Many mathematical models for laminates subjected to static and dynamic loading have been developed. This paper addresses free vibration. The knowledge of the few lower natural frequencies of a structure is utmost importance in order to save it in service from being subjected to unnecessary large amplitude of motion which can cause immediate collapse or ultimate failure by fatigue.

Free vibration analysis of laminated composite beams is presented by P. Subramanian, R. A. Jafari-Talookolaei et al. and A. Pagani [1-3] reference [1] used two higher order displacement based shear deformation theories, while references and [2, 3] used the first order shear deformation theory. M. Rueppel et al. [4] studied the damping of carbon fibre and flax fibre angle-ply composite laminate. Torabi K. et al. [5] Investigated on the effects of delamination size and its thickness-wise and lengthwise location on the vibration characteristics of cross-ply laminated composite beams. Analytical solutions for free vibration and buckling of composite beams using a higher order beam theory presented by He G. et al. [6]. Vibration prediction of thin-walled composite I-beams using scaled models analyzed by M. E. Asl et al. [7]. Within that study, which is an extension of Authors’ previous work on design of scaled composite models [8-10], similitude theory is applied to the governing equations of motion for vibration of a thin walled composite I-beam. Algarray et al. [11] studied the effects of end conditions of Cross-Ply laminated composite beams on their dimensionless natural frequencies

2. Modeling analysis

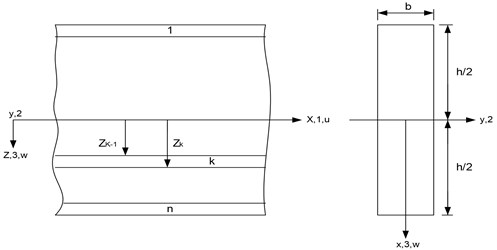
Fig. 1. Showed a composite laminated beam made up of $n$ layers with different orientation, thickness, and properties. Where $L$ is the length, b is breadth and $h$ is depth.

Fig. 1Composite laminated beam

Treat the beam as a plane stress problem and employ first-order shear deformation theory. The longitudinal displacement ($U$) and the lateral displacement ($W$) can be written as follows:

where $u$ and $w$ are the mid-plane longitudinal and lateral displacements, $\varphi$ is the rotation of the deformed section about the $y$-axis, $z$ is the perpendicular distance from mid-plane to the layer plane, and $t$ is time.

The Strain-Displacement Relations:

where: ${\epsilon }_1$ is the longitudinal strain, and ${\epsilon }_5$ is the through-thickness shear strain.

Fig. 2Composite laminated beam with 3-noded lineal element

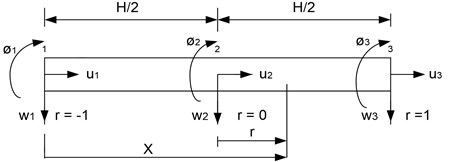
By employing 3-noded lineal element as shown in Fig. 2.

The displacements can be expressed in terms of shape function $N_i$ and nodal displacements:

The shape functions are: $N_1=-\frac{r}{2}\left(1-r\right)$, $N_2=1-r^2$, $N_3=\frac{r}{2}\left(1+r\right)$.

From Eqs. (2), (3), the strains can be written as:

where:

And $a^e$ is the vector of nodal displacements $a^e={\left[\begin{array}{ccc}{u}_i& w_i& {\varnothing }_i\end{array}\right]}^T$, $i=$ 1, 2, 3.

The stress-strain relation:

where $\sigma ={\left[\begin{array}{cc}{\sigma }_1& {\sigma }_5\end{array}\right]}^T$, $ϵ={\left[\begin{array}{cc}{ϵ}_1& {ϵ}_5\end{array}\right]}^T$ and the matrix containing the transformed elastic constants:

Substitute Eq. (4) in Eq. (5):

The strain energy:

where:

The kinetic energy:

where $\rho$is density and the dot denotes differentiation with time:

where:

In the above derivation it is assumed the motion is harmonic and $\omega$ is circular frequency.

In the absence of damping and external nodal load, the total energy is:

The principle of minimum energy requires that:

The condition yields the equation of motion:

where:

and $n$ is number of elements. To facilitate the solution of Eq. (10), we introduce the following quantities:

where $K_f$ is the shear correction factor.

The transformed elastic constants are:

In which:

And $\theta$ is the angle of orientation of the ply with respect to the beam axis:

Non-dimensional quantities used in the analysis are:

The element stiffness matrix:

The mass matrix is 9×9 symmetrical matrix:

3. Results and discussion

3.2. Effect of axial movements of the ends

From the results of Table 1, that the values of the non-dimensional frequencies of the transverse modes are not affected by the longitudinal movements of the ends since these modes are generated by lateral movements only (at the yellow shaded). However, the values of the natural frequencies of longitudinal modes are found to be the same for all beams with movable ends since they are generated by longitudinal movements only. Table 3 shows this observation for symmetric [60/–60/–60/60] laminated beams with aspect ratio of 10.

Fig. 3Effect of aspect ratio on natural frequencies of a symmetric [45/–45/–45/45] cross-play clamped-free beam

Fig. 4Effect of aspect ratio on natural frequencies of a symmetric [45/–45/–45/45] cross-play clamped-clamped beam

Fig. 5Effect of aspect ratio on natural frequencies of a symmetric [45/–45/–45/45] cross-play free-free beam

Table 1 also shows the fundamental modes of longitudinal vibration for various beams with immovable ends for the symmetric case [30/–30/–30/30] and for two aspect ratios, 10, and 50. It could be noticed that the values of non-dimensional natural frequencies of the longitudinal vibration for the clamped-free and hinged-free beams are equal, and those of the other beams are also the same. This phenomenon occurs since both clamped-free and hinged-free beams with immovable ends are the same when restricted from executing longitudinal motion at the ends. Similarly, the rest of beams with immovable ends have the same longitudinal end conditions.

Table 3The first two non-dimensional modes of longitudinal free vibration of [60/–60/–60/60] laminated beams with aspect ratio 10

Beam ends	Mode No.	Beam type
		CF	HH	CC	HC	HF	FF
Immovable	1	5.6426	11.2852	11.2852	11.2852	5.6426	11.2852
	2	16.9279	22.5705	22.5705	22.5705	16.9279	22.5705
Movable	1	11.2852	11.2852	11.2852	11.2852	11.2852	11.2852
	2	22.5705	22.5705	22.5705	22.5705	22.5705	22.5705

4. Conclusions

In this paper, free vibration of four layered composite beams has been studied. Both secondary effects of transverse shear deformation and rotary inertia were included in the analysis. A first-order shear deformation theory was applied in the analysis. A finite element model has been formulated to predict the non-dimensional natural frequencies and to study the influence of aspect ratio and movable ends of fibers on the natural frequencies. Different end conditions were studied which are clamped-free, hinged-hinged, clamped-clamped, hinged-clamped, hinged-free, and free-free beams with immovable and movable ends. The main conclusion is the natural frequencies of a laminated beam generally increase with the aspect ratio and all beams with movable ends have equal longitudinal frequencies of vibration, while those of beams with immovable ends are different. Namely, clamped-free and hinged-free beams with immovable ends have equal longitudinal frequencies, and the other beams have also equal longitudinal frequencies.