High performance estimations of natural frequency of basalt FRP laminated plates with intermediate elastic support using response surfaces method

1. Introduction

The classical and non-classical plates (i.e. Continuous and with intermediate stiffeners plates) are most common in a wide range of engineering fields such as aerospace, structures, vehicles and marine industries. In general, the approximate [1-10] or numerical [11-14] method must be used to compute the natural frequencies and find mode shapes for different boundary conditions for plate ends.

The author has investigated applicability of the FSTM method through a semi-analytical; natural frequency was estimated for basalt FRP laminated, variable thickness plates [15] and with intermediate elastic support. However, a large error of estimation remains due to a lot of computational processes must be performed for estimation of natural frequency. This is the main drawback of the FSTM method. Author also has already applied a predictive model for natural frequency in terms of fiber orientations is then developed using artificial neural networks (ANNs) to improve the estimations performance of FSTM by decreasing the effort to obtain frequency parameters in order to treatment the main drawback of the FSTM method [16]. The developed model via ANNs is trained and tested with a number of the FSTM results then used this predictive model for predicting non- FSTM data not included in FSTM evaluation. An ANNs model is shown to be very effective for predicting the natural frequency of basalt FRP laminated, variable thickness plates with intermediate elastic support.

In light of those difficulties, the present study uses RS method of various elastic restraint coefficients ($K_T$) of basalt FRP laminated, variable thickness plates with intermediate elastic support (Fig. 1) to investigate effect of intermediate elastic support on the natural frequencies; furthermore, an improvement to obtain higher estimation performance is proposed for the FSTM method before making response surfaces. This new method is applied to estimate natural frequencies of plate type under four different ends of the plate; performance of the new estimation method is evaluated analytically herein.

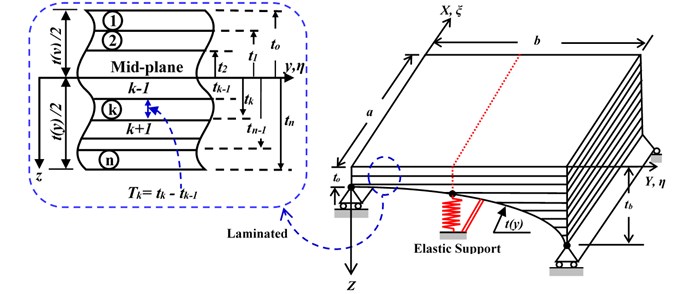
Fig. 1The geometrical model of Basalt FRP laminated variable thickness rectangular plate with intermediate elastic support

2. Governing equations

The formula that governing the dynamics of presented plate model in this study using the classical deformation theory in terms of the plate deflection ${\delta }_o(x,y,\tau )$ after normalized to non-Dimensional variables $\xi$ and $\eta$ can be written as: [15, 17]:

where: $\beta =a/b$ is the aspect ratio, and:

$\rho$ is the material density, $D_{ij}$ is the flexural rigidities matrix are present by:

where $t_{ok}$ as shown in Fig. 1 is measuring between the middle-plane approbating to $t_o$ to the layer bottom $t_{oth}$. And $\overline{q_{ij}^k}$ are the plane stress transformed reduced stiffness coefficients, they can be computed in terms of the longitudinal and transverse Young’s moduli $E_{11}$, $E_{22}$, plane shear modulus of elasticity $G_{12}$ and Poisson coefficients ${\upsilon }_{12}$ and ${\upsilon }_{21}$ as:

2.1. Boundary conditions

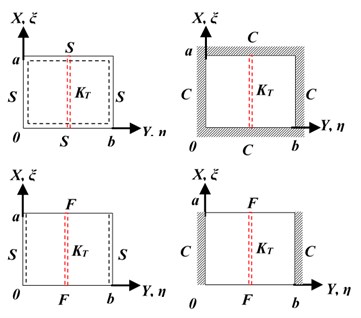
In this study, author applied new method to estimate natural frequencies of plate type under four different classical boundary conditions at the ends of the plate are SSSS, CCCC, SSFF and CCFF (see Fig. 2).

Since the treatment of the intermediate elastic line support conditions are the goal of the present study we can see in Fig. 1, the intermediate elastic line support, $y=b/2$ , the solution of the governing must be continuous at this line, i.e. [2]:

4

$\frac{1}{b}{\left.\frac{\partial {\delta }_o}{\partial \eta }\right|}_{\eta =1^{-}/2}=\frac{1}{b}{\left.\frac{\partial {\delta }_o}{\partial \eta }\right|}_{\eta =1^{+}/2}.$

Fig. 2Schematic representation of different plate ends

3. Finite strip transition matrix (FSTM) with response surfaces (RS) method

3.2. Response surface (RS) method

The RS is more dependent tool for quality engineering areas [18, 19]. The methodology of the RS have two benefits, the approximately of the inverse problems can be solved without take in your account the engineering modeling, and the approximately results of RS can be estimated using powerful statistical tools. In this estimation work, the methodology of the RS was applied to predict the $\mathrm{\Omega }$ from Eigen function. For widly applications of the RS, the functions for the approximately of the inverse problems are polynomials, in order to simplify. For the cases of quadratic polynomials, the RS is addressed as follow:

6

$y={\beta }_0+{\sum }_{j=1}^k{\beta }_j{x}_j+{\sum }_{j=1}^k{\beta }_{jj}{x_j}^2+{\sum }_{i=1}^{k-1}{\sum }_{j=i+1}^k{\beta }_{ij}{x}_i{x}_j,$

where: $k$ is the number of strips. In this case, there are $N$ discrete longitudinal strips spanning (beam strips), $y$ is the estimation results of the $\mathrm{\Omega }$ by RS method and the coefficients $\beta$ are obtained with the least square errors method [18, 19]. The estimations of the $\mathrm{\Omega }$ by RS function in Eq. (6) is expressed as follow:

7

$\mathrm{\Omega }={\beta }_0+{\sum }_{j=1}^N{\beta }_j{S}_j+{\sum }_{j=1}^N{\beta }_{jj}{S_j}^2+{\sum }_{i=1}^{N-1}{\sum }_{j=i+1}^N{\beta }_{ij}{S}_i{S}_j,$

where: $S_i$, $S_j$ are a standard eigenvalue can be obtained from the shape function $W(\xi ,\eta ,t)$ by FSTM, $i=$ 1, 2, 3,…, $N$, $j=$1, 2, 3,…, $M.$

Fig. 3FSTM model on plate

In the case that varies $\mathrm{\Omega }$, the total number of parameters is $n$, the RS can be computed as follows using matrix expression:

8

$Y=X\beta +e,$

where:

$Y=\left\{\begin{array}{l}{y}_1\\ y_2\\ ⋮\\ y_n\end{array}\right\},X=\left[\begin{array}{lllll}1& x_{11}& x_{12}& \dots & x_{1k}\\ 1& x_{21}& x_{22}& \dots & x_{2k}\\ ⋮& ⋮& ⋮& \ddots & ⋮\\ 1& x_{n1}& x_{n2}& \dots & x_{nk}\end{array}\right],\beta =\left\{\begin{array}{l}{\beta }_0\\ {\beta }_1\\ ⋮\\ {\beta }_k\end{array}\right\},e=\left\{\begin{array}{l}{e}_1\\ e_2\\ ⋮\\ e_n\end{array}\right\},$

where: $e$ is an error vector. The unbiased estimator $b$ of the coefficient vector $\beta$ is obtained using the well-known least square error method as follows:

9

$b={\left(X^{T}X\right)}^{-1}+X^{T}Y.$

The variance-covariance matrix of the $b$ is obtained as follows:

10

$Cov\left(b_i,b_j\right)={\sigma }^2{\left(X^{T}X\right)}^{-1},$

where: the $\sigma$ is the error of $Y$. The estimated value of $\sigma$ is obtained as follows:

11

${\sigma }^2=\frac{S{S}_E}{n-k-1}.$

$S{S}_E$ is a square sum of errors, and expressed as follows:

12

$S{S}_E=Y^{T}Y-b^T{X}^{T}Y.$

In order to judge the goodness of the approximation of the RS, the adjusted coefficient of multiple determination $R_{adj}^2$ is used:

13

$R_{adj}^2=1-\left(\frac{S{S}_E}{\left(n-k-1\right)}/\frac{S_{yy}}{\left(n-1\right)}\right),$

where: $S_{yy}$ is the total sum of squares:

14

$S_{yy}=Y^{T}Y-\left({\left({\sum }_{i=1}^n{y}_i\right)}^2/n\right).$

The value of $R_{adj}^2$ is equal to or lower than 1.0. A higher value of $R_{adj}^2$ implies a better fit. When the RS shows a very good fit, $R_{adj}^2$ approaches 1.0. A good fit of the RS means that the RS gives good estimations for the FSTM technique used for the regression. Lower $R_{adj}^2$ values means poorer estimations and the error band of the estimated result is wider.

4. Results and discussion

In this part, the present method is used to estimate the natural frequency of the plate was presented in Fig. 1. The intermediate elastic line support at one direction and we can be expressed as follow, $K_T=T_{b/2}{b}^3/D_{22}$, $T$ is translational stiffness. The plate model was designed of five symmetrically, angle-ply, laminates with the fiber angles $\left[\theta /-\theta /\theta /-\theta /\theta \right]$ is [45°/–45°/45º/–45°/45°] and has linear variable thickness, $\delta \left(y\right)$ as form $\delta \left(\eta \right)=1+\mathrm{\Delta }\eta$, $\mathrm{\Delta }={\delta }_b-{\delta }_o/{\delta }_o$ is the tapered ratio (see Fig. 1). The physical and mechanical properties of the basalt FRP laminate composite plate are shown in Table 1. The frequency parameter $\mathrm{\Omega }$ is evaluated in non-dimensional form, expressed as: $\mathrm{\Omega }={\left(m_{o}t\left(\eta \right){\omega }^2{a}^4/t_o{D}_{22}\right)}^{1/2}$.

4.2. The proposed technique results

Fig. 4 presents the amount of changes of the natural frequencies due to intermediate elastic support of laminated, the plate has the aspect ratio $\beta =$0.5 and tapered ratio $\mathrm{\Delta }=$ 0.5. As shown in the figure, for each frequency of six frequencies, we can observe the increases in the frequency with the increasing of the value of elastic restraint coefficient ($K_T$)

Fig. 5 presents changes of the vibration behavior due to variation in the types of the plate ends (SSSS, CCCC, SSFF and CCFF). As shown in the figure, we can see the variation of the frequencies values, where, the plate with ends of fully clamped (CCCC) and semi-simply supported (SSFF) have been recorded the higher and lower values of the frequencies respectively, and the other types of the plate ends (SSSS and CCFF) are located in intermediate.

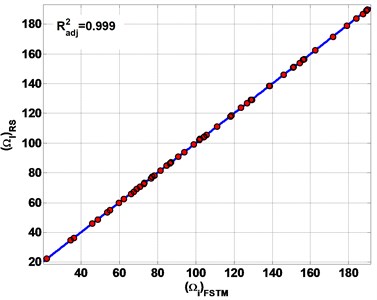
Fig. 6 represents the estimation results of the first six frequencies by RS. The $R_{adj}^2$ of estimation results are 0.999, 0.996, 0.987 and 0.964 for SSSS, CCCC, SSFF and CCFF plate respectively.

Fig. 4The relation between non-dimensional frequencies parameter (Ω) and elastic restraint coefficient (KT)

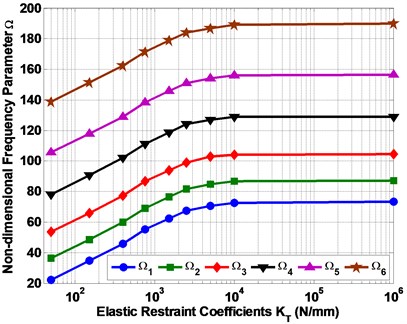
Fig. 5The effect of the variation boundary conditions on non-dimensional frequencies parameter (Ω)

All of the estimation results are plotted on the diagonal line (Fig. 6) to observe the performances of the present RS method to estimate non-dimensional frequencies ($\mathrm{\Omega }$). The error band is defined as the maximum error of the estimated $\mathrm{\Omega }$. The error band from the diagonal line is less than 0.01 for all types of boundary conditions. The results of the estimated $\mathrm{\Omega }$ is presented in Table 3. As a result, the RS gave good estimations for FSTM to calculate $\mathrm{\Omega }$ in composite plate.

Table 3The first six frequencies of Basalt FRP rectangular plate with intermediate elastic line support

	$K_T$	${\mathrm{\Omega }}_1$	${\mathrm{\Omega }}_2$	${\mathrm{\Omega }}_3$	${\mathrm{\Omega }}_4$	${\mathrm{\Omega }}_5$	${\mathrm{\Omega }}_6$	$R_{adj}^2$
SSSS	50	22.2	36.2	53.6	78.2	105.6	138.7	0.999
	150	34.7	48.7	66	90.8	118	151.3	0.999
	400	45.9	59.9	77.3	102	129	162.4	0.999
	750	55.1	69.2	86.6	111.2	138.5	171.6	1.0
	1500	62.5	76.5	94	118.6	146	179	1.0
	2500	67.5	81.6	99	124	151	184	1.0
	5000	70.8	84.9	103	127	154	187	1.0
	10000	72.7	86.8	104	129	156.2	189	1.0
	1E+06	73.2	87.2	104.6	129	156.6	190	1.0
CCCC	50	28.5	46.5	68.9	100.5	135.6	178	0.988
	150	41	59	81.4	113	148	190.6	0.992
	400	52	70	92.5	124	160	201	0.995
	750	61.4	79.4	101.7	133.4	168.5	212	0.998
	1500	69	87	110	141	176	218.5	0.999
	2500	74	92	114	146	181	223.6	0.999
	5000	77	95	117.6	149	184.3	227	1.0
	10000	79	97	119.4	151	186.2	229	1.0
	1E+06	79.5	97.5	120	151.5	187	229.4	1.0
SSFF	50	12.3	20.1	29.7	43.4	58.5	77	0.967
	150	25	32.6	43	56	71	89.5	0.973
	400	36	43.8	53.5	67	82.5	100.7	0.979
	750	45.2	53.1	62.6	76.3	91.4	109.8	0.987
	1500	53	60.5	70	83.8	99	117	0.991
	2500	58	65.7	75	89	104	122	0.994
	5000	61	69	78.6	92	107.6	125.6	0.997
	10000	63	71	80	94	109	127.7	0.999
	1E+06	63.5	71.4	81	94.8	109.5	128	1.0
CCFF	50	19.6	32	47.4	69.2	93.4	122.7	0.899
	150	32	44.8	60	82	106	135.4	0.926
	400	43.4	55.7	71	93	117	146.8	0.94
	750	52.5	65	80.4	102.2	126.4	155.7	0.958
	1500	60	72.7	88	110	133.8	163	0.972
	2500	65	77.9	93	114.8	139	168	0.989
	5000	68.6	81	96	118	142	171.7	0.999
	10000	70	83	98	120	144	173.5	1.0
	1E+06	70.6	83.4	98.7	121	144.6	174	1.0

Fig. 6The estimation results of non-dimensional frequency parameter Ω

a) SSSS

b) CCCC

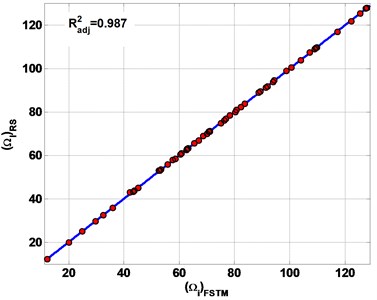
c) SSFF

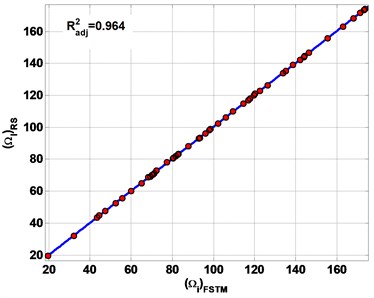
d) CCFF

5. Conclusions

In the present study, the FSTM with RS approach is applied. The method has successfully estimation of the natural frequency ($\mathrm{\Omega }$) in one step of computing using the FSTM output of standard eigenvalue, in order to eliminate the iteration that implemented in FSTM algorithm to compute the natural frequency and decrease the error of estimation. Based on the FSTM results and the results estimated by RS, the following conclusions are drawn for basalt FRP laminated variable thickness rectangular plates with intermediate elastic support.

1) The RS data are provided excellent estimations of the natural frequency of basalt FRP plates for each case of boundary conditions and different $K_T$.

2) The increases in the frequency with the increasing of the value of elastic restraint coefficient ($K_T$) is observed.

3) On other hand, the variation of the frequencies values, where, the plate with ends of fully clamped (CCCC) and semi-simply supported (SSFF) have been recorded the higher and lower values of the frequencies respectively, and the other types of the plate ends (SSSS and CCFF) are located in intermediate.

4) The RS technique could estimate $\mathrm{\Omega }$ with high adjusted coefficient $R_{adj}^2$ for $K_T=$50 are 0.999, 0.988, 0.967 and 0.899 for SSSS, CCCC, SSFF and CCFF plate respectively, for $K_T=$1E+06 are 1.0 for each boundary conditions.

5) It is observed that the adjusted coefficient $R_{adj}^2$ increases with increasing values of $K_T$ of intermediate elastic support.

6) The boundary condition SSSS has the highest performance of estimate $\mathrm{\Omega }$, the boundary condition CCFF has the lowest performance of estimate $\mathrm{\Omega }$, while the other boundary conditions are laid in between, with descending order of CCCC and SSFF respectively.

7) As a result, the method reveals excellent performance of estimations of natural frequencies.

8) Finally, author have revealed that the response surfaces are better than artificial neural networks for this inverse problem.