Numerical calculation of wing-bending moment with real-time strain monitoring by FBG modulation

3.1. Experiment

The experiment is carried out on the basis of the full-scale fatigue test. There are a large number of loading points and support points distributed on the whole aircraft to ensure simulated flight conditions and maintain aircraft balance [11]. Simultaneously, the whole aircraft is covered with a wide variety of sensors for monitoring various parameters for aircraft evaluation, and for feedback and control of load states.

The method of this experiment is able to imitate a great deal of flight conditions and flight postures, including the excursion of the wing's center of pressure, etc. When analyzing the load and strain conditions of the wing, the wing root can be seen as fixed support to the fuselage. The wings are mainly subjected to the bending moment, torque and shear force, both in actual flight and in the simulated conditions of this test. All loading forces applied to the wing can be simplified as a center of pressure load on the aircraft wing. The bending moment, the torque and the shear force on the wing can be considered to be caused by the center of pressure load on the aircraft wing which is simplified by the actual load force on the wing. As shown in Fig. 1, the bending moment ($M$) is the moment that points to aircraft heading along the rigid axis of the wing; the torque ($T$) is the moment revolve round the rigid axis of the wing; the shear force ($Q$) is inner force that the line of action is located at the cross section of the wing.

Fig. 1The bending moment, the torque and the shear force

In order to measure the bending moment of the wing, five fiber Bragg grating sensors are distributed on the rigid axle position of the lower wing. The five fiber grating sensors are distributed on a single fiber, and kept away from the stress concentration on the wing. Simultaneously, the last FBG sensor near the wingtips should appear in the circle of the change of the center of pressure according to the analysis of the wing's center of pressure. The five sensors, as shown in Fig. 2, divide the wing into six sections. This paper assumes that each of these “sections”, which are defined herein as the area from one adjacent fiber optic sensor to another, has a consistent structural behavior within each section, but the structural behavior may be different in different sections. For temperature compensation, there is several temperature FBG sensors distributed on the wing. These FBG temperature sensors are distributed at areas where strain can be neglected on the wing, used to correct the influence of temperature on the calculated strain results.

Fig. 2FBG sensors for strain monitoring on the wing

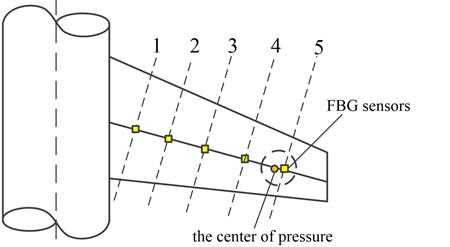
The fiber grating sensors sampling frequency in this experiment is 200 Hz. The center wavelengths of the fiber grating are filtered. The curve of the center wavelength of fiber grating vary with time are obtained, and the wave crest and trough value are taken to study and for strain calculation. The real-time strains at the location of the sensors are calculated by Eq. (5), and the coefficients $K_T$ and $K_{\epsilon }$ in this equation are obtained in advance by the calibration test for the optical fiber used in this experiment.

3.2. Correlation between strain and bending moment

Five strains of the cross section are obtained by the five sensors which divided the wing into six sections. In the actual engineering application, the local cross section bending moment has an approximate proportional relationship with the strain of the local cross section:

where $M_i$ is the bending moment of the local cross section $i$, ${\epsilon }_i$ is the strain of the local cross section $i$, and $K_i$ is the coefficient of proportion relation between bending moment and strain of the local cross section $i$.

When analyzing the load, bending moment and strain of the wing, the wing root can be considered as fixed support to the fuselage. And the wing is regarded as a cantilever beam structure with a single point of force at the end. As shown in Fig. 3, studying from a one-dimensional state, the shear force on the wing is equal to the constant amount of the force on center of pressure, and the bending moment is a diagonal line.

Fig. 3Simplified wing model

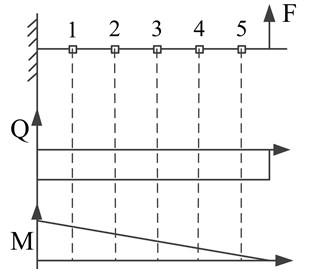
On account of the bending moment is a diagonal line, there is a proportional relationship between the bending moments of each cross section and the strains of each cross section:

where $x$ is the distance between the center of pressure and the location of the fifth sensor, and $a_i$ is the distance between the location of $i$th sensor and the location of the fifth sensor.

Only with the strain data of five sensors, it is not able to get the solution of Eq. (7). One of the conditions in this experiment is the excursion of the wing's center of pressure. When the wing’s center of pressure moves proximately to the location of the fifth sensor, the strain measured at fifth sensor is close to 0, and the formula of the proportional relationship between the bending moments of each cross section and the strains of each cross section transforms into:

For the distance between the location of $i$th sensor and the location of the fifth sensor $a_i$ is known, the coefficients $K_i$ can be solved by the Eq. (8). The result of the solution can be expressed as:

Accordingly, the real-time bending moments of the wing are able to be calculated by the strain measured in real time by Eq. (9).