Solutions of active vibration suppression associated with web structures on face gear drives

1. Introduction

Face gear dynamics is focused by many scholars, due to operating conditions of first-stage gear drives in helicopter main gear boxes. There are a vast of lectures discussing dynamic behaviors of face gear drives in the past few years [1-8]. However, the solutions of active vibration suppression on face gear drives are not constructed. Thus, in the study, a solution of active vibration suppression associated with web structures on face gear drives is proposed, and dynamic behaviors of an example case of face gear drives associated with the proposed solution are simulated. The results indicate the fidelity of the proposed solution of active vibration suppression of face gear drives could be accepted. These contributions would be helpful to improve vibration suppression studies of face gear drives in the future.

2. Web structures

Based on a viewpoint, namely, the rotation of the wheel body being an important factor on flexibilities of gear teeth [9], namely, mesh stiffness and STE (Static Transmission Error) being affected by gear wheel body flexibility sharply, a solution of active vibration suppression associated with web structures on face gear drives, as shown in Fig. 1, is proposed.

Fig. 1The proposed vibration active control solution

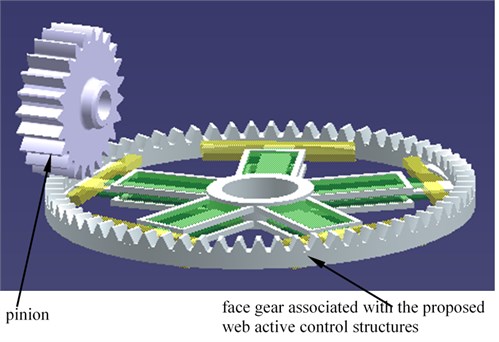
a) A face gear drive associated with web active control structures

b) The local view of web active control structures

As illustrated in Fig. 1, a face gear web could be divided into two version layers. one is an actuating layer, such as the first and the third layers, namely, the layer associated with actuators and not contacted with rims at initial states, the other is a fixed layer, such as the second layer, meaning, the layer without actuators and contacted with rims at initial states.

3. Dynamic model

In order to assess effects of the proposed solution on face gear dynamic behaviors, employed the lumped-mass method, a four DOFs dynamic model of face gear drives, as shown in Fig. 2, is established, and the active control is introduced into the dynamic model by STE.

Fig. 2A four DOF dynamic model of face gear drives

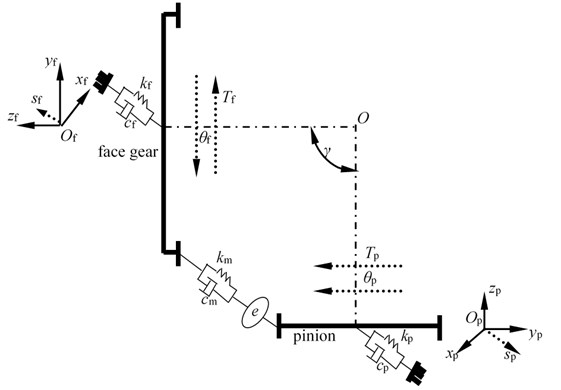
As illustrated in Fig. 2, $\theta$ is a torsion DOF, $s$ is a bending DOF. $T$ is a torsion, $k$ is a bending stiffness, $c$ is a bending damping, subscript $f$ and $p$ express face gears and pinions, respectively, $k_m$ is a mesh stiffness of face gear drives, $c_m$ is a mesh damping of face gear drives, $\gamma$ is a shaft angle of face gear drives, and $e$ is a STE, which could be defined as:

where ${\delta }_t$ is a error caused by complex tooth deformations, and ${\delta }_w$ is a error caused by rotation deformations of wheel bodies of face gear drives.

Based on Fig. 2, the mathematic equations of the dynamic model could be derived by:

where $m$ is a quality, $R_b$ is a radius of basic circles, $I$is a moment of inertia, and $F_m$ could be deduced as:

4. Simulation and analysis

In order to assess effects of the proposed active vibration suppression on dynamic behaviors of face gear drives, parameters of an example case of face gear drives associated with web structures are listed in Table 1.

According to Table 1, Eqs. (1-3) and two version active control methods, namely, STE key point active control (the first version) and STE procession active control (the second version), the dynamic behaviors and the dynamic mesh forces of the example case are simulated, as shown in Fig. 3 and Fig. 4, respectively.

In Fig. 3, compared Fig. 3(a) with Fig. 3(b), the forms are similar, the amplitudes are reduced, and the escape quantities of the small rings are reduced. Meanwhile, compared Fig. 3(a) with Fig. 3(c), the forms are not similar, due to the added rings in the big cycles, the amplitudes are still reduced, and the escape quantities of the small rings are increased.

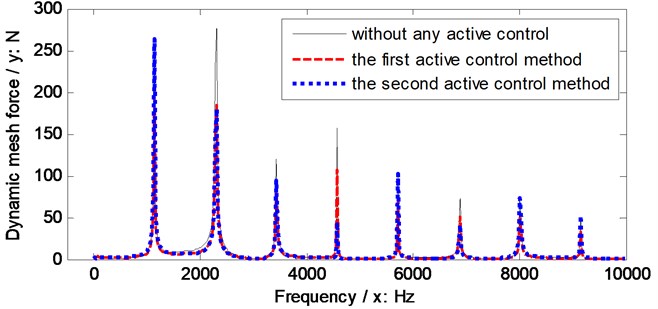
As illustrated in Fig. 4, after the first version active control method, the spectral distribution form of the dynamic mesh force is not changed, and the amplitudes versus frequencies are almost reduced. While, after the second version active control method, the spectral distribution form of the dynamic mesh force is changed.

Fig. 3Dynamic behavior simulations of the example case

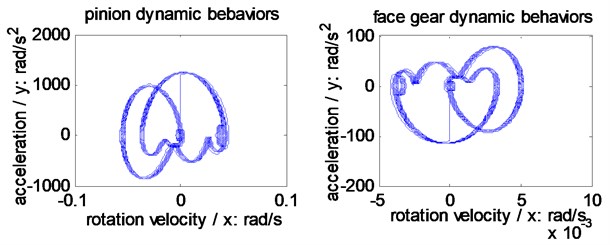
a) Without any active control

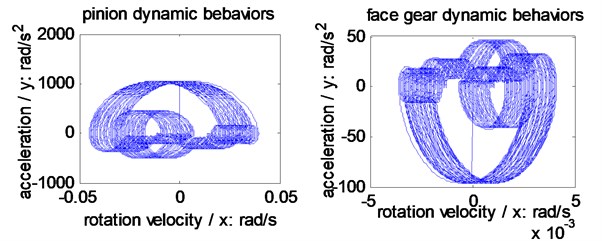
b) The first active control method

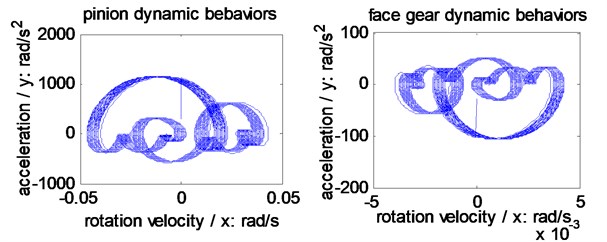
c) The second active control method

Fig. 4Dynamic mesh force simulations of the example case

5. Conclusions

In the study, two works can be extracted as follows:

1) A solution of active vibration suppression associated with web structures on face gear drives is proposed.

2) Vibration suppression effects of face gear drives are significant by the proposed solution.

These contributions would be helpful to improve vibration suppression studies of face gear drives in the future.