Abstract
The thermo-elastic vibration response of simple supported axially moving Euler beam is investigated. The differential equation of moving beam is established by recourse to Hamilton principle and the thermal effects is considered by introducing the equivalent thermal bending moment. A 2-D transient temperature field is calculated by the alternating-directional implicit (ADI) method and the equivalent thermal moment is calculated numerically. The dimensionless equation is discretized by Galerkin method and the modal analysis of gyroscopic system is used to calculate the forced vibration response. The time-history curve of the beam’s upper middle point is obtained for thermal or non-thermal situations.
1. Introduction
In the field of engineering applications, many structures are typical axially moving systems, such as aerial cable tramways, band saws and power transmission chains. Mote [1] studied the band saw vibrations by regarding it as an axially moving beam and the frequency curves were obtained. Simpson [2] analyzed the natural frequencies and mode curves of the axially moving beam with clamped boundary, his calculated results show that modes distorted violently as the moving speed increases. Moreover, Wickert [3] brought forth that axially moving beam belongs to gyroscopic system and researched the forced vibration of axially moving beam by modal analysis and Green’s function method. The above articles conducted their investigations on the vibration performance and response of the axially moving beam under a certain uniform temperature field. However, in the actual situations, many structures are operated in transient thermal environment, and thus the thermal effect must be considered. Guo [4] considered the coupled thermo-elastic vibration characteristics of the axially moving beam, and acquired the curves of complex frequencies versus the axially moving speed. Wang and Li [5] computed the thermo-elastic responses of axially moving viscoelastic sandwich beams using pseudo-normal modes method, and found that the thermal impact has large influence on the beam’s response. However, in the above investigations the vibration responses were mostly calculated under assumed non-constant temperature fields directly, while the others did not mention the specified algorithms they used.
As the problem involves two physical fields, it is important to find an appropriate method to ensure the problem being solved in a reasonable computational cost. In this paper, the temperature fields are calculated firstly by the ADI method, then the superposition method is used to calculate the vibration response.
2. The basic equations
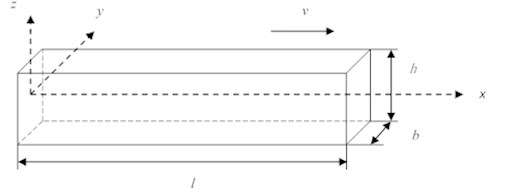
An axially moving beam with uniform cross-section is considered as shown in Fig. 1. The thermal boundaries are: the upper one suddenly getting a certain temperature rise and remains unchanged, while the others remain their original temperatures unchanged. Consider the beam of density ρ, length l, width b, height h, cross-section area A, moment of inertial I, tension R, Young’s modulus E, and coefficient of thermal expansion αT. The beam travels at a uniform constant velocity v between two boundaries. The out-plane motion of the beam is specified by the transverse displacement w(x,t). B and ω are the amplitude and frequency of the external excitation respectively. By inserting the thermal moment MT into the equation derived by Mote [1], we attain the differential equation:
for the simple supports boundary conditions:
where MT(x,t)=b∫h/2-h/2-EαT(T-T0)zdz and T0 is the initial temperature.
Fig. 1Schematic of an axially moving beam
Introducing the dimensionless variables and parameters as follow:
p20=REA,α=h212l2,β=lEA,Fb=BlEA,
where c2=E/ρ is the velocity of the longitudinal wave. Omitting the bars in the dimensionless variables, Eq. (1) can be transformed into dimensionless equation as:
When using the Galerkin method to discretize the equation, the displacement function can be expanded as:
Introducing Eq. (5) into Eq. (4) and moving the thermal terms into right part, the equation becomes:
where:
wT=(((p20-v2)(kπ)2+α(kπ)4)sin(kπx))T.
Multiplying the two sides of Eq. (6) by w and integrating the equation in the dimensionless range [0, 1], let Nf=4, then we have:
where F(t)=Fbsin(ωt), G(x,t)=12EbαTT0αβ∂2χθ/∂x2, and M, K is diagonal matrix (symmetry matrix) and C is skew-symmetry matrix:
Introducing the state variables and general load vectors x=(˙qq), Gf=(2∫10→w(F+G)dx0)T, Eq. (7) can be arranged as:
where I=(M00K), G=(CK-K0). Eq. (9) is a standard form of the gyroscopic system, so it can be solved by the modal superposition method.
According to the research of Meirovitch [6, 7], the solution to the eigenvalue problems:
consists of n pairs of pure imaginary complex conjugates λr=±iωr and n pairs of complex conjugate eigenvectors xr and ¯xr(r=1,2...n). Let yr and zr be the real part and imaginary part of xr and any arbitrary 2n-dimensional vector v can be expressed as a linear combination form:
For the load vector Gf(t), the corresponding general coordinates are:
+yrTIx(0)cosωrt+zrTIx(0)sinωrt,
+zrTIx(0)cosωrt-yrTIx(0)sinωrt.
3. Heat transfer model
For calculating the thermal load in right side, the 2D temperature field of the structure must be solved. The differential equation can be written as the following dimensionless form:
where a=k/cp, b1=a/cl, b2=al/ch2, k represents the thermal conductivity and cp the volume heat capacity. The thermal boundaries are written as:
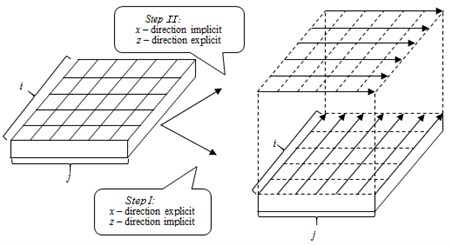
The alternating-direction implicit method [8, 9] is an effective approach in solving the heat-transfer problems which divides each iteration step into two steps for applying implicit format in x and z directions respectively, as shown in Fig. 2. Thus, the equation in each step can be transformed into a tri-diagonal form matrix which can be solved by Thomas algorithm efficiently.
At the first step, applying implicit format in z direction and explicit format in x direction, we have:
then, applying formulation in the contrast directions, we have:
where Sx=b1dt/(dx)2, Sz=b2dt/(dz)2. According to the equations above, the temperature field in any time can be calculated. After numerical integrating and differentiating, χθ and ∂2χθ/∂x2 can be attained. Therefore, the thermal moment will be evaluated.
Fig. 2Illustration of the ADI method
4. Numerical examples
Consider a beam with modulus of elasticity E= 7.0×1011 Pa, density ρ= 2778 kg/m3. Let the length l= 0.5 m, the width b= 0.05 m, the height h= 0.05 m, the axial speed v=c/100, the initial tension R= 6000 N, the force amplitude B= 500 N, the heat conductivity k= 207 W/(m2·K), the specific heat cp= 2484000 J/(m3·K) and the coefficient of thermal expansion αT= 2.39×10-5 m/K.
The whole real-time response will be solved by dividing the heating process into short time-steps and the thermal load can be calculated numerically. At each step, assuming ∂2χθ/∂x2 is constant, the load can be written as:
where X1(t)=2F(2/π,0,2/3π,0)T and Xc is constant vector.
In each time step period [ti,ti+1], the general coordinates ξ(ti+1), η(ti+1) can be written as:
-Fbωrω2-ω2rcos(iωh)sin(ωrh))+2zrTc(-Fbωrω2-ω2rcos((i+1)ωh)
+Fbωrω2-ω2rcos(iωh)cos(ωrh)-Fbωω2-ω2rsin(iωh)sin(ωrh))
+yrTXcsin(ωrh)+zrTXc(1-cos(ωrh))+yrTIx(0)cos(ωrh)+zrTIx(0)sin(ωrh),
ηr(ti+1)=2zrTc(Fbωω2-ω2rsin((i+1)ωh)-Fbωω2-ω2rsin(iωh)cos(ωrh)
-Fbωrω2-ω2rcos(iωh)sin(ωrh))-2yrTc(-Fbωrω2-ω2rcos((i+1)ωh)
+Fbωω2-ω2rcos(iωh)cos(ωrh)-Fbωω2-ω2rsin(iωh)sin(ωrh))
+zrXcsin(ωrh)-yrTXc_(1-cos(ωrh))+zrIx(0)cos(ωrh)-yrTIx(0)sin(ωrh)
where c=(2π023π00000)T.
First, the eigenvalue problem can be solved and the frequencies of the system are presented as in Table 1.
Then, the temperature field can be drawn in Fig. 3.
Fig. 3The transient temperature field in a) 200 and b) 400 dimensionless time
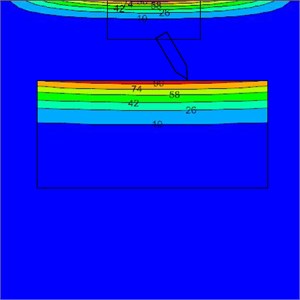
a)
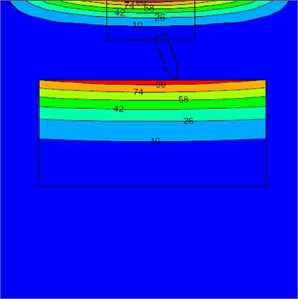
b)
For the thermo-elastic issue, the time-region response under mechanical and thermal loads can be solved as follow. The beating phenomenon appears because the frequency of load is near the fundamental frequency of the system. Fig. 4(a) shows that the deflection at middle point presents the beating phenomenon indeed when the temperature is a constant, which shows the validity of the present numerical method. Fig. 4(b) is the result, when a sudden rise of temperature is applied on the upper boundary, which shows a large additional deflection. When the temperature condition disappears, the equilibrium position returns to the original straight line.
Fig. 4Displacement in middle point for non-thermal and thermal situations
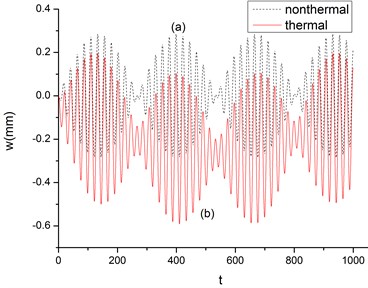
Table 1The first four order frequencies of axially moving beam
Orders | First | Second | Third | Fourth |
Dimensionless frequencies | 0.2833 | 1.1387 | 2.5635 | 4.5604 |
5. Conclusions
The modal superposition method of gyroscopic system has been used to compute the thermo-elastic response of axially moving beam subjected to mechanical and thermal loads. The 2D temperature field is calculated numerically by the ADI method and the differential equation is discretized by the Galerkin method. When the temperature field is a constant, the displacement appears a beating phenomenon under the harmonic load whose frequency is closed to fundamental frequency. The results show that thermal load has important influence on the beam’s deflection which deviates from straight equilibrium line during a rise of temperature condition is applied on boundary. The method used herein allows one to obtain a fast solution of thermo-elastic vibration problem.
References
-
Mote Jr C. D. A study of band saw vibrations. Journal of the Franklin Institute, Vol. 279, Issue 6, 1965, p. 430-444.
-
Simpson A. Transverse modes and frequencies of beams translating between fixed end support. Journal of Mechanical Engineering Science, Vol. 15, Issue 3, 1973, p. 159-164.
-
Wickert J. A., Mote Jr C. D. Classical vibration analysis of axially moving continua. Journal of Applied Mechanics, Vol. 57, Issue 3, 1990, p. 738-744.
-
Xu Xia Guo Analysis of the coupled thermoelastic vibration for axially moving beam. Journal of Sound and Vibration, Vol. 325, Issue 3, 2009, p. 597-608.
-
Wang Jingmei, Li Yinghui Response analysis of the coupled thermoelastic vibration for the axially flaying viscoelastic sandwich beam. Journal of Mechanical Sciences, Vol. 13, Issue 5, 2015, p. 1589-1606.
-
Meirovitch L. A new method of solution of the eigenvalue problem for gyroscopic systems. AIAA Journal, Vol. 12, Issue 10, 1974, p. 1337-1342.
-
Meirovitch L. A modal analysis for the response of linear gyroscopic systems. ASME Transactions. Vol. 42, Issue 2, 1975, p. 446-450.
-
Ashaju Abimbola, Samson Bright Alternating-Direction Implicit finite-difference method for transient 2D heat transfer in a metal bar using finite difference method. International Journal of Scientific and Engineering Research, Vol. 6, Issue 6, 2015, p. 105-108.
-
Wang Ting Yuan, Chen Charlie Chung Ping Thermal-ADI-A linear-time chip-level dynamic thermal-simulation algorithm based on alternating-direction implicit (ADI) method. IEEE Transactions on Very Large Scale Integration (VLSI) Systems, Vol. 11, Issue 4, 2003, p. 691-700.

