Biomechanics analysis of human lower limb during walking for exoskeleton design

2.1. Human motion capture experiment

The experiment is divided into three steps: experiment preparation, experiment starting and experimental data analysis. Experiment preparation is to complete a series of preparations before the experiment, including setting the markers by the Helen Hayes method. Experiment starting is to enter the guidance interface, where the user could complete one time of gait motion acquisition. Experimental data analysis is to calculate the joint torque by the internal algorithm.

2.1.1. Experiment equipment

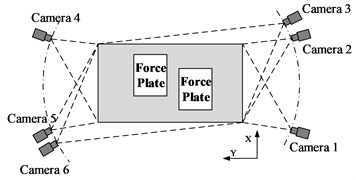
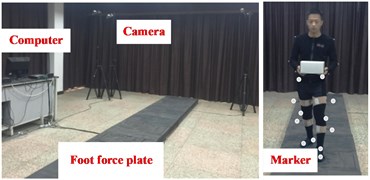
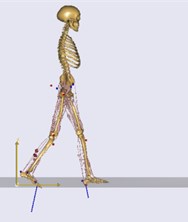
The motion capture system consists of six high speed cameras arranged in the scene, two foot force plates on the pavement (the main specifications is shown in Table. 2), a series of marked points pasted on the right positions of human body and a computer for recognizing coordinate values of marked points and calculating the dynamics of each joint, as shown in Fig. 2.

Fig. 2Human motion capture system

a)

b)

2.1.2. Set the markers

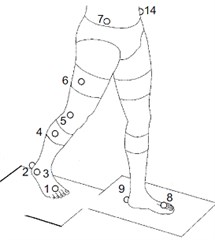
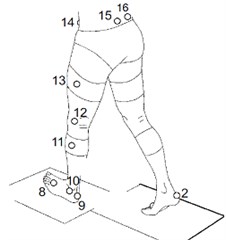
One step during the process of experiment preparation is to set the markers by rule and line. 16 markers in all were installed on the body. Markers 1-7 and markers 8-14 were attached on the left and right lower limbs separately. Marker 15 present sacral and marker 16 was used to distinguish the left and the right (as shown in Fig. 3). Take the right leg as an example, each body’s segment was constructed by three markers, as listed in Table. 3.

Table 2Main specifications

High speed camera		Foot force plates
Manufactures	Point Grey Corp, Canada	Manufactures	By ourselves
Specifications	S250e	Maximum force along X/Y/Z	1600/1600/3200 N
Maximum shooting speed	250 fps	Maximum moment around X/Y/Z	960/640/800 Nm
Dpi	832 (H)×832 (V)	Number	2 pieces
Number	6 pieces	Sensitivity	6.5634 kg/V
Shooting delay time	4 ms	Degree of nonlinearity	1.5 %
Shooting accuracy	5 mm	Hysterisis error	2.0 %
If own image preprocessing module	Yes	Output voltage	–10-10 V

Fig. 3Markers on human body

a)

b)

Table 3Markers list of right lower limb

Makers	1-2-3	3-4-5	4-5-6	5-6-7	6-7-15
Limb	Ankle joint	Shank	Knee joint	Thigh	Hip joint

2.2. Kinematic model based on multi-rigid body kinematics

During the process of experimental data analysis, we analyze the human motion by kinematic model based on multi-rigid body kinematics (seen in Section 2.2) and dynamic model based on Newton Euler mechanics (seen in Section 2.3).

Mathematical model of human is reconstructed by the multi rigid body kinematics. Take knee joint as an example, the triangle whose three vertexes are p3, p4, and p5 presents the shank segment (as shown in Fig. 5). The $vuw$ coordinates are defined by the triangle, where $v$ is the unit vector from point 3 to point 5; $u$ is the unit normal vector of the triangular plane, $w$ is determined by the right-hand rule. The expressions are as Eqs (1-3).

Fig. 43-D coordinate reconstruction

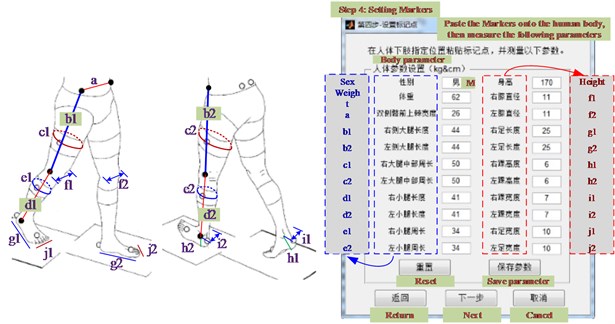
Fig. 53-D coordinate reconstruction

According to the empirical formula, the coordinate of the human’s knee center is derived by using the coordinates of the markers and $uwv$ unit vector:

where, $f_1$ is the width of the knee joint (as shown in Fig. 4). Similarly, the coordinates of the center of the right hip and ankle are $P_{hip}$ and $P_{ankle}$.

The shank and thigh segments are defined by the unit vector of the $i$ axis from the center of gravity of thigh to the center of gravity of shank:

Finally, the angle of knee joint is expressed about the two unit vector using Trigonometric equation:

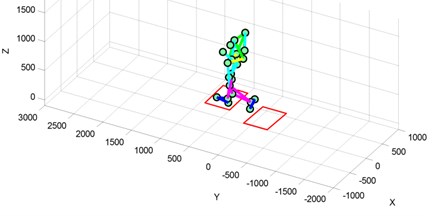
In the same way, ${\alpha }_{LKnee}$, ${\alpha }_{RAnkel}$, ${\alpha }_{LAnkel}$, ${\alpha }_{RHip}$, ${\alpha }_{LHip}$ could be achieved. The numerical values of the six angles change with human walking, whose law is shown is Section 3.2. In the experimental data analysis, we could reconstruct human walking process by driving a 3-D virtual human using the data of joints’ angle. As a result, the motion of the 3-D virtual human is very smooth, almost the same with the employee’s real gait. Therefore, the measured result can be trustworthy.

Fig. 6The reconstructed walking gait by 3-D virtual human

2.3. Dynamic model based on Newton Euler mechanics

Based on the Newton Euler mechanics, the mechanical equation was derived based on the balance equation of force and torque. According to the multi-rigid body theory, the human lower limb was divided into thigh segment, shank segment and foot segment (as shown in Fig. 7).

Fig. 7Relation of force and torque on each joint

For the foot segment, the force and torque of ankle are as follows according to the Newton’s second law:

where, $\overrightarrow{k}=$[0 0 –1]^T, $\overrightarrow{a}$ is the acceleration of $P_{footCG}$, which is the center of gravity of right foot. $m_{Foot}$ is estimated quality by anatomical empirical formula, whose expression is $m_{Foot}$= 0.0083 Weight + 254.5 $g_1{h}_1{i}_1$ – 0.065 (Weight, $g_1$, $h_1$ and $i_1$ are shown in Fig. 4). And the other variables are as follows:

where, ${\dot{L}}_i$, ${\dot{L}}_j$, ${\dot{L}}_k$ are the three components of ${\dot{L}}_{Ankle}$, which is the first-order derivative of right foot’s angular momentum. They are solved as follows:

where, $w_i$, $w_j$, $w_k$ are velocity of right foot about coordinate axis $i$, $j$ and $k$, whose value are updated automatically by computer as walking gait. $I_i$, $I_j$, $I_k$ are rotational inertia of right foot about coordinate axis $i$, $j$ and $k$, whose value are determined by anatomical empirical equation.

According to Eqs. (10)-(13), Eq.(9) could be expressed as:

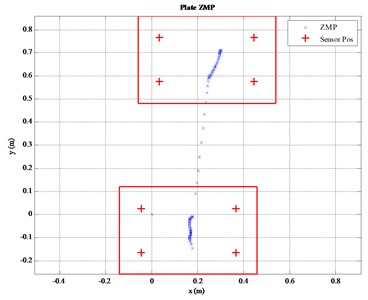
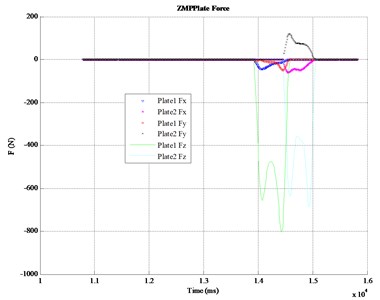
where, $p_{plate}$ is zero moment point (ZMP), i.e. the the center of $F_{plate}$ (as shown in Fig. 8). $F_{plate}$ and $M_{plate}$ are achieved by the foot force measuring system (as shown in Fig. 9). Although $M_{ankle}$ is a three-dimensional vector, what we concern about is the motion on the sagittal plane, i.e. the $i$-$k$ plane. The following discussion is based on this. The experiment results of $M_{ankle}$ will be shown in Section 3.2.

Fig. 8Locomotion trajectory of ZMP (pplate)

As the knee joint and hip joint, the method is the same that will not be repeat here.

2.4. Muscle biomechanics simulation model

Muscles, the drive source of the joint motion, help people walk easy and acclimatize himself to kinds of complex ground comfortably, which is a sophisticated and perfect bio-actuator and pretty useful for the design and control of the exoskeleton actuator. However, muscle’s force is unable measured by test directly because muscles are distributed in body tissues complicatedly. As a result, simulation becomes an effect way [8]. The Any Body Modeling System (ABMS) is a modeling and simulation software that could analyze the mechanics of the live human body working in concert with its environment by computer-aided engineering (CAE). As the external forces and boundary conditions of environment are defined and the posture or motion for the human body is impose on from a set recorded motion data, ABMS then runs a simulation and calculates individual muscle forces, joint forces and moments, metabolism, elastic energy in tendons, antagonistic muscle actions and much more (as shown in Fig. 1).

Fig. 9Fplate and Mplate

a)

b)

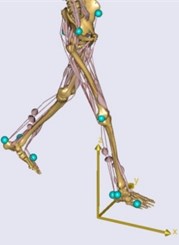
The simulation model is shown as Fig. 10. The greed balls drive the human simulation model to walk, correspond with the markers when doing a human motion capture experiment (as shown in Fig. 3). The length and quality of the simulation model are determined according to the real values of the employee, the same as Fig. 4 and Section 2. 3. When the model walks, all muscles work together to balance the external forces, including force from environment and bone gravity. In ABMS, the force of each muscle is obtained by solving the optimal solution of a target function (as Eq. (15) shows), which means to finish a work with minimal muscle activity.

where, $p$ is power number, $f_i^{\left(M\right)}$ is the muscle force of muscle $i$ and $Ni$ is its area. $n^{\left(M\right)}$ is the total number of muscle.

The constraint condition of Eq. (15) is:

Eq. (16) defines the dynamic equilibrium. Where, $C$ is coefficient matrix of muscle force, d is the vector of external forces.

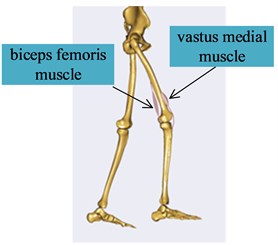
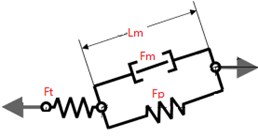
From previous research, it is found that the knee joint plays an important role in vibration reduction and swing during walk. Hidden display other muscles, only the principal muscles driving knee joint are kept for clarity, as shown in Fig. 10(b). So, the article addressed on the analysis of the knee principal muscle’s force. Around the knee joint, the biceps femoris muscle and the vastus medial muscle are a pair of antagonists, expressed by the Hill model [9] (as shown in Fig. 10(c)). $Fm$ presents the force generated by the contractile element, representing the active properties of the muscle fibers. $Fp$ is the force generated by the parallel-elastic element representing the passive stiffness of the muscle fibers. $Ft$ is the force generated by the serial-elastic element representing the elasticity of the tendon. $Lm$ is the length of the contractile element and Lt is the length of the tendon.

Fig. 10Simulation model in ABMS

a)

b)

c)

Fig. 11The main muscles around the knee joint

a)

b)

c)

When all settings are made, we can run simulation, whose simulation time is a cycle of whole walking gait, as shown in Fig. 11. The change law of muscle force for human walking would show in Section 4.

3.1. A whole gait

The natural gait could be roughly divided into the support phase and the swing phase. Compared with the swing phase, the support phase is more complex. According to the GRF, the support phase is divided into three sub-phases further. During the sub-phase I, the heel of the swing leg strikes the ground, and the two legs support on the ground at the same time initially, during which process the center of gravity transfers from the latter leg to the front leg gradually. During the sub-phase II, only the support leg is on the ground and the other leg swings in the air. During the sub-phase III, the original swing leg touches the ground again and the toe of the original support leg begins moving off the ground, preparing to enter the next gait cycle.

3.2. Kinematic and dynamics during the three support sub- phases

Each test is carried for three times repeatedly. The following conclusions could be drawn from Figs. 7-9. Firstly, the whole range of hip’s angle, knee’s and ankle’s were 40°~ –15°, 0°~60° and –17°~20°, respectively. Besides, the ranges of the three joints’ angle were listed as Table 4. Thirdly, all the joint torque curves were approximate sine function, among which the torque of the ankle was largest equal to the sum of knee’s and the hip’s approximately. All of the data perform has a guidance function to the motion control of exoskeleton’s joints during different sub-phases on the support phase.

Fig. 12Hip joint

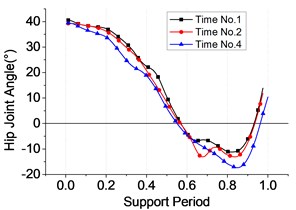
a) Angle

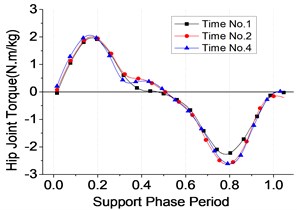
b) Torque

Fig. 13Knee joint

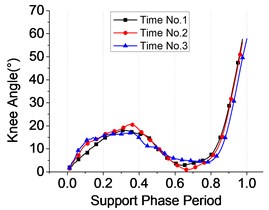
a) Angle

b) Torque

Fig. 14Ankle joint

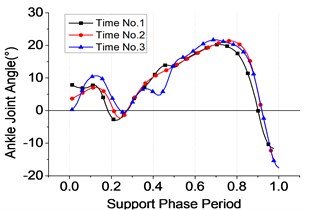
a) Angle

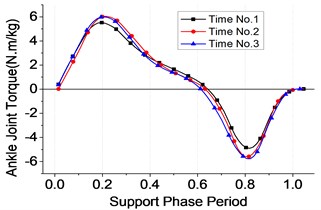
b) Torque

4.1. Vastus medialis muscle

During the support phase (0-0.8 s).

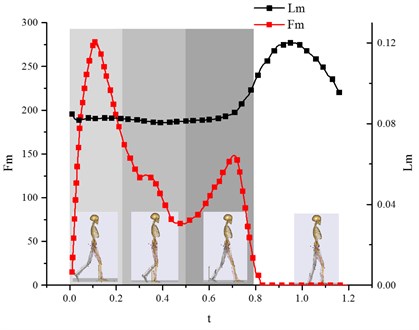
During this period, the vastus medialis muscle generated an active contraction force to stretch the knee joint and the muscle length $Lm$ maintained 0.08 m or so (as shown in Fig. 15) for the muscle being in isometric contraction, which lead to that $Fp$ was near zero (as shown in Fig. 16(a)). At this time, $Ft\approx Fm$, so the vibration of $Ft$ was similar with $Fm$(as shown in Fig. 15 and Fig. 16(b)). From the view of $Fm$, the vastus medialis muscle got a complex vibration during sub-phase I, II and III. $Fm$ reached a maximum value of 280 N or so at 0.1 s during sub-phase I when the leg just touched the ground. However, when the leg entered the real support phase, i.e. sub-phase II and III, the force of the muscle decreased rapidly because the human leg could rely on its own skeleton because it was vertical to the ground.

Fig. 15Variation curve of muscle active force Fm versus the muscle length Lm (vastus medialis muscle)

During the swing phase (0.8 s-1.2 s).

When entering the swing phase, the vastus medialis muscle was stretched passively, no more as an active unit, during which process $Fm$ was zero, as shown in Fig. 15. So, we addressed our research on $Fp$. At this time $Lm$ reached a maximum value of 0.12 m. $Fp$ changed from small to large and then from large back to small. During the process, $Fp$ reached a maximum value of 100 N at the maximal bending angle of the knee joint (as shown in Fig. 16(a)).

4.2. Biceps femoris muscle

In contrast to the vastus medialis muscle, it was passive during the supporting phase and active in the swing phase.

During the support phase (0-0.8 s).

$Lm$ maintained an original length of 0.16 m. Meanwhile, $Fm$ kept zero because the biceps femoris muscle was under relaxation. The value of $Fp$ was very small so that it could be ignored (as shown in Fig. 18(a)).

During the swing phase (0.8 s-1.2 s).

Once entering the swing phase, the biceps femoris muscle started playing an active function. As the decrease of the knee joint’s angle, the muscle shrunken its length from 0.16 m to 0.13 m (as shown in Fig. 17). After that it slowly released back to the original length until the end of the swing phase. The active force $Fm$ reached a maximum of 220 N (as shown in Fig. 17). However, as we know, in the Hill model, $Fp$ should changes significantly as $Lm$ changes (because the parallel-elastic element was in parallel with the active unit, $Lm$ was not only the length of the active unit, but also the length of the parallel-elastic element), but from Fig. 18(a) we could found that things were not that case. Why? A reasonable explanation was that the muscle was a two-element muscle instead of a three-element muscle, i.e. the Hill model.

Fig. 16Variation curve of Fp and Ft versus the muscle length Lm (vastus medialis muscle)

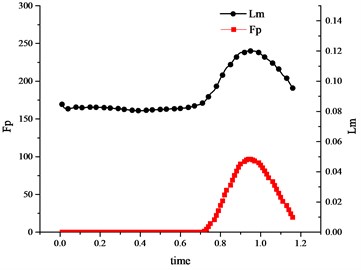
a) Muscle passive force $Fp$

b) Tendon force $Ft$

Fig. 17Variation curve of muscle active force Fm versus the muscle length Lm (biceps femoris muscle)

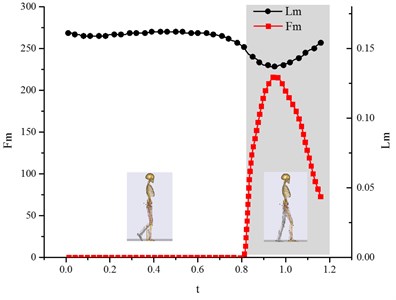
Fig. 18Variation curve of Fp and Ft versus the muscle length Lm (vastus medialis muscle)

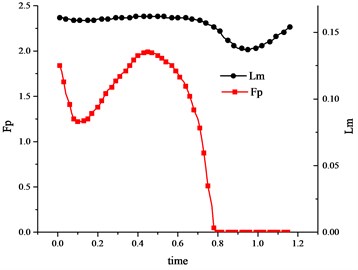
a) Muscle passive force $Fp$

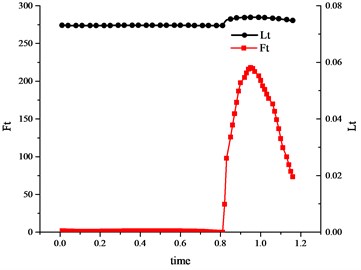
b) Tendon force $Ft$