Abstract
This paper established 6 kinds of different concrete beams, and 5 kinds of models were applied horizontal pre-stress. Their fundamental frequencies and deflections were numerically computed, and the correctness of numerically computational model was experimentally verified. Fundamental frequencies of 6 kinds of models were 46.23 Hz, 68.45 Hz, 69.36 Hz, 70.46 Hz, 72.11 Hz and 157.73 Hz respectively. Concrete beams without being applied pre-stress had a low fundamental frequency. The fundamental frequency of concrete beams could be obviously improved through internally applying pre-stressed tendons to concrete beams. In addition, the improvement effect of Model 5 was more obvious. When pre-stressed tendons were externally applied to the concrete beam, the fundamental frequency was greatly improved. However, externally pre-stressed tendons would limit the use of concrete beams and increase the cost. When other parameters remained unchanged, the fundamental frequency of pre-stressed concrete beams would gradually increase with the increase of pre-stress. When the pre-stress was the same, fundamental frequencies gradually increased with the increase of eccentricity, the number of circular tendons in composite tendons and concrete strength, and gradually decreased with the increase of counterweight. When the applied load was the same, Model 1 was not strengthened and its deflection was the largest. On the contrary, the model which was applied external pre-stress and its deflection was the smallest, but its use was limited and cost was high. In the strengthening proposals of internal pre-stress, composite tendons were applied to Model 5, and it presented a small deflection. When other parameters remained unchanged and the applied load was lower than a value, the deflection of concrete teams gradually increased with the increase of applied load. On the contrary, the deflection of concrete beam tended to be stable with the increase of applied load. When the applied load was the same, the deflection of concrete beams gradually decreased with the increase of eccentricity, the number of circular tendons in composite tendons and concrete strength, and gradually increased with the increase of counterweight.
1. Introduction
Pre-stressed concrete beams are an engineering structure which can actively combine high-strength steel with high-strength concrete. With excellent structural performance, pre-stressed concrete structure is widely applied in civil engineering including housing construction, bridges, water conservancy and so on. A lot of civil engineering has fully proved that pre-stressed concrete structure is an irreplaceable important structure in contemporary engineering construction. The application of pre-stressed concrete technology not only simply saves steel and concrete, but also solves many engineering problems. Compared with reinforced concrete structure, pre-stressed concrete structure has many advantages. For example, pre-stressed concrete overcomes the shortcoming of concrete, namely low resistance to tension, raises the crack resistance and stiffness of components, reduces the development of crack and deformation of components under the load, effectively improves the service performance of components and strengthens the durability of structures. In the meanwhile, the application of pre-stressed concrete technology solves technical problems other structural materials find it difficult to tackle. Pre-stressed concrete technology can be applied to construct all kinds of large-scale and long-span civil engineering.
At present, a lot of studies have been conducted on the strengthening of pre-stressed concrete structure [1-6]. Cuenca [7] added self-compacting fiber to concrete beams to provide pre-stress and experimentally studied the shear behavior of the structure under the force. The design of pre-stressed concrete beams was affected by a number of parameters. Kumar [8] took into account the combination of various parameters and used genetic algorithm to optimize and design concrete beams. However, the characteristics of optimized and designed concrete beams were not experimentally verified. Ren [9, 10] applied pre-stress to high-strength spiral tendons, and inserted the carbon fiber tendons without pre-stress and spiral tendons into concrete beams to conduct bending tests. Results showed that applying pre-stress to spiral tendons could effectively improve the ultimate bearing capacity, stiffness and anti-cracking ability of concrete beams. To reveal the shear performance of pre-stressed high-strength concrete beams, Yao [11] conducted an experiment on the shear performance of 11 pre-stressed high-strength concrete beams and 4 pre-stressed common concrete beams and comparatively analyzed the impact of different parameters on the failure type, load-deflection curve, bearing capacity and strain of experimental beams. To analyze the impact of repeated loads on the deformation performance of pre-stressed concrete beams, Zhao [12] conducted low-frequency and repetitive loading experiments on 6 pre-stressed concrete beams, and studied the deformation performance of pre-stressed concrete beams with different levels of pre-stress. However, the cited researches failed to involve the fundamental frequency and dynamic characteristics of pre-stressed concrete beams. He [13] conducted experimental research on the vibration of PRC beam and obtained a conclusion that the natural vibration frequency of PRC beam would be decreased with the increase of pre-stress when constraint conditions were unchanged. His conclusion was consistent with the conclusion of Abraham [14]. Liu [15] studied the relationship between the fundamental frequency of bending vibration of T-shaped beam and pre-stress. Experiments showed that the pre-stress had an influence on the vibration frequency of concrete beams. Vibration frequency increased with the pre-stress value. Abdalli [16] conducted dynamic tests and analysis on pre-stressed concrete notched beams. Results showed that pre-stress had a little impact on the dynamic characteristics of concrete beams. The impact of pre-stress on the dynamic characteristics of concrete beams was mainly up to the arrangement of pre-stressed cable. If pre-stressed cable was arranged like a parabola, frequency increased with the increase of pre-stress. If pre-stressed cable was arranged like a straight line, the impact could be neglected. Xia [17] obtained the basic dynamic characteristics of pre-stressed concrete beams through conducting forced vibration tests and dynamic analysis on of pre-stressed concrete beams, and further studied the impact of the size, shape and location of pre-stressed tendons on dynamic characteristics.
However, published studies on the fundamental frequency and dynamic characteristics of pre-stressed concrete beams were not systematic and failed to analyze a variety of parameters. Therefore, this paper systematically studied the fundamental frequency and dynamic characteristics of many kinds of pre-stressed concrete beams, analyzed many kinds of parameters affecting fundamental frequency and dynamic characteristics, and provided reference for the structural design of prestressed concrete beams.
2. Numerical computation for fundamental frequencies of pre-stressed concrete beams
As shown in Fig. 1, 6 different kinds of pre-stressed concrete beams were designed. Fig. 1(a) did not applied any pre-stressed tendons. Fig. 1(b) to Fig. 1(e) represented that pre-stressed tendons were applied in concrete beams. Fig. 1(b) represented that circular tendons were applied in the concrete beam. Fig. 1(c) represented that steel plates were applied in the concrete beam. Fig. 1(d) represented that I-shaped tendons were applied in the concrete beam. Fig. 1(e) represented that circular tendons and I-shaped tendons were together applied in the concrete beam. In Fig. 1(f), U-shaped pre-stressed tendons were applied outside the concrete beam to strengthen the concrete beam. The concrete beam was 3 m long, 160 mm wide and 300 mm high.
Fig. 1Schematic diagrams for 6 kinds of pre-stressed concrete beams
a) Model 1
b) Model 2
c) Model 3
d) Model 4
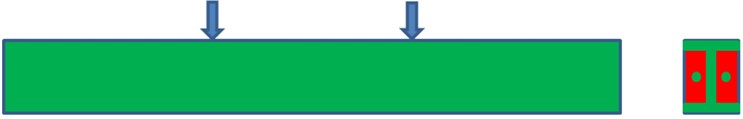
e) Model 5
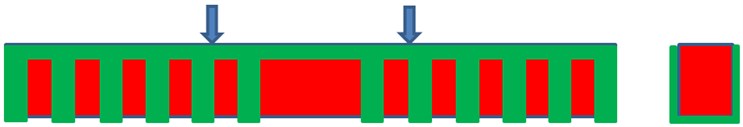
f) Model 6
The establishment of models for pre-stressed concrete beams was described in detail. Concrete adopted Solid65 element; longitudinal tendons and stirrup applied Link8 element; I-shaped tendons used Solid45 element. Modeling methods were basically the same. Therefore, only one modeling method was taken as an example. The modeling method of other models was similar to it. Both ends were fixed, and this model was under the action of symmetric loads. Therefore, we only built a half of model for convenience and simple simulation when the model was established, and finally obtained the whole finite element model through symmetry. A separation model was adopted to make computational results relatively accurate due to there were three kinds of elements. Namely, concrete, circular tendons and I-shaped tendons were established separately. Firstly, concrete and key points at the end section of I-shaped tendons were created. These key points formed lines and a plane, as shown in Fig. 2. Finally, the formed plane turned into an object through the command of stretch. Meshes of end section were generated firstly. Then, the whole model was divided through the command of extension. The size of meshes should be neither too small nor too large. The size of meshes was 20 mm. After meshes were generated, tendon elements were established through connecting nodes after mesh generation. To display tendons by means of link elements, we showed it through the command of ESHAPE. After loads were applied, load step was set as 100 and iteration times were 100 in order to converge the model as soon as possible and simplify computation. As this model was controlled by displacement, the convergence criterion was adjusted to the maximum value, namely 5 %. “Solve” was clicked for solution. Finally, finite element models for 6 kinds of pre-stressed concrete beams were obtained, as shown in Fig. 3. After finite element models for pre-stressed concrete beams were established, related materials were imported into models. The diameter of circular tendons was 14 mm. According to experimental design, the yield strength of tendons was 400 MPa. The design value of tensile strength and compressive strength of the concrete was 1.55 MPa and 15.5 MPa respectively, and its Elasticity modulus was 28 GPa.
Fig. 2Detailed section characteristics of model 5



Finite element model was used to compute frequencies of 6 kinds of pre-stressed concrete beams on top 6 orders, as shown in Table 1. As displayed from this table, fundamental frequencies of 6 kinds of models were 46.23 Hz, 68.45 Hz, 69.36 Hz, 70.46 Hz, 72.11 Hz and 157.73 Hz respectively. Concrete beams without being strengthened had a low fundamental frequency. The fundamental frequency of concrete beams could be obviously improved through internally applying pre-stressed tendons to concrete beams. In addition, the improvement effect of Model 5 was more obvious. When pre-stressed tendons were externally applied to the concrete beam, the fundamental frequency was greatly improved.
Table 1Modal frequencies of different pre-stressed concrete beams
Order | Model 1 / Hz | Model 2 / Hz | Model 3 / Hz | Model 4 / Hz | Model 5 / Hz | Model 6 / Hz |
1 | 46.23 | 68.45 | 69.36 | 70.46 | 72.11 | 157.73 |
2 | 57.91 | 71.09 | 72.91 | 73.29 | 79.81 | 171.52 |
3 | 134.55 | 178.75 | 181.56 | 185.99 | 192.90 | 432.11 |
4 | 142.34 | 189.17 | 199.70 | 201.15 | 205.38 | 446.41 |
5 | 167.56 | 228.85 | 231.17 | 235.06 | 247.50 | 566.72 |
6 | 192.33 | 256.98 | 259.89 | 274.12 | 275.70 | 619.34 |
However, externally pre-stressed tendons would limit the use of concrete beams and increase the cost. In addition, frequencies of Model 5 on other orders were higher than those of other concrete beams with internal pre-stress. Numerical computation showed that frequencies of 6 kinds of pre-stressed concrete beams were different, but modes did not present any changes because pre-stressed tendons would only change the effective stiffness of concrete beams rather than the major structure. As a result, modes of Model 5 on top 6 orders were only extracted, as shown in Fig. 4. Only the mode of the fifth order presented torsional vibration and other modes presented first-order and second-order bending vibrations.
Fig. 3Finite element models for 6 kinds of pre-stressed concrete beams
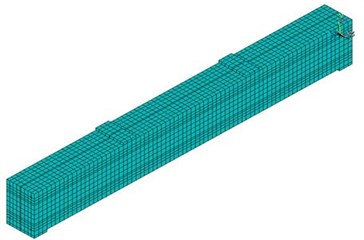
a) Model 1
b) Model 2
c) Model 3
d) Model 4
e) Model 5
f) Model 6
3. Impact of different parameters on fundamental frequencies of pre-stressed concrete beams
Fundamental frequencies of different pre-stressed concrete beams were analyzed. Model 5 was the composite pre-stressed tendon with higher fundamental frequency and advantage. Parameters affecting the fundamental frequency of pre-stressed concrete beams mainly included eccentricity, counterweight, type of composite tendons and concrete strength. Therefore, the finite element model was used to conduct parameter analysis on Model 5.
3.1. Eccentricity
As shown in Fig. 5, 2 pre-stressed tendons were arranged in the concrete beam. They were 0 mm, 75 mm and 150 mm respectively away from the center, and corresponding situations were recorded as Case 1, Case 2 and Case 3. Aimed at each single situation, pre-stress was changed from 0 kN to 200 kN and step size was 50 kN.
Fig. 4modes of pre-stressed concrete beams on top 6 orders
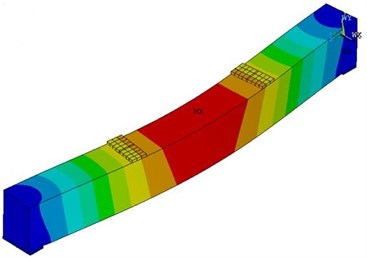
a) First order
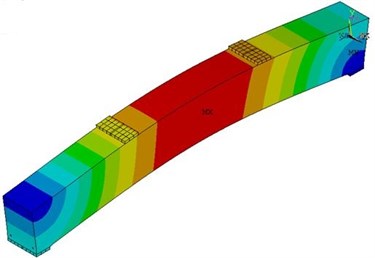
b) Second order
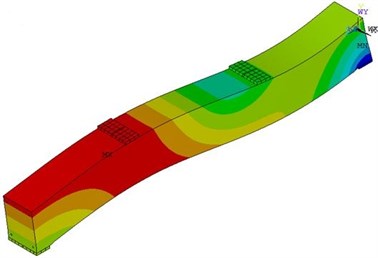
c) Third order
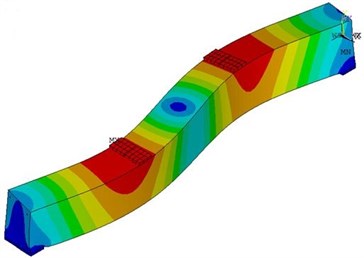
d) Fourth order
e) Fifth order

f) Sixth order
Fig. 5Pre-stressed concrete beams under different eccentricities

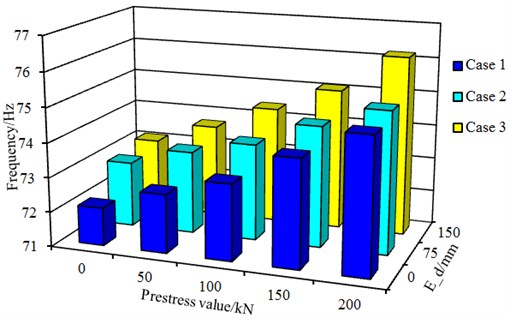
The finite element model was used to compute fundamental frequencies. Fundamental frequencies of pre-stressed concrete beams under three kinds of eccentricities were compared, as shown in Fig. 6. As displayed from Fig. 6, the fundamental frequency of pre-stressed concrete beams had a little change with the increase of pre-stress when the eccentricity was the same. When the eccentricity was 0 mm and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.89 %. When the eccentricity was 75 mm and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.02 %. When the eccentricity was 0 mm and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 4.24 %. When the pre-stressed value was the same, the fundamental frequency of concrete beams gradually increased with the increase of eccentricity because the increase of eccentricity would increase the bending stiffness of beams and improve the fundamental frequency of beams. From the perspective of force, the bottom of pre-stressed concrete beams was in tension when externally vertical loads were applied to pre-stressed concrete beams. Namely, the position where eccentricity was large was in tension. Based on cross-section assumption, the axis of beams was a neutral axis. Therefore, it was the most reasonable to arrange pre-stressed tendons at the bottom of beams.
Fig. 6Relationship between eccentricity and fundamental frequency
3.2. Counterweight
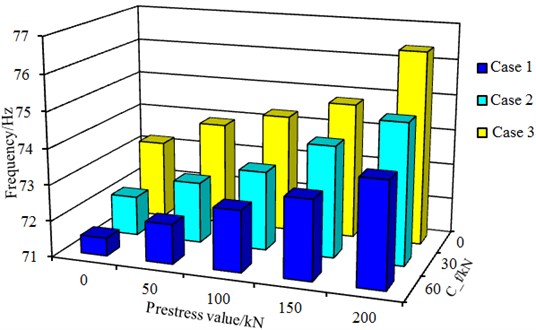
When bridges are in service, there are usually vehicles passing through. Therefore, it is necessary to consider the impact of counterweight on the fundamental frequency of pre-stressed concrete beams. As shown in Fig. 7, counterweights including 0 kN, 30 kN and 60 kN were applied at 1/3 point of the beam, and corresponding situations were recorded as Case 1, Case 2 and Case 3. The eccentricity of pre-stressed tendons was 0 mm. Aimed at each single situation, pre-stress was changed from 0 kN to 200 kN and step size was 50 kN. The finite element model was used to compute fundamental frequencies. Fundamental frequencies of pre-stressed concrete beams under three kinds of counterweights were compared, as shown in Fig. 8. As displayed from Fig. 8, the fundamental frequency of pre-stressed concrete beams was increased with the increase of pre-stress when the counterweight was the same. When the counterweight was 0 kN and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 4.37 %. When the counterweight was 30 kN and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.89 %. When the counterweight was 60 kN and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.36 %. When the pre-stress value was the same, the fundamental frequency of concrete beams decreased with the increase of counterweight. Take the pre-stress value 200 kN as an example. When the counterweight increased from 0 kN to 60 kN, fundamental frequencies decreased from 76.4 Hz to 73.9 Hz. This phenomenon could also be explained by the increase of distributed mass of beams. Namely, the concentrated loads were equivalent to the increase of distributed mass of beams. When the beam was in vibration, loads did not disappear, but moved all the time, which would thus affect the stiffness matrix of beams in the process of vibration and influence the fundamental frequency of beams.
Fig. 7Pre-stressed concrete beams under different counterweights
Fig. 8Relationship between counterweight and fundamental frequency
3.3. Type of composite tendons
As shown in Fig. 9, the combination types of circular tendons and I-shaped tendons were changed and recorded as Case 1, Case 2 and Case 3. The eccentricity of pre-stressed tendons was 0 mm. Its counterweight was 30 kN. Aimed at each single situation, pre-stress was changed from 0 kN to 200 kN and step size was 50 kN. The finite element model was used to compute corresponding fundamental frequencies. Fundamental frequencies of pre-stressed concrete beams under three kinds of composite tendons were compared, as shown in Fig. 9.
Fig. 9Different types of composite tendons

a) Case 1

b) Case 2

c) Case 3
As displayed from Fig. 9, the fundamental frequency of pre-stressed concrete beams had a little change with the increase of pre-stress when the type of composite tendons was the same. When the situation was Case 1 and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.89 %. When the situation was Case 2 and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.25 %. When the situation was Case 3 and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.61 %. When the pre-stress value was the same, the fundamental frequency of concrete beams was increased with the type of composite tendons. Take the pre-stress value 200 kN as an example. When the situation was changed from Case 1 to Case 3, fundamental frequencies increased from 74.9 Hz to 77.4 Hz. When the pre-stress value was the same, the application of many circular tendons would increase the bending stiffness of concrete beams and thus improve the fundamental frequency of beams.
Fig. 10Relationship between types of composite tendons and fundamental frequency

3.4. Concrete strength
The concrete strength of studied beams in the previous was C15. The grade of concrete strength was changed into C15, C25 and C35, and corresponding situations were set as Case 1, Case 2 and Case 3. The eccentricity of pre-stressed tendons was 0 mm. Its counterweight was 30 kN. Aimed at each single situation, pre-stress was changed from 0 kN to 200 kN and step size was 50 kN. The finite element model was used to compute corresponding fundamental frequencies. Fundamental frequencies of pre-stressed concrete beams under three kinds of concrete strength were compared, as shown in Fig. 11.
Fig. 11Relationship between concrete strength and fundamental frequency
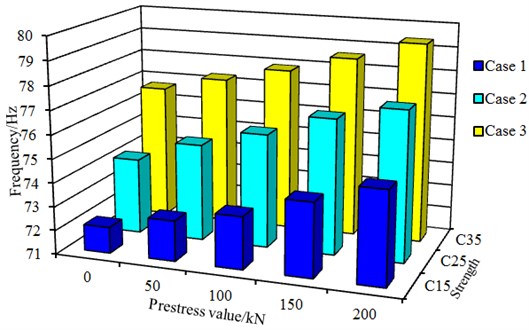
As displayed from Fig. 11, the fundamental frequency of pre-stressed concrete beams had a little change with the increase of pre-stress when the concrete strength was the same. When the concrete strength was C15 and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.89 %. When the concrete strength was C25 and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 4.18 %. When the concrete strength was C35 and pre-stress increased from 0 kN to 200 kN, the fundamental frequency of concrete beams increased by 3.52 %. When the pre-stress value was the same, the fundamental frequency of concrete beams was increased with the concrete strength. Take the pre-stress value 200 kN as an example. When the concrete strength was changed from C15 to C35, fundamental frequencies increased from 74.9 Hz to 79.4 Hz. When the pre-stress value was the same, the higher the grade of concrete strength was, the greater the stiffness of concrete would be.
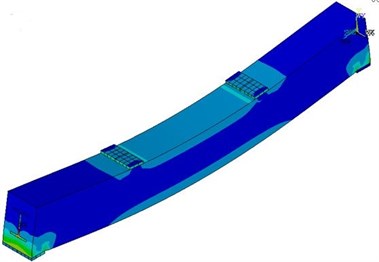
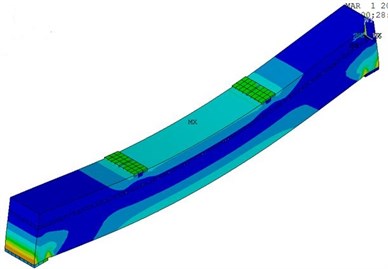
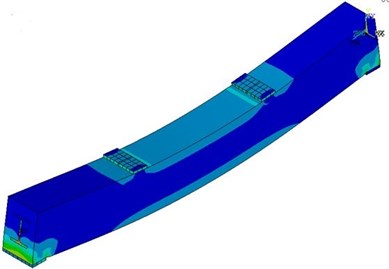
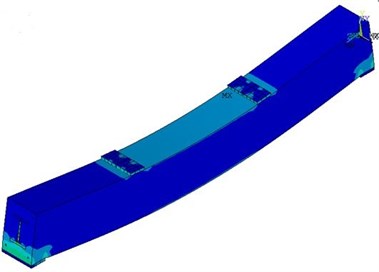
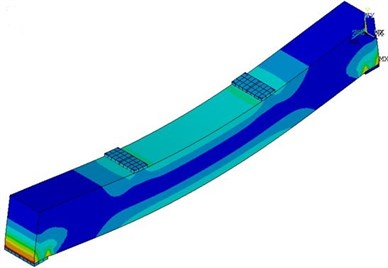
Fig. 12Strains of 6 kinds of pre-stressed concrete beams

a) Model 1
b) Model 2
c) Model 3
d) Model 4
e) Model 5
f) Model 6
4. Numerical computation and experimental verification for the dynamic characteristics of pre-stressed concrete beams
Numerical computation was conducted on the fundamental frequency information of pre-stressed concrete beams, but dynamic characteristics of the structure could not be reflected. Therefore, finite element model was also used to compute dynamic characteristics including strain and deflection of beams, and the computational results were then compared with experimental results to verify the correctness of computational models.
4.1. Numerical computation for dynamic characteristics of pre-stressed concrete beams
Strains and deflections of 6 kinds of models were extracted, as shown in Fig. 12 and Fig. 13. It could be seen that 6 kinds of models had similar strains and large strains were always presented at the fixed ends and the middle position of beams. Model 1 did not take any strengthening measures. When the load was the same, the deflection of Model 1 was the largest. On the contrary, the deflection of Model 6 was the smallest because Model 6 was externally strengthened with pre-stress. As for internal strengthening measures, the deflection of Model 5 was the smallest. In the linear phase, the stiffness of beams had a little change before and after strengthening, which indicated that strengthening measures did not play an obvious role in the elastic phase. When the deformation of concrete beams was large, the stiffness of strengthened beams decreased little. However, the stiffness of un-strengthened beams decreased greatly. As a result, the stiffness of strengthened beams was higher than that of un-strengthened beams when pre-stressed tendons yielded. It was mainly because the strain of pre-stressed tendons in pre-stressed concrete beams was small when the deformation was small. Therefore, distributed stress was also small, had weak effect on restricting the deformation of beams and contributed little to improving the stiffness of beams. When the deformation of concrete beams was large and especially when concrete beams cracked, stress was relieved at the crack part of concrete beams. Most of stress was assigned to pre-stressed tendons. At this time, the stress of pre-stressed tendons increased rapidly to powerfully restrain the deformation of strengthened beams, reduce the deflection of beams and improve the bending stiffness. For a low-strength concrete beam, the bearing capacity of beams cannot be improved obviously without any strengthening measures. The stiffness of externally strengthened beams was improved more obviously than that of externally strengthened beams, but external strengthening will limit the use of beams. Therefore, internal strengthening was usually chosen for concrete beams. Model 5 adopted the type of composite tendons, which achieved a better results.
Fig. 13Deflections of 6 kinds of pre-stressed concrete beams

4.2. Experimental verification for numerically computational model
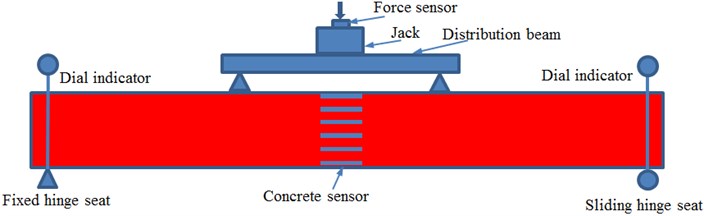
Numerical computation model was relatively complex. Therefore, it was necessary to verify it through experiments. According to numerically computational model, a calibrated oil jack was used for loading. To better simulate the force characteristics of simply supported beam under concentrated loads, thick steel plates were placed in the position of support and action of concentrated loads in order to avoid the local failure of concrete because of excessive stress. Experimental loading device was shown in Fig. 14. Sensors were arranged at the mid-span of concrete beams to test the deflection of concrete beams when it was loaded. Experiments adopted dynamic loading process. Two points of experimental beam were intensively loaded through primary distribution beam. Concrete beam was loaded at the load step of 2 kN before its crack and stopped loading when the load for the theoretical crack of experimental beam was reached. After each loading, the value presented by the instrument kept stable to record data. Experimental deflection was compared with numerical computation, as shown in Fig. 15.
Fig. 14Experimental deflection device of pre-stressed concrete beams
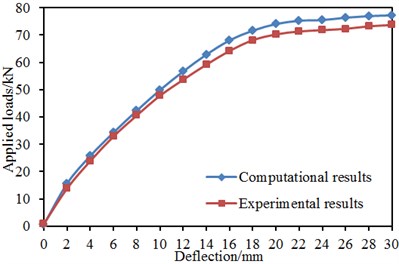
It could be seen from the Fig. 15 that experimental results were well consistent with numerically computational results. For example: The biggest difference between experiment and simulation in 6 kinds was 3.2 kN, 1.8 kN, 1.6 kN, 1.0 kN, 0.9 kN and 0.8 kN. Numerically computational model could replace experiments to complete related analysis and research. However, the stiffness of finite element model was higher than that of experimental beam under the action of the same load at each stage of computational process. As a result, the deflection was lower than experimental value in the end. Reasons were mainly as follows: 1) Before the action of loads, concrete in reality had micro-cracks caused by the dry-shrinkage strain of cement stone. After the action of loads, these internal micro-cracks started to stretch and extend and formed large cracks, which would reduce the stiffness of beams. However, finite element model did not contain micro-cracks, which caused that its stiffness was greater than experimental value. 2) In finite element analysis, suppose that tendons, steel plates and concrete were bonded finely without relative slippage. In the actual situation, the phenomenon of relative slippage inevitably existed between them. This phenomenon was more serious at later stage, which increased the gap between the stiffness of experimental beam and the structure of the finite element.
5. Impact of different parameters on dynamic characteristics of pre-stressed concrete beams
Through referring to analysis in Section 3, parameters affecting the fundamental frequency of pre-stressed concrete beams mainly included eccentricity, counterweight, type of composite tendons and concrete strength. Similarly, these parameters had an obvious impact on dynamic characteristics of concrete beams. Therefore, the verified finite element model was used to study them.
5.1. Eccentricity
As shown in Fig. 5, 2 pre-stressed tendons were arranged in the concrete beam. They were 0 mm, 75 mm and 150 mm respectively away from the center, and corresponding situations were recorded as Case 1, Case 2 and Case 3. Aimed at each single situation, the finite element model was used to compute the deflection. The deflection of pre-stressed concrete beams under three kinds of eccentricities was compared, as shown in Fig. 16. As displayed from Fig. 16, the deflection of concrete beams increased with the increase of loads when the eccentricity was the same and load was less than 100 kN. However, the deflection of concrete beams basically did not change with the load when load was more than 100 kN. It was mainly because pre-stressed tendons in concrete beams played an important role when the deflection reached a certain value. As a result, the stiffness of concrete beams restored to a high value, which basically kept deflection unchanged. However, the deflection of concrete beams gradually decreased with the increase of the eccentricity when the applied load was the same. When the pre-stressed value was the same, the deflection of concrete beams gradually decreased with the increase of the eccentricity because the increase of the eccentricity would increase the bending stiffness of beams. From the perspective of force, the bottom of pre-stressed concrete beams was in tension when externally vertical loads were applied to pre-stressed concrete beams. Namely, the position where eccentricity was large was in tension. Based on cross-section assumption, the axis of beams was a neutral axis. Therefore, it was the most reasonable to arrange pre-stressed tendons at the bottom of beams.
Fig. 15Comparison of deflections of 6 kinds of models between experiment and simulation
a) Model 1
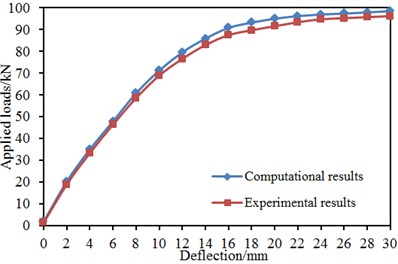
b) Model 2

c) Model 3
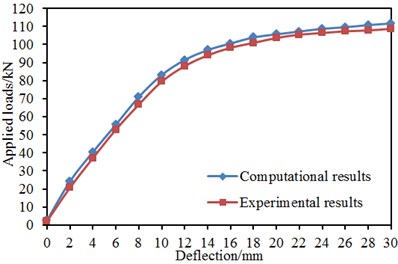
d) Model 4
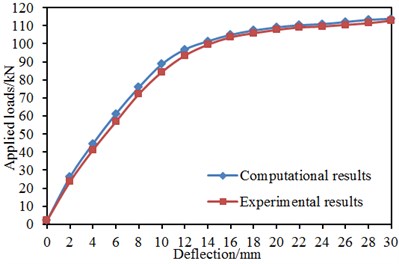
e) Model 5

f) Model 6
5.2. Counterweight
When bridges are in service, there are usually vehicles passing through. Therefore, it is necessary to consider the impact of counterweight on the deflection of pre-stressed concrete beams. As shown in Fig. 7, counterweights including 0 kN, 30 kN and 60 kN were applied at 1/3 point of the beam, and corresponding situations were recorded as Case 1, Case 2 and Case 3. The eccentricity of pre-stressed tendons was 0 mm. Aimed at each single situation, the finite element model was used to compute the deflection. The deflection of pre-stressed concrete beams under three kinds of counterweights was compared, as shown in Fig. 17. As displayed from Fig. 17, the deflection of concrete beams increased with the increase of loads when the counterweight was the same and load was less than 100 kN. However, the deflection of concrete beams basically did not change with the load when load was more than 100 kN. It was mainly because pre-stressed tendons in concrete beams played an important role when the deflection reached a certain value. As a result, the stiffness of concrete beams restored to a high value, which basically kept deflection unchanged. However, the deflection of concrete beams gradually increased with the increase of the counterweight when the applied load was the same. This phenomenon could also be explained by the increase of distributed mass of beams. Namely, the concentrated loads were equivalent to the increase of distributed mass of beams. When the beam was in vibration, loads did not disappear, but moved all the time, which would thus affect the stiffness matrix of beams in the process of vibration and influence the deflection of beams.
Fig. 16Relationship between eccentricity and deflection

Fig. 17Relationship between counterweight and deflection
5.3. Type of composite tendons
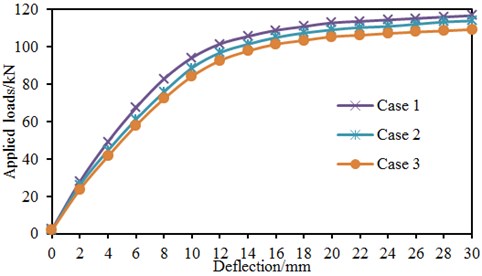
As shown in Fig. 9, the combination types of circular tendons and I-shaped tendons were changed and recorded as Case 1, Case 2 and Case 3. The eccentricity of pre-stressed tendons was 0 mm. Its counterweight was 30 kN. Aimed at each single situation, the finite element model was used to compute corresponding deflection. The deflection of pre-stressed concrete beams under three kinds of composite tendons was compared, as shown in Fig. 18. As displayed from Fig. 18, the deflection of concrete beams increased with the increase of loads when the composite tendon was the same and load was less than 100 kN. However, the deflection of concrete beams basically did not change with the load when load was more than 100 kN. It was mainly because pre-stressed tendons in concrete beams played an important role when the deflection reached a certain value. As a result, the stiffness of concrete beams restored to a high value, which basically kept deflection unchanged. However, the deflection of concrete beams gradually decreased with the composite tendons when the applied load was the same. Take the load 80 kN as an example. When the situation was changed from Case 1 to Case 3, the deflection was 9.1 mm, 7.6 mm and 5.9 mm respectively. When the load was the same, the application of many circular tendons would increase the bending stiffness of concrete beams and thus reduce the deflection of beams.
Fig. 18Relationship between type of composite tendons and deflection
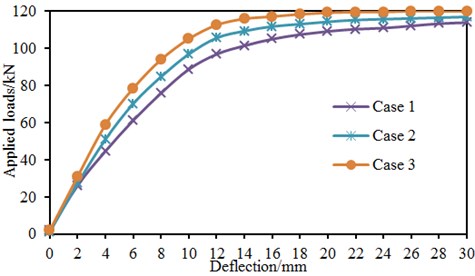
5.4. Concrete strength
The concrete strength of studied beams in the previous was C15. The grade of concrete strength was changed into C15, C25 and C35, and corresponding situations were set as Case 1, Case 2 and Case 3. Aimed at each single situation, the finite element model was used to compute corresponding deflection. The deflection of pre-stressed concrete beams under different concrete strength was compared, as shown in Fig. 19.
Fig. 19Relationship between concrete strength and deflection
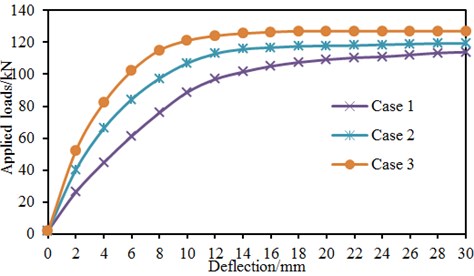
Different from the other parameters, the improvement effect on the concrete strength was the most obvious. The deflection of concrete beams increased with the increase of loads when the concrete strength was C15 and load was less than 100 kN. However, the deflection of concrete beams basically did not change with the load when load was more than 100 kN. The deflection of concrete beams increased with the increase of loads when the concrete strength was C25 and load was less than 114 kN. However, the deflection of concrete beams basically did not change with the load when load was more than 114 kN. The deflection of concrete beams increased with the increase of loads when the concrete strength was C35 and load was less than 120 kN. However, the deflection of concrete beams basically did not change with the load when load was more than 120 kN. It was mainly because pre-stressed tendons in concrete beams played an important role when the deflection reached a certain value. As a result, the stiffness of concrete beams restored to a high value, which basically kept deflection unchanged. However, the deflection of concrete beams gradually decreased with the concrete strength when the applied load was the same. When the load was the same, the higher the grade of concrete strength was, the greater the stiffness of concrete would be.
6. Conclusions
This paper established 6 kinds of different concrete beams, numerically computed corresponding fundamental frequencies and deflections, and experimentally verified the correctness of numerical computation model. Finally, the following conclusions can be achieved:
1) Fundamental frequencies of 6 kinds of models were 46.23 Hz, 68.45 Hz, 69.36 Hz, 70.46 Hz, 72.11 Hz and 157.73 Hz respectively. Concrete beams without being strengthened had a low fundamental frequency. The fundamental frequency of concrete beams could be obviously improved through internally applying pre-stressed tendons to concrete beams. In addition, the improvement effect of Model 5 was more obvious. When pre-stressed tendons were externally applied to the concrete beam, the fundamental frequency was greatly improved. However, externally pre-stressed tendons would limit the use of concrete beams and increase the cost.
2) When the eccentricity, counterweight, type of composite tendons and concrete strength of concrete beams remained unchanged, the fundamental frequency of prestressed concrete beam gradually increased with the increase of prestress. Under the same prestress, the fundamental frequency of concrete beam gradually increased with the increase of eccentricity, the number of circular tendons in composite tendons and concrete strength and gradually decreased with the increase of counterweight.
3) Regarding 6 kinds of different concrete beam structures, large strains always appeared at the fixed ends of and in the middle position of concrete beams. Under the action of the same load, Model 1 did not take strengthening measures and deflection was the largest. On the contrary, Model 6 was externally strengthened with prestress and deflection was the smallest. However, it was restricted in engineering application and cost was high. In internal strengthening schemes, Model 5 internally applied composite tendons to the beam, presenting the smallest deflection.
4) Due to the defects of experimental beam in the process of production, the stiffness of finite element model was higher than that of experimental beam. In the end, deflection value was smaller than experimental value. However, experimental results were well consistent with numerical computation results. Numerical computation model could replace experiments to complete related analysis and research.
5) When the eccentricity, counterweight, type of composite tendons and concrete strength of concrete beams remained unchanged and the applied load was lower than a value, the deflection of concrete teams gradually increased with the increase of applied load. On the contrary, the deflection of concrete beam tended to be stable with the increase of applied load. When the applied load was the same, the deflection of concrete beams gradually decreased with the increase of eccentricity, the number of circular tendons in composite tendons and concrete strength, and gradually increased with the increase of counterweight.
References
-
Chen W., Hao H., Chen S. Numerical analysis of prestressed reinforced concrete beam subjected to blast loading. Materials and Design (1980-2015), Vol. 65, 2015, p. 662-674.
-
Xue W., Tan Y., Zeng L. Flexural response predictions of reinforced concrete beams strengthened with prestressed CFRP plates. Composite Structures, Vol. 92, Issue 3, 2010, p. 612-622.
-
Motavalli M., Czaderski C., Pfyl-Lang K. Prestressed CFRP for strengthening of reinforced concrete structures: recent developments at Empa, Switzerland. Journal of Composites for Construction, Vol. 15, Issue 2, 2010, p. 194-205.
-
Hajihashemi A., Mostofinejad D., Azhari M. Investigation of RC beams strengthened with prestressed NSM CFRP laminates. Journal of Composites for Construction, Vol. 15, Issue 6, 2011, p. 887-895.
-
Akguzel U., Pampanin S. Assessment and design procedure for the seismic retrofit of reinforced concrete beam-column joints using FRP composite materials. Journal of Composites for Construction, Vol. 16, Issue 1, 2012, p. 21-34.
-
Martí J. V., Yepes V., González-Vidosa F. Memetic algorithm approach to designing precast prestressed concrete road bridges with steel fiber reinforcement. Journal of Structural Engineering, Vol. 141, Issue 2, 2014, p. 04014114.
-
Cuenca E., Serna P. Shear behavior of prestressed precast beams made of self-compacting fiber reinforced concrete. Construction and Building Materials, Vol. 45, 2013, p. 145-156.
-
Kumar C. J. D., Venkat L. Genetic algorithm based optimum design of prestressed concrete beam. International Journal of Civil and Structural Engineering, Vol. 3, Issue 3, 2013, p. 644.
-
Ren Z. H., Zeng X. T., Liu H. L., Zhou F. J. Experiment of RC beams strengthened with near-surface mounted carbon fiber reinforced plastic bar and pre-stressed helical rib rebar. Journal of Hohai University (Natural Science), Vol. 40, Issue 4, 2012, p. 370-375, (in Chinese).
-
Ren Z. H., Zeng X. T., Zhou F. J. Analysis for bending capacity of reforced concrete beams multiple strengthened with near-surface mounted carbon fiber reinforced plastic bar and pre-stressed helical rib bar. Fiber Reinforced Plastics/Composites, Vol. 5, 2012, p. 33-37, (in Chinese).
-
Yao D. L., Jia J. Q., Yu F. Experimental study of the shear capacity of prestressed ultra-high reinforced concrete beams with stirrups. Journal of Hunan University (Natural Science), Vol. 42, Issue 3, 2015, p. 23-30, (in Chinese).
-
Zhao Y., Zhang X. G., He S. H. Deformation of prestressed concrete beam under repeated loads. Journal of PLA University of Science and Technology (Natural Science Edition), Vol. 13, Issue 3, 2012, p. 292-297, (in Chinese).
-
Liu L. J., He S. H., Zhao X. X. Experimental study about influence of prestress on vibration frequency of T-shaped concrete grider. Railway Engineering, Vol. 2, 2007, p. 4-6.
-
Abraham M. A., Park S. Y., Stubbs N. Loss of prestress prediction on nondestructive damage location algorithms. SPIE, Smart Structures and Materials, Vol. 2446, 1995, p. 60-67.
-
Liu C. B., Wang B. S., Ou C. C. Prediction of PRC beam’s loss of prestress based on vibration test. Journal of Vibration and Shock, Vol. 22, Issue 3, 2003, p. 95-97, (in Chinese).
-
Abdalli H., Kennedy J. B. Dynamic analysis of prestressed concrete beams with openings. Journal of Structural Engineering, Vol. 121, Issue 7, 1995, p. 1058-1068.
-
Xia Z. H., Zong Z. H. Analysis of influence of prestressing on dynamic characteristics of a concrete beam. Journal of Vibration and Shock, Vol. 26, Issue 7, 2007, p. 129-134, (in Chinese).

