Nonlinear dynamic analysis of a coupled lateral-torsional spur gear with eccentricity

2.1. Lumped-parameter model for the spur gear

For the purpose of illustration, the simplified 4-DOF lumped parameter model taking into account the coupled lateral-torsional vibration is established in Fig. 1. This line of action is defined as the common tangent line of the base circles in the gears having involute tooth form. All other supports/bearings are also modeled as springs. In addition, friction forces due to gear teeth contact and other dissipative effects are captured using damping. The gear is represented by base circles with radius $r_{b1}$ and $r_{b2}$, respectively. $m_1$ and $m_2$ indicate the masses of the gears. $J_1$ and $J_2$ represent the moment of inertia of the driving and driven gears. The eccentricities for the gears are denoted by ${\rho }_1$ and ${\rho }_2$, respectively. $e\left(t\right)$ represents the static transmission error, which is the high-frequency caused by manufacturing and installation errors. The gear mesh has a constant backlash equal to 2$b$ along the line of action. $O_1$ and $O_2$ are the centers of driving and driven gears. $G_1$ and $G_2$ represent the center-of-mass coordinates. The torsional angular displacement of gear is assumed to result from a constant angular velocity term ${\omega }_{i}t$ ($i=$1, 2) plus a small variation displacement ${\theta }_i\left(t\right)$ due to vibrations originating from the flexibility of the mating gear teeth. Therefore, the angle displacements ${\phi }_i\left(t\right)$ of the driving and driven gears can be expressed by the following equations:

where ${\omega }_1$ and ${\omega }_2$ are the constant angular velocity components of the driving and driven gears.

The initial angles of the gears are equal to zero, and the driven gear rotational direction is positive. The centers of mass $G_1$, $G_2$ and the centers of rotation $O_1$, $O_2$ can be written:

Fig. 1Dynamic model of coupled lateral-torsional vibration spur gear system

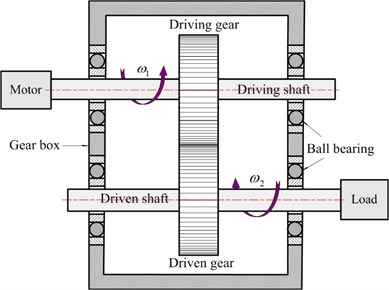
a) Schematic illustration of the coupled system

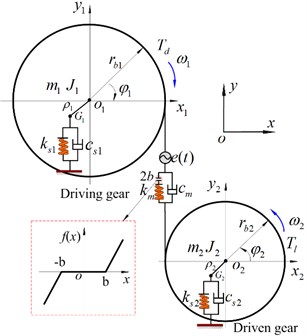
b) Dynamic model of the coupled system

The meshing process of spur gears is shown in Fig. 1, the dynamic meshing force between driving gear and driven gear is closely related to vibration displacement (bending and torsional vibration) of the gears, which represents by relative coordinates along the line of action. The dynamic meshing force between the driving gear and driven gear is given by:

where, $f\left(\delta \right)$ is the backlash function, which is essentially a discontinuous and non-differentiable function, and it is the main source of nonlinearity in the system. So the backlash function $f\left(\delta \right)$ is defined by the following equation:

Ignoring the effect of dynamic meshing force caused by the instantaneous variable along the line of action, $\delta$ is the actual deformation, which is the difference between the dynamic transmission error $\left(y_1+{\theta }_1{r}_{b1}-{\rho }_1\mathrm{s}\mathrm{i}\mathrm{n}{\phi }_1\right)-(y_2+{\theta }_2{r}_{b2}-{\rho }_2\mathrm{s}\mathrm{i}\mathrm{n}{\phi }_2))$ and static transmission error ($e\left(t\right)$). Therefore, the $\delta$ is given by:

Submitting Eq. (5) into Eq. (3), the dynamic meshing force $F_m$ is given by:

where, $e\left(t\right)$ is the static transmission errors, applied along the line of action to model any manufacturing error, installing error, and teeth deformations. As a result, the static transmission error can be approximated as periodic function, and it can be written as follow:

in which, $e_0$ and $e_r$ are the mean and the fluctuation. ${\phi }_e$ represents the initial phase angle, then ${\omega }_i=2\pi n_1{z}_1/60=2\pi n_2{z}_2/60$ is the mesh angular frequency, $z_1$ and $z_2$ are the driving and driven gear number of teeth, $n_1$ and $n_2$ indicate the rotational speed of the driving and driven gears.

In order to simplify the dynamic problem, the output torque is usually assumed to be constant and neglect the fluctuation in the previous literature. Actually, the output torque also has fluctuation. Therefore, the output/input torque $T_i\left(t\right)$ ($i=d$, $l$) can be decomposed into mean $T_{im}$ ($i=d$, $l$) and perturbation $T_{ir}\left(t\right)$ ($i=d$, $l$) parts. This is due to the spur gear is a part of wind turbine gearbox, it essentially fluctuates between low and high values around the stochastic nature of wind speed. Here, the output and input torque, $T_d$, $T_l$, are given by:

where, $T_{dm}$ and $T_{lm}$ are the means, $T_{dr}$ and $T_{lr}$ represent the fluctuations, ${\omega }_i=2\pi n_i/60$ ($i=$1, 2) is the rotational frequency, ${\phi }_d$ and ${\phi }_l$ represent the initial phase angles.

2.2. Equations of the motion

The dynamic equations of coupled gear system, shown in Fig.1, can be described with the four generalized coordinates $y_1$, ${\theta }_1$, $y_2$ and ${\theta }_2$. After expressing the kinetic energy $T$, the potential energy $U$ and dissipation function $R$ of the spur gear system in terms of the generalized coordinates, then they are substituted into Lagrange’s equation in order to obtain the vibration differential equations.

The kinetic energy $T$, can be written as follow:

Considering the shaft, the bearing and tooth deformations, the potential energy $U$ can be expressed as follow:

Due to take into account the mesh damping, bearing damping and shaft damping, the dissipation function $R$ is given by:

The generalized force vector $F$ of the spur gear system can be represented by:

The vibration differential equations are derived using equation, which is given by:

Take the coupled effects of lateral vibration and torsional vibration into account, substitution Eqs. (9)-(12) into Eq. (13), the mathematical model of the gear system can be obtained. And a matrix-vector form can be written as:

where, the spur gear system displacement vector is arranged as:

The displacement vector is a 1×4 column vector in which the elements are the generalized coordinates. $y_i$, ($i=$1, 2) are the lateral linear displacement of the driving gear and driven gear. ${\theta }_i$, ($i=$1, 2) are the torsional angular displacement of the driving gear and driven gear. $\mathbf{M}$, $\mathbf{C}$ and $\mathbf{K}$ are the mass matrix, damping matrix and stiffness matrix respectively, ${\mathbf{F}}_N$ is the nonlinear factor vector, and ${\mathbf{F}}_L$ is the linear factor vector are as shown below. It should be noted that the nonlinear internal force vector ${\mathbf{F}}_N$ is a function of the displacement vector $\mathbf{X}$, the velocity vector $\dot{\mathbf{X}}$, and eccentricity $\rho$. The linear factor vector ${\mathbf{F}}_L$ includes the driving torque, load torque, gravity, and the meshing excitations due to gear error:

here, $k_{s1}$ and $k_{s2}$ represent the equivalent bending stiffnesses of shaft and bearing. $k_{t1}$ and $k_{t2}$ are the equivalent torsional stiffnesses of shaft and bearing; $c_{s1}$ and $c_{s2}$ are the equivalent bending damping of shaft and bearing; $c_{t1}$ and $c_{t2}$ indicate the equivalent torsional damping of shaft and bearing.

3.1. Model validation

To validate the accuracy of the model in this paper, the vibration responses are shown in figure 2 with the comparison of the reference [32]:

In this section, the two models are analyzed with the same parameters. The related dynamic responses are obtained from the he reference (Fig. 2 (a) and (b)) and the presented model (Fig. 2 (c) and (d)). It can be found that the vibration displacement increases and the fluctuation intensifies in the presented model in this paper, which is caused by the influences of the eccentricity, backlash and coupled external/internal excitation. In addition, the frequency multiplication 2$f_m$, 3$f_m$, etc. exist in the presented model. It is clear that the frequencies and amplitudes are different for the two models.

Fig. 2Vibration responses of two models a), c) (reference [32]); b), d) (presented model)

According to Fig. 2, it can be seen that the dynamic responses of the presented model in this paper is more realistic than that of the model in reference [32]. As mentioned previously, the main difference is that the model considers coupled lateral-torsional vibration, eccentricity, etc. Throughout the numerical simulation, the influences of different system parameters on the dynamic responses are investigated for the coupled system.

3.2. Analysis of the effect of the rotational speed $\omega$

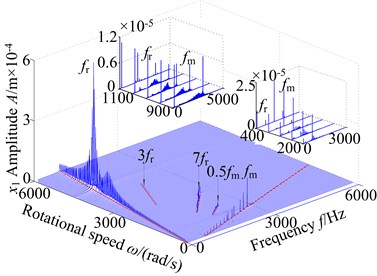
In practical spur gear system, the rotational speed is a commonly used as a control parameter which governs the static positioning of the gears, and other parameters are the same. The dynamic responses of the coupled spur gear system are investigated by using the $\omega$ as a 3-D frequency spectrum and bifurcation diagram control parameter. Fig. 3 and Fig. 4 present the corresponding 3-D frequency spectrum and bifurcation diagram of the vibration system with $x$-direction and $\theta$direction respectively, which show that the rotational speed $\omega$ has a significant influence on the dynamics of the spur gear system. In this analysis, the parametric excitation is varied in the range of [200, 6000] rad/s. it can be seen from Fig. 3 that the coupled lateral-torsional vibration system displays complex dynamic characteristics with the changing rotational speed. The primary resonance in lateral/torsional direction can be observed. Due to the effect of backlash, the jump discontinuity appears. In addition, the frequency of $f_r$ is the main frequency component, and the amplitude is a nonlinear variation along with the changing rotational speed.

Fig. 33-D frequency spectrum of the coupled system: a) lateral direction, b) torsional direction

a)

b)

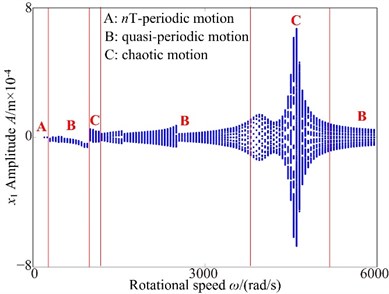
Fig. 4Bifurcation diagram using ω as control parameter: a) lateral direction, b) torsional direction

a)

b)

It can be found that the rotational frequency ($f_r=\omega /2\pi$) and meshing frequency ($f_m=z_1{f}_r$) are the predominant components in the interval [200, 400] rad/s. Frequency multiplication ($n{f}_r$, $n{f}_m$ and $n$ is a positive integer) and frequency combination ($f_m\pm n{f}_r$) components display in the corresponding 3-D frequency spectrum. However, the $f_r$, $0.5f_m$, $f_m$, $1.5f_m$, $2f_m$ components obviously exhibit and other discrete frequency components vanish as $\omega$ = 400 rad/s. Due to increased rotational speed, the $f_r$ and $f_m$ are also the dominant frequency components, and the amplitudes increase gradually. Besides, the frequency multiplication and frequency combination components are not obvious. The amplitude of $f_m$ reaches a peak value at $\omega$ = 850 rad/s, and other frequency components are lower than the meshing frequency. Besides, the obvious jump phenomena also appear. However, as $\omega$ is increased from 900 rad/s to 1150 rad/s, the 3-D frequency spectrum performs coupled phenomenon with multiple frequencies, which are concentrated distribution near $0.5f_m$ at 900 rad/s to 1050 rad/s and the multiple frequency components can be obviously found in the 3-D frequency spectrum at 1100 rad/s to 1150 rad/s. With the increasing of the rotational from 1200 rad/s to 3900 rad/s, the is the main frequency component and the amplitude of $f_r$ increases obviously. In torsional direction, the amplitude of $f_r$ reaches a peak value at $\omega$ = 3900 rad/s, which moves backward relative to the torsional primary resonance ($f_{nt}$ = 31535 Hz), which is caused by nonlinear factors such as backlash, eccentricity and so on. In addition, the 3-rotational frequency and 7-rotational frequency components are contained, but other frequency components are not obvious. In the range of [3900, 4500] rad/s, the 1-rotational frequency amplitude ($f_r$) undergoes a process of decrease first and then increase. The $f_r$ contains a response peak at $\omega$ = 4500 rad/s in addition to a response peak at $\omega$ = 3900 rad/s. which is the lateral primary resonance frequency. By further increasing the rotational speed, the frequency component is simple, the $f_r$ exists only in 3-D frequency spectrum, and amplitude of $f_r$ decreases sharply with obvious jump discontinuous phenomenon.

Fig. 3 (b) presents the 3-D frequency spectrum of the coupled spur gear transmission system in torsional direction using the rotational speed $\omega$ as a parameter. It can be seen from figure that the different dynamic characteristics appear. The torsional primary resonance appears, and the lateral primary resonance cannot be observed. Besides, the $f_r$ and $f_m$ are the dominant frequency components, where the amplitude of $f_r$ decreases gradually, the amplitude of $f_m$ decreases firstly and then increase at $\omega$ < 400 rad/s. Besides, frequency multiplication and frequency combination components appear in the corresponding 3-D frequency spectrum. As the rotational speed is increased further in the range of [500, 850] rad/s, the motion of the vibration system is relatively simple and the $f_r$ and $f_m$ are the main frequency components with different speed. Furthermore, the amplitude of $f_m$ is larger than the amplitude of $f_r$ and other components withdraw obviously. the amplitude of $f_m$ reaches to the peak at $\omega$ = 850 rad/s. As the rotational speed is further increase from $\omega$ = 900 rad/s to $\omega$ = 1050 rad/s, in addition to the $f_r$ and $f_m$, the continuous frequency components appear in the frequency spectrum. At $\omega$ = 900 rad/s the response amplitude of $f_m$ drops causing an abrupt jump down in the 3-D frequency response. Finally, at higher values of $\omega$, i.e. $\omega$ ≥ 1100 rad/s, the $f_r$ reaches to peak at $\omega$ = 3900 rad/s, which is the torsional primary resonance, and the peak appears backwards phenomenon. In addition, the $0.5f_m$ (at $\omega$ = 1100 rad/s-2300 rad/s), $5f_r$ (at $\omega$ = 1100 rad/s-1500 rad/s) and $7f_r$ (at $\omega$ = 1600 rad/s-2400 rad/s) obviously exist in the 3-D frequency spectrum. Analyzing the results of this section, the increases of the rotational speed can decrease the nonlinearity degree of the coupled lateral-torsional spur gear system.

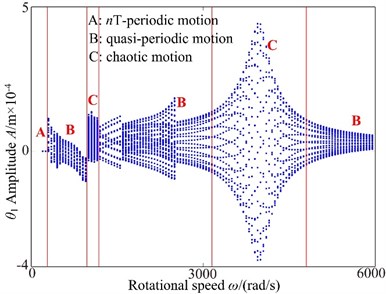
In Fig. 4(a), it can be observed that the spur gear system undergoes periodic motion, quasi-periodic motion to chaotic behavior and then returns to quasi-periodic. As shown in Fig. 4(a), the bifurcation diagram exhibits periodic-1 motion at $\omega$ ≤ 250 rad/s. When $\omega$ increases from 300 rad/s to 850 rad/s, periodic-1 motion is replaced by quasi-periodic motion and performs jump phenomena at $\omega$ = 850 rad/s. By further increasing the rotational speed in the range of [900, 1100] rad/s, the coupled spur gear system exhibits slight chaotic motion, which is a corresponding phenomenon with continuous frequency in 3-D frequency spectrum. As increases $\omega$, the gear system undergoes quasi-periodic motion and chaotic motion in a rotational speed 1150 rad/s < $\omega$ < 5000 rad/s. However, $\omega$ greater than 5000 rad/s, chaotic motion transits to quasi-periodic motion.

In torsional direction, in the interval [200, 6000] rad/s, it can be found from Fig. 4(b) that the motion of the coupled system undergoes periodic-$n$, quasi-periodic and chaotic motions. When $\omega <$850 rad/s, 1$T$-periodic motion is replaced by quasi-periodic motion, and occurs jump phenomena at $\omega =$850 rad/s. When $\omega$ increases from 900 rad/s to 3200 rad/s, the bifurcation diagram shows that the gear system performs chaotic motion transits to $nT$-periodic motion and then quasi-periodic motion. At higher values of the $\omega =$3250 rad/s-6000 rad/s, the dynamical behavior of the spur gear system is found to be chaotic motion and then quasi-periodic motion. From these results, it can be seen that the spur gear system undergoes a different motion as the rotational speed is increased over the range $\omega =$200 rad/s-6000 rad/s.

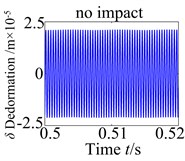
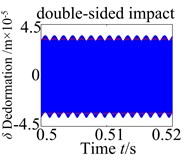
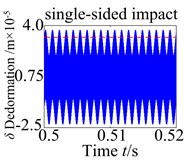
Fig. 5Tooth impact of the spur gear system u with changing ω

a)$\omega =$850 rad/s

b)$\omega =$3850 rad/s

c)$\omega =$4500 rad/s

d)$\omega =$6000 rad/s

In order to detailedly study the influences of the rotational speed, the tooth impact is examined next. It may be also noted that tooth impact may occur because of the gear backlash nonlinearity. There are three possible types of tooth impact cases: no tooth impact (${\delta }_{max}<b$ and ${\delta }_{min}>-b$), single-sided tooth impact (${\delta }_{max}>b$ and ${\delta }_{min}>-b$ or ${\delta }_{max}<b$ and ${\delta }_{min}<-b$) and double-sided tooth impact (${\delta }_{max}>b$ and ${\delta }_{min}<-b$). The various case of tooth impacts can be observed in the time history plots at $\omega =$ 850 rad/s, 3850 rad/s, 4500 rad/s, and 6000 rad/s, respectively. It can be seen from Fig. 5 that there is obviously no tooth impact at low values of the rotational speed, i.e., $\omega =$850 rad/s. Furthermore, as rotational speed increase to 3850 rad/s, which is the torsional primary resonance frequency, the double-sided tooth impact can be clearly observed, and it is the dominant response. As expected, the double-sided tooth impact behavior can be seen occurring around the primary resonance for $\omega =$4500 rad/s. However, when rotational speed is increased to 6000 rad/s, the single-sided tooth impact is the dominant response in the spur gear system. Hence, it can be concluded that the rotational speed has a significant influence on the dynamic behaviors of the coupled lateral-torsional spur gear system.

3.3. Analysis of the effect of the backlash $\mathit{b}$

Gear backlash is an important parameter effect on the dynamic behaviors of the gear system and due it has a strong nonlinear character, which may be designed for better lubrication and to reduce interference, or caused by manufacturing and installing errors. Therefore, the influence of backlash on the dynamic responses is very complex. In order to analyze more deeply, the dynamic characteristics of the coupled gear system is investigated using the backlash $b$ as a 3-D frequency spectrum and bifurcation control parameter in lateral and torsional directions. A transition from simple motion to complex motion and then simple motion dynamical behaviors will be seen when $b$ is increased from 2×10^-5m to 1.0×10^-4m.

Fig. 63-D frequency spectrum of the coupled system: a) lateral direction, b) torsional direction

a)

b)

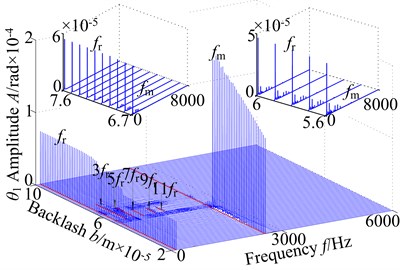
Fig. 6(a) displays the corresponding 3-D frequency spectrum in lateral direction with $b$ as control parameter. It can be seen that the $f_r$ and $f_m$ appear simultaneously and the meshing frequency ($f_m$) is the dominant frequency component, which is greater than other components at $b$ ≤ 5.0×10^-5 m. Besides, the amplitude if $f_m$ have a peak value $b$ = 4.8×10^-5 m, and then the amplitude of $f_m$ exhibits an obvious jump phenomenon at $b$ = 5.0×10^-5 m. The frequency multiplication and frequency demultiplication components do not appear. In the range of [5.3×10^-5, 5.6×10^-5] m, the complicated continuous frequency components appear in lateral direction, which indicates that the coupled spur system displays chaotic motion. By further increasing the backlash $b$, the frequency multiplication ($3f_r$, $5f_r$, $7f_r$, $9f_r$, $11f_r$,) and meshing frequency $f_m$ appear obviously, and the amplitudes decrease gradually in the interval [5.7×10^-5, 6.1×10^-5] m. As the $b$ is increased slightly, the coupled gear system displays complicated higher harmonic components at 6.2×10^-5 m < $b$ < 7.5×10^-5 m. In addition, the $f_r$ and $f_m$ are the main frequency components. When $b$ > 7.6×10^-5 m, the amplitudes of $f_r$ and $f_m$ keep almost unchanged, and other frequency components vanish gradually.

The corresponding 3-D frequency spectrum in torsional direction is shown in Fig. 6(b), it can be found that the amplitudes of $f_r$ and $f_m$ are obvious greater than those in lateral direction. In the range of [2.0×10^-5, 5.0×10^-5] m, the amplitude of $f_r$ is a constant value, and the $f_m$ increases obviously, which reaches the peak value closing to $b$ = 4.8×10^-5m. As the backlash is increased in the interval [5.1×10^-5, 7.5×10^-5] m, the coupled system appears continuous frequency components in the range of [5.1×10^-5, 5.7×10^-5] m, though frequency multiplication components ($3f_r$, $5f_r$, $7f_r$, $9f_r$, $11f_r$, $f_m$) at 5.7×10^-5 m < $b$ < 6.1×10^-5 m, to return to continuous frequency components in the interval of [6.2×10^-5, 7.5×10^-5] m. Finally, in the range of [7.6×10^-5, 10.0×10^-5] m, the $f_r$ is the main frequency component, and the $f_m$ and other frequency components vanish gradually.

Fig. 7Bifurcation diagram using b as control parameter: a) lateral direction, b) torsional direction

a)

b)

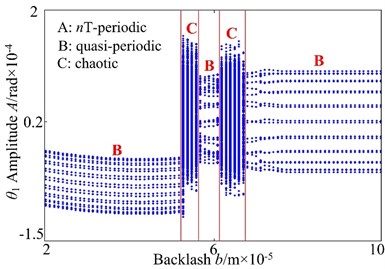
Fig. 7(a) presents the bifurcation diagram of the spur gear system using parameter $b$ as control parameter. In Fig. 7(a), when backlash $b$ is increased, it can identify the system exhibits a sequence of $nT$-period motion, quasi-periodic motion and chaotic response. By analyzing the 3-D frequency spectrum, some key points are selected, which indicate spur gear system motions have been changed with increasing backlash $b$. The spur gear system undergoes quasi-periodic motion at low backlash when $b$ increases from 2.0×10^-5 m to 5.1×10^-5 m. As $b$ increase from 5.2×10^-5 m to 5.5×10^-5 m, the system exhibits chaotic motion through quasi-periodic motion. At higher values of the backlash $b$, the dynamic behavior of the spur gear system is found to be quasi-periodic dynamic behavior at $b=$5.6×10^-5 m-6.1×10^-5 m, and then transits to chaotic motion at $b=$6.2×10^-5 m-6.7×10^-5 m, while the much higher vibration occurs. Finally, $b>$6.8×10^-5 m, the quasi-periodic motion is replaced by $nT$-periodic motion.

The bifurcation diagram for the torsional vibration of the driving gear is shown in Fig. 7(b). It is noted that similar behavior is observed by torsional vibration and lateral vibration. In this section, it only presents a simple description for the bifurcation diagram. When backlash increases from 2.0×10^-5 m to 5.1×10^-5 m, it can be noted that the gear shows quasi-periodic motion. When backlash is further increased, it can see the gear system exhibits a sequence of complicated motion state, the dynamic behavior of the system is found to be chaotic motion at $b=$5.2×10^-5 m to 5.5×10^-5 m, quasi-periodic motion at $b=$5.6×10^-5 m to 6.1×10^-5 m, and then the gear system reverts to a chaotic motion once again at 6.2×10^-5 m to 6.7×10^-5 m. However, as $b$ is greater than 6.8×10^-5 m, the chaotic motion transits to quasi-periodic motion.

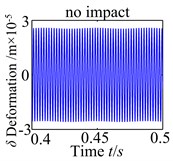
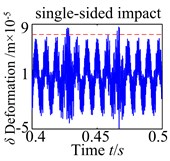
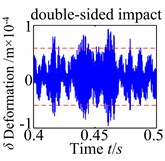
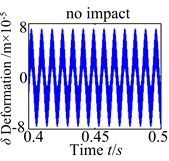
For a better clarity the influence of the backlash $b$, the dynamic behaviors are corresponding with no tooth impact, single-sided tooth impact and double-sided tooth impact as shown in Fig. 8. It investigates four backlash conditions chosen ($b=$3.0×10^-5 m, $b=$5.3×10^-5 m, $b=$7.5×10^-5, $b=$10×10^-5 m), at the low backlash $b$ range ($b=$3.0×10^-5 m), the spur gear behaves have no complicated nonlinearity with no evidence of tooth impact. As the $b$ increases, the characteristics indicate the influence of strongly backlash nonlinearity causing single-sided tooth impact ($b=$5.3×10^-5) and double-sided tooth impact ($b=$7.5×10^-5 m), which have no obvious periodicity and the vibration of the system is in the unsteady state. However, as $b$ is increased further, the single-sided tooth impact and double-sided tooth impact vanish gradually. The spur gear response changes back to no tooth impact.

Fig. 8Tooth impact of the spur gear system with changing b

a)$b=$3.0×10^-5 m

b)$b=$5.3×10^-5 m

c)$b=$7.5×10^-5 m

d)$b=$10×10^-5 m

3.4. Analysis of the effect of the error fluctuation ${\mathit{e}}_{\mathit{r}}$

The transmission error caused by manufacturing error and assembly error, which is a displacement excitation and has an important influence of the dynamic response for the coupled system. To illustrate the influence on the spur gear system of the error fluctuant amplitude $e_r$, a further analysis has been carried out considering several set of simulated of the error fluctuant amplitude. Fig. 8-9 present the 3-D frequency spectrum and bifurcation of the system using $e_r$ as a control parameter, and the values of the other parameters remain unchanged.

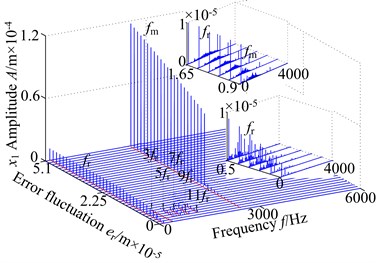
Fig. 93-D frequency spectrum using er as control parameter: a) lateral direction, b) torsional direction

a)

b)

The 3-D frequency spectrum of the coupled gear system in lateral direction with error amplitude $e_r$ is displayed in Fig. 9(a). In a light value of $e_r$, the rotational frequency $f_r$, the frequency multiplication ($f_r$, $n{f}_r$, $f_m$, $n{f}_m$) and frequency combination ($n{f}_m\pm n{f}_r$) appear in the range of [0, 1.05×10^-5] m. In addition, the amplitude of $f_r$ is the maximum value and other amplitudes are lower than the $f_r$. Further increase of the error amplitude in the interval [1.2×10^-5, 5.1×10^-5] m, the amplitude of $f_r$ increases gradually, and the amplitude of $f_m$ becomes large and occurs jump phenomenon suddenly with the increasing error amplitude. Besides, the frequency multiplication and frequency combination components vanish obviously.

Fig. 9(b) shows the 3-D frequency spectrum in torsional direction with error amplitude as control parameter in the range of [0, 5.1×10^-5] m. At low error amplitude at $e_r$ < 1.05×10^-5 m, the $f_r$ and the frequency multiplication components are obvious. The amplitude of $f_m$ is not observed. With the increasing of error amplitude, the amplitude of $f_r$ decreases slightly, and the amplitude of $f_m$ presents diametrically opposite nature and the amplitude has an obvious jump discontinuity unexpectedly at $e_r$ = 1.2×10^-5 m. Increasing the error amplitude even further, the amplitude of $f_r$ keeps approximately the same magnitude, and the amplitude of $f_m$ increasing gradually.

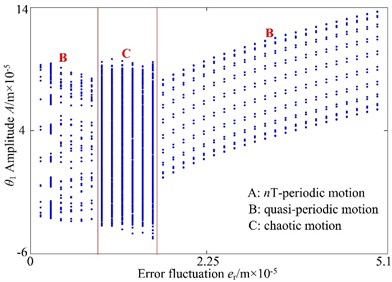
Fig. 10Bifurcation diagram using er as control parameter: a) lateral direction, b) torsional direction

a)

b)

For a better understanding the trend of motion with the error fluctuation, Fig. 10(a) presents the bifurcation diagram using the fluctuation $e_r$ as control parameter in lateral direction. As $e_r$ increases from 0 to 0.9×10^-5 m, the spur gear system presents quasi-periodic motion. However, as $e_r$ is increased from 1.05×10^-5m to 1.8×10^-5 m, the spur gear system exhibits chaotic motion through quasi-periodic motion, which is mainly due to the effect of the backlash. Finally, as the fluctuation $e_r$ is further increased, i.e., $e_r>$1.95×10^-5 m, the chaotic motion is replaced by quasi-periodic motion.

In torsional direction, the effect of the parameter $e_r$ is illustrated in the bifurcation diagram as shown in Fig. 10(b). It can be observed that the gear system exhibits quasi-periodic motion at low values of the fluctuation, i.e., $e_r<$0.9×10^-5 m and then the chaotic motion can be found as the fluctuation is increased over $e_r=$1.05×10^-5 m. At higher values of the fluctuation, i.e., $e_r>$1.95×10^-5 m, the dynamic behaviors of spur gear show quasi-periodic motion.

Fig. 11Tooth impact of the spur gear system with changing er

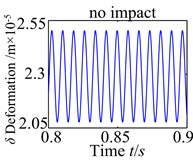
a)$e_r=$0

b)$e_r=$1.05×10^-5 m

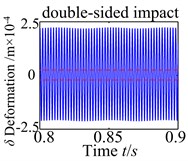
c)$e_r=$1.8×10^-5 m

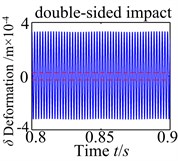
d)$e_r=$5.1×10^-5 m

From the results, under different error fluctuation $e_r$ conditions, as show in Fig. 11, the dynamic responses show that no tooth impact, single-sided tooth impact and double-sided tooth impact are demonstrated in the spur gear. There is obviously no tooth impact at lower fluctuation values. As $e_r$ increases to 1.05×10^-5m, this type of characteristic indicates the effect of transmission error fluctuation causing single-sided tooth impact. The tooth impact continues as the fluctuation increases until double-sided tooth impact begins to show up. When $e_r$ increases to 5.1×10^-5m, the double-sided tooth impact behavior remains dominant response. These results implicate that the fluctuation of the transmission error $e_r$ tends to worse the degree of the nonlinearity.