Study on the vibration characteristics of structural of hydrocyclone based on fluid structure interaction

2.1. The geometric model

The hydrocyclone of main diameter 28 mm with a typical variable diameter pipe structure was selected as the research object, and materials for organic glass, structure of operation, both ends of the pipes are fixed, Considering the vibration of the structure of the hydrocyclone in the internal fluid pulsation, and the structure and the turbulent flow in the pipe form a fluid structure coupling system. Its mechanical model is shown in Fig. 1.

Fig. 1The fluid structure interaction mechanics model of variable diameter pipe

2.2. FSI calculation model and method

Fluid elements of the hydrocyclone with variable diameter pipes satisfy the conservation of mass and momentum equations.

Continuous equation is:

The momentum conservation equation is:

The balance equation of solid part is:

Fluid-structure interaction interface equation is:

here, the temperature change of the fluid in the hydrocyclone was not considered, and the force and displacement are equal in the fluid and the solid interface needs to be satisfied, respectively.

Where, $a_s$ is local acceleration vector of solid (m/s²), $f_f$ and $f_s$ are volume force vector of fluid and solid (N), respectively. ${\rho }_f$ and ${\rho }_s$ are density of fluid and solid (Kg/m³), respectively. $u$ is velocity vector of fluid, ${\sigma }_s$ is Cauchy stress tensor (N), ${\tau }_f$ is interface stress of fluid (N), $s_f$ and $s_s^{}$ are interface displacement of fluid and solid (m).

The inherent characteristics of the structure under the coupling action are studied, and the effect of the fluid pressure on the structure is considered. Without considering the influence of the external force and the damping on the system, the dynamic equation of the hydrocyclone can be changed into:

Here, let us suppose that is:

Then the Eq. (6) is changed to:

where, $\left[M_a\right]$ is a coupled mass matrix.

Let’s assume that:

where, $\{\delta {\}}_i$ is the solution of Eq. (8);${\left\{\varphi \right\}}_i$ is the first order modal vibration mode vector; ${\omega }_i$ is the $i$ order natural frequency of the modal (rad/s).

The Eq. (9) into Eq. (8) to:

The necessary and sufficient conditions for the existence of nonzero solutions of the Eq. (10) are:

The $i$ order natural frequency of the output:

In this way, we can get the modal parameters of hydrocyclone under FSI.

In the numerical calculation method, the fluid field using the finite volume method, the Reynolds stress turbulence model of turbulence is chosen for the calculation of fluid model, and the method of ALE is adapted to for fluid dynamic mesh. Based on the finite element theory with hypothesis of small deformation, the Newmark time integration method is used to solve the dynamic equation of variable diameter pipe structure in solid domain. Using alternating iterative coupling solving method, the two-way FSI calculation model of hydrocyclone with variable diameter pipe is established. Inlet boundary conditions are chosen by the entrances of hydrocyclone, and outlet boundary conditions are adopted by underflow and overflow of hydrocyclone.

3.1. The instruments and equipment of test

Fig. 2 shows hydrocyclone entity of experimental test, the pressure electric sensor was installed directly in the hydrocyclone with variable diameter pipe, the acceleration sensors of PCB ICP were adopted. Vibration signal was obtained using LMS channel 24 SCADAS mobile data acquisition system and signal analysis using analysis software of LMS. Test Lab. The vibration waveform is shown in Fig. 3.

Fig. 2Entity structure of hydrocyclone

Fig. 3Signal gathering analytical instruments

3.2. Experimental parameters

The experiment was carried out with hydrocyclone of organic glass materials under three kinds of conditions, namely: without medium, full of medium and operating condition with three kinds of flow velocity. The test schemes were shown in Table 1.

3.3. Arrangement of measuring points

Ten measuring points were arranged on the hydrocyclone, the position of measuring points as shown in Fig. 4. Considering the geometric model in circular symmetry, the structure is simplified into a bar, and measuring points were measured in the same section position of the $X$ and $Y$ axes direction.

Fig. 4Measuring point distribution on variable diameter pipe

3.4. Test method

According to modal theory to determine the vibration characteristics of the hydrocyclone with variable diameter pipe structure, need to measure the transfer function. The way of single input single output was adopted in this test. The forces acting on the structure depended on the action of pulse hammer or flow medium, and the response of each point was measured, namely, the structure of hydrocyclon with conditions of without medium and full of medium but not flow was applied exciting force by hammer, and the structure with operating condition was applied impact force by fluid. Then the measured signal was processed and analyzed, and the transfer function between the excitation point and the response point was calculated.

In the test device shown in Fig. 2, the foundation of the structure is fixed on the ground, and the admittance of the connection point is zero. According to the vibration theory, influence of low order modal of vibration of the structure, is bigger than higher order modal can be ignored, and the influence of higher order modal can be neglected. So the frequency ranges of 0-500 Hz, and frequency resolution precision is 0.5 Hz.

4.1. Experimental modal analysis under the condition of no medium

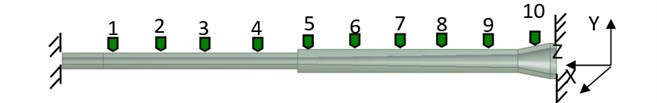
The output signal of ten measuring points of hydrocyclone without medium were measured. Modal identification state diagram as shown in Fig. 5, peaks and troughs of wave of two curves overlap together and at the intersections have a list of S and V letters, S denote that frequency, damping and vector of the pole are stable, V denote that vector is stable, O denote that the noise is presence in this location, the curve of troughs of wave denote imaginary frequency curve, and the curve of peaks of wave denote real frequency curve. The frequency range of 0-500 Hz are shown in in Fig. 5, and we can see the first three order modal identification are clear, the position of peaks and troughs of wave of two curves overlap together are natural frequency of the hydrocyclone structure.

Fig. 5The modal identification state diagram of hydrocyclon

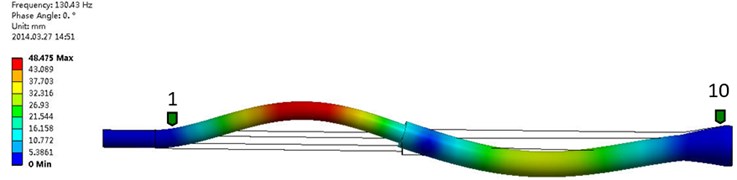
The experimental test and numerical simulation were carried out under the same conditions of no medium, and results of experimental test and numerical simulation were compared and analyzed. The first two natural frequencies and model shape of the hydrocyclone structure were given from Fig. 6 to Fig. 9. The first three natural frequencies and model shape of the hydrocyclone structure were given in table 2. As can be seen from the chart, the natural frequency and model shape of the hydrocyclone structure between numerical simulation and experimental test were similar, the relative error increased with the increase of the order, the error of the three order was the maximum, and the relative error was 8.8 %.

Based on the measured and simulated results, we could have got that measure point 4 and measure point 5 were greatly deformation in the first-order model shape, where is the small conical section of hydrocyclone structure near the junction with the tail section. The deformation of measure point 3 was larger in second-order model shape; this location is near the middle of the tail pipe section.

Fig. 6The first order frequency and modal shape of experimental test (Mode 1: 41.6534 Hz, 4.31 % AMPS)

Fig. 7The first order frequency and modal shape of numerical simulation

Fig. 8The second order frequency and modal shape of experimental test (Mode 2: 142.7441 Hz, 5.17 % AMPS)

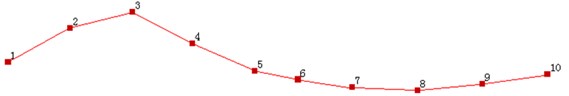
Fig. 9The second order frequency and modal shape of numerical simulation

4.2. Experimental modal analysis under full of medium

In the hydrocyclone, the water was filled and carried on the modal test analysis, and the natural frequency and the model shape of experimental test and numerical simulation were shown in Figs. 10-13 and Table 3. As can be seen from the figure and table, the forms of movement between experimental test and numerical simulation were similar, but the value of the structure with full of medium was lower than natural frequency of without medium, the frequency of experimental test from first order to three order were reduced 2.76 Hz, 12.2 Hz and 6.54 Hz, respectively. And the frequency of numerical simulation from first order to three order were reduced 3.24 Hz, 9.28 Hz and 17.67 Hz, respectively. graphs and tables. This is mainly due to the result in the presence of the internal fluid added mass of the structure.

According to program of Table 1, the modal test analysis of the structure of the hydrocyclone was carried out with inlet velocity of 6.82 m/s, 8.17 m/s and 10.2 m/s, respectively. At the same time the results of numerical simulation were obtained base on fluid structure interaction, and the results were compared between the experimental test and the numerical simulation. Figs. 14-19 showed the first three order model of the inlet velocity of 6.82 m/s. The results of the first three order natural frequencies and the relative error of the different inlet velocity were given in Table 4.

Fig. 10The first order frequency and modal shape of experimental test (Mode 1: 38.8890 Hz, 3.99 % AMPS)

Fig. 11The first order frequency and modal shape of numerical simulation

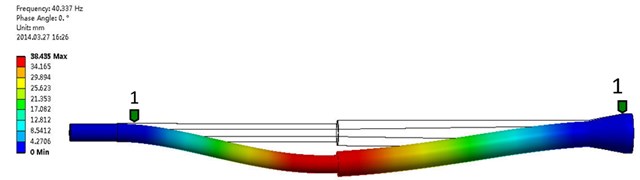
Fig. 12The second order frequency and modal shape of experimental test (Mode 2: 130.5435 Hz, 5.87 % AMPS)

Fig. 13The second order frequency and modal shape of numerical simulation

As can be seen from the chart, the forms of movement between experimental test and numerical simulation were similar. As the inlet velocity increases, the natural frequency is slightly lower, but the range of reduction is smaller. This is mainly in the process medium flows, under the effect of fluid-structure interaction, the force of fluid acted on the structure of hydroclone and embodied by the prestressing form. Under the experimental conditions of range of inlet velocity, the error value of natural frequency of first order between the experimental test and numerical simulation is minimized, while the error value of natural frequency of third order is maximum and maximum is 17.1 %.

Fig. 14The first order frequency and modal shape of experimental test (Mode 1: 36.4213 Hz, 0.11 % AMPS)

Fig. 15The first order frequency and modal shape of numerical simulation

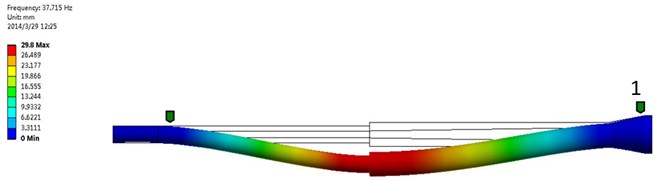
Fig. 16The second order frequency and modal shape of experimental test (Mode 2: 132.4906 Hz, 4.78 % AMPS)

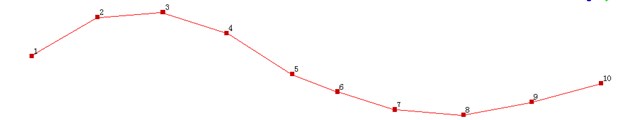
Fig. 17The second order frequency and modal shape of numerical simulation

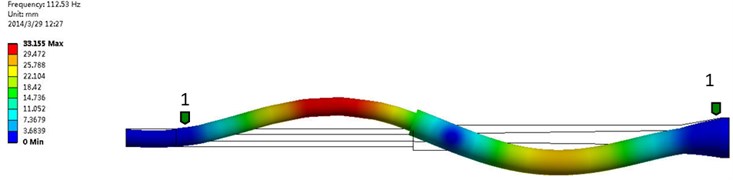
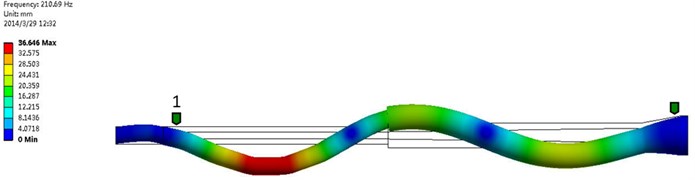
Fig. 18The third order frequency and modal shape of experimental test (Mode 3: 251.1038 Hz, 3.28 % AMPS)

Fig. 19The third order frequency and modal shape of numerical simulation