Differences of dynamic behaviors of face gear drives between time varying and average mesh stiffness

1. Introduction

Face gear drives, due to insensitive manufacture and alignment errors versus spiral bevel gear drives [1-5], are addressed by many scholars, and some research achievements of face gear dynamics are obtained by scholars [6-11]. However, according to the limited published issues, differences between the impact of time varying mesh stiffness on dynamic mesh forces of face gear drives and that of average mesh stiffness on dynamic mesh forces are not to be discussed. Thus, in this study, a mesh stiffness calculation solution of face gear drives, based on the proposed equivalent face gear teeth, is constructed, and a four DOF dynamic model of face gear drives is established. The differences of dynamic behaviors of face gear drives between time varying and average mesh stiffness are investigated. The result indicates an instantaneous impact is existed at start engagement points of face gear drives.

2. Constructed mesh stiffness calculation solution and four DOF dynamic model

2.2. Four DOF dynamic model

A four DOF dynamic model of face gear drives, as given in Fig. 2, is established for evaluating differences of face gear dynamic behaviors between time varying and average mesh stiffness.

Fig. 1An evolution diagram of equivalent face gear teeth

Fig. 2A four-DOF dynamic model of face gear drives

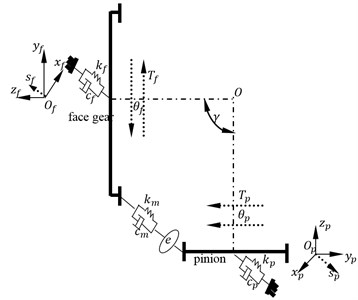
As shown in Fig. 2, mathematic equations of the dynamic model can be derived by:

1

$\left\{\begin{array}{l}{m}_p{s}_p^{\text{'}\text{'}}+c_p{s}_p^{\text{'}}+k_p{s}_p=-F_m,\\ m_f{s}_f^{\text{'}\text{'}}+c_f{s}_f^{\text{'}}+k_f{s}_f=F_m,\\ I_p{\theta }_p^{\text{'}\text{'}}+F_m{R}_{bp}=T_p,\\ I_f{\theta }_f^{\text{'}\text{'}}+F_m{R}_{bf}=-T_f,\end{array}\right.$

where $F_m$ can be expressed as:

2

$F_m=k_{m}sin\left(\gamma \right)\left(s_p-s_f+R_{bp}{\theta }_p-R_{bf}{\theta }_f-e\right)$
$+c_{m}sin\left(\gamma \right)\left(s_p^{\text{'}}-s_f^{\text{'}}+R_{bp}{\theta }_p^{\text{'}}-R_{bf}{\theta }_f^{\text{'}}-e\text{'}\right),$

where $\theta$ is a torsion degree of freedom, $s$ is a bending degree of freedom, $T$ is a torsion, $k$ is a bending stiffness, $c$ is a bending damping, $m$ is a quality, $I$ is a moment of inertia, ($\text{'}$) is first derivative, ($\text{'}\text{'}$) is second derivative, subscript $f$ and $p$ express a face gear and a pinion respectively. In addition, $k_m$ is mesh stiffness, $c_m$ is mesh damping, and $e$ is a comprehensive meshing error.

3. Simulations

In order to assess differences between the impact of time varying mesh stiffness on dynamic mesh forces and that of average mesh stiffness on dynamic mesh forces, geometric parameters, operating conditions and material characteristics of an example case of face gear drives are given in Table 1.

According to the proposed mesh stiffness calculation solution, and the parameters listed in Table 1, the time varying mesh stiffness and average mesh stiffness of the example case are simulated, as shown in Fig. 3. Moreover, the differences of dynamic mesh forces of face gear drives of the example case between time varying and average mesh stiffness, as shown in Fig. 4, are discussed.

Fig. 3Mesh stiffness simulated

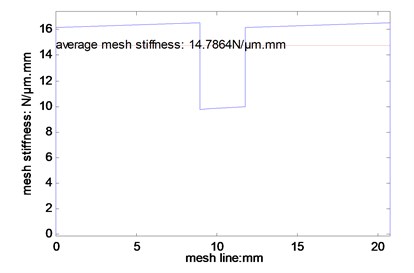
Fig. 4A diagram of the simulated differences

In the case of Fig. 4, a zero frequency incentive of dynamic mesh forces, which is the most important difference of dynamic behaviors between time varying and average mesh stiffness, is obtained. The result indicates a transitory shock at start engagement points of face gear drives is existed. Except the above phenomenon, the other differences almost can be neglected according to the simulation.

4. Conclusions

In this study, two important works can be extracted as follows:

1) A mesh stiffness calculation solution of face gear drives is constructed, which is based on the proposed equivalent face gear teeth.

2) Differences of dynamic behaviors of face gear drives between time varying and average mesh stiffness are obtained, and the result indicates an instantaneous impact is existed at start engagement points of face gear drives.

These contributions would be helpful to improve engineering applications of face gear drives.