Dynamic characteristics of magnetorheological fluid lubricated journal bearing and its application to rotor vibration control

2.1. Fundamental equations

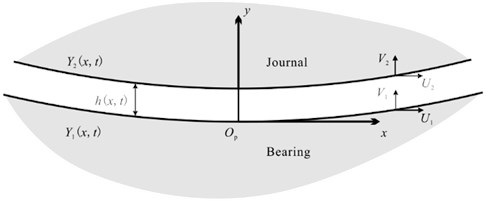
In order to simplify analysis and calculation, (1) the MR fluid is assumed to be incompressible; (2) body forces such as gravity are ignored, and (3) variables (pressure, strain, stress, etc.) along axial direction are treated as constant. Under these assumptions, in the oil film coordinate system shown in Fig. 1, continuity and momentum equations of the lubricants can be written as:

where $u(x,y)$ is the velocity profile, $P\left(x\right)$ is the fluid pressure, $\pi (x,y)$ is the shear stress, and $x$, $y$ are directions defined in the oil film illustrated in Fig. 1. Boundary conditions are:

in which subscripts 1 and 2 denote the inner surface of the bearing and the surface of the shaft, respectively, and $Y(x,t)$ is the $y$ coordinate of the boundary surface.

Fig. 1Geometry of part of the oil film of a journal bearing

Integrating the momentum equation with respect to $y$, the following equations are obtained:

On the other hand, integrating the momentum equation from surface 1 to surface 2, the relationship between ${\tau }_1$ and ${\tau }_2$ can be found:

where $h(x,t)$ is the thickness of the gap between the two surfaces. Eqs. (1), (3) and (4) apply to both liquid and core regions.

The Herschel-Bulkley model can be represented as:

where $\tau$ is the shear stress, ${\tau }_0$ is the yield stress, $\dot{\gamma }$ is the shear rate, and $K$, $n$ are model constants. If $n=\text{1}$, Eq. (4) becomes the expression of Bingham model with $K$ the viscosity; if $n>\text{1}$, the fluid is shear thickening; if $n<\text{1}$, the fluid is shear-thinning. According to Eq. (5), in the pre-yield region, $\tau$ is multi-valued, which may introduce mathematical difficulties. Hewitt and Balmforth [6] introduced a way to avoid this by using the inverse of Eq. (5):

where ${\left(\bullet \right)}_{+}$ indicates $\mathrm{m}\mathrm{a}\mathrm{x}\left(\bullet ,0\right)$. In the present work, this method is adopted, and on previous assumptions, $\dot{\gamma }=\partial u/\partial y$.

In order to simplify the inclusion of HB model into the momentum equation, the following dimensionless form of variables are introduced:

Then Eqs. (3), (4) and (6) can be transformed into dimensionless form as:

wherein $B$ is the Bingham number defined as the ratio of yield stress to viscous stress. In the present model, $B$ takes the form:

Integrating Eq. (10) using boundary conditions Eq. (2) leads to:

Integrating continuity equation in Eqs. (1) with respect to $y$ then to $x$, the following equation is obtained:

where $Q$ is flux at $\stackrel{-}{x}=0$, and:

Eq. (12) and Eq. (13) constitute a pair of algebraic equations to solve for ${\tau }_1$ and ${\tau }_2$ given $h$, $U$, $V$ and $Q$. In the problem of hydrodynamic bearing, however, $Q$ is usually not prescribed, so more equations are needed to close this problem.

2.2. Oil film boundary condition

The following periodic condition is usually implemented as the oil film boundary condition:

where integral region $D$ is the whole circumference. This is essentially the Sommerfeld boundary condition, which has been proved to be far from reality because the oil film cannot sustain large negative pressure due to cavitation. In the present work, a more reasonable Reynolds boundary is applied. Reynolds boundary states that at some point in the oil film (somewhere near the minimum oil film thickness), the pressure gradient and pressure reduce to zero simultaneously, and the negative pressure region is simply set to zero pressure. The Reynolds boundary condition, therefore, can be represented as:

in which ${\stackrel{-}{x}}_0$ denotes the point at which both pressure and pressure gradient are zero. Eqs. (16) introduce two new equations and a new unknown variable ${\stackrel{-}{x}}_0$. Now Eqs. (12), (13) along with Eqs. (16) can be solved for ${\stackrel{-}{\tau }}_1$, ${\stackrel{-}{\tau }}_2$, $Q$ and ${\stackrel{-}{x}}_0$.

2.3. Bearing geometry

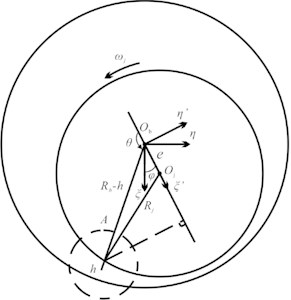
The variation of oil film thickness is crucial for the formation of hydrodynamic pressure. With journal center eccentricity $e$, the film thickness $h$ takes the form:

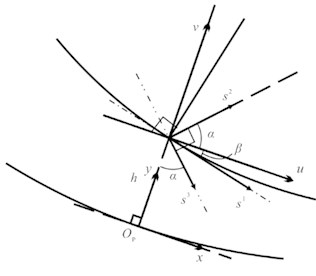
At each position in $x$ direction, a local coordinate system is established on the stationary surface of the bearing, as illustrated in Fig. 1. In this coordinate system, $U_1=0$ and $V_1=0$. A more detailed sketch of geometric relations between journal and bearing is shown in Fig. 2 for deduction of $U_2$ and $V_2$. Velocity at every point on surface 2 is composed by three parts $s^1$, $s^2$ and $s^3$ that take the form:

the physical meaning of which are velocity due to journal rotation, attitude angle change (journal precession) and eccentricity change, respectively. Considering geometric relations, expressions of $U_2$ and $V_2$ at every point on the journal circumference are:

where:

Fig. 2a) Bearing geometry with journal speed ωj, eccentricity e, b) is the detailed view of the oil film geometry corresponding to Fig. 1

a)

b)

After nondimensionalizing quantities in Eq. (7), Eqs. (19) are substituted into Eq. (12), (13) and (16) to solve for the pressure distribution in the oil film.

2.4. Oil film force and dynamic coefficients

Applying the Reynolds boundary condition, oil film force is simply calculated with integral from $\stackrel{-}{x}=0$ to ${\stackrel{-}{x}}_0$. For simplicity, oil film force and dynamic coefficients are first calculated in coordinate system $\xi \text{'}{O}_b\eta \mathrm{\text{'}}$ and then transformed to the fixed bearing coordinate system $\xi O_b\eta$. Relative positions of the two systems are illustrated in Fig. 2. In the $\xi \text{'}{O}_b\eta \mathrm{\text{'}}$ system, oil film forces are obtained from pressure integrals:

where ${\stackrel{-}{B}}_L$ is the dimensionless length of the bearing. Stiffness and damping coefficients in the $\xi \text{'}{O}_b\eta \mathrm{\text{'}}$ system are calculated by definition:

where $\dot{\phi }$ is the rate of change of the attitude angle $\phi$, and is equal to the precession rate ${\omega }_p$. $\xi \text{'}{O}_b\eta \mathrm{\text{'}}$ system leads $\xi O_b\eta$ system by angle $\phi$, so $K_{\xi \mathrm{\text{'}}{O}_b\eta \mathrm{\text{'}}}$ and $C_{\xi \mathrm{\text{'}}{O}_b\eta \mathrm{\text{'}}}$ are transformed to $K_{\xi O_b\eta }$ and $C_{\xi O_b\eta }$ in the following way:

where $T$ is the transformation matrix.

3.1. Static characteristics

Equations listed above are solved numerically. First, Eqs. (12) and (13) are solved for ${\tau }_1$ and ${\tau }_2$ using assumed flux $Q$. Then $Q$ is solved iteratively based on Reynolds boundary condition Eqs. (16), where ${\stackrel{-}{x}}_0$ is the point at which ${\stackrel{-}{\tau }}_1={\stackrel{-}{\tau }}_2$. Every value of $Q$ is used to solve Eqs. (12) and (13) in the first step and the iteration goes on. When values all four unknowns are converged, the static condition of the bearing is determined. Investigation of bearing with constant load is also carried out by finding out suitable eccentricity and attitude angle. Therefore when $Q$, ${\tau }_1$, ${\tau }_2$ and ${\stackrel{-}{x}}_0$ are determined, Newton-Raphson method with Aitken acceleration is applied to simultaneously solve for $e$ and $\phi$ at different rotating speed.

A journal bearing with radius $R_j=$0.03 m, length $B_L=$0.06 m and clearance $c_b=\mathrm{}$9×10^-5 m is used in this work for analysis. Selection of values of parameters $K$, $n$ and ${\tau }_0$ in the HB model results in models of Newtonian fluid ($n=\text{1}$, ${\tau }_0=\text{0}$), Bingham fluid ($n=\text{1}$, ${\tau }_0>\text{0}$) and viscoplastic fluid ($0<n<\text{1}$, ${\tau }_0>\text{0}$). In the Newtonian fluid model, $K$ is actually the dynamic viscosity $\mu$. For reference, the value of $K$ is set to 42×10^-3 from the Lord MRF-122EG technical data [20] in the above three models.

3.1.1. Rheology in oil film

Rheology forms in oil film of Bingham model and HB model is presented in Fig. 3. Different values of $n$ ($n=\mathrm{}$1, 0.75) representing Bingham and shear-thinning HB model are selected to investigate rheological behavior and oil film pressure with $K=$42×10^-3, ${\tau }_0=\mathrm{}$100 Pa and the journal rotating at speed 500 rpm. Results with relatively high ($\epsilon =\mathrm{}$0.8) and low ($\epsilon =\mathrm{}$0.2) relative eccentricity are shown in Fig. 3 and Fig. 4.

Fig. 3Core formation and dimensionless oil film pressure, ε= 0.8

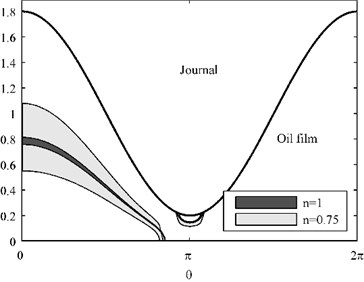
a) Core region of Bingham and shear-thinning HB fluids

b) Dimensionless pressure and pressure gradient

Whether the eccentricity is high or low, HB model predicts a larger core region, whereas the pressure is generally lower. Possible reason for this is the apparent viscosity affected by $n$. When $n<\text{1}$, apparent viscosity decreases as the shear rate increases, therefore with the same shear rate, shear stress of the HB model is lower than that of the Bingham model, which results in a larger non-yield region. On the other hand, pressure mainly depends on viscosity when shear rate is constant. Lower apparent viscosity produces lower oil film pressure. Cores are primarily formed in two typical regions in both situations. One is the area near the bearing surface where $\theta <\pi$. The other is a small area near $\theta =\pi$, where the oil film thickness reaches its minimum. Core formation and location are determined by bearing surface stress ${\tau }_1$, journal surface stress ${\tau }_2$ and Bingham number B in the following four conditions:

a) $\left|{\tau }_1\right|>B$, $\left|{\tau }_2\right|>B$ and ${\tau }_1{\tau }_2<0$. Floating core.

b) $\left|{\tau }_1\right|>B$, $\left|{\tau }_2\right|>B$ and ${\tau }_1{\tau }_2>0$. No core formed.

c) $\left|{\tau }_1\right|<B$, $\left|{\tau }_2\right|>B$. Core attached to the bearing surface.

d) $\left|{\tau }_1\right|<B$, $\left|{\tau }_2\right|<B$. Core attached to the journal surface.

Fig. 4Core formation and dimensionless oil film pressure, ε= 0.2

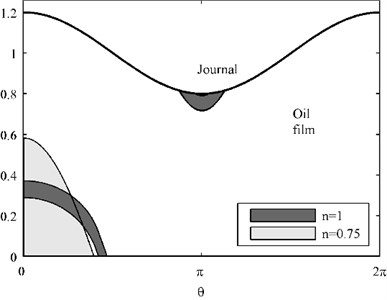
a) Core region of Bingham and shear-thinning HB fluids

b) Dimensionless pressure and pressure gradient

Fig. 5Dimensionless shear stress of bearing and journal surfaces

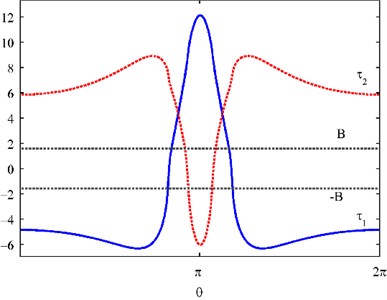
a) Relative eccentricity $\epsilon =\mathrm{}$0.8

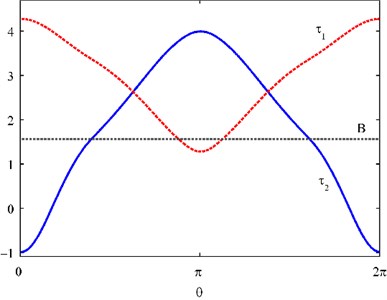
b) Relative eccentricity $\epsilon =\mathrm{}$0.2

Fig. 6Core region and oil film pressure of lubricants with yield stress 100 Pa and 1000 Pa

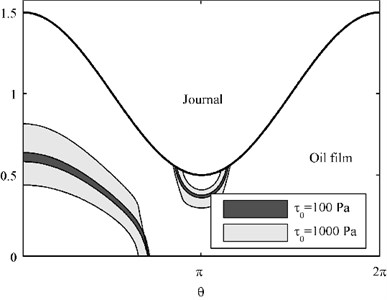
a) Core region

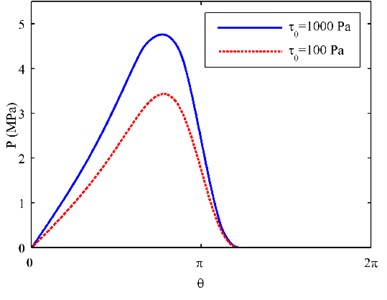
b) Oil film pressure distribution

Fig. 5 illustrates the dimensionless shear stress at oil film surfaces in the situation of $n=\mathrm{}$0.75 and (a) $\epsilon =\mathrm{}$0.8, (b) $\epsilon =\mathrm{}$0.2, which are corresponding to the $n=\mathrm{}$0.75 situation in Fig. 3(a) and Fig. 4(a), respectively. It is obvious core formation depends on the relative values of ${\tau }_1$, ${\tau }_2$ and $B$. Comparing Fig. 3 and Fig. 4, we can conclude that eccentricity is the main factor in determining the shape of the core region, whereas the magnitude of yield stress determines the area of core region.

Fig. 6 shows the core region and oil film pressure of two Bingham lubricants with $K=$42×10^-3, yield stress ${\tau }_0=$ 100 Pa and ${\tau }_0=\mathrm{}$100 Pa, at journal speed 500 rpm, and a moderate relative eccentricity $\epsilon =\mathrm{}$0.5. It again demonstrates that when other conditions are identical, higher yield stress produces larger core region and oil film pressure.

3.1.2. Load capacity and journal locus

Properties of the MR fluid are affected by several factors including viscosity of the carrier fluid, ratio of the carbonyl powder, average and distribution of the radii of the carbonyl particles, etc. Therefore five kinds of lubricants are adopted here for comparison: (1) a typical Newtonian lubricant with viscosity 12×10^-3 Pa∙s (12 cP), corresponding to $K=$12×10^-3, ${\tau }_0=\mathrm{}$0 and $n=\mathrm{}$1 in the HB model, (2) a Newtonian lubricant with viscosity 42 cP, representing the MR fluid at off state, (3) a Bingham fluid with parameters in HB model $K=$0.1, ${\tau }_0=\mathrm{}$1000 and $n=\mathrm{}$1 to simulate the MR fluid at on state, and (4) a weak shear-thinning model with $K=$0.1, ${\tau }_0=\mathrm{}$1000 and $n=\mathrm{}$0.92, and (5) a strong shear-thinning model with $K=$0.1, ${\tau }_0=\mathrm{}$1000 and $n=\mathrm{}$0.75.

Fig. 7Load capacity vs. eccentricity of the five lubricants at 500 rpm journal speed

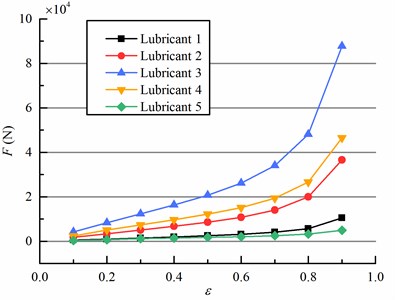
Fig. 8Journal center loci of bearings lubricated with the five lubricants

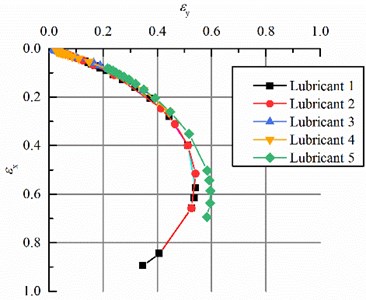
Load capacity is changed when lubricants are either Bingham or HB fluid. Oil film forces of the five situations with journal rotating at 500 rpm and eccentricity $\epsilon =$0.1-0.9 are shown in Fig. 7. Comparison of lubricant 1 and 2 demonstrate that higher viscosity produces higher oil film force for Newtonian fluid. Non-Newtonian lubricant 3 without shear-thinning effect possesses the highest load capacity. The strong shear-thinning lubricant 5 exhibits the lowest load capacity, whereas the weak shear-thinning lubricant 4 produces a relatively high force. Reason for this is that when shear rate is relatively high, the apparent viscosity is low, resulting in low load capacity. Fig. 8 shows the journal center loci with journal speed from 20-660 rpm. At the lowest rotating speed 20 rpm, journal with lubricant 1 has the greatest eccentricity while journal with lubricant 3 has the smallest one. And journal with lubricant 4 incorporating strong shear-thinning effect holds a larger attitude angle. The center locus of journal with weak shear-thinning lubricant 5 is almost the same as that of the Bingham lubricant. As the speed increases, the five loci seem to converge to a certain locus, but values at the same speed are distinct.

3.2. Dynamic characteristics

Viscosity and rheological behavior not only affect the load capacity but also the stiffness and damping properties of the bearing. The previous five lubricants are also adopted to illustrate the stiffness and damping alteration.

3.2.1. Stiffness coefficients

At low journal speed (i.e. rpm < 100), because of the larger eccentricity, bearing with lower viscosity (lubricant 1) provides greater stiffness.

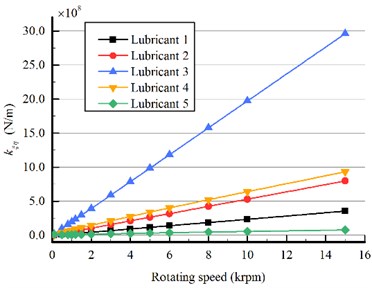
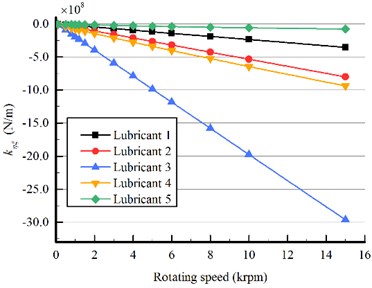
Generally speaking, as journal speed increases bearings with larger viscosity (lubricants 2 and 3) provide larger stiffness. Both direct and cross stiffness show this tendency as illustrated in Fig 9 and Fig. 10. The strong shear-thinning lubricant 5 still shows unique characteristics. Similar to its load capacity trend, the stiffness coefficients of the bearing lubricated with lubricant 4 are relatively low and increase most slowly with journal speed compared to those of the other lubricants.

Fig. 9Direct stiffness of bearings lubricated with the five lubricants, journal speed 20-660 rpm

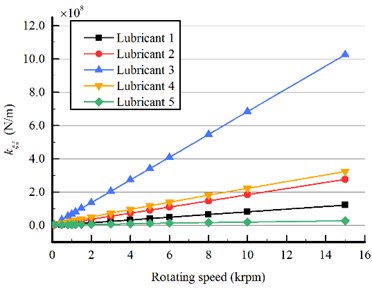
a) Vertical stiffness coefficient $k_{\xi \xi }$

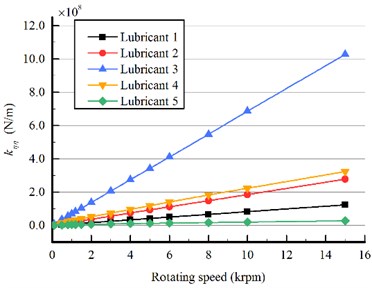
b) Horizontal stiffness $k_{\eta \eta }$

Fig. 10Cross stiffness of bearings lubricated with the five lubricants, journal speed 20-660 rpm

a) Cross stiffness coefficient $k_{\xi \eta }$

b) Cross stiffness coefficients $k_{\eta \xi }$

It is obvious from Fig. 9 and Fig. 10 that as journal speed increases, differences of the absolute magnitude of the four stiffness coefficients also increases, and stiffness of lubricants with no or weak shear-thinning effect (lubricants 3 and 4) increases more rapidly. At journal speed 8000 rpm, difference between lubricant 2 and 3, representing the off and on state of the MR fluid, in the vertical direct stiffness $k_{\xi \xi }$ reaches to 5.4747×10⁸$-$1.4772×10⁸$=$ 3.9975×10⁸ N/m, an increase as high as (3.9975$-$1.4772)×10⁷$/$(1.4772×10⁷)$\approx$170.6 %. This phenomenon suggests that as journal speed increases, contribution of the rheological effect to bearing stiffness becomes more significant.

3.2.2. Damping coefficients

Damping properties of the bearings lubricated with the five lubricants show trends similar to the stiffness while still bear differences, as shown in Fig. 11 and Fig. 12. Large eccentricity still dominates the damping behavior when journal speed is low. And higher viscosity (lubricants 2 and 3) still provides greater damping as rotating speed increases. Unlike the stiffness coefficients, however, when some journal speed is passed (about 2000 rpm), the influence of rheological behavior on damping tends to be constant, except the Bingham model (lubricant 3). And the differences between the damping coefficients of other four lubricants almost cease to change with the journal speed. This phenomenon suggests that the shear-thinning effect, whether strong or weak, has great effects on damping coefficients.

Fig. 11Direct damping of bearings lubricated with the five lubricants, journal speed 20-660 rpm

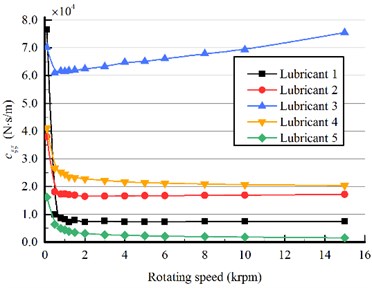
a) Vertical damping coefficient $c_{\xi \xi }$

b) Horizontal damping coefficient $c_{\eta \eta }$

Fig. 12Cross damping of bearings lubricated with the five lubricants, journal speed 20-660 rpm

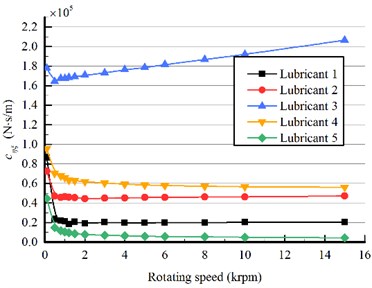
a) Cross damping coefficient $c_{\xi \eta }$

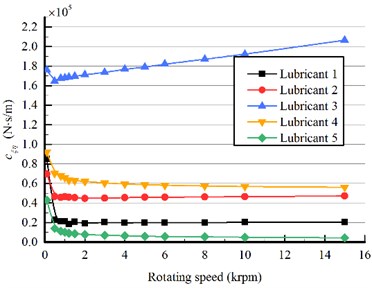
b) Cross damping coefficient $c_{\eta \xi }$

From Fig. 9-Fig. 12, different effects of rheological behavior on stiffness and damping can be observed. Generally speaking, as journal rotating speed increases, divergence of values of the stiffness coefficients increases significantly, while those of damping coefficients remain almost constant when some speed is passed.

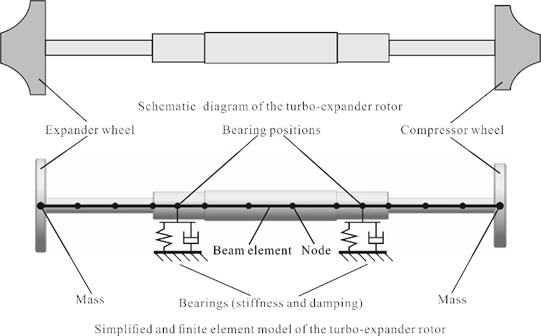
4.1. Eigenfrequency analysis

Eigenfrequencies (critical speeds) are first calculated to estimate the typical behavior of the system. Since the rotor constantly runs under 15000 rpm, eigenfrequencies below 250 Hz (i.e. 15000 rpm) are considered here. Table 1 lists the eigenfrequencies of the rotor lubricated with the aforementioned five lubricants.

Figs. 9-12 show that at speeds above 1000 rpm magnitudes of stiffness and damping coefficients of the five lubricants descends in the order of lubricant 3, 4, 2, 1 and 5. The eigenvalue analysis shows, however, this is not necessary the case for the eigenfrequencies. Due to the asymmetry of the stiffness and damping of the bearings, the order 1 and order 2 are two tilting mode frequencies. For lubricants 3 and 5, their first two frequencies are relatively close, because near those frequencies stiffness in the $\epsilon$ and $\eta$ direction and damping in the $\epsilon$ and $\eta$ direction are close. Moreover, the inequality of the masses of the two disks make the rotor an asymmetric one, therefore there are two different bending mode frequencies which correspond to the third and fourth order of the rotor eigenfrequencies. Unlike the two tilting frequencies, the bending frequencies are less changed by the properties of different lubricants. Reason for this is that bending behaviors mostly depend on the stiffness and internal damping of the rotor itself while the rigid tilting modes frequencies are determined by the supporting stiffness and damping.

The higher bending frequencies (compared to tilting frequencies) also suggest that the stiffness of the shaft is larger than that of the supporting bearings. Therefore before the rotor reaches it’s first bending critical speed, it will first pass the tilting frequencies. Since lubricants 2-5 represent properties of MR fluids at on and off states, the changes in tilting mode frequencies implies that MR fluids are applicable for altering the rotor tilting frequencies to avoid fierce vibrations when passing through these frequencies.

4.2. Rotor speeding-up simulation

In actual applications rotor vibration is more crucial when the rotor is accelerating or decelerating passing various critical speeds. Theoretically the deceleration process is the inverse of the acceleration, so the speeding-up process is investigated in this study. The eccentricity is set to be $mr=$8×10^-5 kg∙m on the expander wheel, representing an eccentric mass of $m=$1 g at radius $r=$80 mm. The process of the turbo-expander starting up in 10 seconds from 0 to 15000 rpm is simulated with speed-dependent bearing stiffness and damping, as calculated in section 3. Ordinary oil lubricant 1 is selected as a reference here to demonstrate the effects on MR fluid. Since vibration of the rotor is transferred to the turbo-expander base through the supporting bearings, vertical vibrations at the two nodes where bearings locate are measured. Fig. 14 illustrates the vibrations of the five lubricants during a start-up process in 10 seconds.

Fig. 14Speed-up vibrations of lubricants 2 to 5 compared with lubricant 1

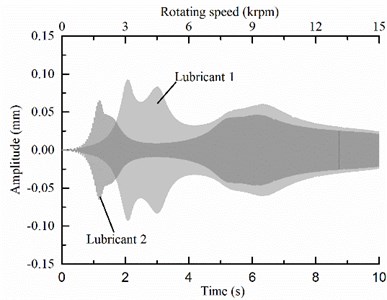
a) Lubricant 2 vs. lubricant 1

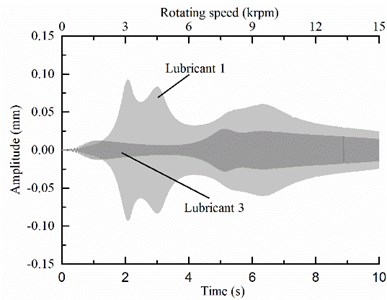
b) Lubricant 3 vs. lubricant 1

c) Lubricant 4 vs. lubricant 1

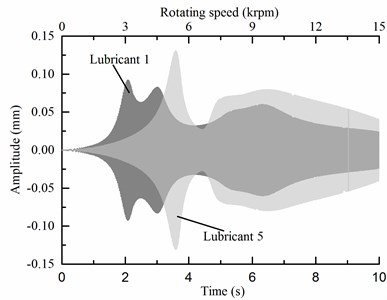
d) Lubricant 5 vs. lubricant 1

Peaks of curves in Fig. 14 corresponds to the eigenfrequencies of the various lubricants as listed in Table 1. For lubricants 3 and 5, because their first two tilting frequencies are very close, the two tilting peaks merge into one in Fig. 14 b) and d). Compared to ordinary oil lubricant 1, MR fluid at off state (lubricant 2) exhibits smaller amplitudes at tilting and bending critical speeds. The Bingham model MR fluid (lubricant 3) greatly suppresses the vibration at all speeds, especially at the tilting critical speeds. When shear-thinning effect is considered, behavior of the slightly shear-thinning MR fluid (lubricant 4) differs from the Bingham model, and the vibration-suppression effect becomes moderate. Fig. 14 d) shows that if the MR fluid strongly shear thins, it may fail to reduce the vibration amplitudes, but can largely alter the tilting frequencies.

The eigenfrequency and speeding-up analysis suggests the possible applications of MR fluid lubricants in rotor vibration control. First, since the shear-thinning effect of MR fluid is unavoidable, the Bingham model is inappropriate for modeling MR fluids, especially types strongly shear-thin. Second, for weak shear-thinning MR fluids, they can suppress the rotor vibration near critical speeds. Therefore when this type of MR fluids are used, during the accelerating or decelerating process, these fluids can be activated to avoid fierce vibration near critical speeds. Third, for strong shear-thinning MR fluids, they can greatly change the critical speeds, especially the tilting ones, even if they can barely control the vibration amplitude. This type of fluids thus are capable of avoiding severe vibrations near tilting critical speeds. For example, when we compare lubricant 2 and 5, which are the off and on states of the strong shear-thinning MR fluid, respectively, at about 2400 rpm, the fluid can be activated to avoid the tilting vibration. Then after 3000 rpm, the fluid can be deactivated to reduce shear-thinning effect, achieving a better performance.