Earthquake response of large-span reinforced concrete structures with haunch braces under vertical ground motions

1. Introduction

A new type of reinforced concrete (RC) frame structure with haunch braces (RCFB) consists of a general RC frame structure and diagonal haunch braces, which are installed locally at the vicinity of beam–column joints to protect the panel zone and to create an enhanced hierarchy of strength. The haunch braces can significantly alter the mechanism by which internal forces are transferred to the general RC frame structure at the beam-column joint region, as well as dramatically improving the mechanical and economic performance of the structure. The haunch braces are rigidly connected to the beam and column of the frame, which can provide adequate protection from excessive damage by re-directing the stress-flow around the joint region and forcing a plastic hinge in the beam to relocate. The angle between the axis of the haunch braces and the axis of the frame elements can be modified depending on the structural design requirements so as to meet the needs of the application and the bearing load within reasonable limits. The use of haunch braces does not affect the architectural function of buildings and can be applied to large-span structures, whether single-span or multi-span, equal or unequal span, single-story or multi-story. The RCFB has the following characteristics and advantages over a general RC structure. Haunch braces can alter the location of the original critical sections in the frame beam and column. The critical sections of the frame beam and column are usually located at the end of the beam and the column in the general RC structure, whereas the critical sections of the RCFB are located at the points where the haunch braces connect to the framing elements. A rigid connection between the haunch braces, the frame beam and column can reduce the span of the frame beam and column: both ends of the haunch braces are considered to support the beam and the column, where the maximum moment generally appears. In this way, the moment and the shear at the end of the beam and the column and the deflection at the mid-span of the frame beam can be largely reduced both for vertical and horizontal loads.

A critical discussion on how haunch braces affect the internal response of frame systems can be found in Pammanin et al. [1]. Diagonal haunch braces were originally proposed as part of a haunch retrofit solution that was developed for steel moment-resisting frames, following the observation of a significant number of weld fractures after the Northridge earthquake [2, 3]. The primary scope of the haunch retrofit solution was to relocate the plastic hinge away from the welded connection to protect the welds from premature cracking. A retrofit solution for existing RC frame buildings, which were designed without capacity design considerations, was developed to eliminate the damage in the beam-to-column panel zones while enhancing the global response of non-seismically designed RC frames by reversing the hierarchy of strength. Local haunch type elements were introduced in the vicinity of the beam-to-column connection to protect the joint panel zone from undesirable brittle failure mechanisms and to ensure the reversal of the strength hierarchy by forcing a flexural plastic hinge in the beam close to where the haunch was connected. Capacity design considerations prevent shear failure mechanisms in both beams and columns when relocated flexural hinges form in the beams. Based on numerical investigations on steel moment-resisting frames, Christopoulos and Filiatrault [3] proposed that haunch-type elements be used as stiffening elements or as passive elastic-plastic devices. Appa Rao et al. [4, 5] developed a non-invasive scheme in which the joint mechanics were modified to prevent the joint from reaching shear failure by relocating the plastic hinges in the beams. The effect of knee bracing or haunch elements on the optimum location and orientation that produced the maximum reduction in the joint shear stress was investigated. The practical application of RC frame structures with haunch braces to new construction structures has rarely been reported. Cai et al. [6] analyzed the mechanical behavior of RC frame structures with diagonal haunch braces under vertical and horizontal loads and were the first to use these structures in a practical application, i.e., in new construction structures, for many experimental tests [7, 8]. Recently, Zhou and Yin et al. [9] experimentally studied the seismic performance of the RC frame with viscous damper haunch braces under sinusoidal waveform steady-state excitations. The results of their investigation showed that the beam-column joint internal forces of the RC frame with viscous damper haunch braces were greatly reduced. The formation and development of plastic hinges on the top of columns was delayed, which effectively protected the beam-column joint and improved the seismic performance of the RC frames. Wu and Yang et al. [10] and Yang and Wu et al. [11] developed a new type of SRC (Steel Reinforced Concrete) beam transfer structure with supplemental energy dissipation haunch braces to improve the seismic behavior. In their research, two 1:4 scale SRC transfer frame specimens with and without energy dissipation haunch braces were tested under cyclic loading: the test results showed that the haunch brace effectively controlled the development of beam-column plastic hinges at the panel zone and relocated these hinges to the haunch-transfer beam joint.

A 32.0 m large-span prestressed RC frame structure with haunch braces was successfully constructed in the sports education building of South China Normal University in China, as shown in Fig. 1, and used to perform experimental validation tests and numerical analysis [6-8]. This sports education building exhibits the common disadvantage of being a single large-span structure: there is no auxiliary support frame in the middle of the building for the first two stories, which are vertically and horizontally large and empty, whereas the upper stories have large floor loads. The use of a general prestressed frame system in this building cannot guarantee reinforcement by potential plastic hinge regions, resulting in a vulnerable panel zone region. To meet the required architectural functions and loading capacity, the structural system of the novel large-span prestressed RC frame structure with haunch braces was adequately investigated and ultimately adopted. The RCFB is sensitive to vertical vibrations because of its 32.0 m large span; therefore, the effect of vertical seismic action should be considered.

Near-fault earthquake ground motion is an extreme load condition in structural design, and velocity pulse-like effects of this type of ground motion are one of the primary causes of structural damage in the near-fault region [12-15]. Many researchers have conducted extensive research on the basic characteristics of near-fault ground motion and the ensuing damage to engineering structures [16]. In recent years, investigations on the seismic damage from several worldwide strong earthquake events has shown that many engineering structures show signs of vertical seismic action during structural destruction, which occasionally even results directly in total collapse [17-21]. The vertical earthquake effect can be quite significant especially in the near-fault zone [20, 21]. Therefore, to better guide the earthquake-resistance design of large-span structures, it is imperative to explore the structural response to vertical pulse-like ground motion. The seismic design of large-span frame structures is more challenging than that of ordinary frame structures subjected to near-fault ground motion because of the rigorous detail required in analysis and modeling of these large-span structures. Moreover, there is a complex coupling between the near-fault velocity pulse-like action and the large span of a structure, especially for single- and large-span structures. For these reasons, the large-span prestressed RCFB has become a novel research subject. Current specifications, such as the peak value of the earthquake acceleration, require the analysis of an unfavorable earthquake response of the present large-span prestressed RCFB under the most dangerous action, such as the near-fault vertical ground motions.

Fig. 1Practical application of a novel RC frame structure with haunch braces in a sports education building

a) Global view

b) Local view

In this study, we used a dynamic time-history analysis to determine the elastic-plastic seismic response of a RCFB subjected to vertical near-fault ground motions: a study is conducted on a large-span prestressed RCFB in the SCNU sports education building subjected to horizontal ground motion [6, 8]. The vertical mid-beam displacement and the axial force of the base of the bottom column of the structure were compared under vertical pulse-like and vertical non-pulse-like ground motions, and the effects of the structural system parameters on the vertical mid-beam displacement were analyzed. This study provides a scientific basis and theoretical guidance for earthquake-resistant design and for the future operation and maintenance, etc. of the same type of building structures.

2. Records selection

Different causal mechanisms can greatly affect the near-fault pulses of earthquake ground motions: directivity effects are the presumed cause of many near-fault pulses. With the objective of mitigating the effects of these causal mechanisms, 10 records of pulse-like and non-pulse-like ground motion in the Taiwan Chi-chi earthquake event were selected from the Pacific Earthquake Engineering Research Center (PEER) strong ground motion database, as shown in Table 1. The near-fault pulse records were chosen because the velocity time history of the ground motion clearly contained pulses: the PGV was above 35 cm/s, the closest distance was less than 15 km, and the pulse indicator value [22] was greater than 0.95. The pulse indicator takes values between 0 and 1: high values correspond to a pulse-like ground motion, and values above 0.85 and below 0.15 are classified as pulses and non-pulses, respectively. The non-pulse ground motion had a PGV below 25 cm/s, a closest distance above 50 km, and the pulse indicator value was smaller than 0.15.

The ground motions were scaled to the same peak ground acceleration (PGA), and the elastic response spectrum with 5 % damping was analyzed. Near-fault pulse-like ground motions differed from far-field ground motions in that they often contained a long period high velocity pulse in the fault-normal direction and permanent ground displacement. The response spectrum of the near-fault pulse-like ground motion was different from that of the non-pulse-like ground motion. The acceleration of the response spectrum attenuated slowly as the spectral period increased relative to the response spectrum of non-pulse ground motions, because the pulse-like ground motion was rich in low-frequency pulse components, whereas the velocity and the displacement spectrum increased considerably over large periodic segments [8].

A previous study [18] has shown that the ratio of the peak vertical ground acceleration to the peak horizontal acceleration in an earthquake depends primarily on the earthquake magnitude, the rupture causal mechanism, the fault distance, the site conditions, etc. The acceleration ratio may exceed 1.0 at different instants; however, the ratio falls off with distance. The complete near-field data show significant distance dependence: a commonly acceptable acceleration ratio is close to 2/3. Thus, in the present study, a ratio of 0.65 for the value of the vertical PGA to that of the horizontal PGA, as specified in the Chinese seismic design code for buildings [23], was used in the vertical ground motion time history analysis.

3. Descriptions of structures and modeling

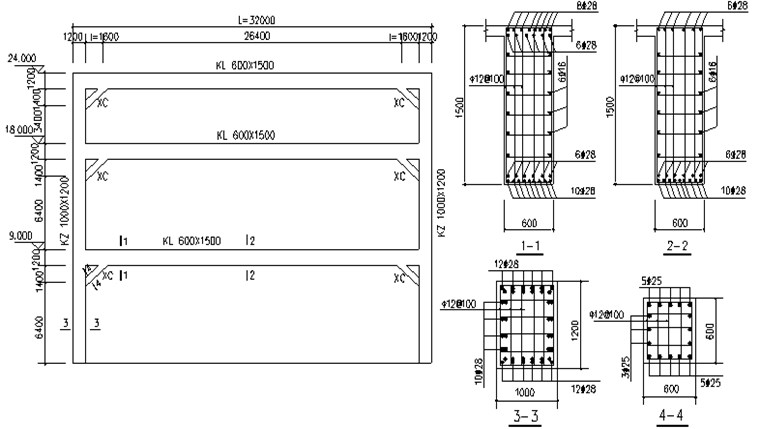
A single-span RCFB was constructed in the sports education building (see Fig. 1) of South China Normal University, which is located in a seismic region of Guangzhou in China. It is important to evaluate the vertical earthquake response because of the length of the dual-direction structural span. To evaluate this approximation, a parametric study on simplified planar structures was designed considering the different dimensions and characteristics of this sports education building. Therefore, the following specifications were used for the regular and symmetric frame structures with haunch braces, which are schematically shown in Fig. 2: (1) four different spans ($L$) for the bays, i.e., 24, 28, 32 and 34 m, for 3 stories; (2) four different ratios of the haunching lengths ($l$) to the beam span ($L$), i.e., $l/L=$ 0.03, 0.05, 0.07 and 0.09, with a typical haunching height of 0.4 m; (3) four vertical first mode periods were obtained for all of the structures by changing the vertical mass; (4) two earthquake intensity levels, i.e., the seismic records were scaled to have maximum PGA values of 0.143 m/s² and 0.201 m/s², corresponding to a rare acceleration of the vertical ground motion (corresponding to an exceeding probability of 2-3 % over a 50-year design reference period) in the seismic areas of seven degree (design basic PGA values of 0.10 g and 0.15 g), as specified by the Chinese seismic design code for buildings [23]. Using practical engineering considerations, for simplicity, all of the RC beams were considered to have a $T$ cross section with a flange thickness $t=$ 0.15 m and a flange width of 1.2 m. For simplicity, all of the columns beams and braces were assumed to have identical cross sections (see Fig. 2).

Fig. 2The geometric configuration and details of the analyzed structure (: yield strength of 400 MPa; : yield strength of 300 MPa)

The professional structural analysis procedure Zeus-NL of the MAE Center (Mid-America Earthquake Center) [24] was used to perform dynamic time history analysis. The Zeus-NL program had been used successfully to investigate the seismic vulnerability of concrete structures [25]. In the detailed Zeus-NL modeling, each structural member was assembled using a number of cubic elasto-plastic beam-column elements capable of representing the spread of inelasticity within the member cross-section and along the member length via the fiber analysis approach. Column member was modeled using five subelements, and beam member was modeled using eight subelements, as illustrated in Fig. 3. The sectional force-deformation state of members are obtained through the integration of the nonlinear uniaxial stress-strain response of the individual fibres in which the section was subdivided, distinguishing steel, confined and unconfined concrete, as illustrated in Fig. 4.

Fig. 3Structural element modeling of study frame

a) Element mesh

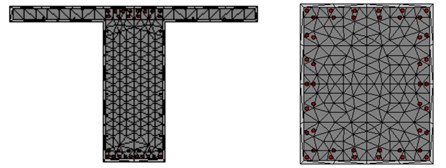
b) Cross-section mesh of beam and column

The adopted element cubic formulation allows both the representation of the spread of inelasticity along the member length as well as the implicit incorporation of interaction between axial force and transverse deformation of the element. The sufficient subelements in per structural member permit the reproduction of plastic hinge (in their full length), typical of members subjected to high levels of material inelasticity. The spread of inelasticity across the section and along the member length was thus achieved without requiring expertise calibration of any lumped plasticity element. Structural members were represented by means of frame elements, with finite length and assigned cross-sections. Structural inertia mass were introduced in distributed fashion, whilst joint elements were used to model consolidation constraint connections. Employing such element types, a number of different members (columns, beams and beam-column joints, etc.) and boundary conditions (consolidation foundations) could be represented. The fibre-discretization provided possible for a realistic modeling of different materials, and their distribution, that made up a given member cross-section. The employed material constitutive models may feature different levels of accuracy/complexity in their definition; the Menegotto-Pinto model was used for steel, whilst the core concrete of members was characterized by nonlinearity with constant confinement constitutive laws. The constitutive material models are illustrated in Fig. 5.

Fig. 4Diseretization of a typical reinforced concrete cross-section

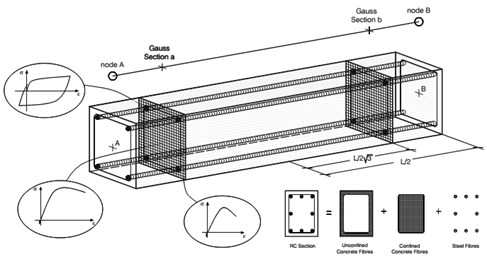
Fig. 5Constitutive material models

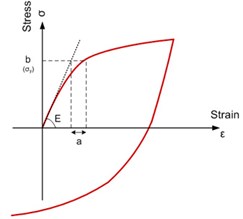
a) Steel

b) Concrete

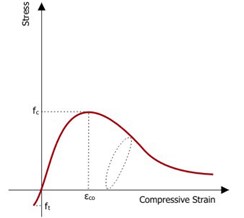
A steel bar has an elastic modulus of 2.0×10⁵ MPa and a yielding strength of 400 MPa. Concrete has a cylinder compressive strength of 21.44 MPa, a tensile strength of 1.54 MPa, and a strain at peak stress of 0.002 mm/mm. The constant confinement factor was computed from the lateral transverse reinforcement. Other details of the material models can be found in the Zeus-NL manual. The basic loading combination (1.0D+1.0L) and the distributed mass (1.0D/g+0.5L/g) were allocated to the frame beam. The prestressing tendons were equivalent to an axial pressure load and a static distributed load [26], which produced an approximate reverse deflection ratio of 1/2200 based on practical engineering measurements. In the present study, Newmark integration scheme with values $\beta =$ 0.25 and $\gamma =$ 0.5, and convergence criteion based displacement (0.001 mm) is used to conduct the numerical integration.

A series of experimental tests on a 1/4-scaled RCFB structure [7] in a 2-D configuration for different stories and haunch braces characteristics was performed in the Structural Laboratory of the South China University of Technology to verify the constructability and efficiency of the proposed structural system and to validate the design procedure (Fig. 6). However, experimental tests have never been conducted on a RCFB structure subjected to vertical dynamic loading; therefore, one of the experimental results from quasi-static tests under a horizontal load was adopted and simulated to validate the analysis of the Zeus-NL program and to select the calibration parameters. Fig. 7 compares the results of the numerical analysis and the experimental test for the load-displacement curve. The numerical results generally agreed with the experimental results.

Fig. 6Test in the structural laboratory

a) Test setup

b) Damage of the vicinity of the beam-to-column

c) Damage of the vicinity of the haunch brace to column

Fig. 7Comparison of experimental and numerical results

4. Analysis results

4.1. Comparisons of the dynamic responses and static responses

First, a modal analysis was performed on a plane RCFB ($L=$ 32 m, $l/L=$ 0.05). The first horizontal vibration period $T_{h1}=$1.0103 s and the first third vertical vibration period were 0.3264 s, 0.3006 s and 0.2833 s, respectively. The vertical dynamic elastic-plastic time history analysis was performed using the pulse-like and non-pulse-like ground motion records, where the PGA of the earthquake records was scaled to 143.0 cm/s² and 201.5 cm/s², respectively, as shown in Table 1. The variation rule of the displacement, the internal force and other responses of the vital parts of the structure were investigated, and the differences between the vertical dynamic response for pulse-like and non-pulse-like ground motions were analyzed. Under a vertical earthquake action, the typical instant time-history response of the mid-beam displacement relative to the end-beam displacement is shown in Fig. 8(a). The instant time-history response of the axial compression ratio for the bottom column is shown in Fig. 8(b). The mean value, standard deviation and amplitude of the peak responses are shown in Table 2.

Table 2 and Fig. 9 show that the large-span RCFB structure clearly exhibited the vertical dynamic response characteristics under both pulse-like and non-pulse-like ground motions. Vertical ground motions are known to strongly affect the vertical beam displacement and greatly increase the beam deflection. In this study, the following results were obtained under the earthquake actions of two PGA sets, relative to the static response of the structure under a static load: the axial compression peak value at the bottom column increased by 19.01 %-35.59 % on average, the displacement peak value of the beams increased by 32.16 %-62.20 % on average, and the beam on the third story was more strongly affected than the beams on the other two stories. The greater the PGA of the ground motion, the greater was the vertical dynamic response. A few mid-beam displacements exceeded the limits of the Chinese code (1/400) [27]. As the beam deflection increases, the vertical deformation ability of the beam needs to be improved; the column axial compression ratio increases with the axial compression, which inevitably leads to the reduction of the structural horizontal ductility capacity and the structural earthquake-resistance performance. Therefore, vertical earthquake effects should be considered in the seismic design of this type of large-span RCFB structure.

Fig. 8Comparison of the structural response time-history under pulse-like and non-pulse-like ground motions

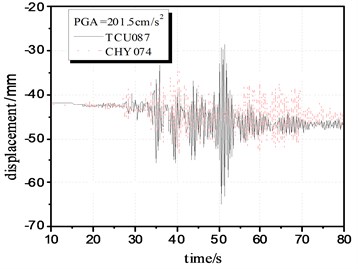
a) Displacement time-history of a mid-beam on the second story

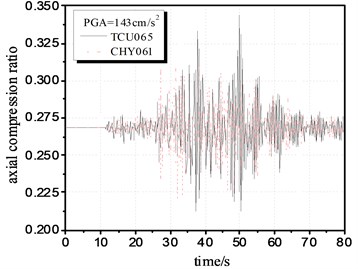
b) Axial compression ratio time-history of a bottom column

Fig. 9Comparison of maximum and minimum mean results of responses under pulse-like and non-pulse-like ground motions

a) Comparison of the axial force

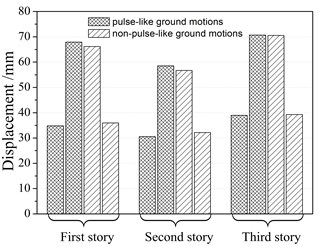
b) PGA = 143.0 cm/s²

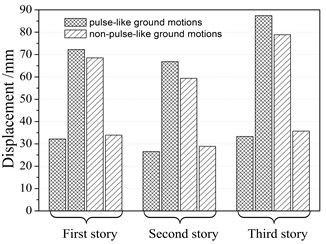
c) PGA = 201.5 cm/s²

The static axial force at base of bottom column for the basic loading combination is 6148.5 kN. The initial position of mid-beam is 45.99 mm on the first story, 42.88 mm on the second story and 48.63 mm on the third story.

Table 2Comparison of the structural peak response under pulse-like and non-pulse-like ground motions

Earthquake records		PGA = 143.0/ cm/s2
		First story				Second story		Third story
		Axial force at base of bottom column/ kN		Displacement of mid-beam/ mm		Displacement of mid-beam/ mm		Displacement of mid-beam/ mm
		MAX	MIN	MAX	MIN	MAX	MIN	MAX	MIN
Pulse-like	Mean	7688.7	4737.6	67.92	34.81	58.55	30.56	70.69	38.93
	Standard deviation	585.5	598.7	9.48	5.32	7.09	5.35	8.06	3.78
	Amplitude	1540.2	−1410.9	21.93	−11.18	15.67	−12.32	22.06	−9.70
Non-pulse-like	Mean	7317.4	5033.4	66.15	35.95	56.67	32.18	70.56	39.27
	Standard deviation	388.0	151.6	4.29	2.31	3.91	2.57	5.21	2.63
	Amplitude	1168.9	−1115.1	20.16	−10.04	13.79	−10.70	21.93	−9.36
Earthquake records		PGA = 201.5/ cm/s2
		First story				Second story		Third story
		Axial force at base of bottom column / kN		Displacement of mid-beam / mm		Displacement of mid-beam / mm		Displacement of mid-beam / mm
		MAX	MIN	MAX	MIN	MAX	MIN	MAX	MIN
Pulse-like	Mean	8336.9	4053.3	72.24	32.18	66.78	26.57	87.42	33.35
	Standard deviation	640.9	629.7	7.70	4.90	7.83	6.52	12.93	6.11
	Amplitude	2188.4	−2095.2	26.25	−13.81	23.90	−16.31	38.79	−15.28
Non-pulse-like	Mean	7815.4	4362.3	68.50	33.93	59.38	28.88	78.88	35.73
	Standard deviation	516.5	515.4	5.73	3.34	7.59	5.77	10.54	3.57
	Amplitude	1666.9	−1786.2	22.51	−12.06	16.50	−14.00	30.25	−12.90

5. Coupling effects between structural parameters and ground motions

The seismic grade and detailed measurement of a large-span RCFB structure are strictly required in seismic design. To guarantee the carrying capacity and deformability capacity of a structure, it is imperative that the coupling between the structural system parameters and the impulse on the seismic response are investigated. The effects of the first vertical vibration period, the span of the primary beam and the position of haunch braces on the beam, are analyzed below.

5.1. Effect of the period of vertical vibration

The distribution of the beam mass was varied under the same conditions to obtain four types of RCFB with different vertical periods using a modal analysis. The first horizontal and vertical vibration period of four types of RCFB were $T_{h1}=$0.812 s and $T_{v1}=$0.257 s, $T_{h1}=$1.010 s and $T_{v1}=$0.326 s, $T_{h1}=$1.085 s and $T_{v1}=$0.353 s, $T_{h1}=$1.145 s and $T_{v1}=$0.378 s, respectively. The typical first three modal characteristics of the vertical vibrations are shown in Fig. 10.

Fig. 10First three modal characteristics of vertical vibration

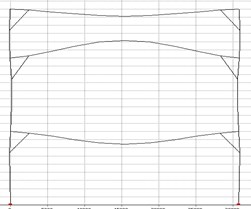
a)$T_{v1}=$0.3264 s

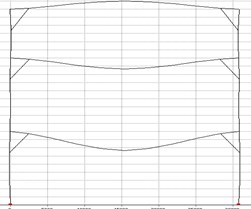
b)$T_{v2}=$0.3006 s

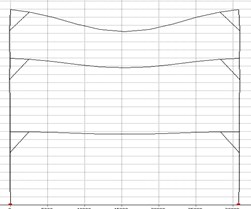
c)$T_{v3}=$0.2833 s

The effect of the vertical vibration period on the mid-beam deflection and the axial force at the bottom column are shown in Fig. 11. On the whole, the mid-beam deflection and the axial force at the bottom column increased with the vibration period. The greater the vertical vibration period was, the greater the mid-beam displacement and the axial force at the bottom column were. The mid-beam deflection was sensitive to the period of the vertical vibration, because although the vibration period increased with small gradients, the beam at the third story had already reached the damage limit.

The structural response under a pulse-like ground motion was generally larger than that under a non-pulse ground motion. The mid-beam displacement and the axial force at the bottom column of the structures with a greater vertical period slightly exhibited a divergent change, and the greater the PGA value for the ground motions was, the more significant this phenomenon became (shown in Fig. 11). This result relatively reflected the coupling effects between near-fault impulsive effect of ground motions and the period of the vertical vibration.

Fig. 11Structural response for different vertical periods

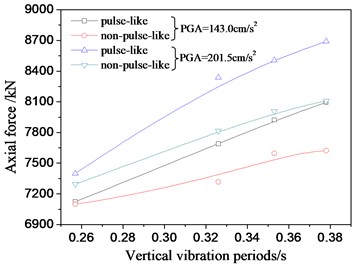
a) Comparison of the axial force

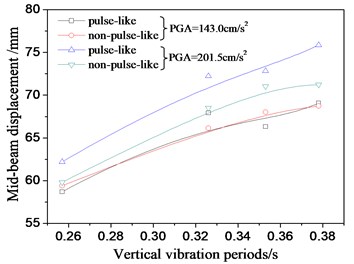
b) Comparison of mid-beam displacement for the first story

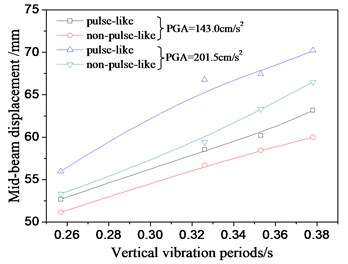
c) Comparison of mid-beam displacement for the second story

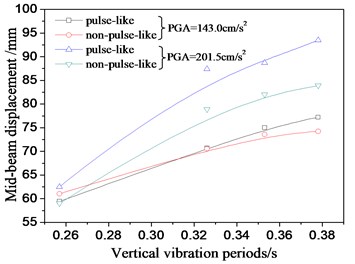
d) Comparison of mid-beam displacement for the third story

5.3. Effect of the haunch braces spans

The location of the haunch braces in the beam-column joint region significantly affected the mid-beam displacement response of the RCFB ($T_{v1}=$0.326 s, $L=$ 32.0 m, $l/L=$ 0.03-0.09). Fig. 13 shows that the mid-beam displacement decreased as the ratio of the horizontal lengths of the haunch braces to the span of the primary beam increased. The horizontal span of the haunch braces increased the vertical earthquake effect in the RCFB structure, which was similar to the decrease of the span of the primary beam. Therefore, the structural response under a pulse-like ground motion was generally larger than that under a non-pulse ground motions, and the coupling effects between near-fault impulsive effect of ground motions and the haunch braces spans was also similar to the effects of the structural spans. It can be observed that the differences between the mid-beam displacement responses under pulse-like ground motions and that under non-pulse-like ground motions increased as the ratio of the horizontal lengths of the haunch braces to the span of the primary beam increased, namely, the horizontal span of the haunch braces weakened the impulsive effect of ground motions to some extent. In brief, optimizing the location of haunch braces is very critical in RCFB structural design.

Fig. 12Mid-beam displacement response for different structural spans

a) PGA = 143.0 cm/s²

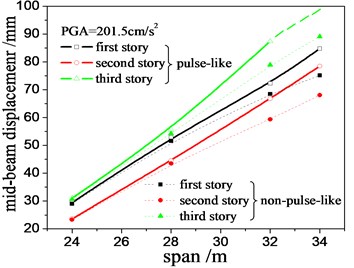
b) PGA = 201.5 cm/s²

Fig. 13Mid-beam displacement response for different location of the diagonal haunch braces

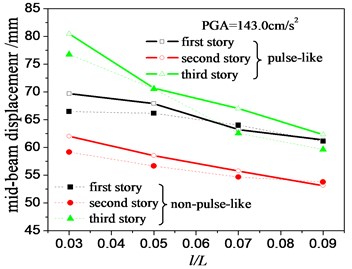
a) PGA = 143.0 cm/s²

b) PGA = 201.5 cm/s²

6. Optimal design

As mentioned above, there were many factors that affected the vertical dynamic response and global seismic performance of the large-span RC structures with diagonal haunch braces. But the main difference between a general frame and a novel frame was the diagonal haunch braces, which could significantly change the failure mechanism and dramatically improve the economic performance of the structure. To provide an economical structural design, it might be necessary to investigate how the structural cost of RCFB structure changed as the location of the haunch braces in the beam-column joint region changed. Therefore, the optimal analysis was conducted to find a design with the minimum structural cost as well as the minimum mid-beam vertical displacement while satisfying the constraints on the member strengths.

6.3. Application cases

The optimal case was the practical engineering of the novel 32.0 m large-span RCFB in the sports education building of South China Normal University as mentioned above. The building was located in Guangzhou of China, where the site grade is II (stiff soil) and 2nd Group, and the seismic intensity is 7 grades with a design basic acceleration of 0.1 g. The importance factor and occupancy category of structures are 1.0 and II, respectively. According to China’s current Code [23, 27], the loads considered in this study were a dead load (D), a live load (L), an earthquake load (E) and a basic load combination of them. The candidate sections for columns and beams, and the horizontal and vertical spans of haunch braces consisted of four available values and are summarized in Table 3. The diagonal haunch braces were designed to have identical cross section and enough bearing capacity. The width of beams and columns was constantly 600 mm and 1000 mm, respectively.

Table 3Candidate sections for columns and beams and the horizontal and vertical spans of haunch braces

Depth of beams / mm	Depth of columns / mm	Horizontal spans of braces / mm	Vertical spans of braces / mm
1400	1000	1400	1200
1500	1100	1600	1400
1600	1200	1800	1600
1700	1300	2000	1800

Table 4Preferably combinations of design variables for the novel RCFB

No.	Depth of beams / mm	Depth of columns / mm	Horizontal spans of braces / mm	Vertical spans of braces / mm
1	1400	1300	1800	1400
2	1500	1200	1600	1400
3	1600	1200	1400	1400

As shown in Fig. 14, a total of 256 solutions were obtained from the seismic analysis. There existed significant variation in terms of both initial cost and maximum deflection ratio depending on the selection of design variables. Generally, the initial cost increases with a decrease in the maximum deflection ratio. Each point in the left side of red-line represents a combination of design variables that had satisfied the design checks according to China’s current Codes. The findings suggested that three combinations of design variables were preferably selected in accordance with the results of two-objective optimization, as summarized in Table 4.

Fig. 14Results of two-objective optimization

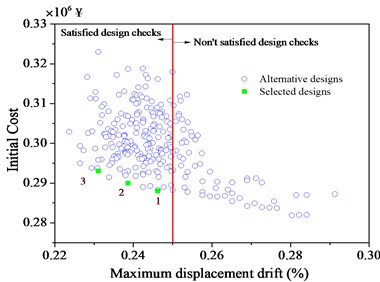
7. Conclusions

The seismic elastic-plastic response of a large-span frame structure with haunch braces under pulse-like and non-pulse-like vertical ground motions were analyzed, and a coupling effects between structural system parameters and impulsive effect of ground motions were investigated. The following conclusions were drawn from this study.

A large-span RCFB structure subjected to vertical ground motions exhibited a significant vertical dynamic response. The vertical earthquake effect should be considered in the seismic design of a RCFB structure, even for a seismic area with a PGA of only 0.1 g. Compared with non-pulse-like ground motions, near-fault pulse-like earthquake effects will probably increase the mid-beam deflection and the axial compression of bottom columns, especially when there is a significant vertical displacement response of the beam. The structural system parameters and the impulsive effect of ground motions had a distinct coupled effect on the vertical response of RCFB structure. Increasing the vertical vibration period significantly increased the axial compression of the base of the bottom column and the beam deflection of structure under near-fault pulse-like ground motions, and the beam deflection distinctly increased with the span. Increasing the horizontal span of the haunch brace can significantly decrease the beam deflection of RCFB structure under pulse-like and non-pulse-like vertical ground motions, and also weaken the impulsive effect of ground motions to some extent. Optimizing the location of haunch braces was very critical in RCFB structural design. Three combinations of design variables were preferably selected in accordance with results of two-objective optimization.