Research on the heat transfer and internal residual stress of multi-phase systems

2.1. Powder preparation, XRD characterization and SEM analysis

Pure B₄C powder and the mixed B₄C and Hf powder with the molar ratio of 4:1 and 6:1 were chosen as the raw materials, respectively. NaCl and KCl with the molar ratio of 1:1 was mixed with the raw materials with the same mass. After mixing the powders uniformly in a Al₂O₃crucible. The crucibles containing the mixing powder were heated in a 1200℃ and argon atmosphere to make the raw materials react with each other by the following equation in the molten salt.

B₄C+Hf → C+HfB₂

After the in-situ reaction, the cooled-down molten salt bulk containing the reacted powder were washed by deionized water to remove the residual salt and the as-reacted C. The powders were finally dried to remove the residual water before a series of the following microstructural characterization.

In order to get the phase composite of reacted powder, we performed the XRD analysis on the dry powder. The result is shown below.

Fig. 1XRD patterns of molten-salt reaction

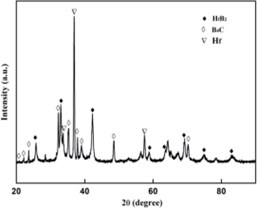
Fig. 2SEM image of molten salt reaction

The compositions of the products are identified in the Fig. 1. It indicates that the pure products contain Hf, B₄C and HfB₂. Among those elements and compounds, traces of Hf can be found, which is excessive in the first step of the reaction.

To get the microstructural morphology of the reacted powders, we employed the SEM analysis to record the detail microstructural feature of the reacted powders. The microstructural feature of the as-reacted powder of B₄C and HfB₂ is shown in Fig. 2. It can be clearly seen that the HfB₂ (white contrast) covered the B₄C (dark contrast), as labeled by the yellow arrows. However, as indicated by the XRD results, there still existed regions without the HfB₂, this region was indicated by the yellow circles.

2.2. Spark Plasma Sintering, XRD Characterization and SEM analysis

To get the bulk material, we employed SPS technique. The SPS process was performed at a temperature of 2000 ℃ and a vacuum state of 100 Pa. After the temperature cools down to room temperature, the bulk has a 98 % density of the theoretic density. In order to characterize the microstructure of the bulk, we performed the XRD and SEM on the as-received bulk, results are shown in the following sections.

Based on the XRD pattern shown in Fig. 3. The bulk material synthesized by SPS processing contains only B₄C and HfB_2. Compared to the Fig. 1, the disappearance of Hf proves that Hf has been fully reacted with B₄C and results in HfB₂ phase.

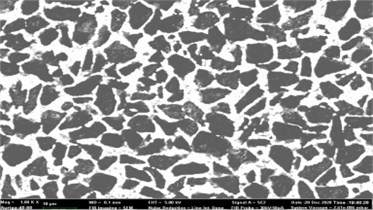
SEM was used to probe the detail microstructural feature of the bulk material. Fig. 4 presents a clear hybrid microstructure, of which, the white contrast is HfB₂, and the dark contrast is B₄C. From a two-dimensional view point shown in the SEM image, the B₄C and HfB₂ make up a typical network microstructure, which proves that the from the three-dimensional point of view, the B₄C were encapsuled by HfB₂, and results in a honey-comb structure.

Fig. 3XRD pattern by SPS processing

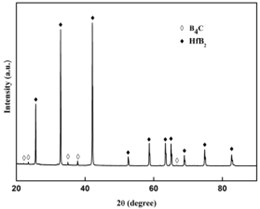
Fig. 4SEM image of the B4C-HfB2 bulk composites

2.3. Thermal properties and the crack propagation behavior

From the Fig. 5, it can be observed that with higher ratio of HfB₂, the thermal conductivity of composition increases under each temperature condition, which illustrates that the hybrid structure greatly enhances thermal conductivity of the composition. Fig. 6 shows a typical crack propagating behavior in the B₄C-HfB₂ composite, the crack is clearly observed to propagate along the HfB₂ surface when it encounters the HfB₂particle.

Fig. 5Thermal conductivity ofB4C-HfB2

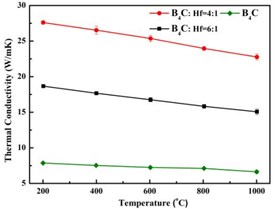
Fig. 6Crack propagation in the B4C-HfB2 composite

2.4. Experimental setup

To create a multi-phase system as the typical model to study. We design a special honey-comb structure with a high thermal conductivity HfB₂ and a low thermal conductivity B₄C materials. The novel molten-salt method was successfully adopted to synthesize the pre-requisite powders. Subsequent SPS was used to synthesize the bulk composites. The composition and microstructural feature of the bulk composites were characterized by employing the XRD and SEM techniques. It clearly revealed that the final bulk material is made up of B₄C and HfB₂phases, and the special-designed structure was also achieved. This provides us a good model system to be investigated. The thermal conductivity of B₄C-HfB₂ composite increases with the increasing of HfB₂content, and the crack propagates in an interesting way, which is related with the residual internal stress, and will be investigated below.

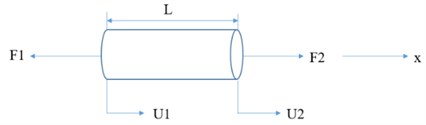
3.1. Hanics analysis

For mechanical analysis of composite materials, we must first establish a balance equation. Establish geometric deformation equation, the strain of the rod element that subjected to an external force can be expressed as ${\epsilon }_x=∆L/L$, where $∆L$ is the amount of deformation along the axial direction ($x$ axis), $L$ is the original length of the element. The physical equations of the element can be established by stress and strain ${{\sigma }_x=E\epsilon }_x$, where $E$ is defined as elastic modulus of the material, which is equivalent to the stiffness coefficient $K$ in the FE analysis, and it can be expressed by stiffness matrix in FE simulation $F_1={\sigma }_{1}XA=E{\epsilon }_{x}A=\frac{EA}{L}(U_1,U_2)$. The formula can be written in matrix form and simplified:

where [$K$] is Element stiffness matrix, $\left\{f\right\}$ is load matrix, $\left\{u\right\}$ is displacement vector.

In the finite element calculation, the displacement is used as the basic variable, when the displacement of each node calculated, the strain can be obtained through the relationship between the displacement and strain. Finally, the stress can be obtained through the physical equation.

3.2. Thermal analysis

Considering the heat transfer analysis, the temperature gradient is $∆T=\underset{n0}{\to }\frac{\partial T}{\partial n}$. Heat flux $\underset{q}{\to }=-\underset{n0}{\to }\frac{dQ}{dt}/S$, where $dQ/dt$ is the heat flux rate and $\underset{q}{\to }$ is the density of heat flux. According to Fourier’s law: the heat flux is directly proportional to the temperature gradient and in the opposite direction $\underset{q}{\to }=-k∆T$. The formula can derive $k=\frac{dQ}{dt}/\frac{\partial T}{\partial n}S$, where $k$ is the thermal conductivity. Density of heat flux can also be expressed in $q=k\frac{\partial T}{\partial n}$. According to the above analysis, the Fourier heat conduction equation can be obtained:

According to the laws of thermodynamics, thermodynamic equilibrium equations can be established $Q_1+Q_2=Q_3$, where $Q_1$, $Q_2$ and $Q_3$ are the heat obtained by the object from the outside, the heat generated by the internal heat source and the energy required by the object to raise the temperature respectively. According, the Green’s formula, We can conclude:

After simplification, the heat conduction equation can be obtained ${k\nabla }^{2}T+W-c\rho \frac{\partial T}{\partial t}=0$.

According to the types of boundary conditions for thermodynamic problems, the calculation of thermal structure coupling can be realized through finite element analysis.

3.3. Thermal properties

To predict the heat transformation behavior and the thermal properties of the composites. We established two-dimensional models, which were based on the real-structural SEM images of the composites, as shown in the Fig. 8. A series of the following analysis were established on the above models.

Fig. 8SEM images of B4C-HfB2 composites and the corresponding models

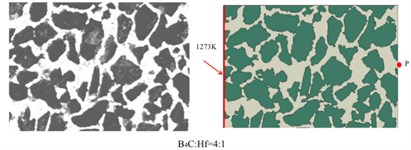
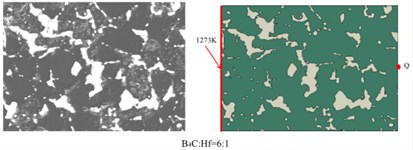
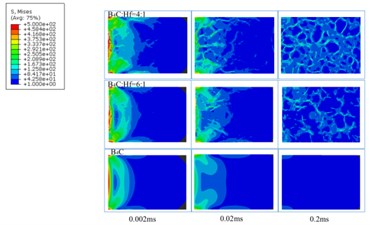
Based on the above models and the formulas in the analysis section, we got the temperature distribution as a function of time in B₄C-HfB₂ composites with molar ratio of B₄C/Hf = 4:1, 6:1 and pure B₄C, respectively, as shown in Fig. 9. As it can be seen that within the same time duration, the temperature is higher at a certain distance from the left side of the boundaries, which were set to be 1270 K and as the initial boundary condition. It proves that heat transfers faster in the composites with higher HfB₂ concentration, i.e. the HfB₂ phase improves the overall thermal conductivity of the composites.

To have a comparison between the different composites quantitatively, we measured the temperature of middle points on the right boundaries of each composites, i.e., P, Q and S, respectively. The positions were shown in Fig. 8. The temperature of P, Q and S points as a function of time is shown in Fig. 10. It demonstrates in a quantitative way that higher molar ratio of HfB₂ helps improve the thermal conductivity of composites. This is well consistent with the results obtained by experimental measurement as indicated in Fig. 5.

Fig. 9Temperature distribution in B4C-HfB2

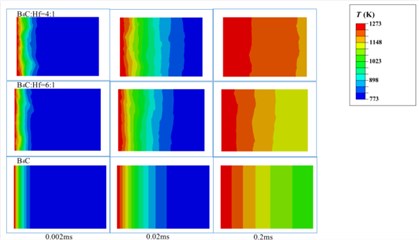
Fig. 10Temperature of the right-hand boundary

3.4. Internal stress distribution

We set the boundary condition of 500 N on the left-hand side, and based on the models established on the SEM images and the above-mentioned methods, we got a quantitative distribution of internal stress in three samples as shown in Fig. 11. It can be clearly seen that the most of the internal stress was distributed along the interface between the boundaries of B₄C and HfB₂. It is also indirectly proved by the crack propagation along the boundaries between B₄C and HfB₂, as indicated by Fig. 6. Therefore, the more the boundaries of the composites, the more residual stress, which is obviously not benefit for the improvement of mechanical properties. In contrast, in pure bulk B₄C, almost no internal stress was found. The internal stress is hard to be detected by experimental method, which proves the importance of our methods.

Fig. 11Internal stress distribution in the B4C-HfB2 composites with different B4C/HfB2 molar ratios