Structural damage assessment using a transmissibility based quantitative parameter

1. Introduction

It is estimated that termites cause damage to trees, plants, homes worth US $50 billion worldwide per year. There are at least 200 species of termites spread over the world. There is not much work published on termite damage assessment techniques. This paper describes one of the technique here which if used, can result in huge cost saving. Interior hidden damage in load bearing wooden members of the walls is a prevailing problem of wooden houses. The invisible damage of the structure is commonly attributed to attack by termites. Therefore, it is very important to gage and evaluate the damage to prevent huge economical losses and catastrophe. The aim of damage assessment is to scrutinize and identify the difference between the damaged and undamaged patterns by studying their structural characteristics [1]. The most commonly used damage detection techniques are either based on ultrasonic or x-ray scanning, but are relatively cumbersome and expensive. Therefore, the aim of paper is to contribute to the development of a simple technique for assessing the internal structural damage for the planned maintenance of the buildings. Vibration techniques can be a low cost, fast and reliable method for assessing damage and have cost saving viability. A number of vibration based techniques have been extensively used for assessing the internal structural damage that is not visually apparent. An overview of vibration based techniques can be found in [1-3] where detection of the existence of damage is done, by comparing and monitoring resonant frequency shifts using modal data or by monitoring the changes operating deflection shapes. However, the shift in the frequency measurements can be unreliable for detecting damages located in low stress regions. There-fore, an appropriate method may be by using broadband FRF data i.e. the FRF curvature and transmissibility methods [4, 5]. In this method the curvature functions obtained from the displacement functions are further processed to acquire damage index. Frequency and curvature based experimental method can be highly sensitive for locating even small amount of damage by experimentally comparing the results with resonant gapped-smoothing method. Broadband methods also reduce the time required for analysis, since no prior knowledge of the modal resonant parameters is needed and the curve-fitting step in modal analysis can be dropped. Another advantage of the method is that the procedure can be fully automated, by writing program routines and codes; since it does not require the curve-fitting process, which is more dependent on engineering judgement and knowledge of the analyst. Owing to the many advantages FRF possess over modal data, this paper applies the broadband methods for damage detection in an actual wall structure. The method presented in this paper require a starting point vibration response of the test structure to be attained experimentally first. The proposed method can be useful for any type of wall structure such as concrete.

2. Experiment set up:

An enclosed room: 2.45 m (height) × 2.1 m (width) × 1.96 m (depth) with two by four wooden columns and gypsum plasterboard, was constructed inside a laboratory to simulate realistic conditions. The construction protocol was similar to a classic wall in buildings and houses, where uneven column spacing and erratic usage of nails in walls are commonly come across. The thickness of the gypsum board was 1.21 cm and each wooden column was 3.81 cm thick and 8.52 cm wide. As it may be time consuming and cumbersome to perform measurements for the entire room, the experiment was restricted to one of the four walls as shown in the Fig. 1. The wall of interest has a six upright wooden posts (A to F) that measured approximately 2.45 m × 1.95 m. Nails were used along the four turns of the room using a hand gun to keep it intact. In addition, nails were also used for the wall of interest to lock the base to the ground; one between pillars B and C and the other between struts D and E.

Fig. 1Model of the wall with mock damage

Fig. 2Vibration experimental setup

The experimental set-up used for performing modal analysis is schematically shown in Fig. 2. Data was acquired using the Model DSPT Sig-Lab with bandwidth up to 25 kHz range and a 3300-resolution line ability. The excitation of the structure was carried out using an impact hammer and a laser vibrometer was used for gathering the vibration response. Input excitation was carried out using a PCB Piezo-tronic force hammer with force range of 0 to 50 kg. For exciting a large array of frequencies a metal tip was attached to the head of the hammer. Velocity was measured using a Polytec laser vibrometer with an optical box and controlling unit. The vibrometer was kept at a distance of about 61 cm from the goal by setting the sensitivity parameter to 26 (mm/s)/V. As shown in Fig. 2, the hammer was linked to channel 1 of Sig-Lab by a signal amplifier and the vibrometer was linked straight to channel 4. Data was acquired using a bandwidth of 0 to 210 Hz with a resolution, of 0.6 Hz (approximately 810 blocks and 0 % overlap) by connecting SigLab system to a computer. Force exponential frame was used to minimize seepage. ME’scopeVES vibro-acoustic software was used for the post processing of data i.e. curve fitting, mode shape animation and deflection shapes of the wall structures. Rational Fraction Polynomial i.e. RFP technique was used through global curve fitting, for pulling out factors. As shown in Figure 1 the wall was discretized into a total of 42 equidistant grid points which were situated along the span of the wooden columns. Initially, measurements were done at many locations with the laser and were compared to find the point where most of the resonant peaks show up (FRF curve). The transducer will sense less response related with a mode if the reference response is situated at a nodal point of one or many of the resonant modes. Therefore, the laser beam coming from vibrometer was directed at point #21 (a point where response is at acceptable level) on the outside of the room. Minimal difference was observed in response during the trial case when the room was hit from inside or outside. In the current study for ease of use, the wall was hit from outside.

3. Procedure

The 42 grid points was tapped sequentially using the impact hammer after calibrating it.

The force and velocity measurements picked up by the hammer and vibrometer were processed into FRF plots through the fast Fourier transform technique by Sig-Lab.

FRF signal (which is ratio of the output i.e. velocity or acceleration level of the structure to input excitation force) was exported to ME’scope VES’ for post-processing. Before introducing mock damage on to the wall a baseline FRF data set was acquired. In order to maintain the quality of frequency measurements, the coherence of all points was verified before the measurements. The coherence data was found to exhibit direct link between two measurements where reasonable data can have a coherence value of close to one over the range of frequencies. Poor coherence data points located near boundary were not used in the analysis. The low coherence at the boundary may be attributed to the non-relationship between the force and the velocity data.

A hand drill was used for inducing three distinct subsequent stages of damage i.e. holes mimicking mock termite infestation in the wooden posts, see Fig. 1. The downside of this is that it can cause loss in mass. The first level damage was formed in column D between lattice points 28 to 34, second level damage in column B between lattice points 8 to 14 and third level, extra mock damage between lattice points 21 to 39, from the bottom of column C up to a level of 110 cm. The level of damage created (i.e. material removed) was found to be 3 % for the 1st level case, 6 % for the 2nd level(cumulative), and 14 % for the 3rd level case(cumulative). This percentage damage is measured by estimating the level of damaged section over the total length of all the wooden posts inside the wall of interest. For each subsequent level of damage, all the 42 lattice points were reimpacted using the hammer and the response was obtained through the non-contact laser vibrometer. Post processing was done to obtain damage sensitive factors for detecting and locating the mock termite infected region.

4. Results and discussion

4.2. Broadband FRF curvature method

This approach is based upon the assumption that the spacial curvature of a vibrating structure will show an abrupt change near or around the damage. The receptance (displacement/force) data at each DOF (or discretized grid point) of the structure is required in this method. The receptance magnitude can easily be obtained from software. Since the responses were acquired in terms of mobility (velocity/force) using a laser vibrometer, each FRF was integrated to obtain the receptance FRF. The curvature at each DOF for the undamaged and damaged cases was then computed over a chosen frequency range using Eq. (1):

1

${\alpha }^C(\omega {)}_{i,j}=\frac{\alpha (\omega {)}_{i+1,j}-2\alpha (\omega {)}_{i,j}+\alpha (\omega {)}_{i-1,j}}{h^2},$

2

$\mathrm{\Delta }{{\alpha }^C}_{i,j}={\sum }_{\omega }\left|{{\alpha }_{Damage}}^C(\omega {)}_{i,j}-{\alpha }^C(\omega {)}_{i,j}\right|,$

where $\alpha {\left(\omega \right)}_{i,j}$ is the receptance FRF magnitude measured at location ‘$i$’ for a force input at location ‘$j$’ at a discrete frequency and; $h$, is the distance between two adjacent grid points. Better accuracy can be attained by increasing the number of discretized DOFs. The absolute difference between the damaged and undamaged case is computed over a chosen frequency range using Eq. (2). Sampaio et al. [5] says a low frequency range for curvature computation is giving accurate results. However, both low and high frequency ranges were used in this analysis for comparison. The three frequency ranges that were used include low frequencies between 0-25 Hz, intermediate frequencies between 25-75 Hz and high frequencies between 75-100 Hz.

Fig. 3Receptance curvature difference, ∆αi,jC(FRF/m2) over a range of frequencies

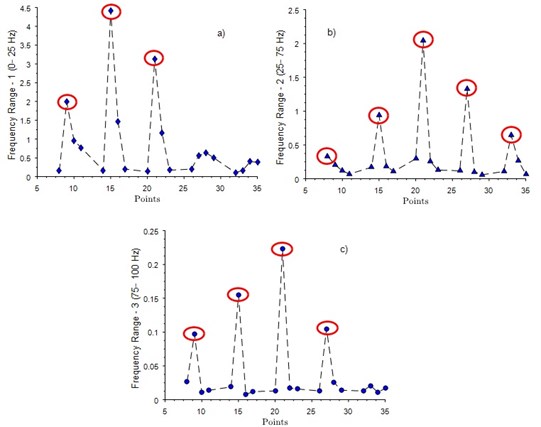
Fig. 3 shows the computed curvature difference between the baseline undamaged and the cumulative 3rd level damage case, over these three ranges using receptance data. From Fig. 3, it can be observed that all points located within the damaged region exhibit higher curvature values, compared to other points. A few points like #15 also shows a high value, although it is not located within the damage region. However, it should be noted that this grid point is located along column #C where large level of damage was induced (at #21, 27 and 33). Therefore, the curvature at #15 could have been affected due to the high deformation in that column. The results are better for the 2nd (25-75 Hz) and 3rd range (75-100 Hz), than the low frequency (0-25 Hz) range. The 1st frequency range, however, gives a rough estimate of where the damage is located but does not pinpoint it, unlike the higher frequency ranges. Based on these results, it is suggested that higher frequency ranges be used, instead of the low frequency range recommended by Sampaio. One reason for this disagreement could be attributed to the difference in boundary condition used by Sampaio et al. [26], and the one used in this investigation. Sampaio reports studies performed on a simple ideal 10 lumped-spring-mass system with 10 DOFs and no damping. Real life structures, like the one used for this research, have more complicated boundary conditions. Also, as shown in previous sections, the structural deformation pattern is more sensitive to damage at higher frequencies.

5. Conclusions

Two quantitative vibration based methodologies for gaging structural damage are described in this article. For pin-pointing the damage (within 5 cm), new quantitative techniques are developed which can precisely position the damage and are easy to use. The two quantitative approaches i.e. transmissibility and curvature, attempted to use broadband FRF rather than resonant modal data for damage assessment. The procedure can be automated and a complete modal analysis is not required. These methods are fast and accurate. The location of damage is revealed by identifying discretized grid points that exhibit higher curvature or transmissibility values. It was also observed that these methods work better over the high frequency range. These two approaches show promise and demonstrate that it is possible to utilize FRF magnitude data as a quantitative tool for locating the damaged region. This technique if commercialized, can save billions of dollars in pest control worldwide.