Vibroimpact mechanism in one separate case

Ragulskis, K.; Ragulskis, L.

doi:10.21595/mme.2019.20818

Mathematical Models in Engineering

Browse Journal

Submit article

Published: 30 June 2019

Check for updates

Vibroimpact mechanism in one separate case

K. Ragulskis¹

L. Ragulskis²

¹Kaunas University of Technology, K. Donelaičio str. 73, LT-44249, Kaunas, Lithuania

²Vytautas Magnus University, Vileikos str. 8, LT-44404, Kaunas, Lithuania

Corresponding Author:

K. Ragulskis

Cite the article Download PDF

Downloads 1263

CrossRef Citations 4

Abstract

It is known that in the vibroimpact system at the chosen values of parameters linear relationship between impact velocities and eigenfrequencies may exist. The purpose of this paper is to reveal the qualities of the systems of this type. Investigations are performed by analytical and numerical methods. It is determined that in the systems of this type nonlinear solutions with infinite series of harmonics exist. Multivalued stable and unstable regimes do not exist in the systems. The obtained analytical relationships enabled to reveal new qualities of the systems and to make useful conclusions.

1. Introduction

Investigation of dynamics of vibroimpact systems is presented in a number of publications, where the main fundamental achievements are presented including recent years for the cases when the vibrations of the system are with stiff and soft stiffness characteristics. Here the system of intermediate type is investigated. Investigations were performed also graphically, and they enabled to reveal the qualities of the system, which enable to create energetically more effective mechanisms.

Resonances and velocity jumps in nonlinear dynamics are investigated in [1]. Basic theory of vibrating systems with impacts is presented in [2]. Vibro-impact dynamics under periodic and transient excitations is investigated in [3]. Modeling of nonlinear dynamics of a system with clearance is performed in [4]. Dynamical behavior of a vibro-impact oscillator is investigated in [5]. Stabilization of periodic nonlinear systems is analysed in [6]. Basic ideas of vibrating systems in engineering are presented in [7]. Contemporary methods of vibration theory are described in [8]. Basic concepts of mechanical vibrations are presented in [9]. Nonlinear dynamics of inertial actuators is investigated in [10]. Nonlinear effects in dynamics of bearings are presented in [11]. Nonlinear contact dynamics of ultrasonic actuator is investigated in [12]. Non-sinusoidal dynamics of interacting oscillators is analysed in [13]. Free vibration analysis of piezoelectric cylinder is performed in [14]. Free vibrations of nonlinear oscillators are investigated in [15]. Synchronization of impacting mechanical systems is analysed in [16]. Chatter in mechanical systems with impacts is investigated in [17]. Dynamics of systems with impact and friction is analysed in [18]. Periodic orbits of mechanical systems with impacts are investigated in [19]. Vibro-impact nonlinear behavior and energy transfer are described in [20]. Modeling of particle impact is performed in [21]. Impacts in novel mechanisms and their applications are investigated in [22]. Resonant type impact mechanism is analysed in [23]. Positioning using impact drive mechanism is investigated in [24]. Impact mechanics of collisions and experimental results are analysed in [25]. Nonlinear rotor system with vibration absorbers is investigated in [26]. Active vibration absorber for impulse excitation is described in [27]. Nonlinear vibrations of a beam with piezoelectric actuators are investigated in [28]. Nonlinear effects and their use for vibration isolation are analysed in [29]. Nonlinear vibration absorber is investigated in [30]. Nonlinear free vibrations of beams are analysed in [31]. Electro-mechanical coupling vibrations of structures are investigated in [32]. Nonlinear dynamic analysis of vehicle system is performed in [33]. Wideband vibration attenuation is investigated in [34]. Nonlinear vibrations with interactions are analysed in [35]. Piezoelectric nonlinear vibrations are investigated in [36]. Nonlinear analysis of free vibrations of beams is performed in [37]. Nonlocal free and forced vibrations of beams are investigated in [38]. Vibration system with nonlinear coupling is analysed in [39]. Nonlinear vibrations of a system with piecewise linear spring are investigated in [40]. Nonlinear vibrations of piezoelectric plates are analysed in [41]. Free and forced nonlinear vibrations of beams are investigated in [42]. Nonlinearities in piezoceramic actuators are analysed in [43]. Nonlinear free and forced vibrations of beams are investigated in [44]. Nonlinear free vibrations of shells are analysed in [45]. Nonlinear free vibrations of plates are investigated in [46].

The system is described in the following way:

1

m \ddot{x} + H \dot{x} + C x = F s i n ω t, x < 0,

2

{\dot{x}}^{+} = - R {\dot{x}}^{-}, x = 0,

where the collision of the vibrating mass is considered as an instantaneous process, ${\dot{x}}^{-}$ denotes velocity before the impact and ${\dot{x}}^{+}$ denotes velocity after the impact, the coefficient of restitution of the impact velocity of the mass is denoted as $R$ and it is in the interval 0 $\leq R \leq$ 1.

The equation is rearranged:

3

\ddot{x} + 2 h \dot{x} + p^{2} x = f s i n ω t, x < 0,

where:

2 h = \frac{H}{m}, p = \sqrt{\frac{C}{m}}, f = \frac{F}{m} .

2. Conservative motion of the system, decaying vibrations when $f = 0$

It is assumed that the impact number $i$ of the mass $m$ to the support takes place when:

4

t = 0, x = 0, \dot{x} = {\dot{x}}_{i}^{-} > 0,

and the next impact number $i +$ 1 takes place when:

5

t = T_{i}, x = 0, \dot{x} = {\dot{x}}_{i + 1}^{-} > 0 .

According to the Eq. (3) motion after $t \geq$ 0 is:

6

x = e^{- h t} (C_{1} c o s \sqrt{p^{2} - h^{2}} t + C_{2} s i n \sqrt{p^{2} - h^{2}} t),

where the constant quantities $C_{1}$ and $C_{2}$ are found from the conditions Eq. (4) by assuming ${\dot{x}}_{i}^{+}$ after impact according to the Eq. (4).

Thus:

7

x = - \frac{R {\dot{x}}_{i}^{-}}{\sqrt{p^{2} - h^{2}}} e^{- h t} s i n \sqrt{p^{2} - h^{2}} t,

8

\dot{x} = - \frac{R {\dot{x}}_{i}^{-}}{\sqrt{p^{2} - h^{2}}} e^{- h t} (- h s i n \sqrt{p^{2} - h^{2}} t + \sqrt{p^{2} - h^{2}} c o s \sqrt{p^{2} - h^{2}} t),

9

\ddot{x} = \frac{R {\dot{x}}_{i}^{-}}{\sqrt{p^{2} - h^{2}}} e^{- h t} [(p^{2} - h^{2}) s i n \sqrt{p^{2} - h^{2}} t + 2 h \sqrt{p^{2} - h^{2}} c o s \sqrt{p^{2} - h^{2}} t] .

The next impact number $i +$ 1 takes place at the conditions Eq. (5). By taking into account the Eqs. (7) and (8) it is obtained:

10

T_{i} = \frac{π}{\sqrt{p^{2} - h^{2}}} .

During the time of the cycle of motion between the impacts $i$ and $i +$ 1 the change of velocities is lost, which is estimated by the dummy coefficient:

11

R_{f_{i + 1, i}} = \frac{{\dot{x}}_{i + 1}^{-}}{{\dot{x}}_{i}^{-}} = + R \cdot \exp (- \frac{h}{\sqrt{p^{2} - h^{2}}} π) = - R \cdot \exp (\frac{h / p}{\sqrt{1 - {(h / p)}^{2}}}) .

Further graphical material representing dynamics of the investigated system is presented for various parameters of the system in Fig. 1, Fig. 2 and Fig. 3.

Fig. 1Dynamics of the system when the initial conditions of motion t= 0, x0= 0, x˙0= –1 and p= 1 for h= 0 (thin line), h= 0.25 (line of medium thickness) and h= 0.5 (thick line)

Dynamics of the system when the initial conditions of motion t= 0, x0= 0, x˙0= –1 and p= 1 for h= 0 (thin line), h= 0.25 (line of medium thickness) and h= 0.5 (thick line)

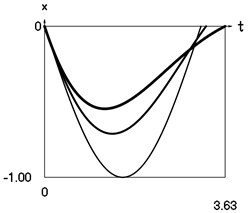
a) Displacement as function of time

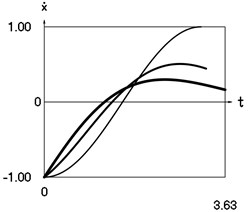
b) Velocity as function of time

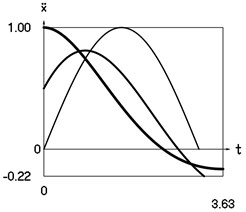
c) Acceleration as function of time

d) Velocity multiplied by acceleration as function of time

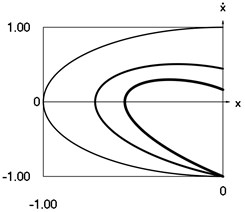
e) Phase trajectory: velocity as function of displacement

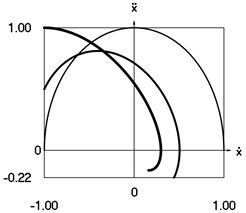
f) Phase trajectory: acceleration as function of velocity

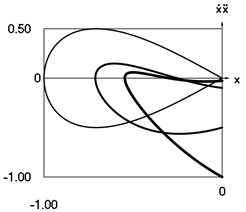
g) Phase trajectory: velocity multiplied by acceleration as function of displacement

Fig. 2Dynamics of the system when h= 0 and p= 1 for the initial conditions of motion t= 0, x0= 0, x˙0= –1 (thin line), t= 0, x0= 0, x˙(0)= –2/3 (line of medium thickness) and t= 0, x0= 0, x˙(0)= –1/3 (thick line)

Dynamics of the system when h= 0 and p= 1 for the initial conditions of motion t= 0, x0= 0, x˙0= –1 (thin line), t= 0, x0= 0, x˙(0)= –2/3 (line of medium thickness) and t= 0, x0= 0, x˙(0)= –1/3 (thick line)

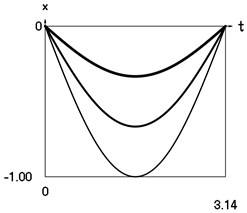
a) Displacement as function of time

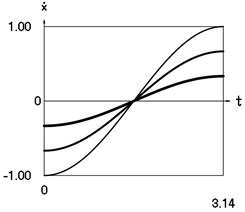
b) Velocity as function of time

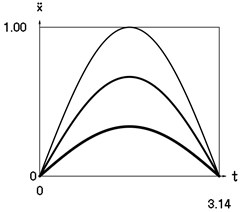
c) Acceleration as function of time

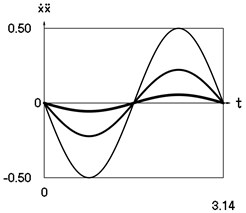
d) Velocity multiplied by acceleration as function of time

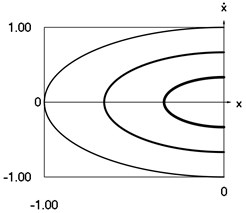
e) Phase trajectory: velocity as function of displacement

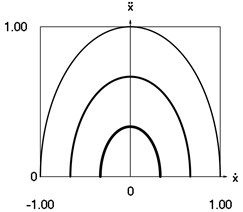
f) Phase trajectory: acceleration as function of velocity

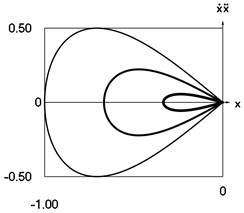
g) Phase trajectory: velocity multiplied by acceleration as function of displacement

3. Dynamics of the conservative system

Case: conservative system, that is when:

12

h = f = 0, R = 1 .

In this case the Eqs. (7)-(10) take the following form when:

13

x < 0,

14

x = - \frac{{\dot{x}}^{-}}{p} s i n p t,

15

\dot{x} = - {\dot{x}}^{-} c o s p t,

16

\ddot{x} = {\dot{x}}^{-} p s i n p t,

17

\bar{T} = \frac{π}{p} .

Period of motion $\bar{T}$ is the eigenperiod of vibrations of the system and $\bar{ω}$ is the eigenfrequency of vibrations of the system, that is by equating the right sides of the Eqs. (17) it is obtained:

18

\bar{ω} = 2 p, \bar{T} = \frac{π}{p} = \frac{2 π}{\bar{ω}} .

Fig. 3Dynamics of the system when h= 0.5 and p= 1 for the initial conditions of motion t= 0, x0= 0, x˙0= –1 (thin line), t= 0, x0= 0, x˙(0)= –2/3 (line of medium thickness) and t= 0, x0= 0, x˙0= –1/3 (thick line)

Dynamics of the system when h= 0.5 and p= 1 for the initial conditions of motion t= 0, x0= 0, x˙0= –1 (thin line), t= 0, x0= 0, x˙(0)= –2/3 (line of medium thickness) and t= 0, x0= 0, x˙0= –1/3 (thick line)

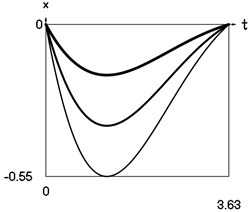
a) Displacement as function of time

b) Velocity as function of time

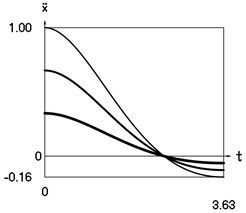
c) Acceleration as function of time

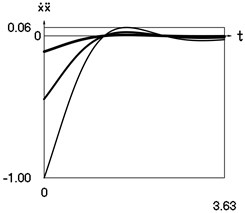
d) Velocity multiplied by acceleration as function of time

e) Phase trajectory: velocity as function of displacement

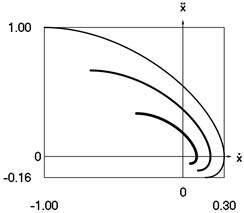
f) Phase trajectory: acceleration as function of velocity

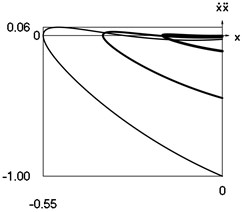
g) Phase trajectory: velocity multiplied by acceleration as function of displacement

By expanding the functions of displacement, velocity and acceleration into the Fourier series the following expressions of the first terms of the series are obtained. The laws of motions may be expanded into the Fourier series. From the Eqs. (14-16) it is obtained:

19

x = - \frac{{\dot{x}}^{-}}{p} \{\frac{2}{π} - \frac{4}{π} \sum_{n = 1}^{n = \infty} [\frac{1}{(2 n - 1) (2 n + 1)} c o s n \bar{ω} t]\},

20

\dot{x} = - {\dot{x}}^{-} \frac{8}{π} \sum_{n = 1}^{n = \infty} [\frac{n}{(2 n - 1) (2 n + 1)} s i n n \bar{ω} t],

21

\ddot{x} = p {\dot{x}}^{-} \{\frac{2}{π} - \frac{4}{π} \sum_{n = 1}^{n = \infty} [\frac{1}{(2 n - 1) (2 n + 1)} c o s n \bar{ω} t]\},

where $\bar{ω} = 2 p .$

From the Eqs. (14) and (15) it is obtained:

22

{\dot{x}}^{2} + {(p x)}^{2} = {({\dot{x}}^{-})}^{2},

that is in the system of coordinates $\dot{x} 0 p x$ there is a circle the length of radius of which is equal to ${\dot{x}}^{-} .$

From the Eqs. (15) and (16) it is obtained:

23

{(\frac{\ddot{x}}{p})}^{2} + {\dot{x}}^{2} = {({\dot{x}}^{-})}^{2},

that is in the system of coordinates $\frac{\ddot{x}}{p} 0 \dot{x}$ there is a circle the length of radius of which is equal to ${\dot{x}}^{-} .$

Further graphical material of amplitude frequency characteristics is presented in Fig. 4.

Fig. 4Amplitude frequency characteristics (constant part and first three harmonics) when h= 0 and p= 1

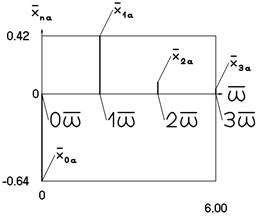
a) Displacement frequency $c o s$ characteristic

b) Velocity frequency $s i n$ characteristic

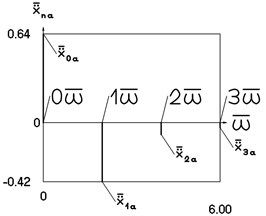
c) Acceleration frequency $c o s$ characteristic

d) Velocity multiplied by acceleration frequency $s i n$ characteristic

4. Conclusions

On the basis of the presented results the qualities of dynamic behavior of the nonlinear vibroimpact mechanism in separate case, when the contacting surface of the vibrating part of the system with the impacting surface is in the position of static equilibrium, are investigated.

Analytical relationships describing the motion of the system and amplitude frequency characteristics have been determined and are presented in the paper. Graphical relationships for typical parameters of the system were obtained and are investigated. It is shown that the values of eigenfrequencies of vibroimpact vibrations do not depend on the values of amplitudes of excitations. Because of this fact multivalued stable and unstable regimes can not take place in the vicinities of resonances.

The presented results enable to perform the design of vibrating vibroimpact systems of this type.

References

Wedig W. V. New resonances and velocity jumps in nonlinear road-vehicle dynamics. Procedia IUTAM, Vol. 19, 2016, p. 209-218.

Publisher
Ragulskienė V. Vibro-Shock Systems (Theory and Applications). Mintis, Vilnius, 1974, (in Russian).

Search CrossRef
Li T., Gourc E., Seguy S., Berlioz A. Dynamics of two vibro-impact nonlinear energy sinks in parallel under periodic and transient excitations. International Journal of Non-Linear Mechanics, Vol. 90, 2017, p. 100-110.

Publisher
Wang Y., Li F.-M. Nonlinear dynamics modeling and analysis of two rods connected by a joint with clearance. Applied Mathematical Modelling, Vol. 39, Issue 9, 2015, p. 2518-2527.

Publisher
Fu Y., Ouyang H., Davis R. B. Nonlinear dynamics and triboelectric energy harvesting from a three-degree-of-freedom vibro-impact oscillator. Nonlinear Dynamics, Vol. 92, 2018, p. 1985-2004.

Publisher
Zaitsev V. A. Global asymptotic stabilization of periodic nonlinear systems with stable free dynamics. Systems and Control Letters, Vol. 91, 2016, p. 7-13.

Publisher
Bolotin V. V. Vibrations in Engineering. Handbook, Vol. 1. Mashinostroienie, Moscow, 1978, (in Russian).

Search CrossRef
Inman D. J. Vibration with Control, Measurement, and Stability. Prentice-Hall, New Jersey, 1989.

Search CrossRef
Lalanne M., Berthier P., Der Hagopian J. Mechanical Vibrations for Engineers. John Wiley and Sons, New York, 1984.

Search CrossRef
Borgo M. D., Tehrani M. G., Elliott S. J. Identification and analysis of nonlinear dynamics of inertial actuators. Mechanical Systems and Signal Processing, Vol. 115, 2019, p. 338-360.

Publisher
Peixoto T. F., Cavalca K. L. Investigation on the angular displacements influence and nonlinear effects on thrust bearing dynamics. Tribology International, Vol. 131, 2019, p. 554-566.

Publisher
Geetha G. K., Mahapatra D. R. Modeling and simulation of vibro-thermography including nonlinear contact dynamics of ultrasonic actuator. Ultrasonics, Vol. 93, 2019, p. 81-92.

Publisher
Dellavale D., Rossello J. M. Cross-frequency couplings in non-sinusoidal dynamics of interacting oscillators: acoustic estimation of the radial position and spatial stability of nonlinear oscillating bubbles. Ultrasonics Sonochemistry, Vol. 51, 2019, p. 424-438.

Publisher
Rabbani V., Bahari A., Hodaei M., Maghoul P., Wu N. Three-dimensional free vibration analysis of triclinic piezoelectric hollow cylinder. Composites Part B: Engineering, Vol. 158, 2019, p. 352-363.

Publisher
Qu H., Li T., Chen G. Multiple analytical mode decompositions (M-AMD) for high accuracy parameter identification of nonlinear oscillators from free vibration. Mechanical Systems and Signal Processing, Vol. 117, 2019, p. 483-497.

Publisher
Baumann M., Biemond J. J. B., Leine R. I., Wouw N. V. D. Synchronization of impacting mechanical systems with a single constraint. Physica D: Nonlinear Phenomena, Vol. 362, 2018, p. 9-23.

Publisher
Dankowicz H., Fotsch E. On the analysis of chatter in mechanical systems with impacts. Procedia IUTAM, Vol. 20, 2017, p. 18-25.

Publisher
Pournaras A., Karaoulanis F., Natsiavas S. Dynamics of mechanical systems involving impact and friction using an efficient contact detection algorithm. International Journal of Non-Linear Mechanics, Vol. 94, 2017, p. 309-322.

Publisher
Spedicato S., Notarstefano G. An optimal control approach to the design of periodic orbits for mechanical systems with impacts. Nonlinear Analysis: Hybrid Systems, Vol. 23, 2017, p. 111-121.

Publisher
Li W., Wierschem N. E., Li X., Yang T. On the energy transfer mechanism of the single-sided vibro-impact nonlinear energy sink. Journal of Sound and Vibration, Vol. 437, 2018, p. 166-179.

Publisher
Marshall J. S. Modeling and sensitivity analysis of particle impact with a wall with integrated damping mechanisms. Powder Technology, Vol. 339, 2018, p. 17-24.

Publisher
Esa M., Xue P., Kassem M., Abdelwahab M., Khalil M. Manipulation of impact feedbacks by using novel mechanical-adaptor mechanism for UAV undercarriage applications. Aerospace Science and Technology, Vol. 70, 2017, p. 233-243.

Publisher
Yokozawa H., Doshida Y., Kishimoto S., Morita T. Resonant-type smooth impact drive mechanism actuator using lead-free piezoelectric material. Sensors and Actuators A: Physical, Vol. 274, 2018, p. 179-183.

Publisher
Tan Y., Lu P., Zu J., Zhang Z. Large stroke and high precision positioning using iron-gallium alloy (Galfenol) based multi-DOF impact drive mechanism. Precision Engineering, Vol. 49, 2017, p. 401-411.

Publisher
Zhang S., Villavicencio R., Zhu L., Pedersen P. T. Impact mechanics of ship collisions and validations with experimental results. Marine Structures, Vol. 52, 2017, p. 69-81.

Publisher
Taghipour J., Dardel M., Pashaei M. H. Vibration mitigation of a nonlinear rotor system with linear and nonlinear vibration absorbers. Mechanism and Machine Theory, Vol. 128, 2018, p. 586-615.

Publisher
Wang X., Yang B. Transient vibration control using nonlinear convergence active vibration absorber for impulse excitation. Mechanical Systems and Signal Processing, Vol. 117, 2019, p. 425-436.

Publisher
Przybylski J., Gasiorski G. Nonlinear vibrations of elastic beam with piezoelectric actuators. Journal of Sound and Vibration, Vol. 437, 2018, p. 150-165.

Publisher
Feng X., Jing X. Human body inspired vibration isolation: beneficial nonlinear stiffness, nonlinear damping & nonlinear inertia. Mechanical Systems and Signal Processing, Vol. 117, 2019, p. 786-812.

Publisher
Feudo S. L., Touze C., Boisson J., Cumunel G. Nonlinear magnetic vibration absorber for passive control of a multi-storey structure. Journal of Sound and Vibration, Vol. 438, 2019, p. 33-53.

Publisher
Tang Y., Lv X., Yang T. Bi-directional functionally graded beams: asymmetric modes and nonlinear free vibration. Composites Part B: Engineering, Vol. 156, 2019, p. 319-331.

Publisher
Li H., Wang X., Chen J. Nonlinear electro-mechanical coupling vibration of corrugated graphene/piezoelectric laminated structures. International Journal of Mechanical Sciences, Vol. 150, 2019, p. 705-714.

Publisher
Zhou S., Li Y., Ren Z., Song G., Wen B. Nonlinear dynamic analysis of a unilateral vibration vehicle system with structural nonlinearity under harmonic excitation. Mechanical Systems and Signal Processing, Vol. 116, 2019, p. 751-771.

Publisher
Silva T. M. P., Clementino M. A., Marqui C. D., Erturk A. An experimentally validated piezoelectric nonlinear energy sink for wideband vibration attenuation. Journal of Sound and Vibration, Vol. 437, 2018, p. 68-78.

Publisher
Miyake S., Ozaki R., Hosaka H., Morita T. High-power piezoelectric vibration model considering the interaction between nonlinear vibration and temperature increase. Ultrasonics, Vol. 93, 2019, p. 93-101.

Publisher
Ozaki R., Liu Y., Hosaka H., Morita T. Piezoelectric nonlinear vibration focusing on the second-harmonic vibration mode. Ultrasonics, Vol. 82, 2018, p. 233-238.

Publisher
Lv Z., Qiu Z., Zhu J., Zhu B., Yang W. Nonlinear free vibration analysis of defective FG nanobeams embedded in elastic medium. Composite Structures, Vol. 202, 2018, p. 675-685.

Publisher
Trabelssi M., El-Borgi S., Fernandes R., Ke L. L. Nonlocal free and forced vibration of a graded Timoshenko nanobeam resting on a nonlinear elastic foundation. Composites Part B: Engineering, Vol. 157, 2019, p. 331-349.

Publisher
Huang Z., Song G., Li Y., Sun M. Synchronous control of two counter-rotating eccentric rotors in nonlinear coupling vibration system. Mechanical Systems and Signal Processing, Vol. 114, 2019, p. 68-83.

Publisher
Ranjbarzadeh H., Kakavand F. Determination of nonlinear vibration of 2DOF system with an asymmetric piecewise-linear compression spring using incremental harmonic balance method. European Journal of Mechanics – A/Solids, Vol. 73, 2019, p. 161-168.

Publisher
Zeng S., Wang B. L., Wang K. F. Nonlinear vibration of piezoelectric sandwich nanoplates with functionally graded porous core with consideration of flexoelectric effect. Composite Structures, Vol. 207, 2019, p. 340-351.

Publisher
Rouhi H., Ebrahimi F., Ansari R., Torabi J. Nonlinear free and forced vibration analysis of Timoshenko nanobeams based on Mindlin’s second strain gradient theory. European Journal of Mechanics – A/Solids, Vol. 73, 2019, p. 268-281.

Publisher
Shivashankar P., Kandagal S. B. Characterization of elastic and electromechanical nonlinearities in piezoceramic plate actuators from vibrations of a piezoelectric beam. Mechanical Systems and Signal Processing, Vol. 116, 2019, p. 624-640.

Publisher
Mohamed N., Eltaher M. A., Mohamed S. A., Seddek L. F. Numerical analysis of nonlinear free and forced vibrations of buckled curved beams resting on nonlinear elastic foundations. International Journal of Non-Linear Mechanics, Vol. 101, 2018, p. 157-173.

Publisher
Dong Y. H., Zhu B., Wang Y., Li Y. H., Yang J. Nonlinear free vibration of graded graphene reinforced cylindrical shells: effects of spinning motion and axial load. Journal of Sound and Vibration, Vol. 437, 2018, p. 79-96.

Publisher
Gao K., Gao W., Chen D., Yang J. Nonlinear free vibration of functionally graded graphene platelets reinforced porous nanocomposite plates resting on elastic foundation. Composite Structures, Vol. 204, 2018, p. 831-846.

Publisher

Cited by

Exciters of vibrations with two pairs of impacting surfaces

K. Ragulskis | P. Paškevičius | L. Ragulskis

(2024)

Forced vibrations of vibro impact system with zero tightening with harmonic resonant excitation

K. Ragulskis | L. Ragulskis

(2021)

Investigation of single valued motions in the vibro-impact system in case of harmonic force

K. Ragulskis | L. Ragulskis

(2021)

Qualities of a vibrator, the elastic element of which has different coefficients of stiffness in the two intervals of displacements, in a separate case

(2020)

About this article

Received

20 December 2018

Accepted

16 May 2019

Published

30 June 2019

DOI

https://doi.org/10.21595/mme.2019.20818

Keywords

characteristics of impact velocity and eigenfrequencies

free and decaying vibrations

phase trajectories of motions

harmonics of motions up to infinity

This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Previous article in issue Previous Next article in issue Next

Research article

2021 06 30

Investigation of single valued motions in the vibro-impact system in case of harmonic force

K. Ragulskis, L. Ragulskis

Research article

2021 03 31

Forced vibrations of vibro impact system with zero tightening with harmonic resonant excitation

K. Ragulskis, L. Ragulskis

Research article

2020 04 02

Free vibration analysis of a discretized aircraft with an integrated biodynamic pilot model – modal approach

Hari Gowtham C, Adarsha Balila, Ankush B N, Chandrashekara C V

Research article

2020 03 31

Dynamics of a single mass vibrating system impacting into a deformable support

K. Ragulskis, L. Ragulskis

K. Ragulskis and L. Ragulskis, “Vibroimpact mechanism in one separate case,” Mathematical Models in Engineering, Vol. 5, No. 2, pp. 56–63, Jun. 2019, https://doi.org/10.21595/mme.2019.20818

Copy Extrica

Copied to clipboard!

TY  - JOUR
DO  - 10.21595/mme.2019.20818
UR  - https://doi.org/10.21595/mme.2019.20818
TI  - Vibroimpact mechanism in one separate case
T2  - Mathematical Models in Engineering
AU  - Ragulskis, K.
AU  - Ragulskis, L.
PY  - 2019
DA  - 2019/06/30
PB  - JVE International Ltd.
SP  - 56-63
IS  - 2
VL  - 5
SN  - 2351-5279
SN  - 2424-4627
ER  - 

Copy Ris

Copied to clipboard!

@article{Ragulskis_2019,
	doi = {10.21595/mme.2019.20818},
	url = {https://doi.org/10.21595/mme.2019.20818},
	year = 2019,
	month = {jun},
	publisher = {{JVE} International Ltd.},
	volume = {5},
	number = {2},
	pages = {56--63},
	author = {K. Ragulskis and L. Ragulskis},
	title = {Vibroimpact mechanism in one separate case},
	journal = {Mathematical Models in Engineering}
}

Copy Bibtex

Copied to clipboard!

[1]K. Ragulskis and L. Ragulskis, “Vibroimpact mechanism in one separate case,” Mathematical Models in Engineering, vol. 5, no. 2, pp. 56–63, Jun. 2019, doi: 10.21595/mme.2019.20818.

Copy IEEE

Copied to clipboard!

Ragulskis, K., and L. Ragulskis. “Vibroimpact Mechanism in One Separate Case.” Mathematical Models in Engineering 5, no. 2 (June 30, 2019): 56–63. https://doi.org/10.21595/mme.2019.20818.

Copy Chicago

Copied to clipboard!

Vibroimpact mechanism in one separate case

Abstract

1. Introduction

2. Conservative motion of the system, decaying vibrations when f=0

3. Dynamics of the conservative system

4. Conclusions

References

Cited by

About this article

Related Articles

2. Conservative motion of the system, decaying vibrations when $f = 0$