Impact of seismic-type shock parameters on the soil-structure interaction effect in the USC mining region

1. Introduction

Surface vibrations affecting buildings could be majorly caused by natural earthquakes. However, earthquakes are not the one only source of ground and building vibrations. So-called paraseismic (corresponding to human activity), various vibrations may also occur [1-6]. Mine-induced tremors are the most intensive of the paraseismic events. Their random occurrence (as with earthquakes) could be dangerous for buildings.

Additionally, the difficulties in the analysis of mining rockbursts increase because of the soil-structure interaction (SSI) effect, analogous to this SSI phenomenon corresponding to earthquakes [7-9]. The differences, often significant, in the vibrations registering at the same time on the free-field near a building and on the foundation level in the building can be visible among other observations in the case of SSI.

One can estimate the possible differences between simultaneously developing free-field vibrations next to a building and building foundation vibrations using a ratio of response spectra ($RRS$). This way is commonly used in the case of earthquakes [10-12]. In this study we propose to applicate $RRS$ to the other, but also seismic-type shocks, namely that is, mine-induced tremors.

The fundamental goal of the study is the evaluation of the dependence of the curves of ratio of response spectra ($RRS$) on some parameters corresponding to mine-induced vibrations, i.e. epicentral distance ($re$), level of mining shock energy ($En$), peak value of free-field vibrations ($a_{gmax}$).

Analysis concerns the ratio of dimensionless ($\beta$) and dimensional ($S_a$) acceleration response spectra-denoted as $RRS$($\beta$) and $RRS$($S_a$), respectively. Horizontal vibrations parallel to the transverse ($x$) and longitudinal ($y$) axis of the representative (typical) two-storey, masonry office building is discussed. Calculations are based on the results of in situ surface vibration measurements performed using seismological stations in the seismically active Upper Silesian Coalfield (USC) mining area in Poland (long-term, full-scale monitoring).

2. Data from measurements in USC region

The results of in situ surface vibration measurements performed in the seismically active Upper Silesian Coalfield (USC) mining area in Poland (long-term, full-scale monitoring) were the basis of this study. The focus is on horizontal vibrations parallel to the transverse ($x$) and longitudinal ($y$) axis of the representative (typical) two-storey, masonry office building. Dimensions of the building are: 12.5 m ($x$ direction), 23.8 m ($y$ direction), 7.3 m (height). Building structure is composed of bearing walls in transverse-longitudinal arrangement, without basement, concrete strip foundations (depth 1.4 m). The sub-soil is formed of soil layer, medium and fine sand, partially yellow dust. Locations of epicentre of mine-induced tremors in relation to the building position are put in simply in Fig. 1.

Fig. 1Locations of epicentre of mine-induced tremors in relation to the building position

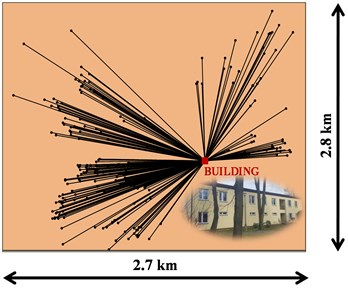
Measurement sensors (‘armed partition’ accelerometers, monitoring) registering the components of vibration accelerations, are located on the ground next to the building and in the building, at the foundation level [13]. Pairs ground-foundation of simultaneously recorded horizontal components of vibrations parallel to the transverse ($x$) and longitudinal ($y$) axis of the considered building, were analysed. Mine-induced rockbursts with energy ($En$) not smaller than from the range of 1.0×10⁵[J]-4.0×10⁹[J] were taken into analysis. The second factor taken into account during the acceptance of the results of measurements for the analysis, has related to peak ground acceleration ($a_{gmax}$) of horizontal vibrations. Only the registered records of accelerations with $a_{gmax}$ larger than 5 cm/s² were analysed. Rockburst epicentral distances ($re$) are from the range $re=$ 230-2045 m.

Number of pairs of ground and building foundation vibrations simultaneously recorded in the considered ranges of epicentral distances ($re$), energy levels ($En$) and peak ground accelerations ($a_{gmax}$), is given in Table 1. The level of magnitude in the cases of mining tremor energies with values of 10⁵[J], 10⁶[J], 10⁷[J], 10⁹[J], are denoted as E5, E6, E7, and E9, respectively. These data are listed separately in both vibration directions $x$ and $y$, parallel to the transverse and longitudinal axis of building. Analogously, the corresponding total number of recorded pairs of vibrations in $x$ and $y$ jointly (together, without distinction), is also shown in Table 1.

3. Ratio of response spectra as a function of rockburst parameters

Differences between the ground and building foundation response spectra (and of course, in fact, at the same time, the differences in the free-field and foundation vibrations) have been estimated using the so-called ratio of response spectra ($RRS$) calculated from Eq. (1) as the followed:

where the response spectrum from building foundation vibrations is denoted as $R{S}_f$, whereas $R{S}_g$ is the response spectrum from simultaneously registered ground vibrations. Non-dimensional ($\beta$) and dimensional ($S_a$) acceleration response spectra were taken into account. The fraction of critical damping $\xi =$ 3 % was taken for the response spectra calculations, according to the results of the previous investigations of buildings [14]. Variants of $RRS$ calculations according to Eq. (1), are shown in Table 2.

$RRS$ were calculated for each of the corresponding pair of response spectra (ground-building foundation). Subsequently, each individual $RRS$ was placed into separate groups (sets). The sets of $RRS$ were created on the basis of the rockburst parameters: epicentral distance ($re$), rockburst energy ($En$), and peak ground acceleration ($a_{gmax}$). Next, the averaged curves of $RRS$ were prepared in the sets corresponding to the considered ranges of mining tremor parameters (cf. Table 1), separately in the case of $x$ direction, $y$ direction, $x$ and $y$ directions together.

Fig. 2 and Fig. 3 show $RRS$ as the function of mining tremor epicentral distances in the case of dimensionless spectra (Fig. 2) and dimensional spectra (Fig. 3). Analogous curves of $RRS$, but corresponding to rockburst energy ($En$), and peak ground acceleration ($a_{gmax}$), are presented in Fig. 4, Fig. 5 and Fig. 6, Fig. 7, respectively. Because of the fact that the sources (mine-induced tremors) of the most of measured vibrations originate from the rockbursts with energy level E5 and E6 (cf. Table 1), the $RRS$ averaged in this range of energies, affect in the biggest degree on the $RRS$ averaged in the whole range of energies. The analogous observation concerns the $RRS$ curves calculated in the range of epicentral distances $re=$ 701-1500 m.

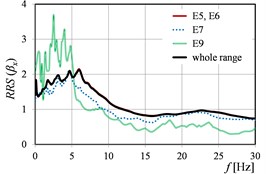
The vibration direction (parallel to the $x$ or $y$ axis of the building) may have a clear influence on the numerical quantity of the $RRS$ in the analysed ranges of mining parameters. Moreover, even different (opposite) ‘trends’ could be visible in $x$ and $y$ directions. $RRS$(${\beta }_x$) and $RRS$(${\beta }_y$) in the function of the ranges of mining tremor energy (Fig. 4), as well as $RRS$($S_{ax}$) and $RRS$($S_{ay}$) in the function of the ranges of epicentral distance (Fig. 3), are the examples of this observation. It is worth mention that the analysis in the two directions ($x$ and $y$) concerns the same ground site (two axes in the same office building). Therefore, the differences in the transmission of the response spectra from free-field to building foundation in $x$ and $y$ directions, could be resulted from the different stiffness of the two-storey building in each of the direction.

Fig. 2RRSβ as the function of mining tremor epicentral distances in directions: a) x, b) y, c) x and y

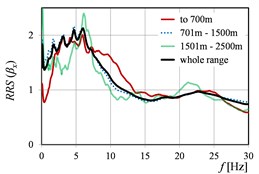
a)

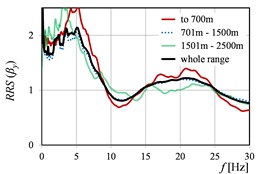
b)

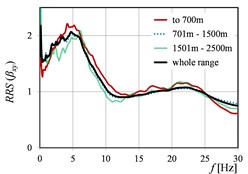
c)

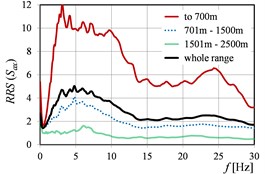
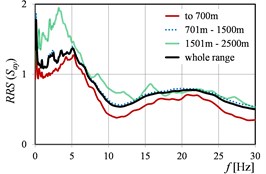
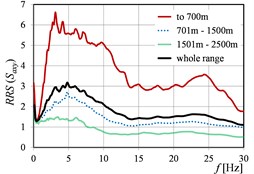
Fig. 3RRSSa as the function of mining tremor epicentral distances in directions: a) x, b) y, c) x and y

a)

b)

c)

Fig. 4RRSβ as the function of mining tremor energy levels in directions: a) x, b) y, c) x and y

a)

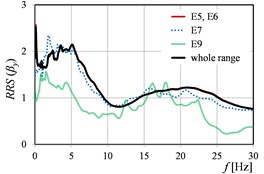
b)

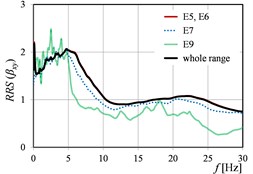
c)

Fig. 5RRSSa as the function of mining tremor energy levels in directions: a) x, b) y, c) x and y

a)

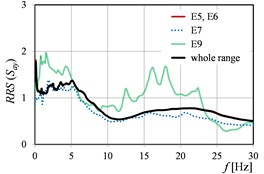
b)

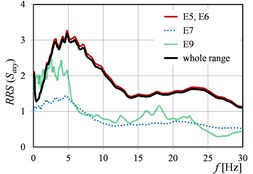
c)

Also, the clear shift of the peak values of $RRS$($S_a$) is visible in the successive ranges, practically in the case of each parameter, cf. Fig. 3, Fig. 5, and Fig. 7.

Generally, it can be stated that all the three considered parameters ($re$, $En$, $a_{gmax}$) affect significantly on the values of $RRS$($S_a$). For $RRS$($\beta$), the differences in the values obtained in the successive ranges of these parameters, are a little smaller than in the case of $RRS$($S_a$), but still essential from the practical point of view.

Naturally, in the all cases of $RRS$ determined on the basis of experimental data, the values calculated in the range of low frequencies (to about 10-12 Hz), are the biggest. This is only confirmed the well-known phenomenon that building works as a low-pass filter.

Fig. 6RRS(β) as the function of peak ground acceleration values in directions: a) x, b) y, c) x and y

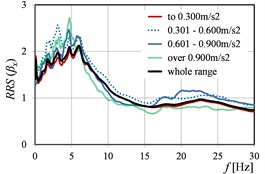
a)

b)

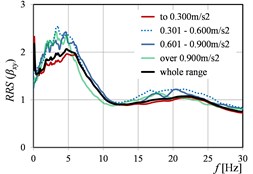
c)

Fig. 7RRS(Sa) as the function of peak ground acceleration values in directions: a) x, b) y, c) x and y

a)

b)

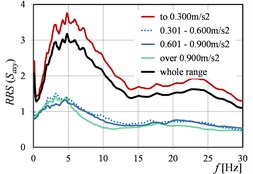
c)

4. Conclusions

From the obtained results, it can be definitely concluded that the influence of the most important mining tremor parameters (i.e. epicentral distance, mining shock energy, peak value of free-field vibrations) on the ratio of response spectra calculated separately in the successive ranges of these parameters, is clearly observed. This conclusion concerning the strong dependence of the nature of the transmission of response spectra from mine-induced ground vibrations to building foundation vibrations on these parameters, is very important in practice.