Simulation on propagation characters of ground vibration induced by in-tunnel excitation source

1. Introduction

With the rapid development of urban rail transit, environmental pollution caused by urban rail transit has gradually become an environment sensitive topic. To meet the needs of urban environment for traffic vibration control, in addition to the vibration control measures taken on the source, the control measures on the propagation path such as trenches and the partition wall and wave impeding block by the virtue of their effective control of low-frequency vibration, low-cost line investment and restoring the track structure to ensure the safe operation of the vehicle are more and more concerned [1]. Jones tested the vibration isolation effect of the vibration along the railway. The results show that when the depth of the trench is 3-5 m, the vibration of 6-8 Hz can be reduced by 5 dB [2]. With C found that the vibration peak of 41-67 % can be reduced in the range of 60 m by studying the vibration reduction effect of the fly ash isolation pile on the parallel railway near Goteborg [3]. Wei Hongliang conducted a numerical analysis of single row of pile vibration isolation [4]. Before the operating of Xi’an Metro Line 2, Ma Meng et al. studied the vibration isolation effect of the isolated row piles on the subway by numerical calculation [5]. Belgian CDM Company through theoretical analysis, model test and field test on the two layers of elastic material composite vibration wall were studied, the results show that the vibration effect is obvious [6].

However, for the issues on vibration mitigation measures at the transmission path, most of recorded academic papers were focused on the vibration isolation measures of the ground vibration source, especially the vibration induced by railway running on the surface of ground. But when the vibration source located in the tunnel, the surface vibration propagation mechanism is very different.

Therefore, in order to research on vibration mitigation measures at the transmission path for in-tunnel excitation source, a numerical calculation model of the tunnel and foundation was constructed by the FEM software ABAQUS. Using measurement vibration as the in-put excitation load, ground vibration propagation characters were calculated in cases of setting two common used vibration isolation measures, including open trench and buried concrete wall barrier.

2. Establishment of numerical simulation model

2.1. Selection of model size and unit size

The size of the finite element model of wave motion is shown in Fig. 1. Due to this model is inerratic relatively, when meshing division, in order to accurately capture the wave effect, the unit size is less than or equal to 1/6 of the minimum wavelength in the soil, the maximum length of soil layer is 0.53 m. In the establishment of the calculation model, the actual unit length is taken as 0.25 m in the vibration response receiver area, the unit type is C3D8R (an 8-node linear brick, reduced integration, hourglass control), the calculation model is shown in Fig. 2.

Fig. 1Size of model

Fig. 2Diagram of calculation model

2.3. Setting of boundary parameter and load parameter

To minimize the effects of boundary reflections, infinite element boundary is used in the model boundary. The modified model is shown in Fig. 3.

Fig. 3Modified model

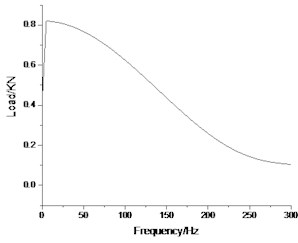
In order to be able to simulate the effect of the real load on the structure, the model uses the vibration load of the vibrating test.

Fig. 4Time history of load

Fig. 5Frequency spectrum of load

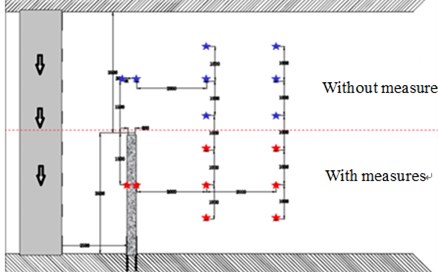
The load is applied to the middle of the tunnel, the south side of the tunnel, the north side of the tunnel, as shown in Fig. 6.

Fig. 6Load position

3. Numerical results

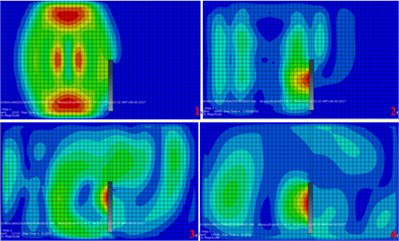
In order to express the qualitative effect of the vibration isolation measures in the propagation path, this paper adopts the cloud diagram of the surface vibration response with time, according to the results of three conditions-no measures, open trench and concrete wall, as shown in Fig. 7 to Fig. 9.

Fig. 7The developmental cloud diagram of surface vibration response with time (no measures)

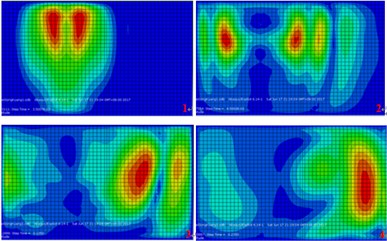
a) Excitation at the north side of the tunnel

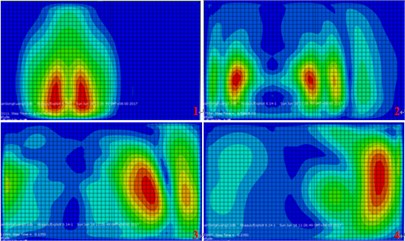
b) Excitation at the south side of the tunnel

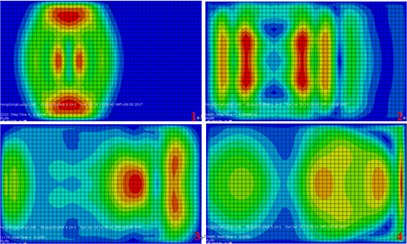
c) Excitation at the middle of the tunnel

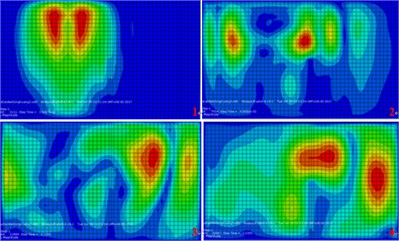
Fig. 8The developmental cloud diagram of surface vibration response with time (open trench)

a) Excitation at the north side of the tunnel

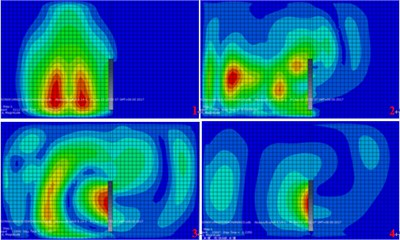
b) Excitation at the south side of the tunnel

c) Excitation at the middle of the tunnel

It is clearly shown through the above cloud diagram:

1) In the case of setting no vibration isolation measures, the surface vibration propagation are good symmetry and consistency for both excitation at both south and north side of the tunnel. It also indicated when the excitation in the tunnel, surface vibration would distributed along the tunnel. And it should be figured out that the maximum amplitude appears is not exactly above the top of the tunnel.

2) When open trench was adopted, the barrier effect was not obvious. When using buried concrete wall barrier, the vibration isolation effect is similar to that of the open trench in the time domain, and it was not obvious either.

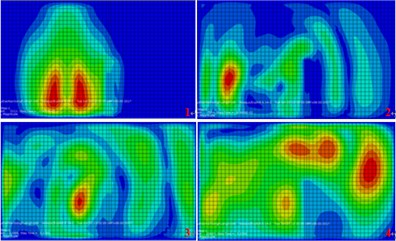
3) When using buried concrete wall barrier, due to the difference of vibration diffusion, the vibration of the surface behind the wall was obviously enlarged. This paper analyzes the causes of the phenomenon: The wave reflection and refraction phenomenon occurred, so that near the wall area, the vibration wave appears the effect of vector superposition. On the other hand, because the concrete wall itself has a natural frequency, a resonance phenomenon occurs, which is equivalent to a new vibration source.

Fig. 9The developmental cloud diagram of surface vibration response with time (buried concrete wall barrier)

a) Excitation at the north side of the tunnel

b) Excitation at the south side of the tunnel

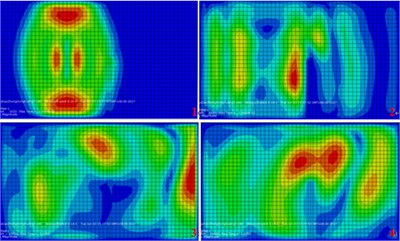
c) Excitation at the middle of the tunnel

4. Conclusions

Calculation results indicated the open trench and buried concrete wall barrier can ideally shield the propagation of vibration induced by in-tunnel excitation source.

However, the findings also indicated that the influence of the depth and width on the effective frequency range of vibration reduction should be further studied for open trench, while the influence of the depth, the wall thickness and the natural frequency on the vibration reduction effect should be paid much attention for concrete continuous wall.