Investigation of influence of high-frequency vibrations on the stability of stationary rotations of Lagrange’s top

1. Statement of the problem

consider the motion of Lagrange’s top (a dynamically symmetric body with the suspension point $O$ and the center of mass $G$ being located on the symmetry axis) in the homogeneous gravity field. Let the point $O$ accomplishes a specified periodic (with frequency $\mathrm{\Omega }$) motion. Its radius-vector relative to the fixed point $O_{\mathrm{*}}$ is given by projections $O_{\mathrm{*}}O=\left(u\right(t),v(t),w(t\left)\right)\mathrm{\text{'}}$ on the axis of the fixed coordinate system $O_{\mathrm{*}}xyz$ (with the axis $O_{\mathrm{*}}z$ directed vertically upward), at that average time values of these projections are zero. Let $m$ be the mass of the body, $A$ and $C$ the equatorial and axial moments of inertia, and length of the radius-vector of the mass center $OG=l$.

Let the maximum removal $h$ of the suspension point from the point $O_{\mathrm{*}}$ is small in comparison with the reduced length $L=A/ml\text{,}$ and the frequency $\mathrm{\Omega }$ great in comparison with the characteristic frequency $\omega =\sqrt{g/L}$. We introduce a small parameter and put:

We will write the equations of motion of the top in the form of canonical Hamiltonian equations. Using the methods of the perturbation theory we can eliminate time explicitly contained in the Hamiltonian in terms up to the fourth order inclusively in $\epsilon$ [1]. Discarding terms of the fifth order in $\epsilon$ and above, we can write the approximate Hamiltonian in the form:

here $\psi$, $\theta$, $\phi$ are the Euler angles describing the orientation of the related to the body coordinate system with axes directed along the principal axes of inertia of the body for the point $O$, and the coordinate system performing the translational motion with the origin at the point $O$ and axis parallel to ones of the fixed coordinate system. Also $P_{\psi }$, $P_{\theta }$, $P_{\phi }$ are canonically conjugate momenta. The potential energy $\mathrm{\Pi }(\theta ,\psi )$ consists of the gravitational ${\mathrm{\Pi }}_g$ and the vibrational ${\mathrm{\Pi }}_v$ potentials, in the last one we have introduces the notations $a_1=a_x-a_z=⟨{\dot{u}}^2⟩-⟨{\dot{w}}^2⟩$, $a_2=a_y-a_z=⟨{\dot{v}}^2⟩-⟨{\dot{w}}^2⟩$, $a_z=⟨{\dot{w}}^2⟩$, $a_{xy}=⟨\dot{u}\mathrm{}\dot{v}⟩$, $a_{yz}=⟨\dot{v}\mathrm{}\dot{w}⟩$, $a_{xz}=⟨\dot{w}\mathrm{}\dot{u}⟩$. The angle brackets denote the average value over time, and the point differentiation by time. The axes $Ox$ and $Oy$ can be always chosen such as to satisfy the relation $a_{xy}=0$.

Further investigation will be carried out in the framework of the approximate system with the Hamiltonian (Eq. (1)). In this system as well as in the initial full system the coordinate $\phi$ is cyclic and the corresponding momentum $P_{\phi }$ is constant.

Consider the particular solutions (positions of equilibrium) of the reduced two-degree-of-freedom system of the form:

where constant values ${\theta }_0$ and ${\psi }_0$ are stationary points of the potential energy $\mathrm{\Pi }(\theta ,\psi )$ satisfying the relations ${\mathrm{\Pi }\mathrm{\text{'}}}_{\theta }=0$, ${\mathrm{\Pi }\mathrm{\text{'}}}_{\psi }=0$.

These solutions correspond to the stationary rotation of the top around the axis of dynamical symmetry retaining a fixed position in the coordinate system moving translational with the suspension point. Angular velocity of rotation can be arbitrary; we assume it is positive.

The aim of this work is to solve the problem of existence and bifurcation of these solutions at fixed values of $P_{\phi }$, and to investigate their stability with respect to the variables $\psi$, $\theta$, $P_{\psi }$ and $P_{\theta }$. We will consider a number of particular cases of motion of the suspension point.

2. Investigation scheme

Let’s introduce perturbations by the formulas $x_1=\theta -{\theta }_0\text{,}$$x_2=\psi -{\psi }_0\text{,}$$y_1=P_{\theta }$, $y_2=P_{\psi }-P_{\phi }\mathrm{c}\mathrm{o}\mathrm{s}{\theta }_0$. The quadratic part of the Hamiltonian of the perturbed motion has the form:

The conditions for sign-definiteness of the quadratic form $H_2$ are the sufficient conditions for stability. Using the Sylvester’s criterion we can find that these conditions are reduced to the system of inequalities of the form:

Under these conditions the corresponding position of equilibrium of the reduced system is the point of minimum for the potential energy $\mathrm{\Pi }(\theta ,\psi )$, while the angular velocity of the rotation can be arbitrary.

The characteristic equation of the linearized equations of motion has the form:

The necessary conditions for stability has the form:

If they satisfy the characteristic equation have only pure imaginary roots.

The sufficient conditions described above are also necessary conditions, so we can search domains where only necessary conditions satisfy. The condition $b>0$ yields inequalities ${\mathrm{\Pi }}_{\psi \psi }^{\text{'}\text{'}}<0$, $\mathrm{\Delta }>0$. This means that the position of equilibrium is the point of maximum for the potential energy. The condition $d>0$ is reduced to the biquadratic in $P_{\phi }^{}$ inequality. Studying this inequality together with the condition $a>0$ provides the lower boundary for the angular velocity of stationary rotation in the form:

If the inequality sign is changed to the opposite or $\mathrm{\Delta }<0$ (in the case of the saddle point of the function $\mathrm{\Pi }(\theta ,\psi )$) the position of equilibrium under consideration is unstable for any angular velocity of the body.

3. Case ${\mathit{a}}_{\mathit{x}\mathit{y}}={\mathit{a}}_{\mathit{y}\mathit{z}}={\mathit{a}}_{\mathit{x}\mathit{z}}=0$

Let us first consider the cases of the top suspension point motions allowing for the vertical position of the axis of the stationary rotation. The analysis shows that this is possible only if $a_{xy}=a_{yz}=a_{xz}=0$.

We introduce the dimensionless parameters, the momenta and time by the formulas:

and rewrite Hamiltonian (Eq. (1)) in the form:

Without loss of generality, we assume that ${\alpha }_1>{\alpha }_2$.

For all values of the parameters ${\alpha }_1$, ${\alpha }_2$ and $\gamma$, there exist positions of equilibrium of the reduced system when ${\theta }_0=0$ (the upper position where the rotation axis is vertical and the mass center of the top above the suspension point) and ${\theta }_0=\pi$ (the lower position where the axis is vertical, the mass center below the suspension point). They correspond to the inverted and hanging “sleeping” Lagrange top. For these values $\theta$ the Hamiltonian of the system is not defined, so when studying the stability, the Hamiltonian obtained by a different choice of the related axes was used.

In addition, if $\left|{\alpha }_1\right|>\text{1}$ then there are solutions of the form $\mathrm{c}\mathrm{o}\mathrm{s}{\theta }_0=1/{\alpha }_1$, ${\psi }_0=\pi /2$ corresponding to the rotation of the top around the axis lying in the plane $OXZ$ and being inclined to the vertical (the lateral position of the first type). If $\left|{\alpha }_2\right|>1$ then there are solutions of the form $\mathrm{c}\mathrm{o}\mathrm{s}{\theta }_0=1/{\alpha }_2$, ${\psi }_0=0$ when the axis of rotation is located in the plane $OYZ$ (the lateral position of the second type).

It was found that for the upper position sufficient and only necessary conditions for stability are realized if ${\alpha }_2<-1$, ${\alpha }_1<-1$ and ${\alpha }_2>-1$, ${\alpha }_1>-1$, $\gamma >{\gamma }_1$ respectively. For the lower position the same conditions are satisfied when ${\alpha }_2<1$, ${\alpha }_1<1$ and ${\alpha }_2>1$, ${\alpha }_1>1$, $\gamma >{\gamma }_2$. The sufficient conditions for the stability of the lateral position of the first type are performed when ${\alpha }_1>1$, while the only necessary conditions do not implement. For the lateral position of the second type, only necessary conditions are satisfied when ${\alpha }_2<-1$, $\gamma >{\gamma }_3$. Here:

In the particular case when vibrations are absent (and ${\alpha }_1=a_2=0$) the necessary stability condition obtained for the upper position coincide with the classical Maievsky-Chetaev stability condition ${\gamma }^2>4$ (in the dimensional parameters $P_{\phi }^2>4Amgl$) for the inverted “sleeping” Lagrange’s top with a fixed suspension point. Case ${\alpha }_1={\alpha }_2$ (i.e. $a_x=a_y$) is previously considered in [2]. The stability of the "sleeping” Lagrange’s top with vertical vibrations of the suspension point of arbitrary frequency and amplitude is investigated in [3].

In the domains where only necessary stability conditions perform nonlinear stability analysis was carried out by means of the well-known methods for investigation of the autonomous two-degree-of-freedom Hamiltonian systems [4].

4. A motion of the suspension point along the inclined straight line

Let the suspension point moves along the straight line in the $OYZ$-plane at the angle $\alpha \in \left(0;\pi /2\right)$ to the horizontal, with the average value $V$ of the square of the velocity. The axes of stationary rotations are located in the same plane, its position is described by the equations ${\psi }_0=0$ and:

Fig. 1Bifurcation curve and graphs of the potential energy πθ

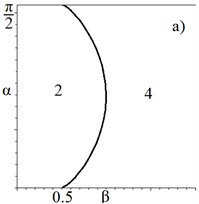
The analysis shows that Eq. (3) has two roots ${\theta }_{01}\in \left(0;\alpha \right)$, ${\theta }_{02}\in \left(\pi /2+\alpha ;\pi \right)$ in domain 2 (Fig. 1(a)), and four roots ${\theta }_{01}$, ${\theta }_{02}$, ${\theta }_{\mathrm{03,04}}\in \left(\pi +\alpha ;3\pi /2+\alpha \right)({\theta }_{03}<{\theta }_{04})$ in domain 4. The curve that separates region 2 and 4, may be described in parametric form by the equations:

The sufficient conditions for stability of the equilibrium positions indicated are reduced to the system of two inequalities of the form:

The first of them coincides with the condition of minimum of the potential energy $\pi \left(\theta \right)$ being obtained from the function $\mathrm{\Pi }\left(\theta ,\psi \right)$ after substitution $\psi =0$. The graphs of the function $\pi \left(\theta \right)$ in domains 2 and 4 are presented in Fig. 1(b, c). Signs of $\mathrm{s}\mathrm{i}\mathrm{n}{\theta }_0$ and $\mathrm{s}\mathrm{i}\mathrm{n}\left({\theta }_0-\alpha \right)$ for the equilibrium positions investigated change only when crossing the boundaries $\alpha =0$ and $\alpha =\pi /2$ of the domain of parameters, and for each position of equilibrium they are fixed. The second condition in Eq. (5) is satisfied for the second and fourth roots.

Thus, the roots ${\theta }_{02}$ and ${\theta }_{04}$ correspond to points of minimum of the potential energy $\mathrm{\Pi }(\theta ,\psi )$, and that is the sufficient condition for the stationary rotation stability. The root ${\theta }_{01}$ corresponds to the point of maximum, and the necessary condition is defined by the inequality of the form:

The root ${\theta }_{03}$ corresponds to the saddle point of the potential energy and the unstable stationary rotation.

5. Case ${\mathit{a}}_{\mathit{x}\mathit{y}}={\mathit{a}}_{\mathit{x}\mathit{z}}=0$

Let now two of three average values of mixed products of the vibration velocity components are equal to zero. For example, this condition is realized in the case of arbitrary motion (within the assumptions made) of the top suspension point in the vertical plane.

We assume that $a_{xy}=a_{xz}=0$ and, without limiting the generality, that $a_{yz}>0$. Let:

where $\mathrm{}\beta >0$, $\alpha \in \left(0;\pi /2\right)$, $\delta \in \left(0;\pi \right)$.

For this case there exist the top stationary rotations of two types. For the first type, the axis of rotation lies in the plane $OYZ$ (${\psi }_0=$0), and the angle of nutation is the root of Eq. (3). The number and location of roots of this equation are described in section 4.

Fig. 2Bifurcation diagrams

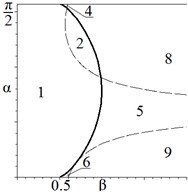
a)

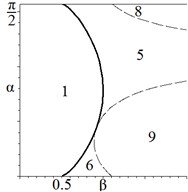
b)

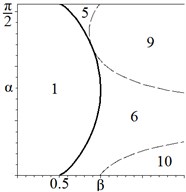
c)

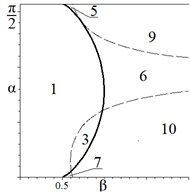
d)

The sufficient conditions for stability are written in form Eq. (5) where $\alpha$ is replaced by $\delta$ in the second inequality. The analysis of the first inequality is fulfilled in section 4. Within the domain considered the left part of the second inequality vanishes if $\mathrm{s}\mathrm{i}\mathrm{n}\left({\theta }_0-\delta \right)=0$, that in the light of Eq. (3) leads to the equation:

Defining the surface in the three-dimensional space of parameters $\alpha$, $\beta$, $\delta$. In the sections $\delta =\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{s}\mathrm{t}$ there are the curves $\beta =\beta \left(\alpha \right)$ shown in Fig. 2 as thin dashed lines. For each fixed value of $\delta$ one of the branches of this curve has a point of tangency with the bifurcation curve Eq. (4) (shown as bold lines), the other branch has two or none points of intersection with the curve Eq. (4). The possible cases of locations of these curves are shown in Fig. 2(a-d) for cases $\delta \in \left(0;\pi /4\right)$ Fig. 2(a), $\delta \in \left(\pi /4;\pi /2\right)$ Fig. 2(b), $\delta \in \left(\pi /2;3\pi /4\right)$ Fig. 2(c) and $\delta \in \left(3\pi /4;\pi \right)$ Fig. 2(d). The curves are divided the domain of the parameters into subdomains marked with numbers 1-10.

The results of the stability investigation of the stationary rotations are presented in Table 1. Symbols “Suff.” and “Nec.” denote that for corresponding domain and root sufficient and only necessary conditions satisfy, to the last one should add restriction Eq. (2) for the angular velocity of the stationary rotation. Symbols “Unst.” denote instability for any angular velocity, and $\cancel{\exists }$ means absence of the root in the domain.

For stationary rotations of the second type the quantities ${\theta }_0$ and ${\psi }_0$ are given by equations:

Defining two positions of the rotation axis being symmetric relative to the plane $OYZ$. These rotations exist if $gA/ml\le \left|a_1^2-a_1{a}_2-a_{yz}^2\right|/\sqrt{a_{yz}^2+{\left(a_1-a_2\right)}^2}$. The analysis based on results of section 2 shows that the sufficient stability conditions has the form $a_1^2-a_1{a}_2-a_{yz}^2>0$, $a_1-a_2>0$, while only necessary conditions are $a_1^2-a_1{a}_2-a_{yz}^2>0$, $a_1-a_2<0$ and Eq. (2).

6. Conclusions

A Lagrange’s top with a suspension point performing fast periodic vibrations in the three-dimensional space was considered. In the framework of the approximate autonomous canonical equations of motion stationary rotations of the top were found for several cases of suspension point motion. Linear and nonlinear analysis of their stability was carried out.