A new secondary instability phenomenon of parametric sloshing

1. Introduction

Parametric sloshing refers to the motion of the liquid free surface due to a periodic excitation perpendicular to the plane of the undisturbed free surface. Faraday [1] first experimentally discovered the parametric sloshing phenomenon in 1831. He found that the parametric instability (or sloshing) started from a small disturbance on the free fluid surface, and then the sloshing amplitude of fluid increased exponentially and finally reached a steady-state limit cycle oscillation. The standing wave oscillates at half the driving frequency, which is called the 1/2 sub-harmonic parametric sloshing. In 1954, Benjamin and Ursell [2] explained the essence of the primary instability with the Mathieu Equation. This parametric instability may be called the first (linear) instability of parametric sloshing, which has been fully studied by many investigators, such as Dodge et al. [3], Woodward and Bauer [4] and Li and Wang [5] etc.

According to the existing research [6] on the parametric oscillation (sloshing), the final response of parametric sloshing to a steady-state excitation is a steady-state oscillation with limited amplitude. However, in this experimental study, the free fluid surface that is in a limit cycle oscillation is observed to undergo a secondary instability. This new instability phenomenon of parametric sloshing is beyond the scope of existing knowledge. In this study, we shall present the experimental phenomenon of secondary instability and the related discussion.

2. Secondary instability phenomenon of parametric sloshing

2.1. Parametric sloshing experiment

In our previous study [5], a two-dimensional parametric sloshing experiment has been conducted for a fluid in the rectangular, circular and U-shaped tanks. The previous experiment was only for the (first) stability analyses of parametric sloshing. In present experimental study, we focus on the stead-state responses of liquid and its secondary instability.

The present test devices, being same as the preceding experiment [5], are illustrated in Fig. 1. The experimental devices consist of three systems, i.e. the excitation system, acceleration acquisition system and wave-height measurement system. A sinusoidal signal is respectively produced and amplified by the signal generator and the power amplifier, and then transmitted to the vibration exciter. The vibration exciter exerts a vertical sinusoidal acceleration on the rectangular tank (contained water). The vertical acceleration signal of the tank is respectively collected, analyzed and recorded by the accelerometer, dynamic signal analyzer, and computer-A. A laser displacement sensor is fixed on the rigid tank to measure the wave height on the liquid free surface. The light signal is captured by the laser displacement sensor and data acquisition instrument, and then analyzed and recorded by signal analyzer and computer-B, respectively.

The test tank (shown in Fig. 2), made of Plexiglas, is constructed as a flat-shape container so as to simulate the two-dimensional sloshing. The inner thickness and width of tank are respectively 20 mm and 200 mm. The water height is 120 mm. The first four theoretical natural frequencies of the contained fluid are $f_1=$1.930 Hz, $f_2=$2.791 Hz, $f_3=$3.420 Hz and $f_4=$ 3.950 Hz, respectively. The measuring coordinate system of wave-height is shown in Fig. 2(b). The water is dyed white so that the liquid free surface can reflect the light that the laser sensor emits.

Fig. 1Experimental diagram

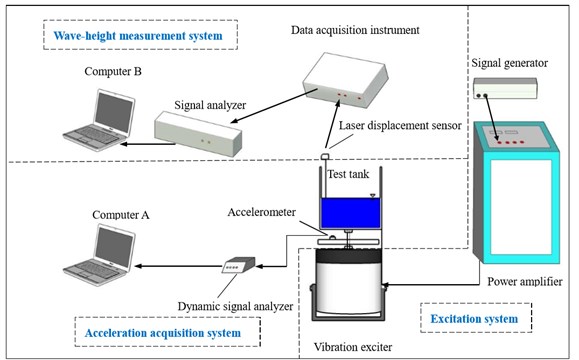
Fig. 2The test tank

a) Photo of tank

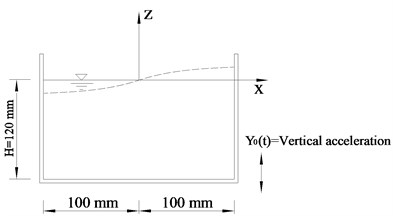
b) Diagram of dimension

2.2. Description of the secondary instability phenomenon

In this parametric sloshing experiment, we incidentally found an amazing secondary instability response. The frequency of signal generator is set as $f=$3.428 Hz. The steady-state time history of the vertical excitation acceleration is recorded and shown in Fig. 3. It is clear that the exciting acceleration, which has been distorted, is not a pure sinusoidal signal. Through a FFT filtering analysis, the acceleration signal $Y_0\left(t\right)$ can be decomposed into the n harmonic components, and represented as the sum of the harmonic components:

1

$Y_0\left(t\right)=Y_1\left(t\right)+Y_2\left(t\right)+Y_3\left(t\right)+Y_4\left(t\right)+...+Y_n\left(t\right),$

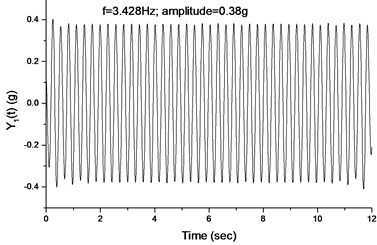
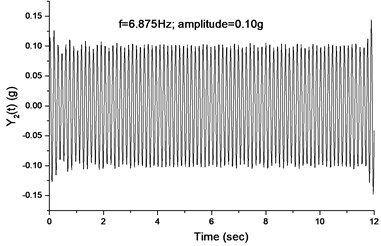
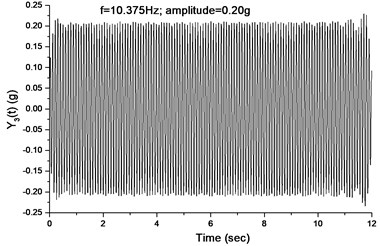
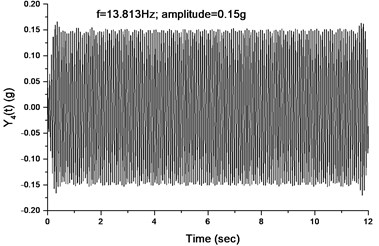
where the harmonic components $Y_1\left(t\right)$, $Y_2\left(t\right)$,..., $Y_n\left(t\right)$ are the stationary sinusoidal waves with the frequencies $f$, $2f$, $3f$, $4f$,…, $nf$. The first four decomposed harmonic components of the excitation are respectively presented in Fig. 4.

Fig. 3A steady-state time history of the vertical excitation acceleration Y0t

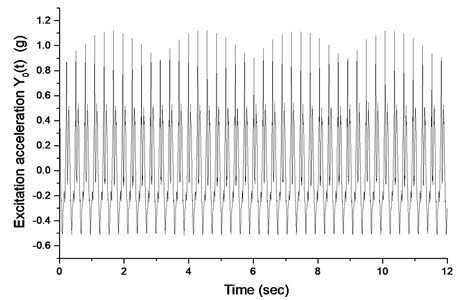
Fig. 4The decomposed harmonic components of the exciting acceleration

a)$Y_1\left(t\right)$

b)$Y_2\left(t\right)$

c)$Y_3\left(t\right)$

d)$Y_4\left(t\right)$

Fig. 5 shows the instability process of wave height at $x=$33.3 mm. The entire response is broadly divided into the four sections. In section (1) (0-15 sec), the first unstable motion is developed in the form of mode III, and the response frequency is 3.453 Hz, which is very close to the third natural frequency. In section (2) (15-45 sec), the free surface is maintained at a steady-state slosh of mode III. In section (3) (45-67.6 sec), the fluid undergoes a secondary instability, in which the 3rd steady-state sloshing mode is transformed into the 1st sloshing mode. The sloshing amplitude of free surface increases greatly, which causes a catastrophic consequence – the fluid is splashed out of the tank. The response frequency of the second instability is 1.844 Hz, which is close to the fundamental frequency $f_1=$1.930 Hz. In section (4) (67.6-100 sec), the free surface shows a free attenuation process after the excitation is stopped. The secondary instability process (from mode III to mode I) of the free surface is displayed in Fig. 6. The experiment has been repeated several times. The similar results have been observed.

Fig. 5The first and second instabilities of wave height

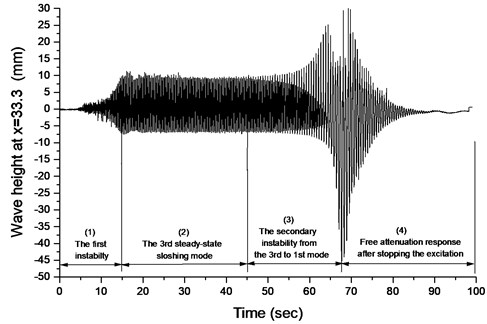
Fig. 6The secondary instability process (from mode III to mode I) of the free surface

3. Discussion on the second instability

It can be seen from Fig. 4 that the excitation frequencies of the first and second harmonic components $Y_1\left(t\right)$ and $Y_2\left(t\right)$ are respectively 3.428 Hz and 6.875 ($\approx$2×3.420) Hz. Because the frequency of the first component is approximately equal to the third natural frequency, i.e., $f\approx f_3$=3.420 Hz, the harmonic response of mode III can be aroused. Meanwhile the frequency of the second component is about double the third natural frequency, i.e., $f\approx 2f_3=$6.840 Hz, the sub-harmonic response of mode III can also be stimulated.

Fig. 7The enlarged diagram of the second instability process

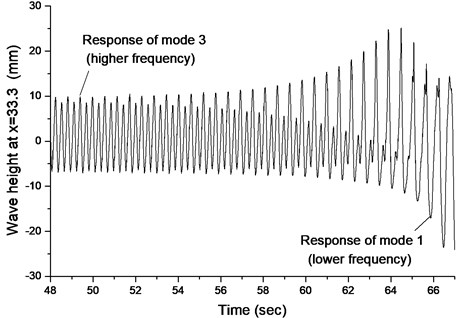
Obviously, the relationship between the first two harmonic excitations is cooperative, instead of competitive. They both have the contributions to the initial unstable motion of mode III. The next liquid motion is a limit cycle motion of mode III. In general, the limit cycle motion is a steady-state oscillation. However, the free fluid surface undergoes a secondary instability in the following parametric sloshing. It can be seen from Fig. 7 that the sloshing energy is transferred from mode III to mode I. It should be noted that the secondary instability is a nonlinear process (vs. the first linear instability) is a newly-discovered unstable phenomenon, whose potential mechanism is a very fascinating problem. We expect the other researchers can give a theoretical explanation.

4. Conclusions

A new secondary instability phenomenon of parametric sloshing was discovered in this experiment. The secondary instability, which is a nonlinear process, is much stronger than the first (linear) instability. The second instability comes with an energy transfer from the higher mode to the lower mode. The mechanism of the secondary instability is needed a further study.