Energy harvesting characteristics of PVDF layers on thin-walled structures with curved surface

1. Introduction

With the development of low-power consumption technique used in microelectronic, wireless-transmission and microelectromechanical systems, the technique of capturing energy from the environment is becoming a research hot spot. Piezoelectric materials, such as PZT and PVDF layers, are good materials in energy harvesting due to their high efficiency in electromechanical conversion, strong anti-interference ability and ease to realize miniaturization. One easy mode to convert mechanical energy into electric energy by PZT or PVDF layers is to install them on the surface of the structures directly, which has little influence on the shape and properties of base structures.

In the past decade, the energy harvesting techniques using PZT or PVDF layers have been investigated extensively [1-3], and the research work can be divided into three aspects as following: (1) Modeling for the energy harvesting structures. (2) Design of the energy harvesting circuits. (3) Design and application of all kind of harvesters. In the modeling of the energy harvesting structures, beam [4-9] or plate [10-12] structures are chosen as the base structures in most research and the characteristics of PZT or PVDF layers on planar beams or plates have been explored comprehensively.

The applications of piezoelectric materials on structures with curved surfaces mostly focused on the actuators and vibration control. In our previous work, the characteristics of laminated PVDF layers and vibration control of cylindrical shells [13, 14], paraboloidal shells [15] and double curved shallow shells [16] have been studied, the theoretical and experimental results indicate that the electromechanical coupling characteristics of PVDF layers employed on curved surfaces are significantly different from that employed on plane surfaces; also the actuation ability of PVDF layers on curved surface is much higher than that on plane surface and the curvature of base structure has significant influence on the actuation property. Moreover, we realized that the characteristics of piezoelectric layers used on plane surfaces structures cannot be applied directly to that used on the curved surface structures. In energy harvesting, the piezoelectric effect is used, although it is not the same as the inverse piezoelectric effect used in actuators, the deformation of the curved structure is coupled inevitably with piezoelectric layers. Whether the PVDF layers used on curved surface possess outstanding ability in energy harvesting as they are used as actuators on curved structures? How do the parameters of curved structures influence the energy harvesting capability of PVDF layers? These are tempting problems to be explored.

To the best knowledge of the author, research work about energy harvesting employing structures with curved surface is still very scarce till now. Yoon [17] et al studied the charge generation and harvesting characteristics of an initially curved PZT unimorph structure. Mossi [18] et al used a thin unimorph prestressed bender to harvest energy and the effect of different parameters on energy harvesting is discussed. Huang [19] et al laminated piezoelectric sensor segments on a circular cylindrical shell and studied the electromechanical coupling effect of the system. In [17-19], all the base structures are curved only in one direction.

In this study, laminated PVDF layers are partially covered on the surface of a double-curved shallow shell to harvest the vibration energy. Analytic expressions of the voltage produced on the PVDF layers are derived. The influence of the radius of curvature on the voltage is discussed. Moreover, the energy harvesting ability of the PVDF layers on the curved shell is compared with that on the planar surface. To verify the theoretical results, energy harvesting experiments are designed and conducted on a straight beam and a curved beam respectively.

2. Mechanical model

2.1. General description of the system

The structure of the harvester is a double curved shallow shell partially covered by several laminated PVDF layers as shown in Fig. 1. The PVDF layers are polarized along the thickness direction and have the same material parameters, which are 1-3 type piezoelectric material. The influence of bonding layers is ignored here in the modeling and the deformation of the shell and PVDF layers are continuous. The vibration of the shell results in deformation of the PVDF layers attached on it, and hence the electrode potential occurs between the electrode pairs due to the piezoelectric effect.

Fig. 1Double-curved shallow shell covered with PVDF layers

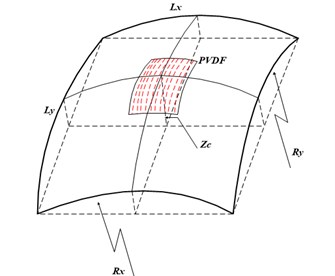
For the shell, it has a rectangle projection on the base plane and its thickness is $h_b$. The maximum rise of the shell is $z_c$. The arc lengths of the middle surface are $l_x$ and $l_y$, respectively. It is assumed that at any point on the mid-surface, the radii of curvature are constant and expressed with $R_x$ and $R_y$, respectively. $u(x,y,t)$, $v(x,y,t)$ and $w(x,y,t)$ are used to express the displacements of the mid-surface in $x$, $y$ and $z$ directions, respectively.

3. Modal voltage and the characteristics of energy harvesting

To probe the energy harvesting performance of the PVDF layers, a simply supported double curved shallow shell is employed and the expression of open circuit voltage is derived under the modal coordinates system. For different bending modes of the shell, the components of the voltage are explored theoretically.

4. Case study

To verify the analysis and results obtained above, open circuit voltages of the PVDF layers covered on the center area of a simply supported double curved shallow shell is calculated for different cases.

The shallow shell has been used in reference [16]. The geometric and material parameters of the shell are listed in Table 1. The geometric and material parameters of the PVDF layers are listed in Table 2. The respective lengths of the PVDF are 1/4 of the lengths of the shell, which means that the area covered by the PVDF layers is only 1/16 of the total area of the base shell.

Case 1: Change the rise of the shell ($z_c$), let the ratio $z_c/h$ increase from 0.001 to 10, then calculate the vibration responses of mode (1, 1) of the shell. The detailed procedure to calculate the system responses is presented in Appendix. Subsequently, strains in the PVDF layer can be obtained, then substituting the strains into Eq. (16), the items of voltages and the total voltage are calculated when only one PVDF layer is adopted and a millimeter displacement is excited at the center of the shell. When $z_c/h=$ 0.001, the shell can be approximated to be a planar plate.

Table 3 shows the magnitudes of the voltages corresponding to the membrane deformation, transverse vibration, bending deformation and the total value expressed in Eq. (15), including the radii of the shell curvature.

Here, it is need to point out that the sign of the $({\tilde{V}}_e{)}_{mem}$ is on the contrary of that before $({\tilde{V}}_e{)}_{R_x{R}_y}$ and $({\tilde{V}}_e{)}_{bend}$ to reflect the phase difference, and the sign before the magnitudes of voltages is included.

Figs. 2-5 show the variations of $({\tilde{V}}_e{)}_{mem}$, $({\tilde{V}}_e{)}_{R_x{R}_y}$, $({\tilde{V}}_e{)}_{bend}$ and $({\tilde{V}}_e{)}_{\mathrm{}}$ with time when $z_c/h=\mathrm{}$0.001, 2, 5 and 10, respectively. Fig. 6 shows the changing regulation of the voltage magnitudes as listed in Table 3.

Fig. 2Variations of (V~e)mem, (V~e)RxRy, (V~e)bend and (V~e) with time when zc/h= 0.001

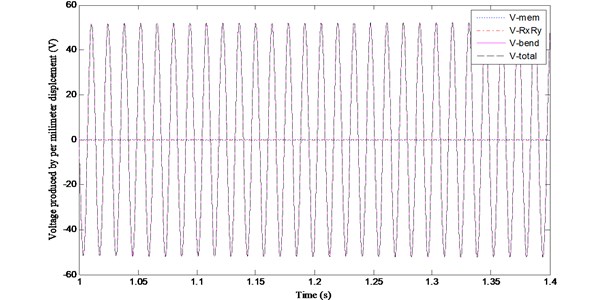
Fig. 3Variations of (V~e)mem, (V~e)RxRy, (V~e)bend and (V~e) with time when zc/h= 2

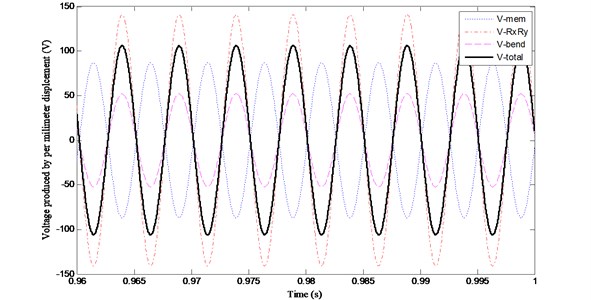
Fig. 4Variations of (V~e)mem, (V~e)RxRy, (V~e)bend and (V~e) with time when zc/h= 5

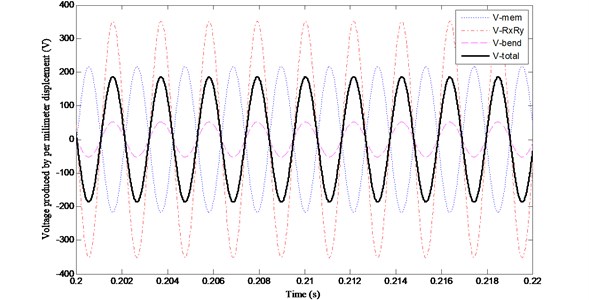
Fig. 5Variations of (V~e)mem, (V~e)RxRy, (V~e)bend and (V~e) with time when zc/h= 10

Fig. 6Magnitudes of the voltages produced by per millimeter displacement of the shell with different rises

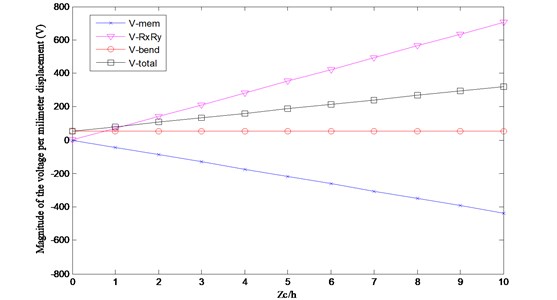
The numerical results indicate that:

(1) With the increasing of the shell’s rise, the radii of the shell decrease significantly, as shown in the 6th and 7th columns in Table 3.

(2) During the vibration of mode (1, 1), $({\tilde{V}}_e{)}_{R_x{R}_y}$ and $({\tilde{V}}_e{)}_{bend}$ have the same phases. The phase of $({\tilde{V}}_e{)}_{mem}$, is different from the phases of $({\tilde{V}}_e{)}_{R_x{R}_y}$ and $({\tilde{V}}_e{)}_{bend}$ and the difference between them is 180°. The phase of the total voltage on the PVDF is the same with that of $({\tilde{V}}_e{)}_{R_x{R}_y}$ and $({\tilde{V}}_e{)}_{bend}$.

(3) For the planar plate ($z_c/h=$ 0.001, as shown in Table 3 and Fig. 2), the magnitudes of $({\tilde{V}}_e{)}_{mem}$, and $({\tilde{V}}_e{)}_{R_x{R}_y}$ are near zero and the total voltage on the PVDF is determined by $({\tilde{V}}_e{)}_{bend}$, which is introduced by the bending deformation of the shell.

(4) For the shell structure, the magnitudes of $({\tilde{V}}_e{)}_{mem}$, and $({\tilde{V}}_e{)}_{R_x{R}_y}$ increase notably with the decreasing of the radii of the shell. But the amplitude of $({\tilde{V}}_e{)}_{bend}$ keeps unchanged for shells with different rises, and the value of $({\tilde{V}}_e{)}_{bend}$ is the same with that produced on the planar plate.

(5) With the decreasing of the radii, the magnitudes of $({\tilde{V}}_e{)}_{mem}$ and $({\tilde{V}}_e{)}_{mem}$, are far greater than that of $({\tilde{V}}_e{)}_{bend}$, and the contribution of $({\tilde{V}}_e{)}_{mem}$ to the total voltage becomes smaller. Namely, $({\tilde{V}}_e{)}_{R_x{R}_y}$ and $({\tilde{V}}_e{)}_{mem}$, play decisive roles to the total voltage in the shell structures, especially the shells with large curvatures. Furthermore, it can be concluded that at the same vibration level, PVDF layers on the shell structures present stronger ability in energy harvesting than that on planar plates.

Case 2: In the simulations above, only one PVDF layer is covered on the center area of the shell. In this case, two PVDF layers are laminated on the center area of the shell. The magnitudes of voltage items and total voltage are calculated when each of the PVDF layer work alone, as well as the PVDF layers work together. Table 4 lists the numerical results when $z_c/h=$ 10.

The results indicate that: (1) The magnitudes of $({\tilde{V}}_e{)}_{mem}$ and $({\tilde{V}}_e{)}_{R_x{R}_y}$ are the same for different PVDF layers, and the magnitude of $({\tilde{V}}_e{)}_{bend}$ increases when the distance between the PVDF layer and the mid-surface of the shell increases. This phenomenon agrees well with the deformation regulation of thin shells. (2) When two PVDF layers work together (connected in series), the magnitudes of the voltage items are the summation of the value produced by each layer working alone, which means that laminated PVDF layers are outstanding in energy harvesting than one PVDF layer.

5. Experimental verification

To validate the analysis and results obtained above, energy harvesting experiments are carried out on a straight beam and a curved beam contrastively. PVDF layers with the same dimensions and parameters are bonded on the beams, respectively.

5.1. Experiment system

The beams and PVDF layers used in the experiments are shown in Fig. 7. If the curved section of the curved beam is unbent, it becomes the same with the straight beam shown in Fig. 7. The geometric and material parameters of the beams and PVDF layers are listed in Table 5 and 6.

For the two beams shown in Fig. 7, contrastive experiments are carried out. In the experiments, there are two different types of external excitation.

5.1.1. Excitation 1: base excitation at tip A

In this case, tip A of the beams is fixed on the vibration exciter and tip B is free. The beams undergo sinusoidal excitation at tip A, as shown in Fig. 8(a), in which the curved beam is viewed for instance. The excitation frequency for different beams is their first natural frequencies, respectively.

Fig. 7Photograph of the beams and PVDF layers

Fig. 8Sinusoidal excitation at the free tip

a) Base excitation

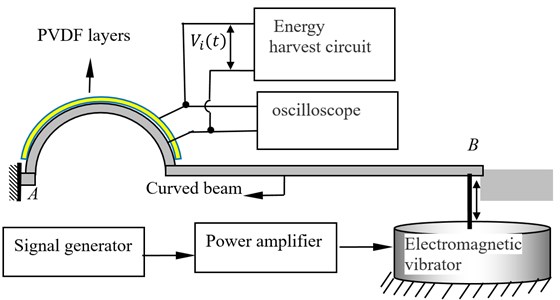
b) Excitation 2

Table 5Geometric and material parameters of the curved beam and straight beam

	Curved beam	Straight beam
Total length of the beam when it spreads(m)	0.3355	0.3355
Width of the beam (m)	0.02	0.02
Thickness of the beam (m)	0.001	0.001
Curvature radius of the curved part (m)	0.025	–
Young’s modulus of the beam (N·m−2)	70×109	70 ×109
Poisson’s ratio of the beam	0.33	0.33
Density of the shell material, ${\rho }_b$(kg·m−3)	2700	2700

Table 6Geometric and material parameters of the PVDF film

Length of the PVDF layer (m)	0.06
Width of the PVDF layer (m)	0.02
Thickness of the PVDF layer (m)	0.0002
Piezoelectric constant of the PVDF, $d_{31}$(pC·N−1)	22-30
Piezoelectric constant of the PVDF, $d_{32}$(pC·N−1)	5-6
Young’s modulus of the PVDF material (N·m−2)	2.1×109

5.1.2. Excitation 2: sinusoidal excitation at the free tip

In this case, tip A of the beams is fixed on the base and tip B of the beams is free as shown in Fig. 8(b). The sinusoidal excitation is applied at the free end of the beams. The excitation frequency equals to the natural frequency of each beam.

During the experiments, the displacements of the excitation points are controlled to be equal for different beams. In the experiments when the base excitation is applied, the displacement amplitudes of tip A of the two beams are equal. For the clamped beams with sinusoidal excitation, the amplitudes of the free ends are controlled to be equal. The experimental setups for different excitations are shown in Fig. (8). The energy harvesting circuit is shown in Fig. 9.

Under the above excitations, the open circuit voltage response on the PVDF layers are recorded. In addition, the voltage on the PVDF is connected to a storage circuit system as shown in Fig. 9 and the DC voltage on the capacitor $C_e$ is tested as well. The capacitance of the capacitor is 1uF.

Fig. 10 shows the photograph of the curved beam excited at the free end.

Fig. 9Energy harvesting circuit

Fig. 10Photograph of the curved beam excited at the free end

5.2. Experimental results

Three groups of experiments are conducted. In group 1, the excitation 1 is adopted and in group 2, the excitation 2 is used. For both groups, only one PVDF layer is partially covered on the straight beam and the curved beam. In group 3, only the curved beam is employed and excitation 1 is considered, but there are two PVDF layers laminated on the curved region. The open circuit voltage on each PVDF layer is tested when the two PVDF layers are in parallel connection and series connection.

5.2.1. Results of group 1

Fig. 11 shows the voltage responses of the PVDF layer on the straight beam when the beam is under the base excitation. The first natural frequency of the beam is 8.75 Hz. The maximum open circuit voltage is 840 mV and the minimum open circuit voltage is –860 mV. Fig. 12 shows the test results of the curved beam. The first natural frequency of the curved beam is 8.25 Hz. The maximum and minimum open circuit voltages are 1400 mV and –1420 mV, respectively. The amplitude of the open circuit voltage introduced by the curved beam is 1.66 times of that introduced by the straight beam, which means that under the same excitation level, the PVDF film on the curved beam presents higher ability in energy harvesting than that on the straight beam.

Fig. 11The voltage responses of the PVDF layer on the straight beam

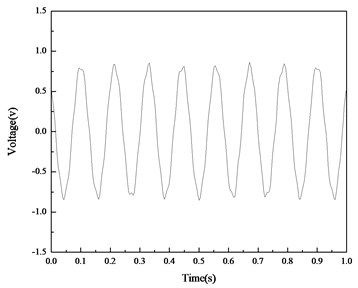
a) Open circuit voltages on the straight beam

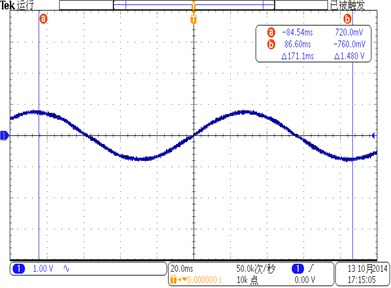
b) Screenshot for the open circuit

Fig. 12The test results of the curved beam

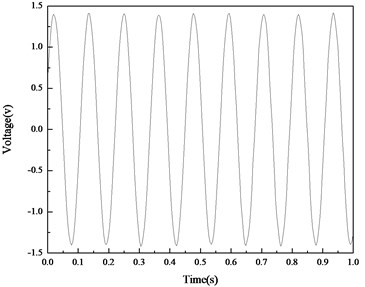
a) Open circuit voltages on the curved beam

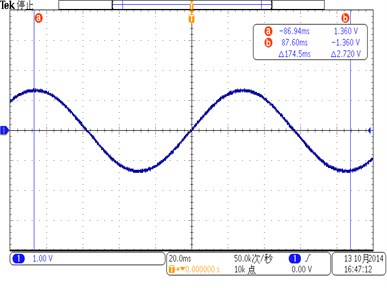
b) Screenshot for the open circuit voltage on the curved beam

Fig. 13 and Fig. 14 show the DC voltage on the capacitor $C_e$ during the energy harvesting for the straight beam and curved beam, respectively. It can be seen that during the capacitor charging process, the slope of the charging curve for the curved beam is much larger than that for the straight beam. According to the test curve, the rate of energy harvesting on the curved beam is about 62.5 mV/s, and that on the straight beam is 25 mV/s. The saturation voltage for the curved beam and straight beam is about 1450 mV and 690 mV, respectively.

The results of this group of experiments show that the energy harvesting ability of the PVDF layer on the curved beam is much higher than that on the straight beam.

Fig. 13DC voltages on the capacitor for the straight beam

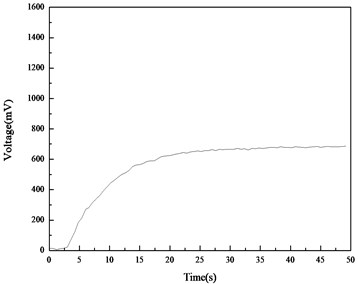
Fig. 14DC voltages on the capacitor for the curved beam

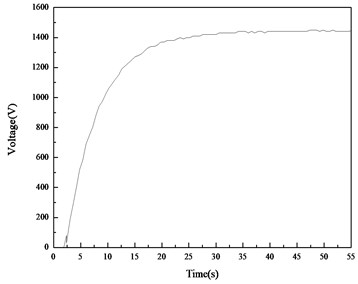
5.2.2. Results of group 2

In this group of experiments, the straight beam and curved beam are clamped at end A respectively and are disturbed at their free end with the same displacements, respectively, as shown in Fig. 8(b). The open circuit voltages on the PVDF layers of the two different beams are tested, together with the DC voltages on the capacitor $C_e$ during the energy harvesting.

Fig. 15Open circuit voltages on the straight beam

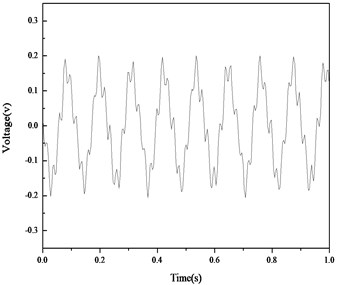
a) Test date

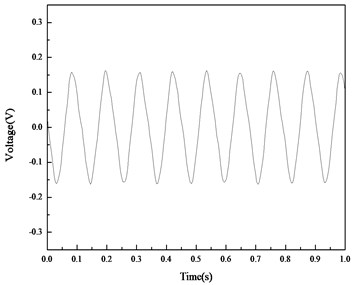
b) Data smoothed

Fig. 16Open circuit voltages on the curved beam

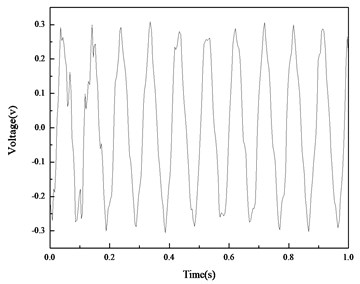
a) Test date

b) Data smoothed

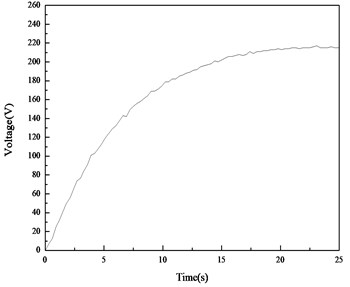
Fig. 15 and 16 show the open circuit voltages. Fig. 17 and 18 give the DC voltages on the capacitor for the two beams. The frequency for the straight beam and curved beam are 8.87 Hz and 10.40 Hz, respectively.

Figs. 14-15 show that under the same disturbance at the free end, the amplitude of open circuit voltage for the straight beam is about 163 mV and that for the curved beam is about 280 mV. The amplitude of the open voltage produced on the curved beam is 1.72 times of that on the straight beam. The results in Fig. 17 and 18 show that the rate of energy harvesting on the curved beam is much higher than that on the straight beam, which is consistent with the conclusion in the experiments of group 1.

Fig. 17DC voltage on the capacitor for the straight beam

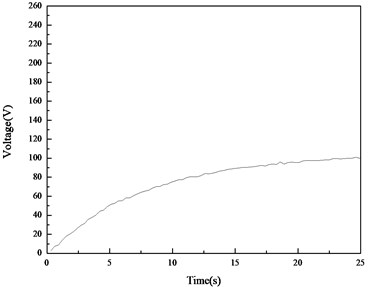
Fig. 18DC voltage on the capacitor for the curved beam

5.2.3. Results of group 3

In the experiments of group 3, only the curved beam is employed to harvest energy and the beam is under the base excitation as shown in Fig. 8(a). There are two PVDF layers laminated on the curved part of the beam. The circuit voltages on the 1st layer (near the shell) and the 2nd layer are tested, including the voltages when the two PVDF layers are in parallel connection and series connection, respectively. It is necessary to point out that for all the tests in group 3, the excitation keeps the same.

Fig. 19 shows the open circuit voltages on the bottom PVDF, and the voltages on the top layer are shown in Fig. 20.

Fig. 19Open circuit voltages on the 1st PVDF layer

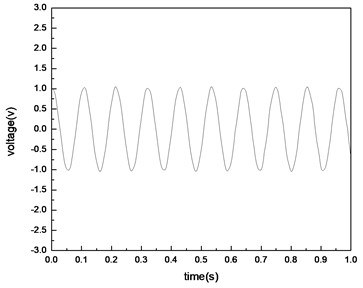
Fig. 20Open circuit voltages on the 2nd PVDF layer

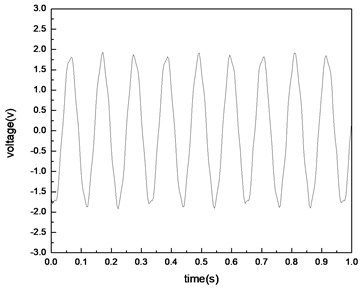
It can be seen that with the same base excitation, the voltage on the top layer is larger than that on the bottom layer, this agrees well with the conclusions obtained from the theory model.

Fig. 21 shows the open circuit voltage responses when the two PVDF layers are connected in series. The voltage responses for the parallel connection are shown in Fig. 22. The schematic diagrams for the series connection and parallel connection are shown in Fig. 23.

Fig. 21Open circuit voltages for series connection

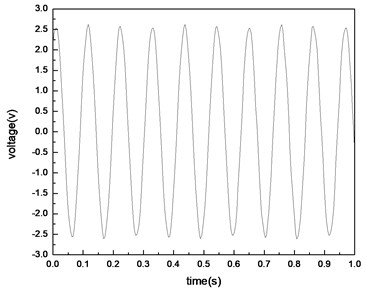
Fig. 22Open circuit voltages for parallel connection

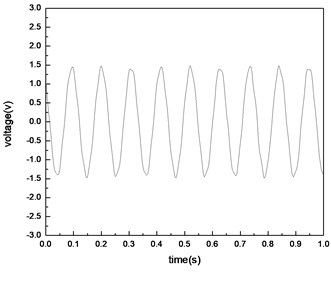
Results in Fig. 21 and 22 show that: (1) The amplitude of the open circuit voltage when the two PVDF layers are in series connection is a little less than the summation of the amplitudes when they work alone, but it is much higher than that when only one PVDF layer is employed. (2) The amplitude of the open circuit voltage when the two PVDF layers are in parallel connection is less than that in series connection and it is even less than the amplitude when the 2nd layer is used alone.

Fig. 23Schematic diagram for circuit

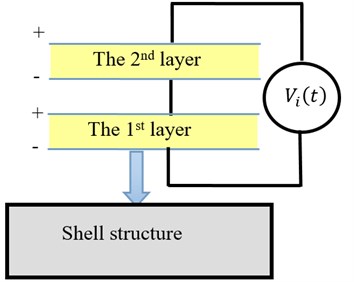
a) In series connection

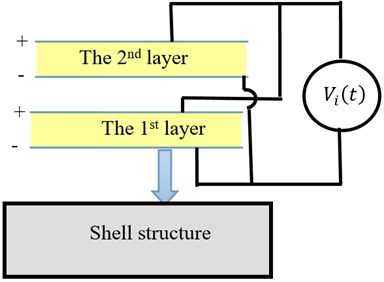
b) In parallel connection

The conclusions obtained from group 3 are consistent with that obtained from the simulations. Moreover, it makes it clear that the connection of several PVDF layers in series mode can improve the energy harvesting efficiency significantly.

6. Conclusions

In this study, energy harvesting characteristics of PVDF layers laminated on double curved shallow shells are investigated numerically and experimentally. Analytical expressions of the open circuit voltages for PVDF layers on double curved shallow shells are derived. Energy harvesting experiments on a straight beam and a curved beam are carried out. Theoretical analysis and experimental results show that:

1) PVDF layers covered on curved shells possess higher energy harvesting ability than that covered on planar plates. The smaller the radii of the shell are; the higher open circuit voltage will be introduced on the PVDF layers.

2) The open circuit voltage introduced by the transverse vibration of the shell plays a decisive role in the total voltage on the PVDF layer. But for a planar plate, this item equals to zero and the total voltage is mainly determined by the bending deformation.

3) When several PVDF layers are laminated on the shell surface and connected in series, the magnitude of the total voltage approximately equals to the summation of the voltages produced by each layer working alone, which means that laminated PVDF layers have advantages over a single PVDF layer in energy harvesting.

4) In this work, the laminated PVDF layers covered on the center area of the base shell and only (1, 1) mode is considered in the analysis. For the vibration of (1, 1) mode, the optimal position for the PVDF layers is the center of the shell, but for the vibrations of other modes, the center position is not the best choice for the PVDF layers. So, the location optimization for the PVDF layers is the subsequent work to be done. Moreover, for a broadband vibration, employing distributed PVDF layers to improve the energy harvesting ability is also to be considered.