Study on an engineering measure to improve internal explosion resistance capacity of segmental tunnel lining structures

2.1. Outlines of the test

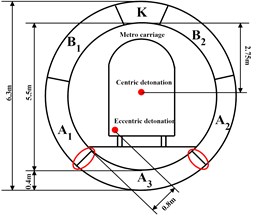
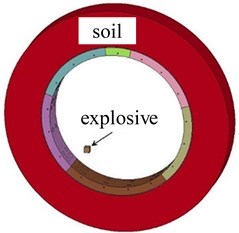
We take the widely acceptable vertical assemblage [19] for shield fabricated linings. Two parallel tunnels were vertically assembled at a testing field (see Fig. 1(a)), each is composed of four staggered rings, and each ring consists of 3 A type segments, 2 B type segments and 1 K segment (see Fig. 1(b)). The ring has a diameter of 6.3 m at extrados and 5.5 m at intrados with a radial thickness of 0.4 m, and its height in tunnel axial direction is 1.2 m. All of the segments are prefabricated by Nanjing Dadi Construction Factory for Nanjing subway engineering.

Given a certain structure, its response to explosion is determined by the type, size, location and ignition method of the charge, as for our problem, these however are determined by the terrorists. According to Federal Emergency Management Agency-Fema2005 [20], for the purpose of protective design, the capacity of a briefcase, a suitcase can be referenced in Table 1. In our tests, the maximum charge weight is set as 20 kg, whereas multiple sources are beyond our scope of study.

Fig. 1Full-scale model test set-up

a) Layout at fields

b) Schematic diagram of internal blast test

c) Position of explosive charge

Central ignition method was adopted and the distance from the charge center to the closest lining interior surface is 0.8 m, as illustrated in Fig. 1. The eccentric location of explosive is determined by the principle of the most adverse condition according to the geometric characteristic of a typical Nanjing metro carriage. The corner of the carriage is closest to lining interior surface, namely the joint contact area of A3 segment and A1 segment (or A2 segment). It is reasonable to understand that lining would behave differently to centric detonation and eccentric one. Some researchers only consider a centric detonation scenario; in that way the loading is underestimated for the near-field structural elements.

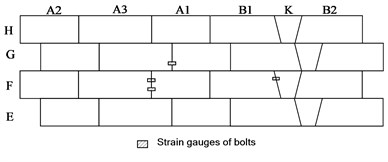
In this paper, the authors mainly focused on the strain of bolts closest and farthest to the explosive. Fig. 2 shows the arrangement of strain gauges.

Fig. 2Arrangement of strain gauges

a) Schematic diagram of layout

b) Strain gauge on bolt

2.2. Test results analysis

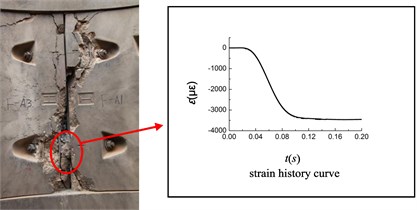
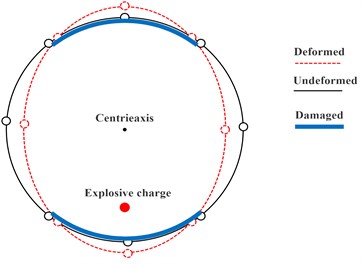
By observing the test results, the basic failure patterns can be outlined. For both centric and eccentric detonations, only localized damages occurred in the joint areas. Especially for the eccentric detonation test, the joint of A3-A1 and the joints along the successive boundaries of the key segments were damaged seriously and presented a crushing destruction, as shown in Fig. 3(a) and Fig. 3(b). Moreover, the bolts deformed so largely that they tended to be straightened and the washers were partly embedded in the concrete. As demonstrated in Fig. 3(d), the damages of other areas focused on the segment joints, mainly presented the crash of concrete outside reinforcement. There were no obvious deformation and damage phenomena in main part of segments and adjacent rings. Fig. 3(c) shows the overall elliptical deformation pattern of the lining ring under the eccentric detonation, this is due to larger loads on near-field and far-field areas. The larger change of curve ratio for near-field area contributes to the damage of the zones (see Fig. 3(b)).

Fig. 3Damage of segments

a) Segment joint of A3-A1 in ring F

b) Segment joint of K-B2 in ring F

c) Elliptical deformation under eccentric detonation

d) Damage in joint areas

The unrecoverable deformation of strain gauges on the circumferential bolts of A1-A3 joint of F ring is shown in Fig. 3(a). The curve in Fig. 3(a) represents the strain history of strain gauge in the middle of circumferential bolt closest to the explosive source, from which we can find that the unrecoverable deformation is 3431 με.

From the test results, characteristics of segmental lining’s dynamic response can be outlined as follows:

1. For both centric and eccentric detonations, the damages localized in the contact areas of joints, meanwhile the ring where explosive charge locates damaged most severely.

2. Stress concentration is produced in the contact area of joints due to the binding effect of circumferential bolts on the outward movement of segment under internal explosive loadings. And stress concentration contributes to failure of concrete and bolts in joint areas.

3. The lining mainly expand outward under the effect of internal explosion, while the deformation in the axial direction is much smaller, which can be supported by the test results that damage on ring joints are minute.

4. Under eccentric detonation, the overall deformation of lining ring is an elliptical pattern with the line connecting, the near-field and far-field as the long axis of the ellipse. So the curve ratio of the intrados of lining increases for the near-field and far-field, which intensify the damage on these areas.

3.1. Basic conceptual ideas

As aforementioned, under internal explosive loading, local binding forces of the bolts are the main reason for stress concentration on joint areas. If the stress concentration can be reduced, then damage in the joint areas should be mitigated, thus improving internal blast-resistance of segmental tunnel linings.

The idea to increase explosion resistance can be preliminarily summarized as follow. On the premise of meeting the requirement of security and stability in daily operation, stress concentration of the contact area of joints under internal explosion could be reduced by certain measures. Under the effect of internal explosive loadings, because of the outward motion of lining segments, the fastening forces on bolts rise. We want it rise below a threshold so that the reinforced concrete in the joint areas will not be damaged, this demands more allowable displacement for the segments bonded together by the jointing bolts. Under the requirements of safe operation and low costs, an effective measure is adopting flexible fastening technology.

The flexible fastening technology of bolts has the characteristics of large deformation, strong energy absorption effects and good deformation compensation ability under stress, so it can withstand the shock loads, vibration loads or thermal loads. The authors propose adding flexible cushion (as shown in Fig. 4) or washer (as shown in Fig. 5) to the joints, thus large deformation is allowed under the dynamic loads and in turn prolong the working time of the impulse, thus damping the amplitude of the loads. The Japan Society of Civil Engineering recommends to apply carbamic acid polyester washer in the jointing bolts to improving seismic resistance performance of segmental lining structures [21], where the mechanism is similar.

Fig. 4Elastic cushion

Fig. 5Carbamic acid polyester flexible cushion

Fig. 6Schematic diagram of joint area closest to explosive

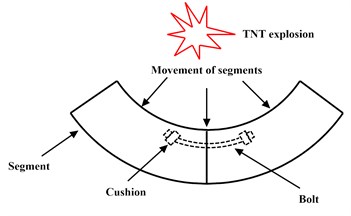
a) Movement

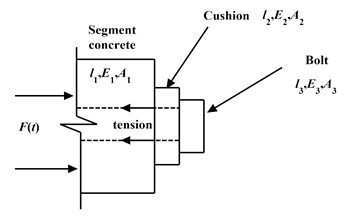
b) Force schematic diagram

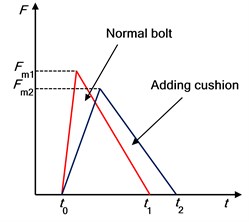
c) Force histories of a normal bolt and one with cushion

Fig. 6 represents the schematic diagram of joint area closest to the explosive. Under internal explosive loading $F\left(t\right)$, the segments will move outwards (see Fig. 6(a)), thus causing the adjoining bolts be stretched, while concrete and cushion compressed (see Fig. 6(b)). With the same impulse that works on the bolt, and with the compressible cushion added, the working time is prolonged, this in turn decreases the magnitude of reaction force on the bolt. The bolt extension can be conceptually expressed as follows:

where $t_0$ and $t_e$ represent the arriving and ending time of shock wave, respectively; $EA$ represents the stiffness, $l$ represents length; $I_m$ is the assumed impulse of the explosive loading that works on this bolt. Once $I_m$ is fixed, $\Delta l$ is determined. If we assume that there is no opening (which we desire for the waterproof of the tunnel) between segments, bolt-cushion-segment, then we will also have:

Since $E_1\gg E_2$(as shown in section 3.2.2), a large proportion of $\Delta l$ will be distributed on the more flexible cushion, which means, the strain of the concrete is drastically relieved.

In other words, after the introduction of flexible cushion, the deformation will be mainly concentrated on the cushion. Thus explosive energy will be more absorbed by the elastic cushion, the stress concentration on segment corners will be relieved, so the resistance performance improved.

3.2. Verification by numerical calculations

In this subsection, the effectiveness of the proposal is verified by numerical simulations, which were performed by nonlinear dynamic FE code of LS-DYNA.

3.2.1. FE model

The tests showed that the damages mainly appear in the ring where the explosive locates. To simplify the calculation, only one ring of segment was taken into account and the effect of longitudinal bolts was ignored. The simplification would increase the internal force and deformation of segments to some extent, so the analysis is conservative.

The simulation took the method of Arbitrary Lagrange Euler to consider the coupling effect between the explosion products, air and the structure. The finite element model is shown in Fig. 7, where the mesh of air is not presented for showing, the air and explosive constituted Euler area, meanwhile the segments, soil, bolts and cushions constituted Lagrange area, air mesh covers the segmental lining. Automatic single face contact method was adopted to model the effect on the interfaces between soil and lining, segments and segments, segments and bolts, etc. The exterior of the soil part is set to be a transmitting boundary to simulate infinite media.

Fig. 7FE model

a) Overall model (air not presented here)

b) Tunnel segments

c) Bolts

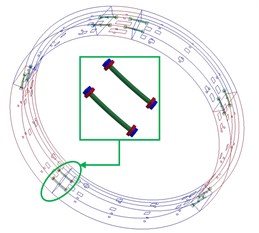
d) Cushions under nuts

3.2.2. Materials model

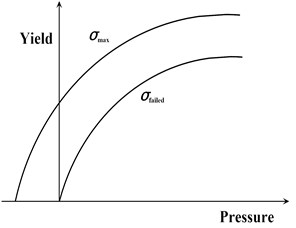
Before applying explosive loadings, the preload of jointing bolts’ fastening force should be applied. In this paper, 3000 N of fastening forces were applied on the bolts by iterative calculation method. The bolts were modeled by the PLASTIC_KINEMATIC model and soil by MORH-COLOMB model. The concrete segments were modeled by PESUDO_TENSOR model [22] (as shown in Fig. 8). The PESUDO_TENSOR model includes two curves respectively, the upper one is the maximum yield curve and the lower one is the failed material curve. ${\sigma }_{\mathrm{m}\mathrm{a}\mathrm{x}}$ and ${\sigma }_{\mathrm{f}\mathrm{a}\mathrm{i}\mathrm{l}\mathrm{e}\mathrm{d}}$ are expressed as Eq. (3) and Eq. (4), where $p$ is pressure, respectively. $a_0$, $a_1$, $a_2$, $a_{0f}$ and $a_{1f}$ are material parameters respectively.

3

${\sigma }_{\mathrm{m}\mathrm{a}\mathrm{x}}=a_0+\frac{p}{a_1+a_{2}p},$

4

${\sigma }_{\mathrm{f}\mathrm{a}\mathrm{i}\mathrm{l}\mathrm{e}\mathrm{d}}=a_{0f}+\frac{p}{a_{1f}+a_{2}p}.$

The material parameters [23] were listed in Table 2, Table 3 and Table 4, where RO, E, PR (or RUN), SIGY, ETAN, BETA, G, GMOD, PHI and CVAL (or A0) represent mass density, Yong modulus, Poisson’s ratio, yield stress, tangent modulus, hardening coefficient, shear modulus, elastic shear modulus, angle of friction and cohesion value, respectively. A1 and A2 are hardening coefficients, A0F and A1F are failure hardening coefficients.

Fig. 8Two-curve model with damage and failure

The equation of state of JWL, which is expressed as Eq. (5) [22] was used to described the detonation products of TNT.

5

$p=A\mathrm{e}\mathrm{x}\mathrm{p}\left(-R_{1}V\right)+B\mathrm{e}\mathrm{x}\mathrm{p}\left(-R_{2}V\right)+\frac{\omega }{V}\left[E-\frac{A}{R_1}\mathrm{e}\mathrm{x}\mathrm{p}\left(-R_{1}V\right)-\frac{B}{R_2}\mathrm{e}\mathrm{x}\mathrm{p}\left(-R_{2}V\right)\right],$

where $V=\frac{{\rho }_0}{\rho }$ is relative volume, $E={\rho }_{0}e$ is detonation energy per unit volume. $A$, $B$, $R_1$, $R_2$ and $\omega$ are coefficients of equation and they are constants in a given explosion simulation.

The parameters of Equation of state for TNT explosive are illustrated in Table 5.

Table 2Materials’ parameters of blots

Material	RO (kg/m3)	E (Pa)	PR	SIGY (Pa)	ETAN (Pa)	BETA
Bolt (M30)	7800	2.1e11	0.238	3e8	2.1e9	0.5

Table 3Materials’ parameters of soil

Material	RO (kg/ m3)	GMOD (Pa)	RUN	PHI	CVAL (Pa)	E
Unsaturated soil	1650	7.4e6	0.3	0.45	e5	3.0e7

Table 4Materials’ parameters of tunnel segments

Material	RO (kg/m3)	G (Pa)	PR	SIGF (Pa)	A0 (Pa)	A1	A2	A0F (Pa)	A1F
Concrete (C50)	2650	2.13e10	0.2	6.44e7	1.35e7	1	0	0	0.385

Table 5Parameters of Equation of state for TNT explosive

Explosive type	A (GPa)	B (GPa)	C (GPa)	$R_1$	$R_2$	$\omega$
TNT	371.2	3.231	1.054	4.15	0.95	0.30

The air was described by the equation of state for ideal gas which is express as Eq. (6) [22]:

6

$p=\left(\mathrm{\gamma }+1\right)\rho e,$

where $p$ is gas pressure (unit: Pa), $\gamma$ is the ratio of specific heats whose value was 1.4 in this paper, $\rho$ is mass density with value of 1.225 kg/m³, $e$ is internal energy per unit volume, here values 4192 MJ/m³.

The main function of cushion (or washer) is to adjust fastening force of joint during the motion process. In this paper, three working conditions were considered during the numerical calculation, as listed in Table 6. As shown in Fig. 7(d), the cushions were placed on the contact area of joints closest to the explosive. The cushions made of carbamic acid polyester were simulated by SOIL_AND_FOAM model. A typical mechanical characteristic curves of cushion were shown in Fig. 9. The material parameters of carbamic acid polyester cushion from the reference [24-26] listed in Table 7 (after conversion) were used in calculations, where RO and G represent mass density and shear modulus, respectively. A0, A1 and A2 are the coefficients of yield function.

Table 6List of working conditions

Number	Working conditions
1	Normal fastening bolts, initial fastening forces were set as 3000 N
2	Adding cushion (thickness 3.8 cm), initial fastening forces were set as 3000 N
3	Removing bolts closest to explosive, namely fastening forces identically equal to 0

Table 7Parameters of cushion model

Material	RO (kg/m3)	G (Pa)	A0 (Pa2)	A1 (Pa)	A2
Carbamic acid polyester	1150	13.8e6	1.21e15	2.018e7	0.084

Fig. 9Typical mechanical properties of cushion [26]

4.1. FEM results analysis

Fig. 10 shows the plastic strain fringe plot in different working conditions and the stripe levels are the same. All the joint areas appear obvious plastic deformation and maxima plastic strains locate in joint areas (shown in Table 8). It can be observed that plastic areas and plastic strain maxima with ordinary bolts are obviously bigger than that of other working conditions. After adding cushions, plastic areas and plastic strain peak values of joint areas decreased. Meanwhile, the plastic strain of bolts far away from the explosive was not obvious. When the two circumferential bolts closest to the explosive source were removed, the plastic area and plastic strain peak values were almost the same with that of adding cushions. The analyses above suggest that appropriate relaxation of fastening condition can greatly reduce the damage of segments.

Fig. 10Plastic strain contours of tunnel segments in different fastening conditions

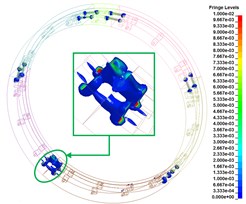
a) Normal fastening bolt

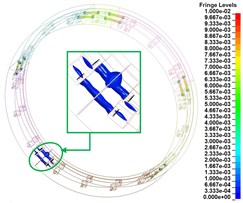
b) Adding cushions

e) Fastening force identically equals to 0 N

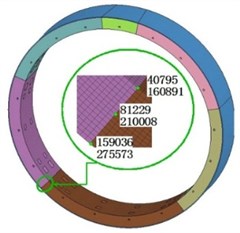
Fig. 11 represents the movement of segments, where the node groups were shown in Fig. 7(b). The history curves of distances between two corresponding nodes were shown in Fig. 11, from which we can find that the opening displacement when adopting ordinary bolts is obvious smaller than other conditions. The former displacement is 3 times of the latter and the trend of history curves is different from each other. In addition, adding cushions or removing the bolts closest to the explosive, relative displacement of joints changed little, what is in accordance with the evolution rules of plastic strain analyzed.

Fig. 11Opening displacement of tunnel segments in different working conditions

a) Node group of 40795-160891

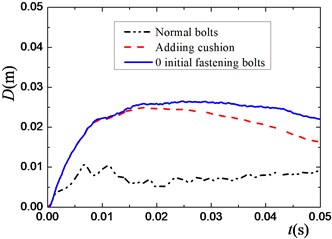
b) Node group of 81229-210008

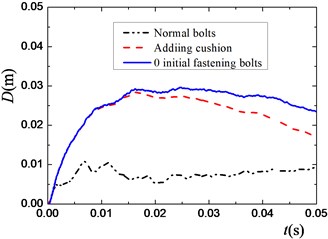
c) Node group of 159036-275573

As shown in Fig. 3(a), the maximum axis strain of circumferential bolts closest to explosion source is 3431 με. The diameter of bolt is 3 cm, so the fastening force corresponding to the strain can be calculated as 490000 N. Fig. 12 represents the fastening force history of bolt, from it, we can find that the maximum fastening force is about 900000 N when adopting normal bolts, which is different from the test data. Analysis shows that the difference is mainly caused by the constraint of surrounding segments in the test. The constraint will decrease the outward movement and deformation of the segments closest to the explosive, thus the destruction of explosion will be decreased in some extent. Moreover, because of the existence of constraint fraction, the fastening force tested from the experiment remained unchanged after the maximum value, as shown in Fig. 3(a). However, the fastening force calculated from the numerical simulation will restore in some extent for not taking the constraint into account. When adding cushions, the maximum value of fastening force is about 200000 N, which is less than the force of normal bolts, and in practical engineering, the value will must be less for the existence of constraints from surrounding segments.

Fig. 12Fastening force history of bolt

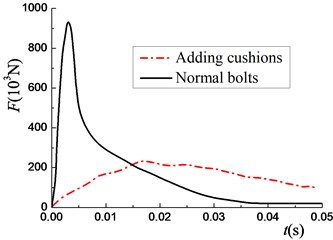
4.2. Engineering application of cushion

Analyses show that the adoption of flexible cushion can reduce the restraint stiffness of joint area of segments, so the destruction of joint area can be greatly decreased under explosion load. In engineering, the design of cushion or washer should meet the following demands:

1) Satisfy the requirement of necessary fastening force in daily operation;

2) The stress relaxation should be small;

3) The system of bolts and cushions can provide the necessary displacement under the explosion load and irreparable damage to the segment structure should not be caused by the enhanced fastening force.

Fig. 13 represents the typical compression curve of cushion, where $S_0$ and $F_0$ represent fastening displacement and fastening force, respectively. $S_m$ and $F_m$ represent maximum compression displacement and corresponding fastening force the cushion can provide, respectively. Calculation shows that the maximum opening displacement of joint is about 3 cm when the fastening force of single circumferential bolt is less than 200000 N, so if the displacement of a single cushion can provide is more than 1.5 cm then the plastic strain of segments will be limited in a small range, and the fastening force during compression progress is less than 200000 N.

Fig. 13Mechanical properties of washer or cushion

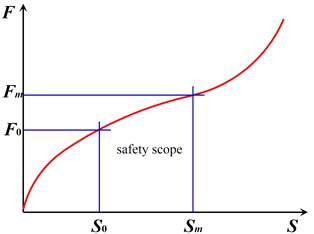
The segmental lining structure studied above was assembled vertically, while in practice projects the structure is assembled horizontally. Luo [27] studied the difference, and showed that the degree of damage for the structure assembled horizontally is relative smaller for the effect of soil constraint when the charge condition is the same, so the authors’ test results are more conservative.