Modeling and simulation of cantilever beam for wind energy harvesting

1. Introduction

In recent years, the increasing production of low-power electronic devices, such as wireless sensors, has been accompanied by increased research about their powering with something different from the traditional batteries, whose recharging or replacement is often expensive or very difficult and sometimes impossible because of their placing. The reduced life of batteries influences and reduces the life of electronic devices when they could still work, having a natural life longer than that of their suppliers [1, 2].

A solution to the described problem has been proposed by Energy Harvesting (EH), defined by Heung Soo et al. as the process that tries to capture “minute amounts of energy from one or more of the surrounding energy sources, accumulating them and storing them for later use” [2].

Energy is wasted everywhere in the environment and according to Sirohi and Mahadik [3] there are several ways to harvest energy, such as the energy harvested from base-excited vibrations, or flow induced vibrations or flutter due to wind flow. This enables vibrations to become an “endless” source of not expensive and non-polluting energy because of the conversion of kinetic energy into electrical one. Piezoelectric materials well fit with this purpose because of their natural property of producing electrical potential when they are mechanically stressed and piezoelectric transducers are more suitable than their electrostatic and electromagnetic counterparts for the conversion kinetic to electric energy because of their higher energy density [4, 5]. Moreover, they have a simple structure and often they have the form of unimorph (with one piezoelectric layer) or bimorph (with two piezoelectric layers) cantilevered beams: the harvester beam is located on a vibrating host structure and the dynamic strain induced in the piezoceramic layers results in an alternating voltage output across their electrodes [6].

Wind is an important renewable energy source, available everywhere in the world and each wind energy harvester can be used and applied universally. EH researchers have been designed different solutions to exploit wind energy, using piezoelectric devices. In 2013, a panel made of wind sensors to convert the wind-induced vibrations into electric energy, called ‘Vibro-Wind’ technology, was proposed and realized by a group of researchers at the Cornell University [7]. Yang et al. presented a rotational piezoelectric energy harvester in which impact-induced resonance is used to enable effective excitation of the intrinsic mode of vibration of piezoelectric cantilevers: this implies an optimum deformation of cantilevers themselves, which is beneficial for the transformation of kinetic into electric energy. The small windmill was realized with piezoelectric bimorph cantilevers locked at one end and placed as a polygon, which has inside some elastic balls. When the wind causes the rotation of device, the balls strike the piezoelectric layers, producing electric potential because of the piezoelectric effect [4]. In the same field of small-scale windmills Rezaei et al. proposed a topology for piezoelectric EH consisting of a lift-based wind turbine and a piezoelectric beam with contactless vibration mechanism. This structure enables to harvest energy from a wide range of wind speeds [8].

The aim of this paper is to validate the modeling and simulation of a wind-stressed piezoelectric cantilever beam, making a comparison between experimental and calculated data. The former ones were detected on a cantilever beam made of Polyvinylidene Fluoride (PVDF) and silver and subjected to wind force; the latter data were obtained from a software simulation of the same device. The validated model can be used to enlarge the number and the range of case studies so enabling the analysis of situations that are difficult to realize experimentally.

In the first section, the paper describes the approach used to validate the simulation environment: the equations and the software used to simulate the behavior of cantilever under wind effect and all elements useful to simulation, such as boundary conditions, are qualified. The section ends with the description of experimental apparatus and the exhibition of numerical results obtained. The last section of paper proposes a comparison between results produced by different materials, belonging to natural crystals, piezo ceramics, piezo polymers and piezocomposite, in order to choose the optimal piezoelectric material for this kind of cantilever. This last study is carried out by characterizing the constitutive matrices for the piezoelectric material in testing and implementing the equations of the mathematical model for each different material.

2. Model validation

2.2. Comsol Multiphysics simulations

Simulations described in this paper have been implemented using Comsol Multiphysics, a general-purpose software platform, based on advanced numerical methods, for modeling and simulating physics-based problems. Comsol Multiphysics reproduced in simulation all situations analyzed with the experimental apparatus, using the previous Eqs. (1), (2), (3) and (4). The cantilever used was built with the same shape, dimensions and materials of that used in experimental apparatus. Fig. 1, realized with Comsol Multiphysics, shows the geometry of cantilever in initial status, without any pressure applied.

Fig. 1The simulated cantilever (Comsol Multiphysics, all dimensions in millimeters)

Fig. 2Boundary conditions

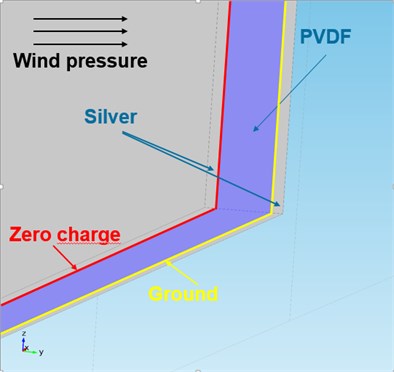
In order to solve Eqs. (1), (2), (3) and (4) and to study piezoelectric materials opportunely, Comsol Multiphysics needs all coefficients of matrices $c^E$, $e$ and ${\epsilon }^S$, as input data. PVDF coefficients were calculated for this work expressly, using an appropriate algorithm. The relations between the coefficients of the four sets of constitutive equations for piezoelectric materials, proposed by IEEE Standard of Piezoelectricity [9], were applied to the coefficients known in literature [10] and the algorithm provided the unknown coefficients. Despite the approximations due to numerical computation, the obtained results had an overall error considerably low and PVDF coefficients used for simulations described in this paper are reported in Eq. (5), (6) and (7), with appropriate units. PVDF belongs to the mm2 crystal class of the orthorhombic system so its constitutive matrices have 17 independent coefficients.

5

$c^E=\left[\begin{array}{cccccc}3.6084e^{-9}& 1.6118e^{-9}& 1.4243e^{-9}& 0& 0& 0\\ 1.6118e^{-9}& 3.1280e^{-9}& 1.3052e^{-9}& 0& 0& 0\\ 1.4243e^{-9}& 1.3052e^{-9}& 1.5889e^{-9}& 0& 0& 0\\ 0& 0& 0& 0.5482e^{-9}& 0& 0\\ 0& 0& 0& 0& 0.5778e^{-9}& 0\\ 0& 0& 0& 0& 0& 0.69e^{-9}\end{array}\right]\text{Pa},$

6

$e=\left[\begin{array}{cccccc}0& 0& 0& 0& -0.0120& 0\\ 0& 0& 0& -0.0121& 0& 0\\ 0.0108& -0.0120& -0.0280& 0& 0& 0\end{array}\right]\text{C}/{\text{m}}^{\text{2}},$

7

${\epsilon }^S=\left[\begin{array}{ccc}7.3217& 0& 0\\ 0& 9.2396& 0\\ 0& 0& 7.9311\end{array}\right].$

Since the analysis involves piezoelectric material, there are two different kinds of boundary conditions to set: mechanical and electrical ones, depicted in Fig. 2. In terms of mechanical conditions, the device is ‘free’ to move under loads caused by wind, except for its lowest surface that is locked. For the electric boundary conditions, the cantilever has at ‘zero charge’ the nearest surface to silver layer where wind pressure is applied and at ‘ground’ the nearest surface to the other silver layer.

2.3. Experimental apparatus

Fig. 3-4 show the cantilever beam used in the experimental apparatus and its pattern. It is a unimorph piezoelectric device because it has only one layer of PVDF put like a sandwich between two layers of silver ink.

Fig. 3Meas LDT1-028k transducer made of PVDF and silver

Fig. 4Pattern of cantilever beam

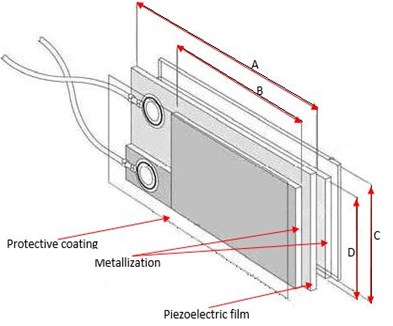
The screen-printed electrodes were supplied with a thin protective coating over the active electrode area to prevent oxidation to the top surface of the silver ink. Its dimensions and properties (Table 1) are provided by its manufacturer [11]. The thickness of protective coating is considered negligible.

Wind bumps the cantilever that reacts deforming itself and generating electrical energy because of the direct piezoelectric effect of PVDF.

Table 1Dimensions and properties of the cantilever beam

	Parameter	Value	Unit
Metallic beam	Short length B	30	mm
	Width	12	mm
	Thickness	6+6	µm
PVDF	Length A	41	mm
	Width	12	mm
	Thickness	28	µm
	Piezo strain constant $d_{31}$	23×10-12	C/N
	Piezo strain constant $d_{33}$	–33×10-12	C/N
	Piezo strain constant $g_{31}$	216×10-3	Vm/N
	Piezo strain constant $g_{33}$	–330×10-3	Vm/N
	Electromechanical coupling factor $k_{31}$	0.12
Plastic coating	Thickness	Negligible

Fig. 5Cantilever on anemometer and wind direction

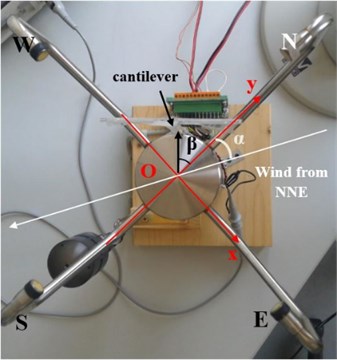
The experimental apparatus was also composed of an ultrasound-based digital anemometer (a Thies Clima’s model US2D, in Fig. 5 as seen from above), an oscilloscope and a pc. A purposely-developed C# .NET application provides velocity and direction of wind impressed on cantilever and, at the same time, it provides the root mean square (RMS) value, the minimum and the maximum value of voltage detected by a digital oscilloscope connected to the cantilever output.

In Fig. 5 angle $\beta =$45° indicates the position of cantilever relative to north.

To convert measured values of wind speed into values of wind pressure useful for simulation, Eqs. (8) and (9) proposed by the IEEE National Electrical Safety Code [12] were used:

8

$v^2$²

9

$v^2$²

where wind pressure is expressed in Pound-force per square foot in Eq. (8) and in Pascal in Eq. (9), while $v$ is the wind velocity expressed in mile per hour in Eq. (8) and in meters per second in Eq. (9).

3. Simulations with different materials

The aim of this section is to find a piezoelectric material with the best electric response for the cantilever analyzed. The validated model was exploited to realize new simulations where the harvester device used had the same shape and dimensions of that used in previous simulation but it was made of three different piezoelectric materials: Quartz (natural crystal), Lead Zirconate Titanate-5H or PZT-5H (piezoceramic) and Cadmium Sulfide or CdS (piezocomposite). All results obtained with new simulations were compared with those obtained with initial cantilever beam made of PVDF that belongs to piezo polymers class. Other parameters of simulation (metallic material used for cantilever beam, pressure applied and boundary conditions) were not changed. Table 3 shows electric values of results obtained.

Fig. 6 shows a plot of comparison among the four RMS absolute values of electric potential developed by the different piezoelectric materials in the situation described above, while the histograms of Fig. 7 show also the minimum and the maximum absolute value of electric potential.

Even if the minimum and maximum value developed of electric potential derives from CdS and Quartz respectively, the comparison of RMS values leads to individuate as the best compromise the polymeric material PVDF for the proposed situation. This is due to the flexibility of this material that induces the cantilever beam to a greater deformation than those realized with the other materials: this implies a higher developed voltage.

Fig. 6Comparison of RMS values of electric potential developed with different piezoelectric materials

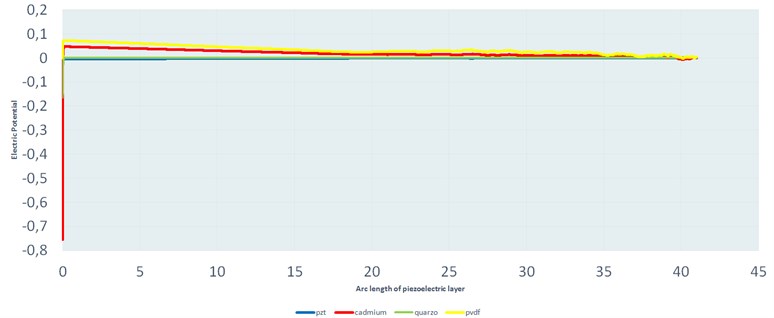
Fig. 7Comparison of electric potential developed with different piezoelectric materials

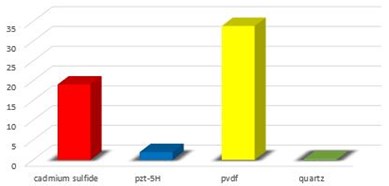
a) RMS (mV)

b)$V_{max}$ (mV)

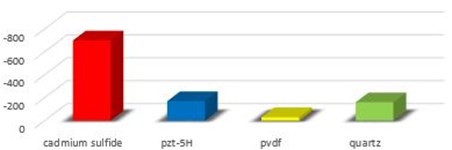
c)$V_{min}$ (mV)

4. Conclusions

In this paper, a study to model and simulate a piezoelectric cantilever beam subjected to wind pressure was described. The study was lead comparing the behavior of a cantilever beam, made of silver and PVDF and subjected to wind force, observed with an experimental apparatus, with that of a FEM model of a device with the same features. The behavior of cantilever was modeled using constitutive equations of piezoelectric materials and mechanical equilibrium equations. The comparison of values of electrical potential developed, because of deformations of beam caused by wind allowed the validation of the simulated device with its geometry, boundaries conditions, constraints and pressure applied. Other simulations were done with different piezoelectric materials to find the best one that allows the cantilever to provide the best values of electrical potential under wind stress, in the fixed analyzed configuration. Comparison leads to individuate as best compromise the polymeric material because of the best RMS value of potential and the less expensive cost.

Ongoing research will investigate a more complex model including laminar flow to simulate wind flow acting on cantilever. Finally, an appropriate electric circuit to store and reuse energy developed by the device described in this paper is going to be realized.