Study on vibration reduction slab track and adjacent transition section in high-speed railway tunnel

1. Introduction

Slab track is a modern railway technology. By using slab track, stability of track can be enhanced, comfort and safety operation of high-speed train can be ensured, and the change rate of track geometry and maintenance work can also be greatly reduced because of the cancellation of the ballast which can cause residual track deformation easily. With the development of slab track technology, slab track has been widely used in high-speed railway in the world, such as German, Japanese and China et al. [1].

Though slab track has many advantages, its stiffness is greater than ballast track, so its influence on environmental vibration is more serious than that of ballast track does in normal conditions. Without vibration reduction measures, it cannot be used directly in some sections of high-speed railway which have special requirements for environmental vibration, for example, the Shiziyang tunnel under the Shiziyang River on Guangzhou-Hong Kong high-speed railway line. The maximum water depth of Shiziyang River is 26.6 m, the maximum water width of Shiziyang River is 3300 m. The foundation of Shiziyang tunnel under the Shiziyang River consists mainly of sand and soft soil, which is very sensitive to environmental vibration.

At present, lots of research work has been done on the dynamic characteristics of slab track of high-speed railway. However, most of these researches are aimed at ordinary slab track structure in normal line [2-9]. Few studies have been made on the dynamic characteristics of vibration reduction slab track structure in vibration reduction section of high-speed railway line, as well as the dynamic characteristics of slab track structure of the transition section between the vibration reduction and normal section of high-speed railway line. Furthermore, former studies mainly concentrated on the vibration characteristic of the coupling system, and few studies considered the dynamic stress of the slab track, which is a key factor for the fatigue failure of slab track. Thirdly, only middle-long random track irregularities, whose wavelength is longer than 1m, is taken into account in the vibration reduction simulation studies, while short-wave random track irregularities, whose wave length is less than 1 m and which has significant influence on the dynamic response of track structure, is not taken into account.

In this paper, based on achievements of the related studies, a high-speed train, slab track and tunnel finite element dynamic model, which takes both short wavelength random track irregularities and middle-long wavelength random track irregularities into account, was established. The corresponding program was developed with MATLAB self-developed program and verified by field test results. With the established dynamic model, taking high-speed trains passing vibration reduction section and adjacent transition section of slab track in Shiziyang tunnel of Guangzhou-Hong Kong line as an example, considering both vibration characteristic of system and dynamic stress of the slab track, the reasonable stiffness value of rubber mat layer under the slab in vibration reduction section of Shiziyang tunnel of Guangzhou-Hong Kong line is studied. By comparing dynamic characteristic of high-speed train-slab track-tunnel system with or without transition section between vibration reduction slab track section and ordinary slab track section, the reasonability of transition section design is verified.

2. Dynamic model

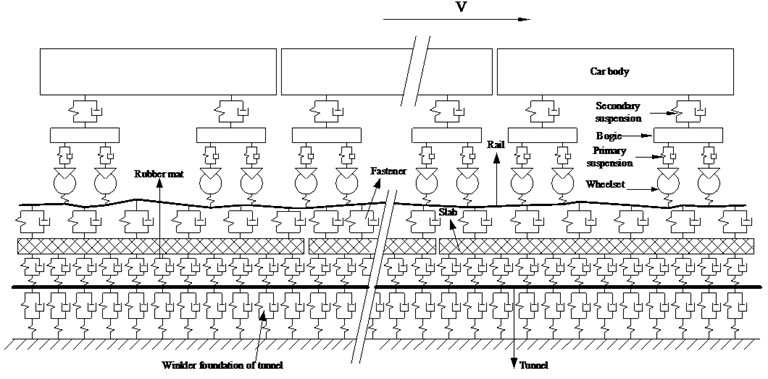
A schematic describing the dynamic model for a high-speed train traveling on a slab track in a tunnel at a constant speed $V$ along the longitudinal direction is shown in Fig. 1.

The model consists of four sub-models, namely, the high-speed train, the slab track-tunnel, wheel-rail interaction, and track irregularity sub-models. The high-speed train sub-model is established based on multi-rigid-body dynamics theory, and the slab track-tunnel sub-model is established based on finite element method. The high-speed train sub-model and slab track-tunnel sub-model are coupled through wheel-rail interaction sub-model considering track irregularities. The four sub-models are explained as follows.

Fig. 1Schematic of the coupling dynamic model

2.2. Slab track-tunnel sub-model

The 3D slab track-tunnel-soil sub-model using volume elements to simulate the slab, tunnel, and surrounding soil can reflect the dynamic characteristics of the slab track-tunnel-soil sub-system. However, the simulation is extremely time-consuming because long model length, small mesh size and small simulation time step are needed in the simulation of a moving high-speed train along the rail with random irregularity of track in this study.

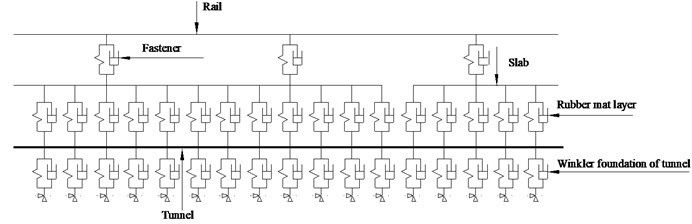
In order to reduce the calculation time, a 2D slab track-tunnel sub-model is used. The sub-model is shown in Fig. 2. The rail, slab, and tunnel are simulated by Bernoulli-Euler beam elements, while fasteners which connect rail and slab, rubber mat layer which connects slab and tunnel, as well as the Winkler foundation of the tunnel are simulated by linear spring-damper elements.

Fig. 22D slab track-tunnel dynamic sub-model

A sophisticated 3D slab track-tunnel-soil model (Fig. 3) is used to determine the equivalent stiffness of the Winkler foundation of the tunnel. In the 3D slab track-tunnel-soil model, the rail is simulated by Bernoulli-Euler beam elements; the slab, rubber mat layer, tunnel, and soil are simulated by volume elements; the fasteners are simulated by linear spring-damper elements.

Fig. 3Sophisticated 3D slab track-tunnel-soil model

a) Cross-section graph

b) Enlarged graph

Static train loads are respectively applied on the 2D slab track-tunnel sub-model shown in Fig. 2 and 3D slab track-tunnel-soil model shown in Fig. 3 to determine the equivalent Winkler foundation stiffness of the tunnel of 2D slab track-tunnel sub-model. If the relative error between the maximum vertical displacement of tunnel in the 2D slab track-tunnel sub-model and that of the 3D slab track-tunnel-soil model is less than 10^-6, the equivalent Winkler foundation stiffness has been found. Otherwise, the equivalent stiffness value should be modified until the above requirement can be met.

By different settings of the stiffness of rubber mat layer between slab and tunnel, the slab track of the vibration reduction section at the normal section and the transition section can all be modeled easily in the 2D slab track-tunnel sub-model shown in Fig. 2.

3. Vibration equations of the coupling dynamic system

Using the principle of total potential energy with the stationary value in elastic system dynamics presented by Zeng [17], one can derive the vibration equation of the high-speed train, slab track and tunnel coupling dynamic model. The equation can be written in matrix form as follows:

where, ${\ddot{X}}_v$, ${\dot{X}}_v$, and $X_v$ denote the acceleration, velocity, and displacement vectors for the DOF of the high-speed train sub-system, respectively; ${\ddot{X}}_t$, ${\dot{X}}_t$, and $X_t$ denote the acceleration, velocity, and displacement vectors for the DOF of the slab track-tunnel sub-system, respectively; $M_{vv}$, $C_{vv}$, and $K_{vv}$ denote the mass, damping, and stiffness matrices of the high-speed train sub-system, respectively; $M_{tt}$, $C_{tt}$, and $K_{tt}$ denote the mass, damping, and stiffness matrices of the slab track-tunnel sub-system, respectively; $F_{vg}$ denotes the gravity sub-load vector of the high-speed train sub-system; $F_{vi}$ and $F_{ti}$ denote the sub-load vector of the wheel-rail interaction forces on high-speed train sub-system and slab track-tunnel sub-system, respectively.

Detailed expressions of the stiffness matrix, mass matrix, damping matrix, and load vector of a single vehicle of the high-speed train sub-model can be founded in reference [10]. Likewise, detailed procedures for obtaining the stiffness matrix, mass matrix, damping matrix, and load vector of the slab track-tunnel sub-system can be found in reference [18]. Solution procedures for the vibration equation of the coupled system are as follows.

1) At time $t=$ 0, the static displacement of the coupled system under the combined effect of gravity load of vehicles and track irregularity is calculated and used as the initial value for the dynamic coupled system.

2) When time $t>$ 0, using the wheel-rail interaction model considering the track irregularity Eq. (1) and an iteration procedure as described in reference [10], the displacement, velocity, and acceleration of each DOF of the coupled system, as well as the wheel-rail interaction forces are calculated. Then, the force of each spring-damping element and the bending moment of each beam element can also be calculated. It should be mentioned that the convergence of the wheel-rail interaction force must be ensured during each time step.

Fig. 4Arrangement of in-situ acceleration sensors

4. Verification of coupling dynamic model

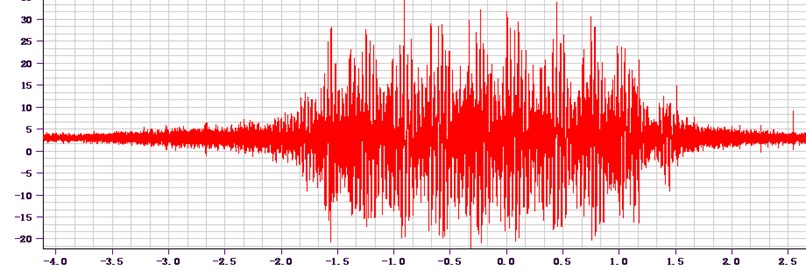
In situ dynamic response measurement was conducted in the vibration reduction slab track section on tunnel of Guangzhou-Hong Kong line. Acceleration sensors were attached to the end side of the slab as shown in Fig. 4. The measurement is used to obtain the accelerations of rail, slab and tunnel when high-speed train runs through the track at speeds from 110 km/h to 300 km/h. The field measured time-history of acceleration of rail, slab, and tunnel are shown in Fig. 5-Fig. 7 when high-speed train runs through the track at the speed of 300 km/h.

Fig. 5Field measured time-history of acceleration of rail

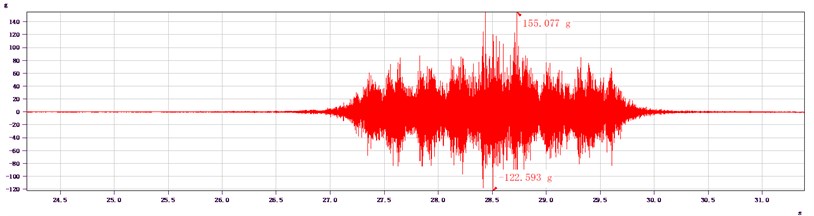
Fig. 6Field measured time-history of acceleration of slab

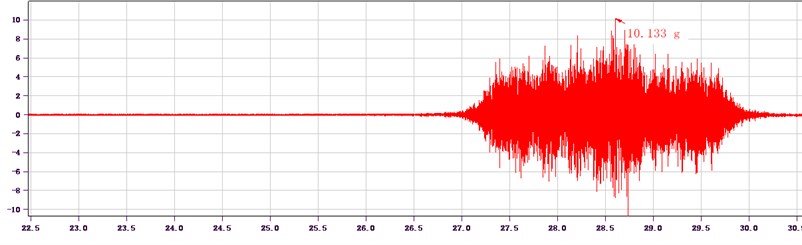
Fig. 7Field measured time-history of acceleration of tunnel

Corresponding numerical simulation was conducted for high-speed train passing vibration reduction section of slab track in Shiziyang tunnel of Guangzhou-Hong Kong high-speed railway line at speed of 300 km/h. The parameters for the high-speed train and those of the slab track-tunnel sub-model are listed in Tables 1 and 2, respectively.

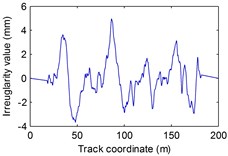
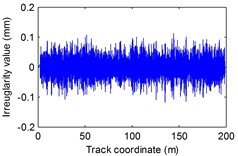
The random track irregularity used in this study is plotted in Fig. 8(a). It is the combination of the sample of middle-long wavelength random track irregularity in Fig. 8(b) and short wavelength random track irregularity in Fig. 8(c).

Time-history curve of maximum acceleration of rail, slab and tunnel calculated by self-developed MATLAB program are shown in Fig. 9-Fig. 11.

Fig. 8a) Samples of the combined random track irregularity, b) middle-long wavelength random track irregularity, c) short wavelength random track irregularity

a)

b)

c)

Comparing Fig. 5-Fig. 7 and Fig. 9-Fig. 11, the maximum acceleration of rail, slab, tunnel calculated by self-compiled MATLAB program are 138.245 g, 11.569 g and 48.5 mg, respectively. And the maximum acceleration of rail, slab, and tunnel measured in situ are 155.077 g, 10.133 g and 35 mg, respectively. The results calculated by the self-compiled MATLAB program are agreed with measured data. Considering that the track irregularities used in this study cannot fully agree with the track irregularities of the field environment and that noise signal has influence on the field test result, the discrepancies between the calculated results and measured data are reasonable, which proves that the dynamics response of train-slab track-tunnels coupling system is correct.

Fig. 9Time-history of maximum acceleration of rail calculated by MATLAB

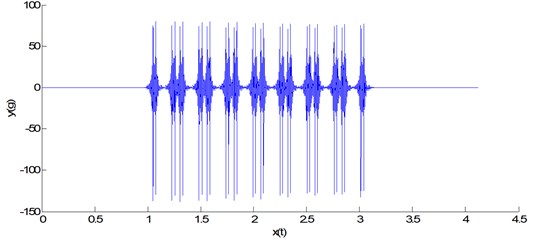
Fig. 10Time-history of maximum acceleration of slab calculated by MATLAB

Fig. 11Time-history of maximum vibration acceleration of tunnel calculated by MATLAB

5. Case studies

With the verified high-speed train-slab track-tunnel finite element coupling dynamic model, taking high-speed train passing vibration reduction slab track with different rubber mat stiffness and ordinary slab track in Shiziyang tunnel at speed of 300 km/h as cases, the dynamic characteristic of high-speed train-slab track-tunnel system for 7 cases was theoretical studied and compared. The stiffness value of rubber mat layer for vibration reduction slab track and the stiffness value of cement asphalt (CA) mortar for ordinary slab track for cases 1-7 are listed in Table 3.

With the verified coupling dynamic model, taking high-speed train passing slab track with different configurations of mat layer at speed of 300 km/h as cases, the dynamic characteristic of high-speed train-slab track-tunnel system with or without transition section between vibration reduction slab track and ordinary slab track are studied and compared. The configurations of length and stiffness of mat layer for cases 8-9 are shown in Table 4.

It should be mentioned that except the stiffness value of rubber mat layer for vibration reduction slab track and the stiffness value of CA mortar for ordinary slab track, which are listed in Table 3-4 for different cases, the other calculation parameters are the same as those in Section 4.

The dynamic response of the car body, bogie, wheel-set, rail, fastening, slab, mat layer, tunnel of the coupling system for Cases 1-9 are listed in Table 5.

From the simulation of the case study, the following points can be obtained:

1) The vibration acceleration of tunnel can be reduced greatly with rubber mat layer used under the slab. The lower the stiffness of rubber mat layer is, the smaller the vibration acceleration of tunnel will be.

2) The rail vertical displacement and the slab bending moment increases significantly with rubber mat layer used under the slab. The lower the stiffness of rubber mat layer is, the larger the rail vertical displacement and the slab bending moment will be.

3) According to the relevant codes and regulations, the vertical displacement of rail of high-speed railway above 300 km/h should not be more than 2 mm. Considering the limited value of rail vertical displacement, the stiffness of rubber mat layer of vibration reduction section of Shiziyang tunnel of Guangzhou-Shenzhen-Hong Kong line should not be smaller than 0.04 N/mm³.

4) Comparing with the ordinary slab track, the acceleration of tunnel decreased 16 dB by adopting vibration reduction slab track with 0.04 N/mm³ stiffness of rubber mat layer. The vibration reduction effect of rubber mat layer is excellent.

5) The bending moment of slab increases substantially with rubber mat layer used under the slab, so the strength of slab should be carefully checked to avoid fatigue failure of slab.

6) Without transition section between vibration reduction slab track and ordinary slab track, the pressure force of rubber mat layer, bending moment of slab, pressure and tension force of fastener, and acceleration of slab and tunnel increase substantially. With transition section between vibration reduction slab track and ordinary slab track, dynamic characteristic of slab track and tunnel are excellent, design of transition section between vibration reduction slab track and ordinary slab track in tunnel of Guangzhou-Hong Kong line is reasonable.

6. Conclusions

The high-speed train-slab track-tunnel finite element coupling dynamic model was established, and corresponding program was developed with MATLAB program language and verified by in situ measured data. Taking high-speed train passing slab track of vibration reduction section and adjacent transition section on tunnel of Guangzhou-Hong Kong line as an example, dynamic characteristics of high-speed train, slab track and tunnel system was theoretical studied and compared. The main conclusions include:

1) The lower the stiffness of rubber mat layer is, the larger the vertical displacement of rail and the bending moment of slab will be. The stiffness of rubber mat layer of slab track in tunnel of Guangzhou-Hong Kong line is controlled by the rail vertical displacement, and the 0.04 N/mm³ stiffness value for rubber mat layer is reasonable. By using rubber mat layer under the slab, vibration of tunnel can decrease 16 dB, and the effect of vibration reduction of rubber mat layer is excellent.

2) With transition section between vibration reduction slab track and ordinary slab track, vibration and dynamic stress of slab track are excellent. The design of transition section between vibration reduction slab track and ordinary slab track in tunnel of Guangzhou-Hong Kong line is reasonable.